Skip to main content
SpringerLink
Log in

Analysis of local discontinuous Galerkin method for time–space fractional convection–diffusion equations

  • Published:
BIT Numerical Mathematics Aims and scope Submit manuscript

Abstract

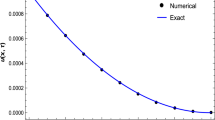
This paper focuses on the time–space fractional convection–diffusion equations with time fractional derivative (of order \(\alpha \), \(0< \alpha <1\)) and space fractional derivative (of order \(\beta \), \(1<\beta <2\)). An approach based on a combination of local discontinuous Galerkin (in space) and finite difference methods (in time) is presented to solve the fractional convection–diffusion equations. The stability and convergence analysis of the method are given. Numerical results confirm the theory of the method for fractional convection–diffusion equations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Bu, W.P., Tang, Y.F., Yang, J.Y.: Galerkin finite element method for two-dimensional Riesz space fractional diffusion equations. J. Comput. Phys. 276, 26–38 (2014)

    Article  MathSciNet  Google Scholar 

  2. Chen, F., Xu, Q., Hesthaven, J.S.: A multi-domain spectral method for time-fractional differential equations. J. Comput. Phys. 293, 157–172 (2015)

    Article  MathSciNet  Google Scholar 

  3. Deng, W.H.: Finite element method for the space and time fractional Fokker–Planck equation. SIAM J. Numer. Anal. 47(1), 204–226 (2008)

    Article  MathSciNet  Google Scholar 

  4. Ford, N.J., Xiao, J.Y., Yan, Y.B.: A finite element method for time fractional partial differential equations. Fract. Calc. Appl. Anal. 14(3), 454–474 (2011)

    Article  MathSciNet  Google Scholar 

  5. Jiang, Y.J., Ma, J.T.: High-order finite element methods for time-fractional partial differential equations. J. Comput. Appl. Math. 235, 3285–3290 (2011)

    Article  MathSciNet  Google Scholar 

  6. Li, J.C., Huang, Y.Q., Lin, Y.P.: Developing finite element methods for Maxwells equations in a Cole–Cole dispersive medium. SIAM J. Sci. Comput. 33, 3153–3174 (2011)

    Article  MathSciNet  Google Scholar 

  7. Li, C.P., Zhao, Z.G., Chen, Y.Q.: Numerical approximation of nonlinear fractional differential equations with subdiffusion and superdiffusion. Comput. Math. Appl. 62, 855–875 (2011)

    Article  MathSciNet  Google Scholar 

  8. Liu, Y., Du, Y.W., Li, H., Li, J.C., He, S.: A two-grid mixed finite element method for a nonlinear fourth-order reaction diffusion problem with time-fractional derivative. Comput. Math. Appl. 70(10), 2474–2492 (2015)

    Article  MathSciNet  Google Scholar 

  9. Liu, Y., Du, Y.W., Li, H., He, S., Gao, W.: Finite difference/finite element method for a nonlinear time-fractional fourth-order reaction diffusion problem. Comput. Math. Appl. 70, 573–591 (2015)

    Article  MathSciNet  Google Scholar 

  10. Liu, Y., Fang, Z.C., Li, H., He, S.: A mixed finite element method for a time-fractional fourth-order partial differential equation. Appl. Math. Comput. 243, 703–717 (2014)

    MathSciNet  MATH  Google Scholar 

  11. Wang, Y.J., Liu, Y., Li, H., Wang, J.F.: Finite element method combined with second-order time discrete scheme for nonlinear fractional Cable equation. Eur. Phys. J. Plus 131(3), 1–16 (2016)

    Article  Google Scholar 

  12. Xu, Q., Hesthaven, J.S.: Stable multi-domain spectral penalty methods for fractional partial differential equations. J. Comput. Phys. 257, 241–258 (2014)

    Article  MathSciNet  Google Scholar 

  13. Ding, H., Li, C.: High-order compact difference schemes for the modified anomalous subdiffusion equation. Numer. Methods Partial Differ. Equ. 32(1), 213–242 (2016)

    Article  MathSciNet  Google Scholar 

  14. Ji, C.C., Sun, Z.Z.: A high-order compact finite difference scheme for the fractional sub-diffusion equation. J. Sci. Comput. 64(3), 959–985 (2015)

    Article  MathSciNet  Google Scholar 

  15. Liao, H.L., Zhang, Y.N., Zhao, Y., Shi, H.S.: Stability and convergence of modified Du Fort–Frankel schemes for solving time-fractional subdiffusion equations. J. Sci. Comput. 61(3), 629–648 (2014)

    Article  MathSciNet  Google Scholar 

  16. Lin, R., Liu, F., Anh, V., Turner, I.: Stability and convergence of a new explicit finite-difference approximation for the variable-order nonlinear fractional diffusion equation. Appl. Math. Comput. 212, 435–445 (2009)

    MathSciNet  MATH  Google Scholar 

  17. Lin, Y.M., Xu, C.J.: Finite difference/spectral approximations for the time-fractional diffusion equation. J. Comput. Phys. 225, 1533–1552 (2007)

    Article  MathSciNet  Google Scholar 

  18. Meerschaert, M.M., Tadjeran, C.: Finite difference approximations for fractional advection dispersion flow equations. J. Comput. Appl. Math. 172(1), 65–77 (2004)

    Article  MathSciNet  Google Scholar 

  19. Shen, S., Liu, F., Anh, V., Turner, I., Chen, J.: A characteristic difference method for the variable-order fractional advection–diffusion equation. J. Appl. Math. Comput. 42, 371–386 (2013)

    Article  MathSciNet  Google Scholar 

  20. Sousa, E.: Finite difference approximations for a fractional advection–diffusion problem. J. Comput. Phys. 228, 4038–4054 (2009)

    Article  MathSciNet  Google Scholar 

  21. Zhuang, P., Liu, F., Anh, V., Turner, I.: Numerical methods for the variable-order fractional advection diffusion equation with a nonlinear source term. SIAM J. Numer. Anal. 47, 1760–1781 (2009)

    Article  MathSciNet  Google Scholar 

  22. Zhang, H., Liu, F., Phanikumar, M.S., Meerschaert, M.M.: A novel numerical method for the time variable fractional order mobile–immobile advection–dispersion model. Comput. Math. Appl. 66, 693–701 (2013)

    Article  MathSciNet  Google Scholar 

  23. Wang, P.D., Huang, C.M.: An energy conservative difference scheme for the nonlinear fractional Schrdinger equations. J. Comput. Phys. 293, 238–251 (2015)

    Article  MathSciNet  Google Scholar 

  24. Wang, K.X., Wang, H.: A fast characteristic finite difference method for fractional advection–diffusion equations. Adv. Water Resour. 34(7), 810–816 (2011)

    Article  Google Scholar 

  25. Yang, Q., Turner, I., Liu, F., et al.: Novel numerical methods for solving the time–space fractional diffusion equation in two dimensions. SIAM J. Sci. Comput. 33(3), 1159–1180 (2011)

    Article  MathSciNet  Google Scholar 

  26. Yuste, S.B., Quintana-Murillo, J.: A finite difference method with non-uniform time steps for fractional diffusion equations. Comput. Phys. Commun. 183(12), 2594–2600 (2012)

    Article  MathSciNet  Google Scholar 

  27. Deng, W.H., Hesthaven, J.S.: Local discontinous Galerkin methods for fractional diffusion equation. ESAIM 47, 1845–1864 (2013)

    Article  Google Scholar 

  28. Deng, W.H., Hesthaven, J.S.: Local discontinuous Galerkin methods for fractional ordinary differential equations. BIT Numer. Math. 55, 967–985 (2015)

    Article  MathSciNet  Google Scholar 

  29. Mustapha, K., Abdallah, B., Furati, K.M.: A discontinuous petrov–Galerkin method for time-fractinal diffusion equations. SIAM J. Numer. Anal. 52(5), 2512–2529 (2014)

    Article  MathSciNet  Google Scholar 

  30. Mustapha, K., Abdallah, B., Furati, K.M., Nour, M.: A discontinuous Galerkin method for time fractional diffusion equations with variable coefficients. Numer. Algorithm 73, 517–534 (2016)

    Article  MathSciNet  Google Scholar 

  31. Mustapha, K., Nour, M., Cockburn, B.: Convergence and superconvergence analyses of HDG methods for time fractional diffusion problems. Adv. Comput. Math. 42, 377–393 (2016)

    Article  MathSciNet  Google Scholar 

  32. Wei, L., He, Y.: Analysis of a fully discrete local discontinuous Galerkin method for time-fractional fourth-order problems. Appl. Math. Model. 38, 1511–1522 (2014)

    Article  MathSciNet  Google Scholar 

  33. Wei, L., He, Y., Tang, B.: Analysis of the fractional Kawahara equation using an implicit fully discrete local discontinuous Galerkin method. Numer. Methods Partial Differ. Equ. 29, 1441–1458 (2013)

    Article  MathSciNet  Google Scholar 

  34. Wei, L., He, Y., Zhang, X., Wang, S.: Analysis of an implicit fully discrete local discontinuous Galerkin method for the time-fractional Schrodinger equation. Finite Elem. Anal. Des. 59, 28–34 (2012)

    Article  MathSciNet  Google Scholar 

  35. Wei, L., Zhang, X., He, Y.: Analysis of a local discontinuous Galerkin method for time-fractional advection–diffusion equations. Int. J. Numer. Methods Heat Fluid Flow 23(4), 634–648 (2013)

    Article  MathSciNet  Google Scholar 

  36. Wei, L., Zhang, X., Kumar, S., Yildirim, A.: A numerical study based on an implicit fully discrete local discontinuous Galerkin method for the time-fractional coupled Schrdinger system. Comput. Math. Appl. 64, 2603–2615 (2012)

    Article  MathSciNet  Google Scholar 

  37. Wang, J., Liu, T., Li, H., Liu, Y., He, S.: Second-order approximation scheme combined with H1-Galerkin MFE method for nonlinear time fractional convection–diffusion equation. Comput. Math. Appl. 73, 1182–1196 (2017)

    Article  MathSciNet  Google Scholar 

  38. Bhrawy, A.H., Zaky, M.A., Mahmoud, A.M., Tenreiro Machado, J.A.: Numerical solution of the two-sided space–time fractional telegraph. J. Optim. Theory Appl. 174, 321–341 (2017)

    Article  MathSciNet  Google Scholar 

  39. Bhrawy, A.H., Zaky, M.A.: A method based on the Jacobi tau approximation for solving multi-term time–space fractional partial differential equations. J. Comput. Phys. 281, 876–895 (2015)

    Article  MathSciNet  Google Scholar 

  40. Zayernouri, M., Karniadakis, G.E.: Fractional spectral collocation method. SIAM J. Sci. Comput. 36, 40–62 (2014)

    Article  MathSciNet  Google Scholar 

  41. Zayernouri, M., Ainsworth, M., Karniadakis, G.E.: A unified petrov–Galerkin spectral method for fractional PDEs. Comput. Methods Appl. Mech. Eng. 283, 1545–1569 (2015)

    Article  MathSciNet  Google Scholar 

  42. Liu, F., Zhuang, P., Anh, V., Turner, I., Burrage, K.: Stability and convergence of the difference methods for the space–time fractional advection–diffusion equation. Appl. Math. Comput. 191, 12–20 (2007)

    MathSciNet  MATH  Google Scholar 

  43. Castillo, P., Cockburn, B., Schotzu, D., Schwab, C.: Optimal a priori error estimates for the hp-Version of the local discontinuous Galerkin method for convection–diffusion problems. Math. Comput. 71(238), 455–478 (2001)

    Article  MathSciNet  Google Scholar 

  44. Zayernouri, M., Karniadakis, G.E.: Discontinuous spectral element methods for time and space-fractional advection equations. SIAM J. Sci. Comput. 36, 684–707 (2014)

    Article  MathSciNet  Google Scholar 

  45. Xu, Q., Hesthaven, J.S.: Discontinuous Galerkin method for fractional convection–diffusion equations. SIAM J. Numer. Anal. 52(1), 405–423 (2014)

    Article  MathSciNet  Google Scholar 

  46. Cockburn, B., Mustapha, K.: A hybridizable discontinuous Galerkin method for fractional diffusion problems. Numer. Math. 130, 293–314 (2015)

    Article  MathSciNet  Google Scholar 

  47. Kilbas, A.A., Srivastava, H., Trujillo, J.: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam (2006)

    MATH  Google Scholar 

  48. Ervin, V.J., Roop, J.P.: Variational formulation for the stationary fractional advection dispersion equation. Numer. Methods Partial Differ. Equ. 22, 558–576 (2005)

    Article  MathSciNet  Google Scholar 

  49. Cockburn, B., Kanschat, G., Perugia, I., Schotzau, D.: Superconvergence of the local discontinuous Galerkin method for elliptic problems on Cartesian grids. SIAM J. Numer. Anal. 39, 264–285 (2001)

    Article  MathSciNet  Google Scholar 

  50. Schotzau, D., Schwab, C.: An hp a priori error analysis of the DG time-stepping method for initial value problems. Calcolo 37, 207–232 (2000)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

We would like to thank anonymous referees for carefully reading the manuscript and for their valuable comments and suggestions, which helped us to considerably improve the manuscript.

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, University of Qom, Isfahan Old Road, Qom, Iran

    M. Ahmadinia & Z. Safari

  2. Department of Mathematics, University of Shahrekord, Shahrekord, Iran

    S. Fouladi

Authors
  1. M. Ahmadinia
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Z. Safari
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. S. Fouladi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. Ahmadinia.

Additional information

Communicated by Jan Hesthaven.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ahmadinia, M., Safari, Z. & Fouladi, S. Analysis of local discontinuous Galerkin method for time–space fractional convection–diffusion equations. Bit Numer Math 58, 533–554 (2018). https://doi.org/10.1007/s10543-018-0697-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10543-018-0697-x

Keywords

Mathematics Subject Classification

Search

Navigation