Influence of combined electromagnetohydrodynamics on microchannel flow with electrokinetic effect and interfacial slip

Abstract

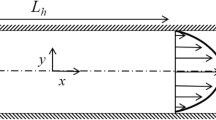
We investigate analytically the combined consequences of electromagnetohydrodynamic forces and interfacial slip on streaming potential mediated pressure-driven flow in a microchannel. Going beyond traditional Debye–Hückel limit, we first derive a closed-form analytical solution for velocity field by considering nonlinear electrical potential distribution, wall slip effects, externally imposed transverse magnetic field, and laterally applied electric field in the plane of flow. The effects of electrical double-layer (EDL) formation and the consequent interfacial phenomena are critically examined under such situations. An expression for induced streaming potential in the microchannel is deduced considering EDL formation and the consequences of finite conductance of the immobilized Stern layer. This simplified analytical expression is later on critically assessed against three-dimensional simulation paradigm of streaming potential mediated flows, which is a first effort of this kind. We demonstrate that flow rate increases progressively with increasing surface potential and eventually approaches to a limiting value. Combination of electromagnetohydrodynamic effect with liquid slip is shown to amplify the flow rate, even at lower values of surface potential. Our study brings out the possibility of achieving an optimum flow rate by judicious application of combined electromagnetohydrodynamics. The present analysis has significant consequence in the design of advanced microfluidic devices with improved efficiency and functionality.

Appendix: Solution of Eq. (11)

Equation 11 is a second-order non-homogeneous differential equation with constant coefficients. The general solution of Eq. (11) takes the following form,

$$u\left( y \right) = u_{aux} + u_{PI}$$

(29)

where u _aux is the solution of the auxiliary homogeneous part of the equation and u _PI is the particular integral. To find u _aux, we need to solve the homogeneous auxiliary equation of Eq. (11) in the form,

$$m^{2} - Ha^{2} = 0$$

(30)

where u _aux(y) = e ^my, (m being a constant) is an arbitrary solution of the homogeneous auxiliary equation.This finally gives the solution of the auxiliary equation as,

$$u_{\text{aux}} = C_{1} \exp \left( {Ha\,y} \right) + C_{2} \exp \left( { - Ha\,y} \right)$$

(31)

where C ₁ and C ₂ are the constants. We decompose the particular integral by the method of superposition as,

$$u_{PI} = u_{PI1} + u_{PI2}$$

(32)

The first part of the particular integral u _PI1 is evaluated as,

$$u_{PI1} = \frac{1}{{D^{2} - Ha^{2} }}\left\{ { - \left( {1 + Ha^{2} S} \right)} \right\} = \frac{{1 + Ha^{2} S}}{{Ha^{2} }}$$

(33)

For the second part of the particular integral u _PI2, we use the variation of parameter method. Accordingly, we may write u _PI2 in the form of

$$u_{PI2} = a_{1} u_{1} + a_{2} u_{2}$$

(34)

where u ₁ = exp (Hay), u ₂ = exp (−Hay).

Therefore, u _PI2 = a ₁ exp (Hay) + a ₂ exp (−Hay). The unknown parameters a ₁ and a ₂ are calculated as

$$a_{1} = \int {\frac{ - F}{W}} \,u_{2} \,\text{d}y,\;a_{2} = \int {\frac{F}{W}} \,u_{1} \,\text{d}y$$

(35)

where

$$F = - \left( {\frac{{4pr^{2} E}}{\zeta }} \right)\left[ {\frac{{\exp \left\{ { - r\left( {1 - y} \right)} \right\} + p^{2} \exp \left\{ { - 3r\left( {1 - y} \right)} \right\}}}{{\left[ {1 - p^{2} \exp \left\{ { - 2r\left( {1 - y} \right)} \right\}} \right]^{2} }}} \right]$$

W is the Wronksian for the system and is given by, $W = \left| {\begin{array}{*{20}c} {u_{1} } & {u_{2} } \\ {u^{\prime}_{1} } & {u^{\prime}_{2} } \\ \end{array} } \right| = - 2\,Ha.$

We proceed further in evaluating the particular integrals to obtain the general solution of Eq. (11) as follows

$$\begin{aligned} u\left( y \right) & = C_{1} \exp \left( {Ha\,y} \right) + C_{2} \exp \left( { - Ha\,y} \right) + \frac{{1 + Ha^{2} S}}{{Ha^{2} }} \\ & - \frac{2prE}{\zeta }\exp \left\{ { - r\left( {1 - y} \right)} \right\}\left[ {\frac{{{}_{2}F_{1} \left( {1,\frac{r - Ha}{2r};\frac{3}{2} - \frac{Ha}{2r};p^{2} \exp \left\{ { - 2r\left( {1 - y} \right)} \right\}} \right)}}{r - Ha}} \right. \\ & \left. { + \frac{{{}_{2}F_{1} \left( {1,\frac{r + Ha}{2r};\frac{3}{2} + \frac{Ha}{2r};p^{2} \exp \left\{ { - 2r\left( {1 - y} \right)} \right\}} \right)}}{r + Ha}} \right] \\ \end{aligned}$$

(36)

Imposing boundary as described in Eqs. (12a)–(12b), the constants C ₁ and C ₂ are evaluated as,

$$C_{1} = \frac{2prEG}{\zeta Ha} - \frac{{\frac{{1 + Ha^{2} S}}{{Ha^{2} }} + \frac{2prE}{\zeta }\left\{ {G_{1} + \frac{{G\left( {1 - \beta Ha} \right)\exp \left( {Ha} \right)}}{Ha}} \right\}}}{{2\left\{ {\cosh \left( {Ha} \right) - \beta Ha\sinh \left( {Ha} \right)} \right\}}}$$

$$C_{2} = - \frac{{\frac{{1 + Ha^{2} S}}{{Ha^{2} }} + \frac{2prE}{\zeta }\left\{ {G_{1} + \frac{{G\left( {1 - \beta Ha} \right)\exp \left( {Ha} \right)}}{Ha}} \right\}}}{{2\left\{ {\cosh \left( {Ha} \right) - \beta Ha\sinh \left( {Ha} \right)} \right\}}}$$

where the constant terms G and G ₁ are given by Eqs. (14a)–(14b).

Substituting the expressions for C ₁ and C ₂ in Eq. (36) and after some simplifications, one can obtain the closed-form analytical solution of u(y), as expressed in Eq. (13).