Abstract
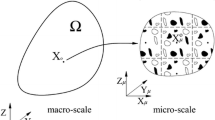
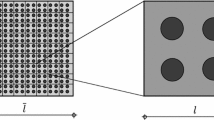
In the past decades computational homogenization has proven to be a powerful strategy to compute the overall response of continua. Central to computational homogenization is the Hill–Mandel condition. The Hill–Mandel condition is fulfilled via imposing displacement boundary conditions (DBC), periodic boundary conditions (PBC) or traction boundary conditions (TBC) collectively referred to as canonical boundary conditions. While DBC and PBC are widely implemented, TBC remains poorly understood, with a few exceptions. The main issue with TBC is the singularity of the stiffness matrix due to rigid body motions. The objective of this manuscript is to propose a generic strategy to implement TBC in the context of computational homogenization at finite strains. To eliminate rigid body motions, we introduce the concept of semi-Dirichlet boundary conditions. Semi-Dirichlet boundary conditions are non-homogeneous Dirichlet-type constraints that simultaneously satisfy the Neumann-type conditions. A key feature of the proposed methodology is its applicability for both strain-driven as well as stress-driven homogenization. The performance of the proposed scheme is demonstrated via a series of numerical examples.








Similar content being viewed by others
Notes
The term Piola stress is adopted instead of the more commonly used first Piola-Kirchhoff stress. Nonetheless, it seems that the term Piola stress is more appropriate for this stress measure. Recall, \(\varvec{P}\) is essentially the Piola transform of the Cauchy stress and ties perfectly to the Piola identity. Also historically, Kirchhoff (1824–1877) employed this stress measure after Piola (1794–1850), see also the discussion in [72].
References
Hill R (1972) On constitutive macro-variables for heterogeneous solids at finite strain. Proc R Soc Lond A 326:131–147
Ogden RW (1974) On the overall moduli of non-linear elastic composite materials. J Mech Phys Solids 22:541–553
Oden JT, Vemaganti K, Moës N (1999) Hierarchical modeling of heterogeneous solids. Comput Methods Appl Mech Eng 172:3–25
Markovic D, Ibrahimbegovic A (2004) On micro-macro interface conditions for micro scale based FEM for inelastic behavior of heterogeneous materials. Comput Methods Appl Mech Eng 193:5503–5523
Lloberas-Valls O, Rixen DJ, Simone A, Sluys LJ (2012) On micro-to-macro connections in domain decomposition multiscale methods. Comput Methods Appl Mech Eng 225–228:177–196
Eshelby JD (1957) The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc R Soc Lond A 241:376–396
Hashin Z, Shtrikman S (1963) A variational approach to the theory of the elastic behaviour of multiphase materials. J Mech Phys Solids 11:127–140
Walpole LJ (1966) On bounds for the overall elastic moduli of inhomogeneous systems-II. J Mech Phys Solids 14:289–301
Mori T, Tanaka K (1973) Average stress in matrix and average elastic energy of materials with misfitting inclusions. Acta Metall 21:571–574
Halpin JC, Kardos JL (1976) The Halpin-Tsai equations:The Halpin-Tsai equations: a review. Polym Eng Sci 16:344–352
Hori M, Nemat-Nasser S (1993) Double-inclusion model and overall moduli of multi-phase composites. Mech Mater 14:189–206
Willis JR (1986) Variational estimates for the overall response of an inhomogeneous nonlinear dielectric. In: Ericksen JL, Kinderlehrer D, Kohn R, Lions J-L (eds) Homogenization and effective moduli of materials and media, volume 1 of the IMA volumes in mathematics and its applications. Springer, New York, pp 247–263
Talbot DRS, Willis JR (1992) Some simple explicit bounds for the overall behaviour of nonlinear composites. Int J Solids Struct 29:1981–1987
Ponte Castañeda P, deBotton G, Li G (1992) Effective properties of nonlinear inhomogeneous dielectrics. Phys Rev B 46:4387–4394
Ponte P (1996) Castañeda. Exact second-order estimates for the effective mechanical properties of nonlinear composite materials. J Mech Phys Solids 44:827–862
Mura T, Shodja HM, Hirose Y (1996) Inclusion problems. Appl Mech Rev 49:118–127
Böhm HJ (1998) A short introduction to basic aspects of continuum mechanics. CDL-FMD Report 3. Technical report, Institute of Lightweight Design and Structural Biomechanics (ILSB), Vienna University of Technology
Nemat-Nasser S, Hori M (1999) Micromechanics: overall properties of heterogeneous materials. Elsevier, Amsterdam
Klusemann B, Böhm HJ, Svendsen B (2012) Homogenization methods for multi-phase elastic composites: comparisons and benchmarks. Eur J Mech A Solids 34:21–37
Miehe C, Schröder J, Schotte J (1999) Computational homogenization analysis in finite plasticity simulation of texture development in polycrystalline materials. Comput Methods Appl Mech Eng 171:387–418
Feyel F, Chaboche J-L (2000) \(\text{ FE }^{2}\) multiscale approach for modelling the elastoviscoplastic behaviour of long fibre SiC/Ti composite materials. Comput Methods Appl Mech Eng 183:309–330
Terada K, Hori M, Kyoya T, Kikuchi N (2000) Simulation of the multi-scale convergence in computational homogenization approaches. Int J Solids Struct 37:2285–2311
Kouznetsova VG, Brekelmans WAM, Baaijens FPT (2001) An approach to micro-macro modeling of heterogeneous materials. Comput Mech 27:37–48
Jiang M, Alzebdeh K, Jasiuk I, Ostoja-Starzewski M (2001) Scale and boundary conditions effects in elastic properties of random composites. Acta Mech 148:63–78
Miehe C, Schröder J, Becker M (2002) Computational homogenization analysis in finite elasticity: material and structural instabilities on the micro- and macro-scales of periodic composites and their interaction. Comput Methods Appl Mech Eng 191:4971–5005
Moës N, Cloirec M, Cartraud P, Remacle J-F (2003) A computational approach to handle complex microstructure geometries. Comput Methods Appl Mech Eng 192:3163–3177
Zohdi TI, Wriggers P (2005) Introduction to computational micromechanics. Springer, Berlin
Segurado J, Llorca J (2006) Computational micromechanics of composites: the effect of particle spatial distribution. Mech Mater 38:873–883
Wriggers P, Moftah SO (2006) Mesoscale models for concrete: homogenisation and damage behaviour. Finite Elem Anal Des 42:623–636
Geers MGD, Coenen EWC, Kouznetsova VG (2007) Multi-scale computational homogenization of structured thin sheets. Modell Simul Mater Sci Eng 15:393–404
Gitman IM, Askes H, Sluys LJ (2007) Representative volume: existence and size determination. Eng Fract Mech 74:2518–2534
Yvonnet J, He Q-C (2007) The reduced model multiscale method (R3M) for the non-linear homogenization of hyperelastic media at finite strains. J Comput Phys 223:341–368
Temizer İ, Zohdi TI (2007) A numerical method for homogenization in non-linear elasticity. Comput Mech 40:281–298
Temizer İ, Wriggers P (2007) An adaptive method for homogenization in orthotropic nonlinear elasticity. Comput Methods Appl Mech Eng 196:3409–3423
Temizer İ, Wriggers P (2008) On the computation of the macroscopic tangent for multiscale volumetric homogenization problems. Comput Methods Appl Mech Eng 198:495–510
Özdemir I, Brekelmans WAM, Geers MGD (2008) Computational homogenization for heat conduction in heterogeneous solids. Int J Numer Meth Eng 73:185–204
Nezamabadi S, Yvonnet J, Zahrouni H, Potier-Ferry M (2009) A multilevel computational strategy for handling microscopic and macroscopic instabilities. Comput Methods Appl Mech Eng 198:2099–2110
Larsson F, Runesson K (2011) On two-scale adaptive FE analysis of micro-heterogeneous media with seamless scale-bridging. Comput Methods Appl Mech Eng 200:2662–2674
Temizer İ, Wriggers P (2011) Homogenization in finite thermoelasticity. J Mech Phys Solids 59:344–372
Coenen EWC, Kouznetsova VG, Bosco E, Geers MGD (2012) A multi-scale approach to bridge microscale damage and macroscale failure: a nested computational homogenization-localization framework. Int J Fract 178:157–178
Nguyen VP, Stroeven M, Sluys LJ (2012) Multiscale failure modeling of concrete: Micromechanical modeling, discontinuous homogenization and parallel computations. Comput Methods Appl Mech Eng 201–204:139–156
Fritzen F, Leuschner M (2013) Reduced basis hybrid computational homogenization based on a mixed incremental formulation. Comput Methods Appl Mech Eng 260:143–154
Javili A, Chatzigeorgiou G, Steinmann P (2013) Computational homogenization in magneto-mechanics. Int J Solids Struct 50:4197–4216
Kochmann DM, Venturini GN (2013) Homogenized mechanical properties of auxetic composite materials in finite-strain elasticity. Smart Mater Struct 22:084004
Yvonnet J, Monteiro E, He Q-C (2013) Computational homogenization method and reduced database model for hyperelastic heterogeneous structures. Int J Multiscale Comput Eng 11:201–225
Balzani D, Scheunemann L, Brands D, Schröder J (2014) Construction of two- and three-dimensional statistically similar RVEs for coupled micro-macro simulations. Comput Mech 54:1269–1284
Keip M-A, Steinmann P, Schröder J (2014) Two-scale computational homogenization of electro-elasticity at finite strains. Comput Methods Appl Mech Eng 278:62–79
Fritzen F, Kochmann DM (2014) Material instability-induced extreme damping in composites: A computational study. Int J Solids Struct 51:4101–4112
Le BA, Yvonnet J, He Q-C (2015) Computational homogenization of nonlinear elastic materials using neural networks. Int J Numer Meth Eng 104:1061–1084
van Dijk NP (2016) Formulation and implementation of stress-driven and/or strain-driven computational homogenization for finite strain. Int J Numer Meth Eng 107:1009–1028
Kanouté P, Boso DP, Chaboche JL, Schrefler BA (2009) Multiscale methods for composites: a review. Arch Comput Methods Eng 16:31–75
Charalambakis N (2010) Homogenization techniques and micromechanics. A survey and perspectives. Appl Mech Rev 63:030803
Geers MGD, Kouznetsova VG, Brekelmans WAM (2010) Multi-scale computational homogenization: Trends and challenges. J Comput Appl Math 234:2175–2182
Nguyen VP, Stroeven M, Sluys LJ (2011) Multiscale continuous and discontinuous modelling of heterogeneous materials: A review on recent developments. J Multiscale Model 3:229–270
Saeb S, Steinmann P, Javili A (2016) Aspects of computational homogenization at finite deformations. A unifying review from Reuss’ to Voigt’s bound. Appl Mech Rev 68:050801
Linder C, Tkachuk M, Miehe C (2011) A micromechanically motivated diffusion-based transient network model and its incorporation into finite rubber viscoelasticity. J Mech Phys Solids 59:2134–2156
Tkachuk M, Linder C (2012) The maximal advance path constraint for the homogenization of materials with random network microstructure. Philos Mag 92:2779–2808
Raina A, Linder C (2014) A homogenization approach for nonwoven materials based on fiber undulations and reorientation. J Mech Phys Solids 65:12–34
Kanit T, Forest S, Galliet I, Mounoury V, Jeulin D (2003) Determination of the size of the representative volume element for random composites: statistical and numerical approach. Int J Solids Struct 40:3647–3679
Kouznetsova VG, Geers MGD, Brekelmans WAM (2002) Multi-scale constitutive modelling of heterogeneous materials with a gradient-enhanced computational homogenization scheme. Int J Numer Methods Eng 54:1235–1260
Khisaeva ZF, Ostoja-Starzewski M (2006) On the size of RVE in finite elasticity of random composites. J Elast 85:153–173
Javili A, Chatzigeorgiou G, McBride A, Steinmann P, Linder C (2015) Computational homogenization of nano-materials accounting for size effects via surface elasticity. GAMM Mitteilungen 38:285–312
Nemat-Nasser S, Hori M (1995) Universal bounds for overall properties of linear and nonlinear heterogeneous solids. J Eng Mater Technol 117:412–432
Miehe C (2002) Strain-driven homogenization of inelastic microstructures and composites based on an incremental variational formulation. Int J Numer Methods Eng 55:1285–1322
Kaczmarczyk L, Pearce CJ, Bićanić N (2008) Scale transition and enforcement of RVE boundary conditions in second-order computational homogenization. Int J Numer Methods Eng 74:506–522
Perić D, de Souza Neto EA, Feijóo RA, Partovi M, Carneiro Molina AJ (2011) On micro-to-macro transitions for multi-scale analysis of non-linear heterogeneous materials. Int J Numer Methods Eng 87:149–170
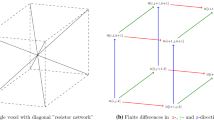
Mesarovic SD, Padbidri J (2005) Minimal kinematic boundary conditions for simulations of disordered microstructures. Philos Mag 85:65–78
Shen H, Brinson LC (2006) A numerical investigation of the effect of boundary conditions and representative volume element size for porous titanium. J Mech Mater Struct 1:1179–1204
Drago A, Pindera M-J (2007) Micro-macromechanical analysis of heterogeneous materials: macroscopically homogeneous vs periodic microstructures. Compos Sci Technol 67:1243–1263
Miehe C (2003) Computational micro-to-macro transitions for discretized micro-structures of heterogeneous materials at finite strains based on the minimization of averaged incremental energy. Comput Methods Appl Mech Eng 192:559–591
Felippa CA, Park KC (2002) The construction of free-free flexibility matrices for multilevel structural analysis. Comput Methods Appl Mech Eng 191:2139–2168
Podio-Guidugli Paolo (2000) Primer in elasticity. J Elast 58(1):1–104
Yuan Z, Fish J (2008) Toward realization of computational homogenization in practice. Int J Numer Methods Eng 73:361–380
Larsson F, Runesson K, Saroukhani S, Vafadari R (2011) Computational homogenization based on a weak format of micro-periodicity for RVE-problems. Comput Methods Appl Mech Eng 200:11–26
Nguyen V-D, Béchet E, Geuzaine C, Noels L (2012) Imposing periodic boundary condition on arbitrary meshes by polynomial interpolation. Comput Mater Sci 55:390–406
Hirschberger CB, Ricker S, Steinmann P, Sukumar N (2009) Computational multiscale modelling of heterogeneous material layers. Eng Fract Mech 76(6):793–812
McBride A, Mergheim J, Javili A, Steinmann P, Bargmann S (2012) Micro-to-macro transitions for heterogeneous material layers accounting for in-plane stretch. J Mech Phys Solids 60:1221–1239
Miehe C, Koch A (2002) Computational micro-to-macro transitions of discretized microstructures undergoing small strains. Arch Appl Mech 72:300–317
Larsson F, Runesson K (2007) RVE computations with error control and adaptivity: the power of duality. Comput Mech 39:647–661
Fish J, Fan R (2008) Mathematical homogenization of nonperiodic heterogeneous media subjected to large deformation transient loading. Int J Numer Methods Eng 76:1044–1064
Javili A, Dortdivanlioglu B, Kuhl E, Linder C (2015) Computational aspects of growth-induced instabilities through eigenvalue analysis. Comput Mech 56(3):405–420
Dortdivanlioglu B, Javili A, Linder C. Computational aspects of morphological instabilities using isogeometric analysis. Comput Methods Appl Mech Eng. doi:10.1016/j.cma.2016.06.028
Acknowledgments
The support of this work by the Cluster of Excellence “Engineering of Advanced Materials ”at the University of Erlangen-Nuremberg, funded by the DFG within the framework of its “Excellence Initiative”, is greatly appreciated.
Author information
Authors and Affiliations
Corresponding author
Additional information
The term semi-Dirichlet exists in mathematics but, in the completely different context of semi-Dirichlet forms and should not be confused with our non-homogeneous Dirichlet-type constraints.
Appendix: Implementation of TBC via the Lagrange multiplier method
Appendix: Implementation of TBC via the Lagrange multiplier method
This methodology is essentially based on solving the incremental minimization problem of homogenization
with \(\mathbf {d}\) being the unknown global vector of deformations which minimizes the average incremental energy of the micro-structure for a given macro deformation gradient. In order to impose the constraint \(\langle {\varvec{F}} \rangle - {}^\text {{M}}\!{\varvec{F}} \overset{!}{=} \varvec{0}\), the Lagrange multiplier method is used which yields the Lagrangian
with \(\varvec{\lambda }\) being the Lagrange multiplier. It can be readily verified that
Next, the derivatives of the Lagrangian functional with respect to its variables are set to zero and the following system of equations is obtained.
Linearization of this system of equations yields
where \(\mathbf {K} = \partial {\mathbf {R}_{\varvec{\text {int}}}}/\partial {\mathbf {d}}\) is the assembled tangent stiffness matrix. In order to represent the system of Eq. (19) in matrix format, all tensor products should be reformulates as matrix multiplications. To do so, we first derive the nodal equivalents of the global matrices \(\partial \mathbf {R}_{\varvec{\text {ext}}}/\partial {}^\text {{M}}\!{\varvec{P}}\) and \(\partial \langle {\varvec{F}} \rangle /\partial {\mathbf {d}}\) as
respectively. The non-standard tensor product \(\overline{\otimes }\) of a second-order tensor \(\varvec{A}\) and a vector \(\varvec{b}\) is the third-order tensor \(\mathbbm {D} = \varvec{A} \, \overline{\otimes }\, \varvec{b}\) with components \({D}_{ijk} = {A}_{ik} {b}_{j}\). Next, the double contraction of the third-order tensor (20)\(_{1}\) with \(\Delta {}^\text {{M}}\!{\varvec{P}}\) and the dot product of the third-order tensor (20)\(_{2}\) with nodal \(\Delta \varphi ^{J}\) in matrix format are derived as
respectively. Hence, the final matrix format representation of the system of Eq. (19) the reads

where n denotes the total number of nodes and \(\text{ Grad }N\) is represented as \(\nabla N\) for the sake of space. This system turns out to be not singular and can be solved without further modifications.
Rights and permissions
About this article
Cite this article
Javili, A., Saeb, S. & Steinmann, P. Aspects of implementing constant traction boundary conditions in computational homogenization via semi-Dirichlet boundary conditions. Comput Mech 59, 21–35 (2017). https://doi.org/10.1007/s00466-016-1333-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00466-016-1333-8