Evolutionary topological design for phononic band gap crystals

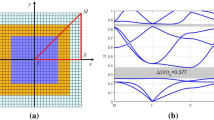
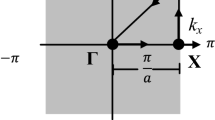
Abstract

Phononic band gap crystals are made of periodic inclusions embedded in a base material, which can forbid the propagation of elastic and acoustic waves within certain range of frequencies. In the past two decades, the systematic design of phononic band gap crystals has attracted increasing attention due to their wide practical applications such as sound insulation, waveguides, or acoustic wave filtering. This paper proposes a new topology optimization algorithm based on bi-directional evolutionary structural optimization (BESO) method and finite element analysis for the design of phononic band gap crystals. The study on the maximizing gap size between two adjacent bands has been systematically conducted for out-of-plane waves, in-plane waves and the coupled in-plane and out-of-plane waves. Numerical results demonstrate that the proposed optimization algorithm is effective and efficient for the design of phononic band gap crystals and various topological patterns of optimized phononic structures are presented. Several new patterns for phononic band gap crystals have been successfully obtained.

Appendix I

Element mass matrix, M, and element stiffness matrix, K, are calculated by the following equations. For out-of-plane waves,

$$ {\mathbf{M}}_e^{out}=\rho {\displaystyle {\int}_{\varOmega }{\mathbf{N}}^T\mathbf{N}d\varOmega } $$

(35)

$$ {\mathbf{K}}_e=\mu {\displaystyle \sum_{m=1}^4{\mathbf{K}}_m^{out}} $$

(36)

where

$$ \begin{array}{l}{\mathbf{K}}_1^{out}={\displaystyle {\int}_{\varOmega}\left(\frac{\partial {\mathbf{N}}^T}{\partial x}\frac{\partial \mathbf{N}}{\partial x}+\frac{\partial {\mathbf{N}}^T}{\partial y}\frac{\partial \mathbf{N}}{\partial y}\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_2^{out}={\displaystyle {\int}_{\varOmega }i{k}_x\left(\frac{\partial {\mathbf{N}}^T}{\partial x}\mathbf{N}-{\mathbf{N}}^T\frac{\partial \mathbf{N}}{\partial x}\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_3^{out}={\displaystyle {\int}_{\varOmega }i{k}_y\left(\frac{\partial {\mathbf{N}}^T}{\partial y}\mathbf{N}-{\mathbf{N}}^T\frac{\partial \mathbf{N}}{\partial y}\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_4^{out}={\displaystyle {\int}_{\varOmega}\left({k}_x^2+{k}_y^2\right)\left({\mathbf{N}}^T\mathbf{N}\right)d\varOmega}\hfill \end{array} $$

For in-plane waves:

$$ {\mathbf{M}}_e^{in}=\rho {\displaystyle {\int}_{\varOmega }{\mathbf{N}}^T\mathbf{N}d\varOmega } $$

(37)

$$ {\mathbf{K}}_e^{in}={\displaystyle \sum_{m=1}^6{\mathbf{K}}_m^{in}} $$

(38)

where

$$ \begin{array}{l}{\mathbf{K}}_1^{in}={\displaystyle {\int}_{\varOmega}\left({\mathbf{B}}_1^T\mathbf{C}{\mathbf{B}}_1\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_2^{in}={\displaystyle {\int}_{\varOmega }i{k}_x\left({\mathbf{B}}_1^T\mathbf{C}{\mathbf{B}}_2-{\mathbf{B}}_2^T\mathbf{C}{\mathbf{B}}_1\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_3^{in}={\displaystyle {\int}_{\varOmega }i{k}_y\left({\mathbf{B}}_1^T\mathbf{C}{\mathbf{B}}_3-{\mathbf{B}}_3^T\mathbf{C}{\mathbf{B}}_1\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_4^{in}={\displaystyle {\int}_{\varOmega }{k}_x^2\left({\mathbf{B}}_2^T\mathbf{C}{\mathbf{B}}_2\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_5^{in}={\displaystyle {\int}_{\varOmega }{k}_y^2\left({\mathbf{B}}_3^T\mathbf{C}{\mathbf{B}}_3\right)d\varOmega}\hfill \\ {}{\mathbf{K}}_6^{in}={\displaystyle {\int}_{\varOmega }{k}_x{k}_y\left({\mathbf{B}}_2^T\mathbf{C}{\mathbf{B}}_3+{\mathbf{B}}_3^T\mathbf{C}{\mathbf{B}}_2\right)d\varOmega}\hfill \\ {}{\mathbf{B}}_1={\mathbf{L}}_1\frac{\partial \mathbf{N}}{\partial x}+{\mathbf{L}}_2\frac{\partial \mathbf{N}}{\partial y}\&{\mathbf{B}}_2={\mathbf{L}}_1\mathbf{N}\&{\mathbf{B}}_3={\mathbf{L}}_2\mathbf{N}\hfill \\ {}\mathbf{C}=\left[\begin{array}{ccc}\hfill \lambda +2\mu \hfill & \hfill \lambda \hfill & \hfill 0\hfill \\ {}\hfill \lambda \hfill & \hfill \lambda +2\mu \hfill & \hfill 0\hfill \\ {}\hfill 0\hfill & \hfill 0\hfill & \hfill \mu \hfill \end{array}\right]\hfill \\ {}{\mathbf{L}}_1=\left[\begin{array}{cc}\hfill 1\hfill & \hfill 0\hfill \\ {}\hfill 0\hfill & \hfill 0\hfill \\ {}\hfill 0\hfill & \hfill 1\hfill \end{array}\right]\kern0.5em \&\kern0.5em {\mathbf{L}}_2=\left[\begin{array}{cc}\hfill 0\hfill & \hfill 0\hfill \\ {}\hfill 0\hfill & \hfill 1\hfill \\ {}\hfill 1\hfill & \hfill 0\hfill \end{array}\right]\hfill \end{array} $$

where Ω denotes the area of an element. N is the shape function matrix of an element corresponding to out of plane waves or in plane waves. In our numerical examples, linear 4-node elements are used and the shape function matrix can be expressed by:

• For out-of-plane waves:

  $$ \mathbf{N}=\left[\begin{array}{cccc}\hfill {N}_1\hfill & \hfill {N}_2\hfill & \hfill {N}_3\hfill & \hfill {N}_4\hfill \end{array}\right] $$

• For in-plane waves:

  $$ \mathbf{N}=\left[\begin{array}{c}\hfill \begin{array}{cccc}\hfill {N}_1\hfill & \hfill 0\hfill & \hfill {N}_2\hfill & \hfill \begin{array}{cc}\hfill 0\hfill & \hfill \begin{array}{cccc}\hfill {N}_3\hfill & \hfill 0\hfill & \hfill {N}_4\hfill & \hfill 0\hfill \end{array}\hfill \end{array}\hfill \end{array}\hfill \\ {}\hfill \begin{array}{cccc}\hfill 0\hfill & \hfill {N}_1\hfill & \hfill 0\hfill & \hfill \begin{array}{cc}\hfill \begin{array}{cccc}\hfill {N}_2\hfill & \hfill 0\hfill & \hfill {N}_3\hfill & \hfill 0\hfill \end{array}\hfill & \hfill {N}_4\hfill \end{array}\hfill \end{array}\hfill \end{array}\right] $$

The differential element mass matrix and element stiffness matrix with respect to design variable, x _e, are:

• For out-of-plane waves:

  $$ \frac{\partial {\mathbf{M}}_e^{out}}{\partial {x}_e}=\left({\rho}_1-{\rho}_2\right){\displaystyle {\int}_{\varOmega }{\mathbf{N}}^T\mathbf{N}d\varOmega } $$

  (39)
  $$ \frac{\partial {\mathbf{K}}_e^{out}}{\partial {x}_e}=\left({\mu}_1-{\mu}_2\right){\displaystyle \sum_{m=1}^4{\mathbf{K}}_m^{out}} $$

  (40)

• For in-plane waves:

  $$ \frac{\partial {\mathbf{M}}_e^{in}}{\partial {x}_e}=\left({\rho}_1-{\rho}_2\right){\displaystyle {\int}_{\varOmega }{\mathbf{N}}^T\mathbf{N}d\varOmega } $$

  (41)
  $$ \frac{\partial {\mathbf{K}}_e^{in}}{\partial {x}_e}={\displaystyle \sum_{m=1}^6\frac{\partial {\mathbf{K}}_m^{in}}{\partial {x}_e}} $$

  (42)

$\frac{\partial {\mathbf{K}}_m^{in}}{\partial {x}_e}$ is calculated by substituting C with $\frac{\partial \mathbf{C}}{\partial {x}_e}$ in equation (38)

$$ \frac{\partial \mathbf{C}}{\partial {x}_e}=\left[\begin{array}{ccc}\hfill \left({\lambda}_2-{\lambda}_1\right)+2\left({\mu}_2-{\mu}_1\right)\hfill & \hfill \left({\lambda}_2-{\lambda}_1\right)\hfill & \hfill 0\hfill \\ {}\hfill \left({\lambda}_2-{\lambda}_1\right)\hfill & \hfill \left({\lambda}_2-{\lambda}_1\right)+2\left({\mu}_2-{\mu}_1\right)\hfill & \hfill 0\hfill \\ {}\hfill 0\hfill & \hfill 0\hfill & \hfill \left({\mu}_2-{\mu}_1\right)\hfill \end{array}\right] $$