A simple coronary blood flow model to study the collateral flow index

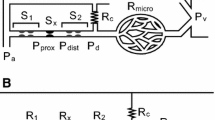
Abstract

In this work, we present a novel modeling framework to investigate the effects of collateral circulation into the coronary blood flow physiology. A prototypical model of the coronary tree, integrated with the concept of Collateral Flow Index (CFI), is employed to gain insight about the role of model parameters associated with the collateral circuitry, which results in physically-realizable solutions for specific CFI data. Then, we discuss the mathematical feasibility of pressure-derived CFI, anatomical implications and practical considerations involving the estimation of model parameters in collateral connections. A sensitivity analysis is carried out, and the investigation of the impact of the collateral circulation on FFR values is also addressed.

Appendix: Governing equations for the blood flow model

1.1 LAD totally occluded

In this case it is assumed that $R_l\rightarrow \infty$, and so $Q_l=0$. Also, the resistance $R_{pl}$ enters in hyperemic state, that is $R_{pl}\rightarrow R_{pl}^h=\frac{R_{pl}}{\text{ CFR }}$. In such situation, the pressure $P_l$ turns into the wedge pressure $P_l^w$. The formulae for the circuit reads

$$\begin{aligned} Q_{pl}&= Q_{rl} + Q_{xl}, \end{aligned}$$

(23a)

$$\begin{aligned} Q_{pl} R_{pl}^h&= P_l^w - P_v, \end{aligned}$$

(23b)

$$\begin{aligned} Q_r R_r + Q_{rl} R_{rl}&= P_a - P_l^w, \end{aligned}$$

(23c)

$$\begin{aligned} Q_x R_x + Q_{xl} R_{xl}&= P_a - P_l^w, \end{aligned}$$

(23d)

$$\begin{aligned} Q_r R_r&= P_a - P_r, \end{aligned}$$

(23e)

$$\begin{aligned} Q_x R_x&= P_a - P_x, \end{aligned}$$

(23f)

$$\begin{aligned} Q_{pr} R_{pr}&= P_r - P_v, \end{aligned}$$

(23g)

$$\begin{aligned} Q_{px} R_{px}&= P_x - P_v, \end{aligned}$$

(23h)

$$\begin{aligned} Q_{rx} R_{rx}&= P_r - P_x, \end{aligned}$$

(23i)

$$\begin{aligned} Q_r&= Q_{pr} + Q_{rl} + Q_{rx}, \end{aligned}$$

(23j)

$$\begin{aligned} Q_x&= Q_{px} + Q_{xl} - Q_{rx}. \end{aligned}$$

(23k)

Combining (23b) and (23a), and then using (23j) and (23k) gives

$$\begin{aligned} \dfrac{P_l^w - P_v}{R_{pl}^h} = Q_{rl} + Q_{xl} = Q_x + Q_r - Q_{pr} - Q_{px}. \end{aligned}$$

(24)

Now, introducing (23e), (23f), (23g) and (23h) yields

$$\begin{aligned} \dfrac{P_l^w - P_v}{R_{pl}^h}= \dfrac{P_a-P_x}{R_x} + \dfrac{P_a-P_r}{R_r} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_x-P_v}{R_{px}}. \end{aligned}$$

(25)

Further manipulations yield

$$\begin{aligned}&\dfrac{P_l^w - P_v}{R_{pl}^h}= \dfrac{P_a - P_v}{R_x} - \dfrac{P_x-P_v}{R_x} \nonumber \\&\quad + \dfrac{P_a-P_v}{R_r} - \dfrac{P_r-P_v}{R_r} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_x-P_v}{R_{px}}. \end{aligned}$$

(26)

Now, we define the pressure drops

$$\begin{aligned} \varDelta P_a&= P_a - P_v, \end{aligned}$$

(27)

$$\begin{aligned} \varDelta P_r^l&= P_r - P_v, \end{aligned}$$

(28)

$$\begin{aligned} \varDelta P_x^l&= P_x - P_v, \end{aligned}$$

(29)

$$\begin{aligned} \varDelta P_l^w&= P_l^w - P_v, \end{aligned}$$

(30)

where the superscript $(\cdot )^l$ makes reference to the fact that we are considering the occlusion of the LAD. Then, expressing equation (26) in terms of pressure drops and rearranging terms yields

$$\begin{aligned}&\dfrac{\varDelta P_l^w}{R_{pl}^h} + \left( \dfrac{1}{R_r}+\dfrac{1}{R_{pr}}\right) \varDelta P_r^l + \left( \dfrac{1}{R_x}+\dfrac{1}{R_{px}}\right) \varDelta P_x^l \nonumber \\&\quad =\left( \dfrac{1}{R_r} + \dfrac{1}{R_x}\right) \varDelta P_a. \end{aligned}$$

(31)

Note that the problem has three unknowns, in short denoted by $\varDelta {\mathbf {P}}_l=(\varDelta P_l^w, \varDelta P_r^l, \varDelta P_x^l)$. We now employ (23c), (23e), (23j), (23g) and (23i) to obtain the second equation

$$\begin{aligned}&P_a - P_l^w = Q_r R_r + Q_{rl} R_{rl} = P_a - P_r + (Q_r - Q_{pr} - Q_{rx}) R_{rl} \nonumber \\&\quad = P_a - P_r + \left( \dfrac{P_a-P_r}{R_r} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_r-P_x}{R_{rx}}\right) R_{rl}. \end{aligned}$$

(32)

Adding and subtracting $P_v$, and manipulating the equation above

$$\begin{aligned}&- \frac{P_l^w-P_v}{R_{rl}} = - \frac{P_r-P_v}{R_{rl}} + \dfrac{P_a-P_v}{R_r} \nonumber \\&\quad - \dfrac{P_r-P_v}{R_r} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_r-P_v}{R_{rx}} + \dfrac{P_x-P_v}{R_{rx}}. \end{aligned}$$

(33)

Using the definition of pressure drops, we finally get

$$\begin{aligned} - \dfrac{\varDelta P_l^w}{R_{rl}} + \left( \dfrac{1}{R_{rl}} +\dfrac{1}{R_r} + \dfrac{1}{R_{pr}} + \dfrac{1}{R_{rx}} \right) \varDelta P_r^l - \dfrac{\varDelta P_x^l}{R_{rx}} = \dfrac{\varDelta P_a}{R_r}. \end{aligned}$$

(34)

The third equation is obtained analogously. Using (23d), (23f), (23h), (23i) and (23k) we get

$$\begin{aligned}&P_a - P_l^w = Q_x R_x + Q_{xl} R_{xl} = P_a - P_x + (Q_x - Q_{px} + Q_{rx}) R_{xl} \nonumber \\&\quad = P_a - P_x + \left( \dfrac{P_a-P_x}{R_x} - \dfrac{P_x-P_v}{R_{px}} + \dfrac{P_r-P_x}{R_{rx}}\right) R_{xl}. \end{aligned}$$

(35)

Rewriting the equation in terms of pressure drops gives

$$\begin{aligned} - \dfrac{\varDelta P_l^w}{R_{xl}} - \dfrac{\varDelta P_r^l}{R_{rx}} + \left( \dfrac{1}{R_{xl}} + \dfrac{1}{R_x} + \dfrac{1}{R_{px}} + \dfrac{1}{R_{rx}} \right) \varDelta P_x^l = \dfrac{\varDelta P_a}{R_x}. \end{aligned}$$

(36)

And, so, we arrive at the system of equations

$$\begin{aligned}&\begin{pmatrix} \frac{1}{R_{pl}^h} &{} \frac{1}{R_r}+\frac{1}{R_{pr}} &{} \frac{1}{R_x}+\frac{1}{R_{px}}\\ -\frac{1}{R_{rl}} &{} \frac{1}{R_r^{\parallel }} &{} -\frac{1}{R_{rx}}\\ -\frac{1}{R_{xl}} &{} -\frac{1}{R_{rx}} &{} \frac{1}{R_x^{\parallel }} \end{pmatrix} \begin{pmatrix} \varDelta P_l^w\\ \varDelta P_r^l\\ \varDelta P_x^l \end{pmatrix} \frac{1}{\varDelta P_a} = \begin{pmatrix} \frac{1}{R_r}+\frac{1}{R_x}\\ \frac{1}{R_r}\\ \frac{1}{R_x} \end{pmatrix} \end{aligned}$$

(37)

where

$$\begin{aligned} \frac{1}{R_r^{\parallel }}&= \dfrac{1}{R_{rl}} +\dfrac{1}{R_r} + \dfrac{1}{R_{pr}} + \dfrac{1}{R_{rx}}, \end{aligned}$$

(38)

$$\begin{aligned} \frac{1}{R_x^{\parallel }}&= \dfrac{1}{R_{xl}} + \dfrac{1}{R_x} + \dfrac{1}{R_{px}} + \dfrac{1}{R_{rx}}. \end{aligned}$$

(39)

1.2 RCA totally occluded

Now, it is assumed that $R_r\rightarrow \infty$, and so $Q_r=0$, and that the hyperemic state renders $R_{pr}\rightarrow R_{pr}^h=\frac{R_{pr}}{\text {CFR}}$, with the wedge pressure being $P_r^w$. The formulae for this new circuit becomes

$$\begin{aligned} Q_{pr}&= -(Q_{rx} + Q_{rl}), \end{aligned}$$

(40a)

$$\begin{aligned} Q_{pr} R_{pr}^h&= P_r^w - P_v, \end{aligned}$$

(40b)

$$\begin{aligned} Q_l R_l - Q_{rl} R_{rl}&= P_a - P_r^w, \end{aligned}$$

(40c)

$$\begin{aligned} Q_x R_x - Q_{rx} R_{rx}&= P_a - P_r^w, \end{aligned}$$

(40d)

$$\begin{aligned} Q_l R_l&= P_a - P_l, \end{aligned}$$

(40e)

$$\begin{aligned} Q_x R_x&= P_a - P_x, \end{aligned}$$

(40f)

$$\begin{aligned} Q_{pl} R_{pl}&= P_l - P_v, \end{aligned}$$

(40g)

$$\begin{aligned} Q_{px} R_{px}&= P_x - P_v, \end{aligned}$$

(40h)

$$\begin{aligned} Q_{xl} R_{xl}&= P_x - P_l, \end{aligned}$$

(40i)

$$\begin{aligned} Q_l&= Q_{pl} - Q_{rl} - Q_{xl}, \end{aligned}$$

(40j)

$$\begin{aligned} Q_x&= Q_{px} - Q_{rx} + Q_{xl}. \end{aligned}$$

(40k)

Combining (40b) and (40a), and then using (40j) and (40k) gives

$$\begin{aligned} \dfrac{P_r^w - P_v}{R_{pr}^h} = -(Q_{rx} + Q_{rl}) = Q_x + Q_l - Q_{px} - Q_{pl}. \end{aligned}$$

(41)

Putting (40e), (40f), (40g) and (40h) results

$$\begin{aligned} \dfrac{P_r^w - P_v}{R_{pr}^h}= \dfrac{P_a-P_x}{R_x} + \dfrac{P_a-P_l}{R_l} - \dfrac{P_x-P_v}{R_{px}} - \dfrac{P_l-P_v}{R_{pl}}. \end{aligned}$$

(42)

After some rearrangements

$$\begin{aligned}&\dfrac{P_r^w - P_v}{R_{pr}^h}= \dfrac{P_a - P_v}{R_x} - \dfrac{P_x-P_v}{R_x} \nonumber \\&\quad + \dfrac{P_a-P_v}{R_l} - \dfrac{P_l-P_v}{R_l} - \dfrac{P_x-P_v}{R_{px}} - \dfrac{P_l-P_v}{R_{pl}}. \end{aligned}$$

(43)

Now, we define the pressure drops

$$\begin{aligned} \varDelta P_a&= P_a - P_v, \end{aligned}$$

(44)

$$\begin{aligned} \varDelta P_l^r&= P_l - P_v, \end{aligned}$$

(45)

$$\begin{aligned} \varDelta P_x^r&= P_x - P_v, \end{aligned}$$

(46)

$$\begin{aligned} \varDelta P_r^w&= P_r^w - P_v, \end{aligned}$$

(47)

with superscript $(\cdot )^r$ indicating that we are occluding the RCA. Then, in term of pressure drops, (43) yields

$$\begin{aligned}&\dfrac{\varDelta P_r^w}{R_{pr}^h} + \left( \dfrac{1}{R_l}+\dfrac{1}{R_{pl}}\right) \varDelta P_l^r + \left( \dfrac{1}{R_x}+\dfrac{1}{R_{px}}\right) \varDelta P_x^r \nonumber \\&\quad = \left( \dfrac{1}{R_l} + \dfrac{1}{R_x}\right) \varDelta P_a. \end{aligned}$$

(48)

The three unknowns in this situation are $\varDelta {\mathbf {P}}_r=( \varDelta P_l^r, \varDelta P_r^w,\varDelta P_x^r)$. We now use (40c), (40e), (40j), (40g) and (40i) to arrive at the second equation

$$\begin{aligned}&P_a - P_r^w = Q_l R_l - Q_{rl} R_{rl} = P_a - P_l + (Q_l - Q_{pl} + Q_{xl}) R_{rl} \nonumber \\&\quad = P_a - P_l + \left( \dfrac{P_a-P_l}{R_l} - \dfrac{P_l-P_v}{R_{pl}} + \dfrac{P_x-P_l}{R_{xl}}\right) R_{rl}. \end{aligned}$$

(49)

Adding and subtracting $P_v$, and manipulating the equation above

$$\begin{aligned}&- \frac{P_r^w-P_v}{R_{rl}} = - \frac{P_l-P_v}{R_{rl}} + \dfrac{P_a-P_v}{R_l} \nonumber \\&\quad - \dfrac{P_l-P_v}{R_l} - \dfrac{P_l-P_v}{R_{pl}} - \dfrac{P_l-P_v}{R_{xl}} + \dfrac{P_x-P_v}{R_{xl}}. \end{aligned}$$

(50)

Using the definition of pressure drops, we get

$$\begin{aligned} - \dfrac{\varDelta P_r^w}{R_{rl}} + \left( \dfrac{1}{R_{rl}} +\dfrac{1}{R_l} + \dfrac{1}{R_{pl}} + \dfrac{1}{R_{xl}} \right) \varDelta P_l^r - \dfrac{\varDelta P_x^r}{R_{xl}} = \dfrac{\varDelta P_a}{R_l}. \end{aligned}$$

(51)

The last equation is obtained similarly. Using (40d), (40f), (40h), (40i) and (40k) we get

$$\begin{aligned}&P_a - P_r^w = Q_x R_x - Q_{rx} R_{rx} = P_a - P_x + (Q_x - Q_{px} - Q_{xl}) R_{rx} \nonumber \\&\quad = P_a - P_x + \left( \dfrac{P_a-P_x}{R_x} - \dfrac{P_x-P_v}{R_{px}} - \dfrac{P_x-P_l}{R_{xl}}\right) R_{rx}. \end{aligned}$$

(52)

In terms of pressure drops, the equation reads

$$\begin{aligned} - \dfrac{\varDelta P_r^w}{R_{rx}} - \dfrac{\varDelta P_l^r}{R_{xl}} + \left( \dfrac{1}{R_{xl}} + \dfrac{1}{R_x} + \dfrac{1}{R_{px}} + \dfrac{1}{R_{rx}} \right) \varDelta P_x^r = \dfrac{\varDelta P_a}{R_x}. \end{aligned}$$

(53)

Hence, the system of equations results

$$\begin{aligned} \begin{pmatrix} \frac{1}{R_l^{\parallel }} &{} -\frac{1}{R_{rl}} &{} -\frac{1}{R_{xl}}\\ \frac{1}{R_l}+\frac{1}{R_{pl}} &{} \frac{1}{R_{pr}^h} &{} \frac{1}{R_x}+\frac{1}{R_{px}}\\ -\frac{1}{R_{xl}} &{} -\frac{1}{R_{rx}} &{} \frac{1}{R_x^{\parallel }} \end{pmatrix} \begin{pmatrix} \varDelta P_l^r\\ \varDelta P_r^w\\ \varDelta P_x^r \end{pmatrix} \frac{1}{\varDelta P_a} = \begin{pmatrix} \frac{1}{R_l}\\ \frac{1}{R_l}+\frac{1}{R_x}\\ \frac{1}{R_x} \end{pmatrix}, \end{aligned}$$

(54)

where

$$\begin{aligned} \frac{1}{R_l^{\parallel }}=\frac{1}{R_{rl}}+\frac{1}{R_l}+\frac{1}{R_{pl}}+\frac{1}{R_{xl}}. \end{aligned}$$

(55)

1.3 LCX totally occluded

Finally, it is considered $R_x\rightarrow \infty$, leading to $Q_x=0$, and therefore $R_{px}\rightarrow R_{px}^h=\frac{R_{px}}{\text {CFR}}$, with the wedge pressure being $P_x^w$. The formulae for this new circuit becomes

$$\begin{aligned} Q_{px}&= Q_{rx} - Q_{xl}, \end{aligned}$$

(56a)

$$\begin{aligned} Q_{px} R_{px}&= P_x^w - P_v, \end{aligned}$$

(56b)

$$\begin{aligned} Q_l R_l - Q_{xl} R_{xl}&= P_a - P_x^w, \end{aligned}$$

(56c)

$$\begin{aligned} Q_r R_r + Q_{rx} R_{rx}&= P_a - P_x^w, \end{aligned}$$

(56d)

$$\begin{aligned} Q_l R_l&= P_a - P_l , \end{aligned}$$

(56e)

$$\begin{aligned} Q_r R_r&= P_a - P_r , \end{aligned}$$

(56f)

$$\begin{aligned} Q_{pl} R_{pl}&= P_l - P_v , \end{aligned}$$

(56g)

$$\begin{aligned} Q_{pr} R_{pr}&= P_r - P_v , \end{aligned}$$

(56h)

$$\begin{aligned} Q_{rl} R_{rl}&= P_r - P_l , \end{aligned}$$

(56i)

$$\begin{aligned} Q_l&= Q_{pl} - Q_{xl} - Q_{rl}, \end{aligned}$$

(56j)

$$\begin{aligned} Q_r&= Q_{pr} + Q_{rx} + Q_{rl}. \end{aligned}$$

(56k)

Combining (56b) and (56a), and using (56j) and (56k) gives

$$\begin{aligned} \dfrac{P_x^w - P_v}{R_{px}^h} = (Q_{rx} - Q_{xl}) = Q_r + Q_l - Q_{pr} - Q_{pl}. \end{aligned}$$

(57)

Putting (56e), (56f), (56g) and (56h) results

$$\begin{aligned} \dfrac{P_x^w - P_v}{R_{px}^h}= \dfrac{P_a-P_r}{R_r} + \dfrac{P_a-P_l}{R_l} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_l-P_v}{R_{pl}}. \end{aligned}$$

(58)

After some rearrangements

$$\begin{aligned}&\dfrac{P_x^w - P_v}{R_{px}^h}= \dfrac{P_a - P_v}{R_r} - \dfrac{P_r-P_v}{R_r}\nonumber \\&\quad + \dfrac{P_a-P_v}{R_l} - \dfrac{P_l-P_v}{R_l} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_l-P_v}{R_{pl}}. \end{aligned}$$

(59)

Now, we introduce the pressure drops

$$\begin{aligned} \varDelta P_a&= P_a - P_v, \end{aligned}$$

(60)

$$\begin{aligned} \varDelta P_l^x&= P_l - P_v,\end{aligned}$$

(61)

$$\begin{aligned} \varDelta P_r^x&= P_r - P_v, \end{aligned}$$

(62)

$$\begin{aligned} \varDelta P_x^w&= P_x^w - P_v, \end{aligned}$$

(63)

where superscript $(\cdot )^x$ denotes occlusion of the LCX. Thus, using the definition of pressure drops, (59) gives

$$\begin{aligned}&\dfrac{\varDelta P_x^w}{R_{px}^h} + \left( \dfrac{1}{R_l}+\dfrac{1}{R_{pl}}\right) \varDelta P_l^x + \left( \dfrac{1}{R_r}+\dfrac{1}{R_{pr}}\right) \varDelta P_r^x \nonumber \\&\quad = \left( \dfrac{1}{R_r} + \dfrac{1}{R_l}\right) \varDelta P_a. \end{aligned}$$

(64)

The unknowns in this case are $\varDelta {\mathbf {P}}_x=(\varDelta P_l^x, \varDelta P_r^x,\varDelta P_x^w)$. We now use (56c), (56e), (56j), (56g) and (56i) to get the second equation

$$\begin{aligned}&P_a - P_x^w = Q_r R_r + Q_{rx} R_{rx} = P_a - P_r + (Q_r - Q_{pr} - Q_{rl}) R_{rx} \nonumber \\&\quad = P_a - P_r + \left( \dfrac{P_a-P_r}{R_r} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_r-P_l}{R_{rl}}\right) R_{rx}. \end{aligned}$$

(65)

Adding and subtracting $P_v$, and manipulating the equation above

$$\begin{aligned}&- \frac{P_x^w-P_v}{R_{rx}} = - \frac{P_r-P_v}{R_{rx}} + \dfrac{P_a-P_v}{R_r}\nonumber \\&\quad - \dfrac{P_r-P_v}{R_r} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_r-P_v}{R_{rl}} + \dfrac{P_l-P_v}{R_{rl}}. \end{aligned}$$

(66)

Using the definition of pressure drops, we get

$$\begin{aligned} - \dfrac{\varDelta P_x^w}{R_{rx}} + \left( \dfrac{1}{R_{rx}} +\dfrac{1}{R_r} + \dfrac{1}{R_{pr}} + \dfrac{1}{R_{rl}} \right) \varDelta P_r^x - \dfrac{\varDelta P_l^x}{R_{rl}} = \dfrac{\varDelta P_a}{R_r}. \end{aligned}$$

(67)

To obtain the last equation is obtained we employ (56d), (56f), (56h), (56i) and (56k) as follows

$$\begin{aligned}&P_a - P_x^w = Q_r R_r + Q_{rx} R_{rx} = P_a - P_r + (Q_r - Q_{pr} - Q_{rl}) R_{rx} \nonumber \\&\quad = P_a - P_r + \left( \dfrac{P_a-P_r}{R_r} - \dfrac{P_r-P_v}{R_{pr}} - \dfrac{P_r-P_l}{R_{rl}}\right) R_{rx}. \end{aligned}$$

(68)

In terms of pressure drops, the equation reads

$$\begin{aligned} - \dfrac{\varDelta P_x^w}{R_{rx}} - \dfrac{\varDelta P_l^x}{R_{rl}} + \left( \dfrac{1}{R_{rx}} + \dfrac{1}{R_r} + \dfrac{1}{R_{pr}} + \dfrac{1}{R_{rl}} \right) \varDelta P_r^x = \dfrac{\varDelta P_a}{R_x}. \end{aligned}$$

(69)

So, the system of equations can be arranged as follows

$$\begin{aligned} \begin{pmatrix} \frac{1}{R_l^\parallel } &{} -\frac{1}{R_{rl}} &{} -\frac{1}{R_{xl}}\\ -\frac{1}{R_{rl}} &{} \frac{1}{R_r^\parallel } &{} -\frac{1}{R_{rx}}\\ \frac{1}{R_l}+\frac{1}{R_{pl}} &{} \frac{1}{R_r}+\frac{1}{R_{pr}} &{} \frac{1}{R_{px}^h} \end{pmatrix} \begin{pmatrix} \varDelta P_l^x\\ \varDelta P_r^x\\ \varDelta P_x^w \end{pmatrix} \frac{1}{\varDelta P_a} = \begin{pmatrix} \frac{1}{R_l}\\ \frac{1}{R_r}\\ \frac{1}{R_l}+\frac{1}{R_r} \end{pmatrix}. \end{aligned}$$

(70)