1 Introduction

In 1971, the memristor which is considered to be the missing fourth passive circuit element was postulated [1]. However, this important postulation has not caused attention in almost 40 years. Until in 2008, Hewlett-Packard Labs announced the development of a memristor based on nanotechnology [2]. As we know, the memristor takes its place along with the other three existing elements: the resistor, the capacitor, and the inductor. Increasing focus was put on the memristor for its potential applications in programmable logic, signal processing, neural networks, and so on [3].

Moreover, as the novel element, the circuit based on the memristor shares many interesting phenomenon. Recently, the research memristor chaotic circuits have become a focal topic [4–10]. In [4], the author presented a novel fourth-order memristor-based Chua oscillator by replacing Chua’s diode with an active two-terminal circuit. The stabilization problem of a memristor-based chaotic system was investigated in [10]. As the most important phenomenon, the synchronization was also discussed [9]. In [9], the adaptive synchronization problem of memristor-based Chua circuits was investigated.

As time goes on, more and more researchers began to realize the important role of the synchronization time. To attain a high convergence speed, many effective methods have been introduced and finite-time control is one of them. Finite-time synchronization means the optimality in convergence time. Much research work has been done on chaos synchronization based on finite time (see for instance [11–22] and the references therein). However, the finite-time synchronization problem has not been fully investigated in the literature, and it still remains open. Motivated by the above discussion, we investigate the finite-time synchronization problem for a memristor-based Chua circuit. Based on the finite-time stability theory, a novel lemma which guarantees the impulsive system is finite-time stable is presented. Then the impulsive [16–18] adaptive control law is proposed to realize finite-time synchronization of the model considered. Numerical simulations demonstrate the effectiveness and correctness of this results.

The paper is organized as follows. Some preliminaries are presented in the next section. Section 3 proposes the main results of this paper. In Section 4, the numerical simulations are presented, which is followed by the conclusion in Section 5.

2 Preliminaries

In [6], the author proposed a novel nonlinear circuits, with a flux-controlled memristor which replaces the Chua diode. Figure 1 shows a memristor-based Chua oscillator with a flux-controlled memristor.

Figure 1
figure 1

Chua’s memristor-based chaotic circuits with a flux-controlled memristor.

Full size image

Applying a Kirchhoff voltage, the current law, and the volt-ampère relationship of the components, the state equation of a Chua memristor-based chaotic system can be described as follows:

$$ \left \{ \textstyle\begin{array}{@{}l} \dot{u}_{1} = \frac{1}{C_{1}} ( u_{2} - u_{1} + Gu_{1} - W ( \phi )u_{1} ), \\ \dot{u}_{2} = \frac{1}{C_{2}} ( u_{1} - u_{2} ) + Ri_{3}, \\ \dot{i}_{3} = - \frac{1}{L}u_{2} + \frac{r}{L}i_{3}, \\ \dot{\phi} = u_{1}. \end{array}\displaystyle \right . $$
(1)

For convenience, letting \(x_{1} = u_{1}\), \(x_{2} = u_{2}\), \(x_{3} = i_{3}\), \(x_{4} = \phi\), \(\alpha = 1/C_{1}\), \(\beta = 1/L_{1}\), \(\gamma = r/L\), \(\xi = G\), \(C_{2} = 1\), and \(R = 1\), then the model can be rewritten as the following equation:

$$ \left \{ \textstyle\begin{array}{@{}l} \dot{x}_{1} = \alpha ( x_{2} - x_{1} + \xi x_{1} - W ( x_{4} )x_{1} ), \\ \dot{x}_{2} = x_{1} - x_{2} + x_{3}, \\ \dot{x}_{3} = - \beta x_{2} + \gamma x_{3}, \\ \dot{x}_{4} = x_{1}. \end{array}\displaystyle \right . $$
(2)

If we set \(\alpha = 10\), \(\beta = 100 / 7\), \(\gamma = 0.1\), \(\xi = 9 / 7\), \(a = 1 / 7\) and \(b = 2 / 7\), and the initial values are \(( 10^{ - 10},0,0, - 0.515 )\), then system (2) is a chaotic system and the chaotic attractor is shown in Figure 2.

Figure 2
figure 2

The chaotic attractor of the memristor-based Chua circuit.

Full size image

In order to compute simply, letting \(x = [ x_{1},x_{2},x_{3},x_{4} ]^{T}\), then system (2) can be described as follows:

$$ \dot{x} = Ax + \phi ( x ), $$
(3)

where

$$A = \begin{bmatrix} \alpha ( \xi - 1 ) & \alpha & 0 & 0\\ 1 & - 1 & 1 & 0 \\ 0 & - \beta & \gamma & 0 \\ 1 & 0 & 0 & 0 \end{bmatrix},\qquad \phi ( x ) = \begin{bmatrix} - \alpha W ( x_{4} )x_{1} \\ 0 \\ 0 \\ 0 \end{bmatrix}, $$

and \(W ( x_{4} ) = a + 3bx_{4}^{2}\), a, b, γ, ξ, α, and β are positive constants.

Similar to [7], the nonlinear functions \(q ( \phi )\), \(W ( \phi )\) are given by

$$\begin{aligned}& q ( \phi ) = a\phi + b\phi^{3}, \end{aligned}$$
(4)
$$\begin{aligned}& W ( \phi ) = \frac{dq ( \phi )}{d\phi} = a + 3b\phi^{2}. \end{aligned}$$
(5)

Throughout this paper, the following assumption and lemma are necessary for our main results.

Assumption 1

System (2) is a chaotic system, namely, the state is bound, we assume that the following assumptions hold:

$$\vert x_{1} \vert \le M_{1},\qquad \vert x_{4} \vert \le M_{2}, $$

where \(M_{1}\), \(M_{2}\) are real constants.

Lemma 1

Suppose the function is continuous and non-negative when \(t \in [ 0,\infty )\) and satisfies the following conditions:

$$ \left \{ \textstyle\begin{array}{@{}l} \dot{V} ( t ) \le - \rho V^{\eta} ( t ),\quad t \ne t_{k}, \\ V ( t_{k}^{ +} ) \le \delta V ( t_{k} ),\quad k = 1,2, \ldots, \end{array}\displaystyle \right . $$
(6)

where \(\rho > 0\), \(0 < \eta < 1\), \(0 < \delta < 1\), \(k = \{ 1,2,\ldots,m\}\), is a finite natural number set and m is a positive integer, then the following inequality holds:

$$ V^{1 - \eta} ( t ) \le V^{1 - \eta} ( t_{0} ) - \rho ( 1 - \eta ) ( t - t_{0} ),\quad t_{0} \le t \le T, $$
(7)

where T is a constant which represents the setting time.

Proof

Without loss of generality, let \(t_{0} = 0\). In order to prove (7) holds, the following function \(H ( t )\) is constructed:

$$ H ( t ) = V^{1 - \eta} ( t ) - V^{1 - \eta} ( 0 ) + \rho ( 1 - \eta )t. $$
(8)

Clearly, if the function \(H ( t )\) satisfies \(H ( t ) \le 0\), then the equality (7) holds.

One can easily observe that

$$ H ( 0 ) = V^{1 - \eta} ( 0 ) - V^{1 - \eta} ( 0 ) \le 0. $$
(9)

Next, we will prove that \(H ( t ) \le 0\) holds for \(t \in [ t_{0},t_{1} ]\). Otherwise, there exists \(t^{ *}\) such that

$$\begin{aligned}& H ( t ) > 0,\quad t \in \bigl( t^{ *},t_{1} \bigr),\qquad H ( t ) < 0,\quad \quad t \in \bigl( t_{0},t^{ *} \bigr), \end{aligned}$$
(10)
$$\begin{aligned}& H \bigl( t^{ *} \bigr) = 0, \qquad \dot{H} \bigl( t^{ *} \bigr) > 0. \end{aligned}$$
(11)

Combing (10) with (11), one has

$$\begin{aligned} \dot{H} \bigl( t^{ *} \bigr) &= ( 1 - \eta )V^{ - \eta} \bigl( t^{ *} \bigr)\dot{V} \bigl( t^{ *} \bigr) + \rho ( 1 - \eta ) \\ &= ( 1 - \eta )V^{ - \eta} \bigl( t^{ *} \bigr) \bigl( - \rho V^{ - \eta} \bigl( t^{ *} \bigr) \bigr) + \rho ( 1 - \eta ) \\ &= 0 \end{aligned}$$
(12)

which contradicts (11). Namely, \(H ( t ) \le 0\) holds for \(t \in [ t_{0},t_{1} )\).

When \(t = t_{1}\), we get

$$\begin{aligned} H ( t_{1} ) - H \bigl( t_{1}^{ -} \bigr) &= H \bigl( t_{1}^{ +} \bigr) - H \bigl( t_{1}^{ -} \bigr) \\ &= V^{1 - \eta} \bigl( t_{1}^{ +} \bigr) - V^{1 - \eta} \bigl( t_{1}^{ -} \bigr) = \bigl( \beta^{1 - \eta} - 1 \bigr)V^{1 - \eta} \bigl( t_{1}^{ -} \bigr) \\ &\le 0. \end{aligned}$$
(13)

It yields

$$ H ( t_{1} ) \le H \bigl( t_{1}^{ -} \bigr) \le 0. $$
(14)

Then we suppose that \(H ( t ) \le 0\) holds for \(t \in [ t_{k - 1},t_{k} ]\). For \(t \in [ t_{k},t_{k + 1} ]\), we have

$$\begin{aligned} H ( t_{k + 1} ) &= V^{1 - \eta} ( t_{k + 1} ) - V^{1 - \eta} ( 0 ) + \alpha ( 1 - \eta )t_{k + 1} \\ &= \beta^{1 - \eta} V^{1 - \eta} \bigl( t_{k + 1}^{ -} \bigr) - V^{1 - \eta} ( 0 )\alpha ( 1 - \eta )t_{{k + 1}}^{ -} \\ &= \bigl[ \beta^{1 - \eta} - 1 \bigr]V^{1 - \eta} \bigl( t_{k + 1}^{ -} \bigr) - H \bigl( t_{k + 1}^{ -} \bigr) \\ &\le \bigl[ \beta^{1 - \eta} - 1 \bigr]V^{1 - \eta} \bigl( t_{k + 1}^{ -} \bigr) - H \bigl( t_{k}^{ +} \bigr) \\ &\le 0. \end{aligned}$$
(15)

From (8)-(15), we know that \(H ( t ) \le 0\) holds. This completes the proof. □

3 Main results

In this section, the finite-time control and synchronization problems via an impulsive adaptive strategy are investigated, respectively. Taking the impulsive adaptive strategy into account in (3), one obtains

$$ \left \{ \textstyle\begin{array}{@{}l} \dot{x} ( t ) = Ax ( t ) + \phi ( x ( t ) ) + u ( t ),\quad t \ne t_{k}, \\ \Delta x ( t_{k} ) = I_{k} ( x ( t_{k} ) ) = - Bx ( t_{k} ),\quad t = t_{k}, k \in \ell, \end{array}\displaystyle \right . $$
(16)

where \(\Delta x ( t_{k} ) = x ( t_{k}^{ +} ) - x ( t_{k}^{ -} )\), \(x ( t_{k}^{ +} ) = \lim_{t \to t_{k}^{ +}} x ( t )\), \(x ( t_{k}^{ -} ) = \lim_{t \to t_{k}^{ -}} x ( t )\), \(\ell = \{ 1,2, \ldots, n,n_{1}, \ldots, n_{k} \}\), is a finite natural number set. For simplicity, it is assumed that \(x ( t_{k}^{ -} ) = x ( t_{k} )\), which means that \(x ( t_{k} )\) is left continuous. Letting \(u ( t ) = - k_{1}x ( t ) - k_{2}\operatorname{sign} ( x ( t ) )\vert x ( t ) \vert ^{\gamma}\), we have the following theorem.

Theorem 1

Suppose Assumption  1 holds. There exists a positive constant γ satisfying \(0 < \gamma < 1\) such that the memristor-based chaotic system is finite-time stable if the following conditions hold:

  1. (i)

    \(q = \lambda_{\max} [ A^{T} + A - ( 2k_{1} + 1 )I ] < 0\);

  2. (ii)

    \(d = \lambda_{\max} ( I + B )^{T} ( I + B ) < 1\).

Proof

Construct the following Lyapunov candidate function:

$$ V ( t,x ) = x^{T}x. $$
(17)

Calculating the derivative along the trajectory of (16) we have

$$\begin{aligned} D^{ +} V ( t,x ) &= \dot{x}^{T}x + x^{T}\dot{x} \\ &= \bigl( Ax + \phi ( x ) + u ( t ) \bigr)^{T}x + x^{T} \bigl( Ax + \phi ( x ) + u ( t ) \bigr) \\ &= x^{T} \bigl( A + A^{T} - 2k_{1}I \bigr)x + 2x^{T}\phi ( t,x ) - 2k_{2}x^{T}\operatorname{sign} \bigl( x ( t ) \bigr)\bigl\vert x ( t ) \bigr\vert ^{\gamma}. \end{aligned}$$
(18)

From Assumption 1, one has

$$\begin{aligned} 2x^{T}\phi ( x ) &\le x^{T}x + \phi^{T} ( x )\phi ( x ) \\ &\le x^{T}x + \bigl[ \bigl( a + bx_{4}^{2} \bigr)x_{1} \bigr]^{2} \\ &\le x^{T}x + x_{1}^{2} \bigl[ a + bM_{4}^{2} \bigr]^{2} \le ( I + J )x^{T}x, \end{aligned}$$
(19)

where \(J = [ [ a + bM_{4}^{2} ]^{2},0,0,0 ]^{T}\).

From (18) and (19), one obtains

$$\begin{aligned} D^{ +} V ( t,x ) &\le x^{T} \bigl[ A^{T} + A - ( 2k_{1} + 1 )I \bigr]x - 2k_{2}x^{T}\operatorname{sign} \bigl( x ( t ) \bigr)\bigl\vert x ( t ) \bigr\vert ^{\gamma} \\ &\le qV ( t ) - 2k_{2}\sum_{i = 1}^{4} \vert x_{i} \vert ^{\gamma + 1}, \end{aligned}$$
(20)

where \(q = \lambda_{\max} [ A^{T} + A - ( 2k_{1} + 1 )I ]\). From the fact that \(0 < \gamma < 1\), one obtains

$$ \Biggl( \sum_{i = 1}^{4} \vert x_{i} \vert ^{\gamma + 1} \Biggr)^{\frac{1}{\gamma + 1}} \ge \Biggl( \sum _{i = 1}^{4} \vert x_{i} \vert ^{2} \Biggr)^{\frac{1}{2}}. $$
(21)

Also

$$ \sum_{i = 1}^{n} \vert x_{i} \vert ^{\gamma + 1} \ge \Biggl( \sum_{i = 1}^{n} \vert x_{i} \vert ^{2} \Biggr)^{\frac{\gamma + 1}{2}} = \bigl( x^{T}x \bigr)^{\frac{\gamma + 1}{2}}. $$
(22)

Then, from condition (1) of Theorem 1, we have for \(t \ne t_{k}\)

$$ D^{ +} V ( t,x ) \le - 2k_{2}V ( x )^{\frac{\gamma + 1}{2}}. $$
(23)

When \(t = t_{k}\), one obtains

$$\begin{aligned} V \bigl( t_{k}^{ +} \bigr) &= ( x + Bx )^{T} ( x + Bx ) \\ &= x \bigl( I + B^{T} \bigr) ( I + B )x \\ &\le dV ( t_{k} ). \end{aligned}$$
(24)

Through condition in Theorem 1 and Lemma 1, we can easily see that the system (16) is finite-time stable, which complete the proof. □

Next, we investigate the problem of finite-synchronization for a memristor-based chaotic system. Based on the drive-response synchronization concept, letting system (3) be the drive system, the response system with control input u is as follows:

$$ \dot{y} = Ay + \phi ( y ) + u. $$
(25)

Taking the impulsive adaptive effects into account, the response system (25) is as follows:

$$ \left \{ \textstyle\begin{array}{@{}l} \dot{y} = Ay + \phi ( y ) + u ( t ), \quad t \ne t_{k}, \\ \Delta y = I_{k} ( y ) = - Be,\quad t = t_{k}, \\ y ( t_{0}^{ +} ) = y_{0},\quad t_{0} \ge 0, \end{array}\displaystyle \right . $$
(26)

where \(e = [ x - y ]^{T} = [ x_{1} - y_{1},x_{2} - y_{2},x_{3} - y_{3},x_{4} - y_{4} ]^{T}\), \(t_{k}\) are the impulsive instants which satisfy \(t_{1} < t_{2} < \cdots < t_{k - 1} < t_{k}\) and \(\lim_{k \to \infty} t_{k} = \infty\). Letting

$$ u = - k_{1}e ( t ) - k_{2}\operatorname{sign} \bigl( e ( t ) \bigr)\bigl\vert e ( t ) \bigr\vert ^{\gamma}, $$
(27)

where the constants \(k_{1}\), \(k_{2}\) are the control strength coefficients to be designed, the real number γ satisfies \(0 \le \gamma < 1\).

Combing (3) with (26), the error system can be described as follows:

$$ \left \{ \textstyle\begin{array}{@{}l} \dot{e} = Ae + \phi ( e ) + u ( t ), \quad t \ne t_{k}, \\ \Delta e = Be,\quad t = t_{k},k = 1,2, \ldots, \end{array}\displaystyle \right . $$
(28)

where \(\phi ( e ) = [\alpha W ( y_{4} )y_{1} - \alpha W ( x_{4} )x_{1},0,0,0]^{T}\). Hence, we have the following theorem.

Theorem 2

Suppose Assumption  1 holds. There exists a positive constant γ satisfying \(0 < \gamma < 1\) such that the memristor-based Chua systems (3) and (26) can be synchronized under the impulsive adaptive strategy if the following conditions hold:

  1. (i)

    \(A^{T} + A - 2k_{1}I - 2abM_{1}M_{2}I < 0\);

  2. (ii)

    \(d = \lambda_{\max} ( I + B )^{T} ( I + B ) < 1\).

Proof

Construct the following Lyapunov candidate function

$$ V ( e ) = e^{T}e. $$
(29)

For \(t \in [ t_{k},t_{k + 1} )\), the derivative of \(V ( t )\) along the trajectory of (28) is

$$\begin{aligned} D^{ +} V ( e ) = 2e^{T}\dot{e} = 2e^{T} ( A - k_{1}I )e ( t ) + 2e^{T}\phi ( e ) - 2k_{2}e^{T}\operatorname{sign} \bigl( e ( t ) \bigr)\bigl\vert e ( t ) \bigr\vert ^{\alpha}. \end{aligned}$$
(30)

From Assumption 1, we have

$$\begin{aligned} 2e^{T}\phi ( e ) &= 2\alpha \bigl[ \bigl( a + by_{4}^{2} \bigr)y_{1} - \bigl( a + bx_{4}^{2} \bigr)x_{1} \bigr]e_{1} \\ &= - 2\alpha e_{1}^{2} + 2ab \bigl( y_{4}^{2}y_{1} - y_{4}^{2}x_{1} + y_{4}^{2}x_{1} - x_{4}^{2}x_{1} \bigr) \\ &\le 2abM_{1}M_{2}e^{T}e. \end{aligned}$$
(31)

Combing (30) with (31), we have

$$ D^{ +} V ( e ) \le e^{T} \bigl( A^{T} + A - 2k_{1}I - 2abM_{1}M_{2}I \bigr)e - 2k_{2}\sum_{i = 1}^{4} \vert e_{i} \vert ^{\gamma + 1}. $$
(32)

From the fact that \(0 < \gamma < 1\), one obtains

$$ \Biggl( \sum_{i = 1}^{4} \vert e_{i} \vert ^{\gamma + 1} \Biggr)^{\frac{1}{\gamma + 1}} \ge \Biggl( \sum _{i = 1}^{4} \vert e_{i} \vert ^{2} \Biggr)^{\frac{1}{2}}. $$
(33)

Also

$$ \sum_{i = 1}^{n} \vert e_{i} \vert ^{\gamma + 1} \ge \Biggl( \sum_{i = 1}^{n} \vert e_{i} \vert ^{2} \Biggr)^{\frac{\gamma + 1}{2}} = \bigl( e^{T}e \bigr)^{\frac{\gamma + 1}{2}}. $$
(34)

Thus, based on the condition (i) in Theorem 2, we have for \(t \ne t_{k}\)

$$ D^{ +} V ( e ) \le - 2k_{2}V ( e )^{\frac{\gamma + 1}{2}}. $$
(35)

When \(t = t_{k}\), one obtains

$$\begin{aligned} V \bigl( t_{k}^{ +} \bigr) &= ( e + Be )^{T} ( e + Be ) \\ &= e \bigl( I + B^{T} \bigr) ( I + B )e \\ &\le dV ( t_{k} ). \end{aligned}$$
(36)

Through Theorem 2 and Lemma 1, we can easily see that the system (28) is finite-time stable. This implies that the response system (26) is synchronized with the drive system (3) in a finite time. □

4 Simulation results

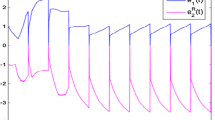
The numerical simulations are carried out using the fourth-order Runge-Kutta method. The initial states of the drive and response systems are \(( 10^{ - 10},0,0,0 )\) and \(( 0,0,0,0 )\). The parameters of the drive systems are \(\alpha = 10\), \(\beta = 100 / 7\), \(\gamma = 0.1\), \(\xi = 9 / 7\), \(a = 1 / 7\), and \(b = 2 / 7\). Solving the inequality in Theorem 2, and choosing \(B = \operatorname{diag}( - 0.9, - 0.9, - 0.9, - 0.9)\), \(k_{1} = 0.02\), \(k_{2} = 0.01\), \(\gamma = 0.3\), the response system synchronizes with the drive system as shown in Figure 3. It is easily shown that the state response curve of the error system is stable.

Figure 3
figure 3

Memristor-based chaotic system under an adaptive impulsive strategy.

Full size image

5 Conclusion

In this paper, the finite-time control and synchronization problems of memristor-based chaotic systems have been investigated. Some novel impulsive adaptive control laws which guarantee the memristor-based Chua circuits is stabilized and synchronized in finite time have been proposed. Moreover, simulation results were given to verify the effectiveness and feasibility of the method. Our future research topics mainly consider the time delay effects on the finite-time stability of the memristor-based nonlinear system.