Dual solutions in MHD flow on a nonlinear porous shrinking sheet in a viscous fluid

Abstract

In this paper, the problem of magnetohydrodynamic (MHD) flow of a viscous fluid on a nonlinear porous shrinking sheet is studied. The boundary layer partial differential equations are first transformed into an ordinary differential equation, which is then solved numerically by the shooting method. The features of the flow for various governing parameters are presented and discussed in detail. It is found that dual solutions only exist for positive values of the controlling parameter.

MSC: 34B15, 76D10.

1 Introduction

Boundary layer flow over a stretching sheet has been studied in various aspects since the pioneering work done by Sakiadis [1]. Lately, many researchers have studied the shrinking sheet boundary layer flow problem due to its important applications in industries which involve packaging process, for example, shrink wrapping. The study on shrinking sheet was first initiated by Wang [2] by considering the stretching deceleration surface. Miklavcic and Wang [3] proved the existence and uniqueness for viscous flow due to a shrinking sheet, and dual solutions were reported for certain range of the suction parameter. Later, Wang [4] also studied the stagnation flow towards a shrinking sheet by considering the two-dimensional and axisymmetric stagnation flows, and dual solutions were also reported only for the two-dimensional case. Further, Sajid et al.[5] and Hayat et al.[6] considered the rotating flow over a shrinking surface. The steady boundary layer flow problems induced by a shrinking sheet can be found in [7–11] in different aspects. On the other hand, the unsteady case is described in papers by Fang et al.[12] and Ali et al.[13, 14]. It is worth mentioning that dual solutions are also found in the papers by Fang [11], Fang et al.[12] and Ali et al.[14].

Recently, Nadeem and Hussain [15] solved analytically the problem of magnetohydrodynamic (MHD) flow of a viscous fluid on a nonlinear porous shrinking sheet using the homotopy analysis method and dual solutions were not reported. Hence, the present paper aims to obtain the dual solutions numerically for the problem considered in Nadeem and Hussain [15] for various controlling parameters and magnetic parameters.

2 Basic equations

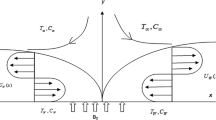
Consider the steady two-dimensional flow of an incompressible electrically conducting fluid towards a nonlinear porous shrinking sheet. The magnetic field $B(x)$ is applied normal to the shrinking and porous sheet. The governing equations of the present problem are

(1)

(2)

where u and v are the velocity components along the x and y directions, respectively, ν is the kinematic viscosity, ρ is the fluid density and σ is the fluid electrical conductivity. The boundary conditions of Eqs. (1) and (2) are

$$\begin{array}{r}u=-c{x}^n,v=-V_0{x}^{(n-1)/2}\text{at }y=0,\\ u=0\text{as }y\to \mathrm{\infty },\end{array}$$

(3)

where $V_0$ is the porosity of the sheet. Following Chiam [16], we assume the external electric field and polarization effects in Eq. (2) are negligible, therefore the magnetic field $B(x)$ is in the form of

$$B(x)=B_0{x}^{(n-1)/2}.$$

(4)

We assume the magnetic Reynolds number is small, so that the induced magnetic field is negligible. Applying the following similarity transformations:

$$\begin{array}{r}u=c{x}^n{f}^{\prime }(\eta ),v=-\sqrt{\frac{c\nu (n+1)}{2}}{x}^{(n-1)/2}[f(\eta )+\frac{n-1}{n+1}\eta f^{\prime }(\eta )],\\ \eta =\sqrt{\frac{c(n+1)}{2\nu }}{x}^{(n-1)/2}y\end{array}$$

(5)

to Eqs. (1) and (2), we obtain the following ordinary differential equation:

$$f^{‴}+f{f}^{″}-\beta f^{\mathrm{\prime }2}-M{f}^{\prime }=0$$

(6)

subject to the boundary conditions

$$f(0)=s,f^{\prime }(0)=-1,f^{\prime }(\mathrm{\infty })=0,$$

(7)

where primes denote differentiation with respect to η, while

$$s=\frac{V_0}{\sqrt{\frac{c\nu (n+1)}{2}}},M=\frac{2\sigma B_0^2}{\rho c(1+n)},\beta =\frac{2n}{n+1}$$

(8)

are the wall mass transfer (suction) parameter, the magnetic parameter and the non-dimensional (controlling) parameter, respectively.

The physical quantities of interest is the skin friction coefficient $C_f$ which is defined as

$$C_f=\frac{2{\tau }_w}{\rho u_w^2},$$

(9)

where the shear stress ${\tau }_w$ is defined as

$${\tau }_w=\mu {\left(\frac{\partial u}{\partial y}\right)}_{y=0}$$

(10)

with μ being the dynamic viscosity. Using (5) and (10), we get

$$R{e}_x^{1/2}{C}_f=\sqrt{2(n+1)}{f}^{″}(0),$$

(11)

where $R{e}_x=u_{w}x/\nu$ is the local Reynolds number.

3 Results and discussion

Equation (6) subject to the boundary conditions (7) has been solved numerically using the shooting method as described in the paper by Meade et al.[17]. This technique is an iterative algorithm which attempts to identify appropriate initial conditions for a related initial value problem (IVP) that provides the solution to the original boundary value problem (BVP). The results of the skin friction coefficient $f^{″}(0)$ obtained in this study are compared with those of Nadeem and Hussain [15], as displayed in Table 1. The agreement between these results is very good. Therefore, we are confident that the present method is accurate.

Table 1 Various values of ${\mathit{f}}^{\mathbf{″}}\mathbf{(}\mathbf{0}\mathbf{)}$ with β when $\mathit{M}\mathbf{=}\mathbf{2}$ and $\mathit{s}\mathbf{=}\mathbf{1}$

Figure 1 shows the variations of the skin friction coefficient $f^{″}(0)$ with the wall mass transfer (suction) parameter s for different values of the controlling parameter β. Dual solutions are found to exist only for positive values of β. It is also found that $f^{″}(0)$ decreases with β. On the other hand, the skin friction coefficient $f^{″}(0)$ increases with s. This is because, physically, suction produces more resistance to the transport phenomena. The critical value of the suction parameter $s_c$ decreases as β increases; therefore, the controlling parameter accelerates the boundary layer separation.

Figure 1

Variation of the skin friction coefficient for different values of β when $\mathit{M}\mathbf{=}\mathbf{0.25}$ .

The variations of the skin friction coefficients with the suction parameter for $\beta =0.5,1.0\text{ and }1.5$ are displayed in Figures 2, 3 and 4, respectively. The skin friction coefficients increase with both the magnetic parameter and the suction parameter. Figures 2 to 4 also show the existence of the dual solutions. The boundary layer separates from the surface at $s=s_c$, and beyond this value, the boundary layer approximation is no longer valid. Therefore, the full Navier-Stokes equation need to be used. Normally, the first solutions are physically stable and this can be verified by performing a stability analysis; see Merkin [18] and Weidman et al.[19]. In Figures 2 to 4, it is found that $s_c$ increases with M. Therefore, we can conclude that the magnetic parameter delays the boundary layer separation from the surface.

Figure 2

Variation of the skin friction coefficient for different values of M when $\mathit{\beta }\mathbf{=}\mathbf{0.5}$ .

Figure 3

Variation of the skin friction coefficient for different values of M when $\mathit{\beta }\mathbf{=}\mathbf{1.0}$ .

Figure 4

Variation of the skin friction coefficient for different values of M when $\mathit{\beta }\mathbf{=}\mathbf{1.5}$ .

Figures 5 and 6 illustrate the dual velocity profiles for various β and M, respectively, with both figures satisfying the boundary conditions (7). This is to prove the dual nature of the present problem. From Figure 5, the boundary layer thickness increases with β. However, the boundary layer thickness decreases as M increases, as displayed in Figure 6. This phenomenon is due to the fact that M creates the Lorentz force which slows down the fluid flow, hence reducing the boundary layer thickness.

Figure 5

Velocity profiles for different values of β when $\mathit{M}\mathbf{=}\mathbf{0.25}$ and $\mathit{s}\mathbf{=}\mathbf{2.4}$ .

Figure 6

Velocity profiles for different values of M when $\mathit{\beta }\mathbf{=}\mathbf{1.5}$ and $\mathit{s}\mathbf{=}\mathbf{2.4}$ .

4 Conclusions

A study is performed for the problem of MHD flow of a viscous fluid on a nonlinear porous shrinking sheet. It is observed that the dual solutions existed only for positive values of the controlling parameter. In this study, we can conclude that the controlling parameter accelerated the boundary layer separation, however, the magnetic parameter delayed the boundary layer separation.