1 Introduction

The applicability and manipulation of electronic and nonlinear optical properties of quantum systems are important for optoelectronic device technology. The development of optical nonlinearity of nanomaterials is an active research topic with important implications in systems such as integrated nonlinear optics, optical signal processing, ultrafast switching and biological imaging [1,2,3,4,5,6]. Especially in low-dimensional heterostructures based on semiconductors, obtaining significant nonlinear optical effects due to the quantum confinement of the carrier shows great superiority in the construction of nonlinear optical devices [7,8,9,10,11,12,13,14]. This fact makes it important to study on the determination of electronic and nonlinear optical properties in quantum systems based on semiconductor nanostructures.

As is known, second-order nonlinear optical effects are closely related to the symmetry of the quantum system. The nonlinear optical effects resulting from the lack of inversion symmetry are important for many applications from classical to quantum regimes [7, 15]. Besides designing the asymmetric geometric confinement potential, the inversion symmetry in low-dimensional semiconductor quantum systems can be broken by externally applied field effects or the configuration of impurity atoms confined in the quantum systems. Nonlinear optical rectification (NOR) has received more attention due to the larger magnitudes of second-order nonlinear susceptibility compared to higher-order ones. Recently, many studies have been performed on the second-order optical nonlinearities in asymmetric quantum dots (QDs) in the presence of an electromagnetic field. For example, Fejer et al. observed extremely large quadratic sensitivity in AlGaA quantum wells under the influence of electric field [16]. On the other hand, the effects of applied magnetics and electric fields on the optical rectification of a disk-like QD in the presence of an impurity atom have been investigated by Soltani-Vala et al. [14]. Lui et al. theoretically investigated the nonlinear optical rectification coefficient in laterally coupled quantum well wires with an applied electric field are using the effective mass approximation [15]. They emphasized that the NOR is greatly influenced by the electric field as well as both the distance and the radius of the coupled system. The NOR of a hydrogenic impurity in an asymmetric lens-shaped quantum dot calculated by Ahadi et al. using the compact density matrix approach and an iterative method [17]. Their results showed that the NOR increases with decreasing the reflection symmetry of the quantum dot and the peak position of the NOR shifts to lower photon energies as the size of the quantum dot is increased.

It is expected that high nonlinear optical responses will be observed in semiconductor systems where the freedom of movement of charge carriers is restricted in two directions [15, 18,19,20]. In this context, the nonlinear optical effects exhibited by disk-like quantum dots will also be interesting for the design and development of nonlinear photonic devices in the future [12, 21]. Undoubtedly, doping these systems can provide significant improvements in their electronic and nonlinear optical effects. Rezaei et al. emphasized that hydrogenic impurity increases the transition energy and hence, the resonant peak of NOR experiences an obvious blueshift in their study [22]. Also, they noted that the presence of impurity leads to the increment in the magnitude of the NOR at strong confinement regimes. In addition, Baskoutas et al. in their study investigating the electronic structure of a spherical quantum dot with parabolic confinement that contains a hydrogenic impurity and is subjected to an electric field, it was shown that both the position of the impurity and the strength of the electric field affect the electronic structure of the system and the nonlinear optical correction process [23].

Recently, research on the electronic and optical properties of a hydrogen molecular ion (\(D_{2}^{ + }\)) in semiconductor nanostructures is increasing rapidly [24,25,26]. One of the main advantages of studying the \(D_{2}^{ + }\) complex confined in low-dimensional heterostructures is that it allows for the investigation of the fundamental properties of quantum confinement and quantum coherence in a control manner. This is particularly important in the development of nanoscale devices and technologies that rely on the manipulation of quantum states. In this context, the \(D_{2}^{ + }\) molecular complex is considered a promising candidate for single-qubit operations, where the main driving mechanisms for coherent electron operation are the usage of external electric fields changing the geometric confinement potential and resonant pulses controlling optical dipole transitions [27, 28]. Furthermore, the confinement can enhance the nonlinear optical response of the \(D_{2}^{ + }\) ion, leading to an increased efficiency of the nonlinear optical effects. Also, it should be emphasized that nanoscale structures including molecules exhibits enhanced nonlinear optical properties, such as higher-order nonlinearities and ultrafast response times [29]. Due to these properties, they have a high potential for applications in data storage, signal processing and sensing [30,31,32].

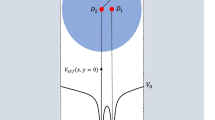
Despite the number of theoretical studies on singly ionized double-donor systems confined in QDs, there are no reports of the effects of anisotropy on nonlinear optical rectification and electronic structure in a quantum dot with a \(D_{2}^{ + }\) molecular complex. In this context, it would be meaningful to investigate the effects of anisotropy and the distance between impurity atoms on the energy spectrum and NOR for a \(D_{2}^{ + }\) complex confined in a Gaussian QD, schematically given in Fig. 1. The format of the article is as follows: the theoretical framework and the details of the calculation are presented in Sect. 2. The numerical results and discussion are given in Sect. 3. Finally, a concise conclusion is given in in Sect. 4.

Fig. 1
figure 1

A representation of the QD system under consideration along the \(xy\) plane. \(R\) is the effective QD size, \(D_{1}\) and \(D_{2}\) denote the first and second donor impurity atoms, respectively

Full size image

2 Theoretical framework

The system we examine in this work consists of a QD with a Gaussian confinement potential containing two positive charge centers interacting with a conduction band electron called the \(D_{2}^{ + }\) molecular complex. The QD system containing the hydrogen molecular ion under consideration consists of GaAs material embedded in AlGaA material. We assume the donor impurity atoms are located at: \(\vec{r}_{{D_{1} }} = x_{1} \hat{x} + y_{1} \hat{y}\) and \(\vec{r}_{{D_{2} }} = x_{2} \hat{x} + y_{2} \hat{y}\). Within the effective mass approximation, the time-independent Schrödinger equation for the hydrogen molecular ion confined in the QD is:

$$\left[ { - \frac{{\hbar^{2} }}{{2m^{*} }}\left( {\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\partial^{2} }}{{\partial y^{2} }}} \right) + V\left( {x,y} \right) + V_{C} + V_{DD} } \right]\psi_{n} \left( {x,y} \right) = E_{n} \psi_{n} \left( {x,y} \right)$$
(1)

where \(m^{*}\) is the electron effective mass, \(V\left( {x,y} \right)\) is the confining potential, \(V_{C}\) is the Coulomb potential between the electron and impurity atoms and \(V_{DD}\) is the repulsive interaction potential between impurity atoms. The confinement potential of the two-dimensional Gaussian QD structure is [33, 34]:

$$V\left( {x,y} \right) = V_{0} \left( {1 - e^{{ - \left( {x^{2} /L_{x}^{2} + y^{2} /L_{y}^{2} } \right)}} } \right)$$
(2)

In Eq. (2), \(L_{x}\) and \(L_{y}\) are the effective strength of the two-dimensional QD in the \(x\) and \(y\) direction, respectively. The asymmetry of the QD is defined as \(\eta = L_{y} /L_{x}\) and effective radius of the structure is described by the parameter \(R = \sqrt {L_{x} L_{y} }\). It should be noted that in the case of \(\eta = 1\) the two-dimensional QD structure has circular symmetry.

The electron–donor interaction is given as follows:

$$V_{C} = \mathop \sum \limits_{i = 1}^{2} \frac{{ - e^{2} }}{{4\pi \varepsilon \sqrt {\left( {x - x_{i} } \right)^{2} + (y - y_{i} )^{2} } }}$$
(3)

The interaction between donor atoms is given as:

$$V_{DD} = \frac{{e^{2} }}{{4\pi \varepsilon \sqrt {\left( {x_{1} - x_{2} } \right)^{2} + (y_{1} - y_{2} )^{2} } }}$$
(4)

For the sake of the simplicity, the positions of the donors are given as \(\vec{r}_{{D_{1} }} = \left( {x_{1} ,0} \right)\) and \(\vec{r}_{{D_{2} }} = \left( {0,0} \right)\). Thus, the internuclear distance between the donors is \(D = \left| {\vec{r}_{{D_{2} }} - \vec{r}_{{D_{1} }} } \right| = x_{1}\). The energy eigenvalues (\(E_{n}\)) and corresponding eigenfunctions (\(\psi_{n}\)) of the resulting eigenvalue equation are obtained using the adiabatic approach and the two-dimensional diagonalization method as shown in Ref. [35]. The wave functions are expanded in a set of eigenfunctions of the rectangle quantum wells with infinite potential barriers, written as

$$\psi_{n} \left( {x,y} \right) = \mathop \sum \limits_{{n_{x} n_{y} }} C_{{n_{x} ,n_{y} }} \varphi_{{n_{x} ,n_{y} }} \left( {x,y} \right)$$
(5)

where

$$\varphi_{{n_{x} ,n_{y} }} \left( {x,y} \right) = \frac{2}{{\sqrt {{\mathcal{L}}_{x} {\mathcal{L}}_{y} } }}\sin \left[ {n_{x} \pi \left( {\frac{x}{{{\mathcal{L}}_{x} }} + \frac{1}{2}} \right)} \right]\sin \left[ {n_{y} \pi \left( {\frac{y}{{{\mathcal{L}}_{y} }} + \frac{1}{2}} \right)} \right].$$
(6)

In these expressions, the parameters \({\mathcal{L}}_{x}\) and \({\mathcal{L}}_{y}\) are the width of the infinite potential well along \(x\) and \(y\) direction, respectively. For a more realistic approach, the dimensions \({\mathcal{L}}_{x}\) and \({\mathcal{L}}_{y}\) should be chosen appropriately larger than the QD dimension. For this purpose, we used a set of sinusoidal functions in a region \({\mathcal{L}}_{x} = {\mathcal{L}}_{y} = {\mathcal{L}} = 80 \;{\text{nm}}\) with quantum numbers \(n_{x}\) and \(n_{y}\) both running from \(1\) to \(25\) leading to \(625\) terms in the wave function expansion, which are sufficiently large to obtain results with an accuracy of \(0.02\;{\text{ meV}}\) for each energy value. After sorting the resulting set of energy states in order of increasing energy, we used eigenfunctions \(\psi_{n} \left( {x,y} \right)\) corresponding to the first 400 states with quantum number \(n\) to diagonalize the Hamiltonian. It should be noted here that each quantum number \(n\) consists of a combination of \(n_{x}\) and \(n_{y}\) quantum numbers. By substitution Eq. (5) into Eq. (1) and integrating the resulting equation over the entire unit cell size, we can obtain the following secular matrix equation:

$$H_{nm} = E\delta_{nm}$$
(7)

where

$$\begin{aligned} H_{nm} & = - \frac{{\hbar^{2} \pi^{2} }}{{2m^{*} {\mathcal{L}}^{2} }}\left( {n_{x}^{2} + n_{y}^{2} } \right)\delta_{nm} + V_{0} \delta_{nm} - V_{0} \mathop \int \limits_{{ - \frac{{\mathcal{L}}}{2}}}^{{ + \frac{{\mathcal{L}}}{2}}} \mathop \int \limits_{{ - \frac{{\mathcal{L}}}{2}}}^{{ + \frac{{\mathcal{L}}}{2}}} \varphi_{{n_{x} ,n_{y} }} e^{{ - \left( {x^{2} /L_{x}^{2} + y^{2} /L_{y}^{2} } \right)}} \varphi_{{n_{x}^{\prime} n_{y}^{\prime} }} dxdy \\ & \quad + \frac{1}{4\pi \varepsilon }\mathop \int \limits_{{ - \frac{{\mathcal{L}}}{2}}}^{{ + \frac{{\mathcal{L}}}{2}}} \mathop \int \limits_{{ - \frac{{\mathcal{L}}}{2}}}^{{ + \frac{{\mathcal{L}}}{2}}} \varphi_{{n_{x} ,n_{y} }} \left( {\mathop \sum \limits_{i = 1}^{2} \frac{{ - e^{2} }}{{\sqrt {\left( {x - x_{i} } \right)^{2} + (y - y_{i} )^{2} } }}} \right)\varphi_{{n_{x}^{\prime} n_{y}^{\prime} }} dxdy \\ \end{aligned}$$
(8)

where \(n = \left( {n_{x} ,n_{y} } \right)\), \(m = \left( {n_{x}^{\prime} ,n_{y}^{\prime} } \right)\), and \(n,\;m = 1, 2, \ldots , 400.\) The third and fourth terms are calculated numerically. In this study, we used Wolfram Mathematica software for numerical calculations. It should be noted that the interaction between donor atoms is not included in Eq. (8) because, as is known, this term (\(V_{DD}\)) has no effect on electron binding. It should be taken into account when calculating the total energy of the system [24].

As is known, the compact density matrix formalism for the transition between two energy levels is a valuable framework for explaining the interaction between monochromatic light field and nanostructures under steady-state conditions [36, 37]. In numerical calculations, we assume that the system is under the influence of an \(x\)polarized electromagnetic radiation \(E\left( t \right) = Ee^{ - i\omega t} + E^{*} e^{i\omega t}\). By means of the compact density matrix approach and the iterative procedure as Refs. [35, 38], the NOR \(\chi_{0}^{\left( 2 \right)}\) can be written as:

$$\chi_{0}^{\left( 2 \right)} = \frac{{4e^{3} \rho_{s} M_{12}^{2} \left| {M_{22} - M_{11} } \right|\left[ {\omega_{12}^{2} \left( {1 + {\Gamma }_{2} /{\Gamma }_{1} } \right) + \left( {\omega^{2} + {\Gamma }_{2}^{2} } \right)\left( {{\Gamma }_{2} /{\Gamma }_{1} - 1} \right)} \right]}}{{\varepsilon_{0} \hbar^{2} \left[ {\left( {\omega_{12} - \omega } \right)^{2} + {\Gamma }_{2}^{2} } \right]\left[ {\left( {\omega_{12} + \omega } \right)^{2} + {\Gamma }_{2}^{2} } \right]}}$$
(9)

where \(M_{12} = \left| {\psi_{1} {|}x{|}\psi_{2} } \right|\) and \(M_{ii} = \left| {\psi_{i} {|}x{|}\psi_{i} } \right|\) (\(i = 1, 2\)) are the transition matrix elements, \(E_{12} = E_{2} - E_{1}\) is the energy difference between ground and first excited states, \(\omega_{12} = E_{12} /\hbar\), \(e\) is the electron charge, \(\varepsilon_{0}\) is the vacuum permittivity, \(\rho_{s}\) is the density of electrons, \(\omega\) is the angular frequency of the incident photon, \(\psi_{1}\)(\(\psi_{2}\)) are the ground (first-excited) state eigenfunctions and \({\Gamma }_{1}\)(\({\Gamma }_{2}\)) are the diagonal (off-diagonal) relaxation rates. The maximum value of \(\chi_{0}^{\left( 2 \right)}\) can be observed at the resonance condition \(\omega = \omega_{12}\) which is:

$$\chi_{{0, {\text{max}}}}^{\left( 2 \right)} = \frac{{4e^{3} \rho_{s} M_{12}^{2} \left| {M_{22} - M_{11} } \right|}}{{\varepsilon_{0} \hbar^{2} {\Gamma }_{1} {\Gamma }_{2} }}$$
(10)

3 Results and discussion

At this stage, we present numerical results on the effects of anisotropy and QD size on the energy levels and NOR of a molecular complex confined in the QD. The parameters used for obtaining the numerical results are: \(\varepsilon_{0} = 8.85 \times 10^{ - 12} \;{\text{C/Vm}}\), \(V_{0} = 228\; {\text{meV}}\), \({\Gamma }_{1} = 1/1\; {\text{ps}}\), \({\Gamma }_{2} = 1/0.2\; {\text{ps}}\), \(\rho_{s} = 5 \times 10^{24} /{\text{m}}^{{3}}\), \(\varepsilon = 12.85\) and \(m* = 0.067m_{0}\), where \(m_{0}\) is the free electron mass. These physical parameters are widely used for GaA-based heterostructures [39].

Examining the wave function behavior for a general understanding of the combined effect of anisotropy and internuclear distance on carrier confinement will also contribute to the following explanations. In the NOR expression given in Eq. (9), \(1 \to 2\) or \(1 \to 3\) optical transitions are allowed, depending on the polarization of the incident photon field. For this purpose, the probability densities corresponding to the first three energy levels are given for different anisotropy and internuclear distance values in Fig. 2. As can be clearly seen from the figure, in general the wave function corresponding to the ground state (\(\psi_{1}\)) exhibits full axial symmetry, while the wave functions representing the \(E_{2}\) and \(E_{3}\) states (\(\psi_{2}\) and \(\psi_{3}\)) are aligned along the \(x\)- or \(y\)-axes, depending on whether the QD is in prolate or oblate shape, respectively. Therefore, depending on the geometric shape of the QD, the variation of the wave functions corresponding to the \(E_{2}\) and \(E_{3}\) energy levels with the anisotropy parameter \(\eta\) differs as expected. The main reason for this is that in the oblate case (\(\eta < 1\)), the effective size of the system along the \(x\)-axis (\(y\)-axis) \(L_{x}\) (\(L_{y}\)) increases (decreases) with the anisotropy parameter, and in the prolate case, the opposite behavior occurs. Detailed explanations regarding the analysis of wave functions will be given while focusing on the effects of anisotropy on energy levels and NOR.

Fig. 2
figure 2

The contour plots of the electron probability densities corresponding to the first three energy levels for different values of the anisotropy parameter, a \(\eta = 0.2, \;0.4, \;0.6, \;1\) and b \(\eta = 1, \;1.4,\; 1.8, \;2\). The results are presented for \(R = 5 \;{\text{nm}}\) and \(D = 1 \;{\text{nm}}\)

Full size image

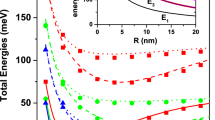
In Figs. 3 and 4, we show the variation of the first three energy states of the \(D_{2}^{ + }\) complex confined in a two-dimensional QD as a function of the anisotropy parameter for \(R = 5, \;10, \;20{\text{ nm}}\) and \(D = 1\; {\text{nm}}\) and \(D = 5\;{\text{ nm}}\), respectively. It is clearly seen from Fig. 3 that in the case of nearly circularly symmetric QD (\(\eta \cong 1\)), the ground-state energy (\(E_{1}\)) shows a minimum, being an increasing function of the anisotropy. Because in this case where the donor atoms are very close to each other (\(D = 1 \;{\text{nm}}\)), the QD for \(\eta \cong 1\) indeed has almost circular symmetry. It should also be emphasized that when the two-dimensional system has circular symmetry, the geometric confinement is weaker compared to oblate (\(\eta < 1\) ) or prolate (\(\eta > 1\)) QD cases. This feature explains why the ground-state energy is minimal in the case where the system is almost circularly symmetric. It is also observed that the anti-crossing between the \(E_{2}\) and \(E_{3}\) energy levels takes place at a critical value of the anisotropy parameter \(\eta_{c}\) for all considered values of QD size \(R\). As seen in Fig. 3, for \(R = 5 \;{\text{nm}}\), the anti-crossing point is observed at \(\eta_{c} = 1.02\), while for \(R = 10\) and \(20\;{\text{ nm}}\), it occurs at \(\eta_{c} = 1.04\). The physical explanation for the occurrence of anti-crossing at values of the anisotropy parameter slightly larger than 1 can be given as follows: although the donor atoms are very close to each other (\(D = 1 \;{\text{nm}}\)), the first donor atom \(D_{ 1}\) positioned at \(x_{1}\) slightly distorts the circular symmetry of the system. The QD needs to be slightly compressed along the \(x\)-axis for the system to become circularly symmetric again (i.e., the anisotropy parameter should be \(\eta > 1\)). When this condition is met at \(\eta = \eta_{c}\), the \(E_{2}\) and \(E_{3}\) energy levels tend to cross with each other, but the energy levels cannot cross each other due to their mutual coupling [40]. To ensure that it is really the case of energy levels anti-crossing, the enlarged graph containing the density functions of the region enclosed in the rectangular symbol in Fig. 3 is shown in Fig. 5. It is clearly seen in Fig. 5 that beyond the anti-crossing region the density functions \(\left| {\psi_{2} } \right|^{2}\) and \(\left| {\psi_{3} } \right|^{2}\) completely exchanged their symmetries, without mixing. This behavior is a characteristic of energy level anti-crossing [40,41,42,43]. On the other hand, in the case of \(\eta < 1\), where the system turns into an oblate QD, the ground state increases as the anisotropy parameter decreases, while the degeneracy of the first two excited states disappears as a result of the loss of axial symmetry, leading to the splitting of these levels. This splitting first increases as the anisotropy parameter decreases and starts to decrease after a certain value of the parameter \(\eta\). First of all, we can explain that the ground state increases with the decrease of \(\eta\) as follows: In the case of \(\eta < 1\), while the effective width of the QD along the \(x\)-axis \(L_{x}\) increases with the anisotropy parameter, its effective width along the \(y\)-axis \(L_{y}\) decreases. For example, for \(R = 5 \;{\text{nm}}\), at \(\eta = 0.2\), \(L_{x} = 11.18 \;{\text{nm}}\) while \(L_{y} = 2.23 \;{\text{nm}}\). Therefore, in this limit, the two-dimensional QD becomes an almost one-dimensional system with increasingly smaller \(L_{y}\) and larger \(L_{x}\). Thus, in the considered limit (at sufficiently small values of \(\eta\)), the geometric confinement is quite strong compared to the two-dimensional QD, and as a result an increase in the ground-state energy is observed. This is an important conceptual point because it shows how anisotropy controls the dimension and energy levels of the QD structure with molecular ion. On the other hand, since the wave functions corresponding to the \(E_{2}\) and \(E_{3}\) states are aligned along the \(x\)-axis and \(y\)-axis, respectively, the variation of these states with anisotropy depends predominantly on the anisotropy-induced change of \(L_{x}\) and \(L_{y}\). In the case of oblate QD, as mentioned above, decreasing the anisotropy parameter values increases (decreases) the effective size of the QD in the \(x\)-direction (\(y\)-direction) characterized by the expansion (contraction) of the wave functions in the \(x\)-direction (\(y\)-direction). This behavior is seen more clearly, especially in the evolution of the wave functions corresponding to the \(E_{1}\) and \(E_{2}\) energy levels according to the anisotropy parameter (see Fig. 2a). As a result of this change in the size of the QD, the \(E_{2}\) (\(E_{3}\)) energy level decreases (increases) until a certain value of the anisotropy parameter (\(\eta \cong 0.4\)), and the reverse behavior is observed for sufficiently small values of \(\eta\). This feature is especially evident at small \(R\) values (see Figs. 3a and 4a). When the evolution of wave functions corresponding to these energy levels in Fig. 2a is examined, it is seen that after a critical \(\eta\) value (\(\eta \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{ < } 0.4\)), there is a significant increase in the extension of \(\psi_{2}\) along the \(x\)-axis, and the \(\psi_{3}\) function, which was initially aligned along the \(y\)-axis, is also aligned along the \(x\)-axis. This result explains that the change of \(E_{2}\) and \(E_{3}\) energy levels according to the anisotropy parameter is mainly a result of the change in geometric confinement. Another feature that should be emphasized here is that if the distance between the donor atoms is relatively large (\(D = 5 \;{\text{nm}}\)), and the anti-crossing point between the \(E_{2}\) and \(E_{3}\) states is observed at larger \(\eta\) values (see Fig. 4). This can be attributed to the greater distortion of the symmetry of the system as the distance between nuclei increases. For example, while \(\eta_{c} \cong 1\) for all \(R\) values considered for \(D = 1 \;{\text{nm}}\), in the case of \(D = 5 \;{\text{nm}}\), \(n_{c} = 1.37\) for \(R = 5 \;{\text{nm}}\), \(n_{c} = 1.44\) for \(R = 10 \;{\text{nm}}\) and \(n_{c} = 1.71\) for \(R = 20\;{\text{nm}}\). Finally, the effect of dimensionality on electronic confinement, especially in the case of \(\eta > 1\) where \(L_{x} < L_{y}\), is clearly visible from the results shown in Figs. 3 and 4. As seen in these figures, due to the exchange in the symmetry of the relevant wave functions, the energy level \(E_{2}\) (\(E_{3}\)) decreases (increases) linearly with the anisotropy parameter after the anti-crossing point for all considered values of \(D\) and \(R\). In fact, this behavior is a result of the change in geometric confinement size being inversely proportional to the change in energy levels as known. It should be noted that as a result of the exchange in the wave function symmetry after anti-crossing point the wave functions \(\psi_{2}\) and \(\psi_{3}\) become aligned along the \(y\) and \(x\)-axes, respectively (see Fig. 2b).

Fig. 3
figure 3

Change of the lowest three energy states as a function of the anisotropy parameter for a \(R = 5 \;{\text{nm}}\), b \(R = 10 \;{\text{nm}}\) and c \(R = 20 \;{\text{nm}}\). The results are presented for \(D = 1 \;{\text{nm}}\)

Full size image
Fig. 4
figure 4

Change of the lowest three energy states as a function of the anisotropy parameter for a \(R = 5 \;{\text{nm}}\), b \(R = 10 \;{\text{nm}}\) and c \(R = 20 \;{\text{nm}}\). The results are presented for \(D = 5 \;{\text{nm}}\)

Full size image
Fig. 5
figure 5

Enlarged graph of the region enclosed by a rectangle in Fig. 3a, with the plots of the squared wave functions for selected states around the anti-crossing region

Full size image

Figure 6 shows the NOR max value of the molecular complex \(D_{2}^{ + }\) as a function of the anisotropy parameter \(\eta\) for \(R = 5\), \(10\) and \(20 \;{\text{nm}}\). Moreover, since the peak value of the NOR is proportional to \(M_{12}^{2} \left| {M_{22} - M_{11} } \right|\) as seen in the definition of \(\chi_{{0,{\text{max}}}}^{\left( 2 \right)}\) given in Eq. (10), the evolution of the terms \(M_{11} ,\) \(M_{22}\), \(M_{12}^{2}\) and \(\left| {M_{22} - M_{11} } \right|\) as a function of the anisotropy parameter for \(R = 5, \;10 \;{\text{and}}\; 20{\text{ nm}}\) is given in Fig. 7. According to the results obtained in the figure, the anisotropy and the geometric size of the system have a significant effect on the NOR of the molecular complex. In order to explain the effect in question in detail, it will be useful to analyze the change of probability densities corresponding to the ground and first excited energy levels for different values of the anisotropy parameter and QD size. For this purpose, the probability densities corresponding to the ground and first excited state are given in Figs. 8 and 9 for different \(\eta\) and \(R\) values. It is clearly seen from Fig. 6 that the NOR max value \(\chi_{{0,{\text{max}}}}^{\left( 2 \right)}\) increases as the anisotropy parameter decreases in the case of \(\eta < 1\) for \(R = 5 \;{\text{nm}}\). In this case, since the probability densities corresponding to the ground and first excited states are mostly aligned along the \(x\)-axis, the probability densities expand along this axis as \(L_{x}\) increases with decreasing \(\eta\) values (see Fig. 2a). Thus, the terms \(M_{12}^{2}\) and \(\left| {M_{22} - M_{11} } \right|\), to which the peak value of the NOR is proportional, increase with decreasing the anisotropy (see Fig. 7b and c). However, the behavior differs in the curves corresponding to \(R = 10\) and \(20{\text{ nm}}\). The main reason for this difference is that as the QD size increases, the sensitivity of first excited state probability density \(\left| {\psi_{2} } \right|^{2}\) to the anisotropy parameter is greater and its extension along the \(x\)-axis is larger compared to the case of \(R = 5 \;{\text{nm}}\) (see Figs. 8a and a). In addition, as seen in Fig. 7a, especially at large QD sizes (\(R = 20 \;{\text{nm}}\)), there is no significant change in the \(M_{11}\) term, while the amplitude of the \(M_{22}\) term rapidly approaches zero as \(\eta\) decreases, resulting in a reduction in the maximum value of NOR. On the other hand, in the case of prolate QD (\(\eta > 1\)), for \(R = 5 \;{\text{nm}}\) and \(R = 10\;{\text{ nm}}\), \(\chi_{{0,{\text{max}}}}^{\left( 2 \right)}\) starts to decrease with increasing \(\eta\) values and then starts to increase after a certain value (\(\eta \cong 1.5\)). However, for \(R = 20 \;{\text{nm}}\), \(\chi_{{0,{\text{max}}}}^{\left( 2 \right)}\) increases continuously with \(\eta\). This behavior can again be clarified by wave function analysis. In that case, analyzing Figs. 2b, 8b, and 9b one can conclude that while the ground state density expands along the y-axis as \(\eta\) increases, the first excited state density, which has \(p_{x}\)-symmetry at \(\eta = 1\), acquires a \(p_{y}\)-symmetric character around \(\eta \approx 1.4\). As a result of this exchange, the \(M_{22}\) term begins to reach large negative values around \(\eta \approx 1.5\), as observed in Figure 7a. Comparing Figs. 2b, 8b and 9b, we note that in the prolate QD case the symmetry of the probability density corresponding to the first excited state breaks more and more and spread over a larger region with the \(\eta\) parameter as the QD size increases. As a result of this fact, it is clear from Fig. 6 that the NOR maximum will be larger for a prolate QD with a large geometric size compared to the oblate QD case. Because the integral range contributing to the matrix elements where NOR is directly proportional is larger. It should be emphasized that the increase in \(\chi_{{0,{\text{max}}}}^{\left( 2 \right)}\) in the case of high asymmetry of the system is consistent with previous studies [17, 35].

Fig. 6
figure 6

Change of the NOR max value \(\chi_{{0,{\text{max}}}}^{\left( 2 \right)}\) as a function of the anisotropy parameter for different values of QD size, \(R = 5\), \(10\), \(20 \;{\text{nm}}\) and \(D = 1 \;{\text{nm}}\) and \(D = 5 \;{\text{nm}}\)

Full size image
Fig. 7
figure 7

Variation of the terms a \(M_{ii} \left( {i = 1, 2} \right)\), b \(M_{12}^{2}\) and c \(\left| {M_{22} - M_{11} } \right|\) as a function of the anisotropy parameter for \(R = 5, \;10\; {\text{and}}\; 20 \;{\text{nm}}\) and \(D = 1 \;{\text{nm}}\)

Full size image
Fig. 8
figure 8

The contour plots of the electron probability densities corresponding to the first two energy levels for different values of the anisotropy parameter, a \(\eta = 0.2, 0.4, 0.6, 1\) and b \(\eta = 1, 1.4, 1.8, 2\). The results are presented for \(R = 10 \;{\text{nm}}\) and \(D = 1 \;{\text{nm}}\)

Full size image
Fig. 9
figure 9

Contour plots of the electron probability densities corresponding to the first two energy levels for different values of the anisotropy parameter, a \(\eta = 0.2, \;0.4, \;0.6, \;1\) and b \(\eta = 1, \;1.4, \;1.8, \;2\). The results are presented for \(R = 20 \;{\text{nm}}\) and \(D = 1 \;{\text{nm}}\)

Full size image

In addition, another important aspect regarding NOR analysis is determining the asymmetry dependence of the peak position of NOR, which is determined by the energy difference \(E_{12}\). The evolution of the energy difference \(E_{12}\) as a function of the anisotropy parameter is given in Fig. 10 for different values of internuclear distance \(D\) and QD size \(R\). In the case of prolate (oblate) QD, it can be observed that the energy difference \(E_{12}\) increases (decreases) as the asymmetry of the system increases, indicating a blueshift (redshift) of the NOR. The variation of the energy levels \(E_{1}\) and \(E_{2}\) as a function of the anisotropy parameter clarifies the evolution of the energy difference \(E_{12}\) with respect to the symmetry of the system. As seen in Fig. 3, since the rate of increase of the ground-state energy value according to the \(\eta\) parameter is quite different in prolate and oblate QD cases, the change of \(E_{12}\) also differs depending on the asymmetry of the system, as expected. This behavior can be considered as a new degree of freedom in tuning the NOR of the molecular complex confined in the QD to suit the purpose in the field of application. Furthermore, the energy difference decreases as the QD size increases, for all \(\eta\) and \(D\) values considered. Also, in line with this result, it can be observed that the energy difference \(E_{12}\) decreases as the distance between the nuclei \(D\) increases, for all \(R\) values considered. As the distance between the nuclei increases, the barrier height between the Coulomb centers increases and the effective confinement width decreases, resulting in an increment in the energy difference \(E_{12}\).

Fig. 10
figure 10

Variation of the energy difference \(E_{12}\) as a function of the anisotropy parameter for \(R = 5, \;10\;{\text{and}}\;20 \;{\text{nm}}\) and \(D=1\;{\text{nm}}\) and \( D = 5 \;{\text{nm}}\)

Full size image

4 Conclusion

In this paper, the impact of the anisotropy, internuclear distance and QD size on the low-lying energy levels and nonlinear optical rectification of a hydrogen molecular ion confined in a quantum dot were investigated. The numerical results are obtained using the two-dimensional diagonalization method within the effective mass approximation. The results show how the asymmetry of the system controls the dimension, energy levels and nonlinear optical rectification of the QD structure containing a molecular complex. We conclude that the evolution of the energy spectrum depends predominantly on the anisotropy-induced change of geometric confinement size. Another important result we obtained is that the peak position of the nonlinear optical rectification shifts to lower or higher energies as the asymmetry of the system increases, depending on whether the QD is in the oblate or prolate case. This feature can be considered as a new degree of freedom in tuning the nonlinear optical properties of systems based on two-dimensional semiconductor heterostructures. Additionally, especially for prolate QDs, it has been determined that the nonlinear optical rectification amplitude is larger in large-sized QDs and in cases of high asymmetry of the system.