Quantum coherence in neutrino oscillation in matter

Abstract

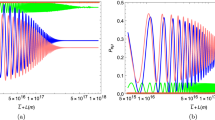
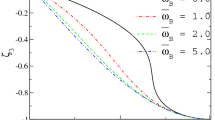
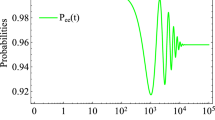
A closer and more detailed study of neutrino oscillation, in addition to assisting us in founding physics beyond the standard model, can potentially be used to understand the fundamental aspects of quantum mechanics. In particular, we know that the neutrino oscillation occurs because the quantum states of the produced and detected neutrinos are a coherent superposition of the mass eigenstates, and this coherency is maintained during the propagation due to the small mass difference of neutrinos. In this paper, we consider the decoherence due to the neutrino interaction in the material medium with constant density in addition to the decoherence coming from the localization properties. For this purpose, we use $l_1\text {-norm}$ in order to quantify the coherence and investigate its dependence on the matter density. According to our results, in general, the coherence in material medium is less than vacuum. However, there exist exceptions; for some matter densities, the localization coherence lengths become infinite. So, for these cases, $l_1\text {-norm}$ in matter is more than the vacuum.

Appendix A: A method for diagonalizing neutrino Hamiltonian in uniform matter

The scattering of neutrino off electrons in the matter causes the electron neutrinos to feel an additional potential V which varies with position, consequently t, when the density of matter varies with the position. In general, V(x) leads the dispersion relation between the momentum $|\vec {p}|$ and the energy E of neutrinos to be changed. Therefore, the investigation of time evolution of the realistic three generations scheme becomes complicated, generally speaking. Many authors have considered this problem [16, 22,23,24,25,26,27,28,29,30,31,32]. In particular, neutrino oscillation in the matter has been treated comprehensively in Refs. [26, 28]. More simplified and accurate arguments have been performed for the oscillations in uniform matter density versus baseline divided by neutrino energy plane by using a perturbative framework in Ref. [16]. As was said, we use the method developed in Ref. [16] to diagonalize the Hamiltonian for a uniform matter. Here, we give a brief of it.

We suppose the flavor eigenstates are written in terms of mass eigenstates as follows:

$$\begin{aligned} \left| \nu _\alpha \right\rangle = \sum _{i=1}^3 U^*_{\alpha i}\left| \nu _i\right\rangle , \end{aligned}$$

(A1)

where $U_{\alpha i}$ is giving in Eq. 3. One can decompose $U_{\text {PMNS}}$ as follows:

$$\begin{aligned} U_{\text {PMNS}}=U_{23}(\theta _{23})I_\delta U_{13}(\theta _{13})I_\delta ^*U_{12}(\theta _{12}), \end{aligned}$$

(A2)

in which

$$\begin{aligned} U_{23}(\theta _{23})=\begin{bmatrix} 1 &{}\quad 0 &{}\quad 0 \\ 0&{}\quad c_{23} &{}\quad s_{23} \\ 0&{}\quad -s_{23} &{}\quad c_{23} \\ \end{bmatrix},\,\,\,\,\, I_\delta =\begin{bmatrix} 1 &{}\quad 0&{}\quad 0 \\ 0&{}\quad 1 &{}\quad 0 \\ 0&{}\quad 0 &{}\quad e^{i\delta } \\ \end{bmatrix},\,\,\,\,\, U_{13}(\theta _{13})=\begin{bmatrix} c_{13} &{}\quad 0&{}\quad s_{13} \\ 0&{}\quad 1 &{}\quad 0 \\ -s_{13}&{}\quad 0&{}\quad c_{13} \\ \end{bmatrix},\,\,\,\,\, U_{12}(\theta _{12})=\begin{bmatrix} c_{12} &{}\quad s_{12} &{}\quad 0 \\ -s_{12}&{}\quad c_{12} &{}\quad 0 \\ 0&{}\quad 0 &{}\quad 1 \\ \end{bmatrix}. \end{aligned}$$

(A3)

The time evolution of the neutrino state in the presence of matter potential is governed by the following Schrodinger equation:

$$\begin{aligned} i\partial _t|\nu _\alpha ,t\rangle =\frac{1}{2E}\left[ U_{\text {PMNS}}{\text {diag}}\left( 0,\Delta m_{21}^2,\Delta m_{31}^2 \right) U_{\text {PMNS}}^\dagger +{\text {diag}(2EV(x),0,0)}\right] |\nu _\alpha ,t\rangle , \end{aligned}$$

(A4)

where $V(x)=\sqrt{2}G_FN_e(x)$. $N_e$ denotes the density of electron in the medium and hereafter we assume it to be constant. We know that the neutrino interactions with matter in the medium do not alter CP phase $\delta$ and mixing angle $\theta _{23}$. So Eq. (A4) is covariant under the inverse transformation $U^{-1}_{23}(\theta _{23})$ and $I^{-1}_{\delta }$. Therefore, we can write the Hamiltonian as follows:

$$\begin{aligned} \acute{H}=\frac{1}{2E}\left[ U_{13}(\theta _{13})U_{12}(\theta _{12}){\text {diag}}\left( 0,\Delta m_{21}^2,\Delta m_{31}^2 \right) U_{12}^\dagger (\theta _{12}) U_{13}^\dagger (\theta _{13}) + {\text {diag}(2EV,0,0)}\right] . \end{aligned}$$

(A5)

Now, one can diagonalize $\acute{H}$ by applying two consecutive rotations in the matter. First, one needs to do the $\theta _{13}^m$ rotation:

$$\begin{aligned} H'=U_{13}^{\dagger }(\theta _{13}^m)\acute{H}U_{13}(\theta _{13}^m), \end{aligned}$$

(A6)

where

$$\begin{aligned} U_{13}^{\dagger }(\theta _{13}^m)=\begin{bmatrix} \cos \theta _{13}^m &{}0&{}-\sin \theta _{13}^m \\ 0&{}1 &{}0 \\ \sin \theta _{13}^m&{}0&{}\cos \theta _{13}^m \\ \end{bmatrix}, \end{aligned}$$

(A7)

with

$$\begin{aligned} \theta _{13}^m=\dfrac{1}{2}\arctan \left[ \dfrac{\epsilon \sin {2\theta _{13}}}{\epsilon \cos {2\theta _{13}}-2EV}\right] . \end{aligned}$$

(A8)

Here, $\epsilon$ is given by

$$\begin{aligned} \epsilon =\Delta m_{31}^2-\Delta m_{21}^2\sin ^2{\theta _{12}}. \end{aligned}$$

(A9)

Therefore, $H'$ is obtained as follows:

$$\begin{aligned} H'= & {} \dfrac{1}{2E}\begin{bmatrix} l_1&{}0&{}0\\ 0&{}l_2&{}0\\ 0&{}0&{}l_3\\ \end{bmatrix}\nonumber \\&+\dfrac{\Delta m_{21}^2\sin {2\theta _{12}}}{4E}\begin{bmatrix} 0&{}\cos (\theta _{13}-\theta _{13}^m)&{}0\\ \cos (\theta _{13}-\theta _{13}^m)&{}0&{}-\sin (\theta _{13}-\theta _{13}^m)\\ 0&{}-\sin (\theta _{13}-\theta _{13}^m)&{}0\\ \end{bmatrix}, \end{aligned}$$

(A10)

in which

$$\begin{aligned} l_2= & {} \Delta m_{21}^2\cos ^2{\theta _{12}}, \end{aligned}$$

(A11)

$$\begin{aligned} l_{1,3}= & {} \dfrac{1}{2}\left[ \left( \Delta m_{31}^2+2EV+\Delta m^2_{21}\sin ^2\theta _{12}\right) \mp \sqrt{(2EV)^2+\epsilon ^2-4EV\epsilon \cos {2\theta _{13}}}\right] . \end{aligned}$$

(A12)

Second, a rotation with $\theta _{12}^m$ angle must be performed such a way that $H'$ is transformed as follows:

$$\begin{aligned} H''=U_{12}^{\dagger }(\theta _{12}^m)H'U_{12}(\theta _{12}^m)=H_0+H_1, \end{aligned}$$

(A13)

with

$$\begin{aligned} H_0=\begin{bmatrix} E^m_1&{}\quad 0&{}\quad 0\\ 0&{}\quad E^m_2&{}\quad 0\\ 0&{}\quad 0&{}\quad E^m_3\\ \end{bmatrix}, \end{aligned}$$

(A14)

where

$$\begin{aligned} E^m_3= & {} \frac{l_3}{2E}, \end{aligned}$$

(A15)

$$\begin{aligned} E^m_{1,2}= & {} \frac{1}{4E}\left[ (l_1+l_2) \mp \sqrt{(l_1-l_2)^2+\Delta m_{21}^4\sin ^2{2\theta _{12}}\cos ^2({\theta _{13}}-\theta _{13}^m})\right] , \end{aligned}$$

(A16)

and

$$\begin{aligned} H_1=\dfrac{1}{4E}\Delta m_{21}^2 \sin {2\theta _{12}}\sin (\theta _{13}-\theta _{13}^m)\begin{bmatrix} 0&{}0&{}\sin {\theta _{12}^m}\\ 0&{}0&{}-\cos {\theta _{12}^m}\\ \sin {\theta _{12}^m}&{}-\cos {\theta _{12}^m}&{}0\\ \end{bmatrix}. \end{aligned}$$

(A17)

$U_{12}^{\dagger }(\theta _{12}^m)$ is defined by

$$\begin{aligned} U_{12}^{\dagger }(\theta _{12}^m)=\begin{bmatrix} \cos \theta _{12}^m &{} -\sin \theta _{12}^m &{}0 \\ \sin \theta _{12}^m&{}\cos \theta _{12}^m &{}0 \\ 0&{}0 &{}1 \\ \end{bmatrix}, \end{aligned}$$

(A18)

in which

$$\begin{aligned} \theta _{12}^m=\dfrac{1}{2}\arctan \left[ \Delta m_{21}^2\dfrac{\sin {2\theta _{12}}}{l_2-l_1}\cos (\theta _{13}-\theta _{13}^m)\right] . \end{aligned}$$

(A19)

In Eq. (A13), $H_0$ has the main contribution in the total Hamiltonian $H''$ and one can treat perturbatively with $H_1$. However, in this paper we ignore the $H_1$ contribution because it will have no remarkable effects on our results.

In the case of anti-neutrino, the following changes are necessary:

$$\begin{aligned} I_\delta \longrightarrow I_\delta ^*, \end{aligned}$$

(A20)

and

$$\begin{aligned} V\longrightarrow -V. \end{aligned}$$

(A21)

Therefore, the final parameters of the anti-neutrino oscillation in matter are given by

$$\begin{aligned} {\bar{\theta }}_{13}^m= & {} \dfrac{1}{2}\arctan \left[ \dfrac{\epsilon \sin {2\theta _{13}}}{\epsilon \cos {2\theta _{13}}+2EV}\right] , \end{aligned}$$

(A22)

$$\begin{aligned} {\bar{\theta }}_{12}^m= & {} \dfrac{1}{2}\arctan \left[ \Delta m_{21}^2 \cos (\theta _{13}-{\bar{\theta }}_{13}^m)\dfrac{\sin {2\theta _{12}}}{{\bar{l}}_2-{\bar{l}}_1}\right] , \end{aligned}$$

(A23)

$$\begin{aligned} {{\bar{E}}}^m_3= & {} \frac{{\bar{l}}_3}{2E}, \end{aligned}$$

(A24)

$$\begin{aligned} {{\bar{E}}}^m_{1,2}= & {} \dfrac{1}{4E} \left[ ({\bar{l}}_1+{\bar{l}}_2)\mp \sqrt{({\bar{l}}_1-{\bar{l}}_2)^2 +\Delta m_{21}^4\sin ^2{2\theta _{12}}\cos ^2({\theta _{13}}-\bar{\theta }_{13}^m})\right] , \end{aligned}$$

(A25)

with

$$\begin{aligned}&\bar{l}_2=l_2=\Delta m_{21}^2\cos ^2{\theta _{12}}, \end{aligned}$$

(A26)

$$\begin{aligned}&\bar{l}_{1,3}=\dfrac{1}{2}\left[ \left( \Delta m_{31}^2-2EV+\Delta m_{21}^2\sin ^2{\theta _{12}}\right) \mp \sqrt{(2EV)^2+\epsilon ^2+4EV\epsilon \cos {2\theta _{13}}}\right] . \end{aligned}$$

(A27)