Abstract
In this work, we present a detailed derivation of novel exact massless scalar quasibound state a static magnetized Ernst black hole background. We successfully solve the governing covariant Klein–Gordon equation and discover the exact radial solutions in terms of the Confluent Heun functions. With the exact radial wave solution in hand, applying its polynomial condition leads to the discovery of the quantized energy levels expression that depends on the black hole’s mass M, magnetic field strength \(B_0\) and also the magnetic and main quantum number \((m_\ell , n).\) A massive scalar field around the black hole has complex valued energy levels while massless particle has purely imaginary energy levels. Further investigation in small black hole limit and zero magnetic field, the massless scalar’s purely imaginary energy expression is recovered. We also discover the equivalence between massless scalar field around an Ernst black hole with massive scalar field around a Schwarzschild black hole. In the last section, the Hawking radiation in investigated and applying the Damour–Ruffini method, the Hawking temperature is obtained out of the radiation distribution function.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
In 1976, Ernst was considering and calculating black hole solution in a magnetized background [1] and 13 years later, the full exact black hole solution was published by Aliev and Galtsov [2]. The so called Ernst space-time describes a static chargeless axially symmetric massive black hole solution immersed in an external homogeneous magnetic field which is characterized by two parameters, i.e. mass M and magnetic field \(B_0\). By nulling the magnetic field, Ernst black hole becomes a static spherically symmetric Schwarzschild black hole.
After the gravitational wave signal of a binary black hole merger was directly detected for the first time [3], black hole spectroscopy has been becoming a hot research subject. The quasibound states, quasinormal modes, and shadows of black holes are among the most interesting characteristic of such an astrophysical objects in the observational measurable spectra that is generated as particles crossing into the black hole [4]. For astrophysical black holes, the black hole quasistationary spectrum is characterized entirely by the black hole mass and angular momentum and is unique to black holes. Quasibound states have complex frequencies where the real part is associated as the scalar’s energy while the imaginary part determines the stability of the system. It is possible, in principle, to extract some information about the physics of black holes as well as to validate some alternative/modified theories of gravity from these quasibound states [4]. Analogously to atomic transitions emitting photons, level transitions of axions around black holes emit gravitons [5]. Thus, it is very important to be able to calculate the exact quasibound states frequency analytically.
Several non-exact analytical methods have recently developed to calculate the quasibound states of various types black holes [6,7,8] where all of the obtained analytical formulas contain the main minding energy expression similar to the Hydrogenic’s \(\frac{1}{n^2}\) energy level followed by higher order terms. The decay, represented by the imaginary part of the complex energy levels, is minimized in the so called small black hole limit [9], i.e. \(M_{black hole}\ll \frac{m_{Plank}^2 c^2}{E_{rest}}\),-which is equivalent to \(r_s \mu \ll \frac{\hbar }{c}\),-where \(m_{Plank}=\sqrt{\frac{\hbar c}{G}}\) is Plank mass, \(E_{rest}= \mu c^2\) is the scalar particle’s rest energy where \(\mu \) is its mass, \(r_s=\frac{2G M_{black hole}}{c^2}\) is the Schwarzschild radius of the black hole with mass \(M_{black hole}\) and c is the speed of light.
In recent years, thanks to the development of the Confluent Heun functions, several authors have [10,11,12,13] successfully found exact scalar quasibound states’ solutions around an analog systems to the Schwarzschild black hole, chargeless Lense–Thirring black hole and Reissner Nordstrom black hole respectively. The importance of this particular special function in black hole physics was mentioned in [14]. In this work, the work is generalized by including electromagnetic field, i.e. a charged Lense–Thirring space-time [15]. In this work, we are going to investigate the behavior of massless scalar field bound to a static magnetized Ernst black hole. We will show that the radial wave equation of the governing Klein–Gordon equation can be exactly solved and the solutions are given in terms of the Confluent Heun functions. The obtained exact solutions in terms of special functions allow us not only to discover the energy quantization out of their polynomial condition, but also to investigate the Hawking radiation to finally obtain the Hawking temperature of the black hole’s apparent horizon by applying the Damour–Ruffini method [16] and obtain the Hawking temperature of the black hole’s apparent horizon. All of the solutions are parametrized by the black hole’s mass M, the magnetic field \(B_0\), magnetic quantum number m and the main quantum number n. However, further investigation to the energy expression and comparison to [12], we find an equivalence between the case of a massive scalar field in a static spherically symmetric Schwarzschild space-time with a massless scalar in static spherically symmetric magnetized Ernst space-time.
2 The Ernst metric and the Klein–Gordon equation
2.1 The metric
In this section, we are going to investigate the behavior of a scalar particle in the space-time around an Ernst black hole black hole. We start with writing the Ernst metric in Schwarzschild coordinate as follows,
where,
and \(B_0\) is a weak magnetic field [2]. The metric is singular when \(f=0\), i.e. at \(r=0\) and \(r=r_s\).
2.2 The Klein–Gordon equation
The Klein–Gordon equation is a covariant wave equation that describes the behavior of a scalar field in a general curved space-time given by [17],
In general, there are 16 components to be worked out, but, most of them are zeros due to the nature of the diagonal metric of the Ernst space-time. First, let us consider the square root of the metric determinant \(\sqrt{-g}\) as follows,
and next, we calculate the explicit expression of each term in the Laplace–Beltrami operator \(\nabla ^2\) as follows,
Combining them into Eq. (5), we get the full Klein–Gordon equation,
Due to the time independence and angular symmetry of the space-time, we apply the separation of variables ansatz [18] and write the wave function in this following form,
where E is the particle’s relativistic energy and m is the azimuthal harmonic number.
Substituting \({\Lambda }^N\approx 1+\frac{N}{4}B^2_0r^2{{\sin }^{{2}} \theta \ }\) and \(\psi \) to the Klein–Gordon equation (11) and multiplying the whole equation by \(r^2{\Lambda }^2\psi ^{-1}\), separate the polar from the radial part as follows,
Now, the polar part can be separated as follows,
The solution is a linear combination of the Associated Legendre functions of the first and second types [18], respectively \(P^m_l\left( {\cos \theta \ }\right) \) and \(Q^m_l\left( {{\cos } \theta \ }\right) \). However, \(Q^m_l\) is singular at \(\theta =0\) and \(\theta =p\) that it must be is omitted. Thus, we get the polar solution as follows,
2.3 The radial equation
Now, he radial equation is successfully isolated,
The radial equation can be written explicitly by rewriting f(r) explicitly from (3), expanding the first term by operating the differential operator to \(r^2f\), followed by multiplying the whole equation by \(\frac{1}{r\left( r-r_s\right) }\). We get,
The observable quasibound states lie outside the apparent black hole horizon, i.e. \(r\ge r_s\). So, we prefer a new radial coordinate that has zero at \(r=r_s\). Thus, we define \(xr_s=r-r_s\rightarrow {\partial }_r=r^{-1}_s{\partial }_x\). The radial equation in terms of x looks like as follows,
Here, we are going to use some dimensionless energy parameters \(\Omega =\frac{{Er}_s}{\hbar c}\) and dimensionless magnetic parameter \({\mathcal {M}}=B^2_0m^2r^2_s\) for the sake of notation simplicity. And with the help of fractional decomposition, we also get,
Now, the radial wave equation can be written in terms of \(\frac{1}{x}\) and \(\frac{1}{x+1}\) as follows,
Following the exact method by [12], to get the exact solution, the normal form of the radial equation must be obtained first. This is done by recognizing the p and q functions as follows,
following the method in Appendix A, we get the normal form of the Eq. (19),
Now we make a final substitution \(x=-z\), to get,
and comparing (24) with the normal form of the Confluent Heun differential equation (see Appendix B), we find the Heun function’s parameters as follows,
2.4 The energy quantization
The polynomial condition of the Heun functions leads to the particle’s energy quantization as follows,
Now, let us compare the energy expression of a massive scalar field around a non magnetic static spherically symmetric Schwarzschild black hole [12, 19] as follows,
where in the limit \(\Omega \propto Er_s\rightarrow 0\), we obtain this following real valued energy levels expression,
2.5 Wave functions near horizon and far away from the horizon
After conditioning the Heun function to be a polynomial function, the behaviour of the quasibound states solution near the apparent horizon, i.e. \(r \rightarrow r_s\) will be investigated. In this particular limit, the Confluent Heun functions’ argument \(x=\frac{r-r_s}{r_s}\) is approaching \(x=0\), thus, \({\text {HeunC}}(0)={\text {HeunC}}'(0)\approx 1\). Also \(e^{-\frac{1}{2}\alpha \left( \frac{r-r_s }{r_s}\right) }\approx 1\). Thus, we get,
Now, let us define a new radial variable \(\frac{r-r_s}{\delta _r}=\zeta r-\zeta _0\) and expressing \(\beta \) explicitly as (26) or equivalently, \(\beta = i|\beta |\) to get this following wave function expression near the horizon,
and using the complex relation \(z^i = e^{1{\text {ln}}(z)}\) together with \(\cos {z}=\frac{1}{2}\left( e^{iz} + e^{-iz}\right) \), we get,
which represent a purely ingoing wave. So, the wave function solution describes ingoing wave near the event horizon and tend to zero at asymptotic infinity.
2.6 “Mass” from black hole’s the magnetic field
Comparing (31) and (32), we can conclude that the case of a massless scalar particle around an Ernst black hole with magnetic parameter \({\mathcal {M}}\) is equivalent to the case of a massive particle around a Schwarzschild black hole, where the particle’s rest energy is determined by,
And in the small Ernst black hole limit, \(\Omega \propto Er_s\rightarrow 0\), we discover real valued energy levels expression,
Different from a massive scalar around the Schwarzschild case (34), where the quantized energy levels depends on the black hole’s mass \(r_s\), particle’s mass \(E_0\) and the main quantum number n, the quantized energy levels of a massless scalar around Ernst black hole depends on the black hole’s mass \(r_s\), magnetic field strength \(B_0\), the magnetic quantum number m and the main quantum number n.
2.7 Schwarzschild limit
However, further investigation in the limit \({\mathcal {M}} \rightarrow 0\), we obtain this following the energy levels expression,
this recovers the relativistic energy expression in Schwarzschild space-time, is purely immaginary which is in agreement with [12, 19]. So, any massless particle can only be absorbed by the rotating black hole. However, this is expected as there is one unstable circular orbit of massless particle around the static spherically symmetric Schwarzschild black hole [20].
While the complete exact wave function is obtained as follows,
3 Hawking radiation
In this section we will follow the Damour–Ruffini [16] method to calculate Hawking temperature of the black hole’s apparent horizon by making use the obtained the exact solutions of the radial wave equation. We start with writing the radial solutions as follows,
Approaching the apparent horizon, \(r \rightarrow r_s \), the radial wave functions can be approximated as follows, \({\text {HeunC}}(0)={\text {HeunC}}'(0)=1\) also \(e^{-\frac{1}{2}\alpha \left( \frac{r-r_s }{r_s}\right) }=1\), that we obtain,
The radial solution is understood that it comprises of two parts, i.e. the ingoing and outgoing waves as follows,
When there is an ingoing wave hitting the horizon \(r_s\), a particle-antiparticle pair are induced. The particle is reflected and will enhance outgoing wave while the antiparticle counterpart becomes the transmitted wave crossing the horizon. Analytical continuation of the wave function \(R \left( \frac{r-r_s }{r_s}\right) \) can be calculated by using this following trick,
This allows us to obtain the expression for \(R_{-out}\) as follows,
which lead to,
The total probability must be normalized to be one,
i.e. the total probability of the particle wave going out to infinity and the antiparticle wave going inside the black hole must be equal to 1. Near the \(r_s\), thus, we have,
or can be rewritten as follows,
The Hawking temperature \(T_H\) of the corresponding horizon is to be extracted from the thermal spectrum known as radiation distribution function by calculating the modulus square of the ratio between the normalization constant between the outgoing and incoming waves as follows,
and then doing this following modification,
Finally, after comparing with the boson distribution function \(e^{\frac{\hbar \omega }{k_B T}}\) we obtain the apparent horizon temperature as follows,
4 Conclusions
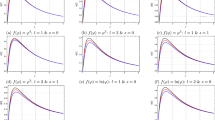
In this work, the exact analytical quasibound state’s quantized energy levels (31) and their wave functions (43) of a massless scalar particle around an Ernst black hole are obtained. We also discover that there is an equivalence between a massive scalar in static spherically symmetric Schwarzschild space-time and a massless scalar in static spherically symmetric magnetized Ernst space-time. After further investigation, we find that the exact solution describes ingoing wave near the event horizon and tend to zero at asymptotic infinity. It is important to mention that the exact radial solution is valid for all region of interest, i.e. \(r_s \le r < \infty \), a significant improvement of the asymptotical method that solves for either region very close to the horizon of very far away from the horizon.
In small black hole limit, we obtain real valued energy levels expression of the massless scalar around the static spherically symmetric magnetized Ernst black hole (40) which depends on the black hole’s mass \(r_s\), magnetic field strength \(B_0\), the magnetic quantum number m and the main quantum number n. By taking \(B_0\rightarrow 0\) we recover the purely imaginary relativistic energy expression which is exactly the same with the case of massless scalar in Schwarzschild space-time [12, 19],
that is also consistent with previously published results [6,7,8, 21].
With exact relativistic wave functions solution in hand, the [16] method is applied to calculate the Hawking temperature of the black hole’s apparent horizon (59) which is in agreement with [14], – after nulling the charge parameter of the Reissner–Nordstrom black hole surrounded by a magnetic field. It uses the Klein pair production scenario where the pair production occurring at the horizon is induced by an incoming particle. The induced particle goes to infinity while the induced anti-particle goes towards the black hole. The modulus square of the ratio between particle and antiparticle wave functions represents the probability function of Hawking radiation.
Data Availability Statement
This manuscript has no associated data or the data will not be deposited. [Authors’ comment: The research is theoretical, so there is no experimental data.]
References
F.J. Ernst, Black holes in a magnetic universe. J. Math. Phys. 17, 54–56 (1976)
A.N. Aliev, D.V. Galtsov, Exact solutions for magnetized black holes. Astrophys. Space Sci. 155, 181 (1989). https://doi.org/10.1007/BF00643854
B.P. Abbott, Observation of gravitational waves from a binary black hole merger. Phys. Rev. Lett. 116, 061102 (2016). https://doi.org/10.1103/PhysRevLett.116.061102
H.S. Vieira, Quasibound states, stability and wave functions of the test fields in the consistent 4D Einstein–Gauss–Bonnet gravity. Universe 9(5), 205 (2023). https://doi.org/10.3390/universe9050205
A. Arvanitaki, M. Baryakhtar, X. Huang, Discovering the QCD axion with black holes and gravitational waves. Phys. Rev. D 91, 084011 (2015)
D. Baumann, H.S. Chia, R.A. Porto, Probing ultralight bosons with binary black holes. Phys. Rev. D 99, 044001 (2019). https://doi.org/10.1103/PhysRevD.99.044001
D. Baumann, H.S. Chia, J. Stout, L. Haar, The spectra of gravitational atoms. J. Cosmol. Astropart. Phys. 2019(12), 006 (2019). https://doi.org/10.1088/1475-7516/2019/12/006
D. Baumann, G. Bertone, J. Stout, G.M. Tomaselli, Ionization of gravitational atoms. Phys. Rev. D 105, 115036 (2022). https://doi.org/10.1103/PhysRevD.105.115036
A.B. Gaina, I.M. Ternov, Energy spectrum of the Klein–Gordon equation in Schwarzschild and Kerr fields. Sov. Phys. J. 31(10), 830–834 (1988). https://doi.org/10.1007/BF00920128
H.S. Vieira, K.D. Kokkotas, Quasibound states of Schwarzschild acoustic black holes. Phys. Rev. D 104, 024035 (2021). https://doi.org/10.1103/PhysRevD.104.024035
H.S. Vieira, K. Destounis, K.D. Kokkotas, Analog Schwarzschild black holes of Bose–Einstein condensates in a cavity: quasinormal modes and quasibound states. Phys. Rev. D 107, 104038 (2023). https://doi.org/10.1103/PhysRevD.107.104038
D. Senjaya, Exact analytical quasibound states of a scalar particle around a slowly rotating black hole. J. High Energy Astrophys. 40, 49–54 (2023). https://doi.org/10.1016/j.jheap.2023.10.002
D. Senjaya, Exact analytical quasibound states of a scalar particle around a Reissner–Nordström black hole. Phys. Lett. B (2023). https://doi.org/10.1016/j.physletb.2023.138373
H.S. Vieira, V.B. Bezerra, Confluent Heun functions and the physics of black holes: resonant frequencies, Hawking radiation and scattering of scalar waves. Ann. Phys. 373, 28–42 (2016). https://doi.org/10.1016/j.aop.2016.06.016
C. Lämmerzahl, M. Maceda, A. Macías, 36(1), 015001 (2018). https://doi.org/10.1088/1361-6382/aaeca7
T. Damour, R. Ruffini, Black-hole evaporation in the Klein–Sauter–Heisenberg–Euler formalism. Phys. Rev. D 14, 332 (1976)
D. Senjaya, A.S. Rivera, Canonical quantization of neutral and charged static black hole as a gravitational atom. J. Phys. Conf. Ser. 1719, 012019 (2021)
F.W.J. Olver, D.W. Lozier, R.F. Boisvert, C.W. Clark, NIST Handbook of Mathematical Functions, 1st edn. (Cambridge University Press, Cambridge, 2010)
D. Senjaya, A.S. Rivera, Canonical quantization of neutral and charged static black hole as a gravitational atom. Phys. Rev. D 1719, 012019 (2021)
C. Bambi, Introduction to General Relativity. Undergraduate Lecture Notes in Physics. (Springer, New York, 2018). Paperback
H.S.C. Daniel Baumann, R.A. Porto, Probing ultralight bosons with binary black holes. Phys. Rev. D 99, 044001 (2019)
A. Ronveaux, Heun’s Differential Equations, 1st edn. (Clarendon Press, Oxford, 1995)
Funding
This study was funded by Faculty of Science, Mahidol University (6437484SCPY/D).
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix A: Normal form
There is a trick to simplify a second order linear differential equation so called normal form method [18]. The normal form method is designed to remove the first order derivative terms in a linear second order ordinary differential equation. Suppose we have the aforementioned equation as follows.
Now, substitute this following expression,
A lot of things cancel each other and we get this following equation without the first order derivative term,
Appendix B: Normal form of confluent Heun equation
The Confluent Heun differential equation is a linear second order ordinary differential equation as follows, [22].
the solution is written as linear combination of Confluent Heun functions,
The Confluent Heun function \({\text {HeunC}}\left( \alpha ,\beta ,\gamma ,\delta ,\eta ,x\right) \) can become a polynomial function with degree \(n_r\) if this following condition is fulfilled,
The normal form of the Confluent Heun differential equation can be found using the method in Appendix A, where p and q are as follows,
Finally, combining everything, we get the Confluent Heun equation in its normal form,
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3. SCOAP3 supports the goals of the International Year of Basic Sciences for Sustainable Development.
About this article
Cite this article
Senjaya, D. Exact massless scalar quasibound states of the Ernst black hole. Eur. Phys. J. C 84, 57 (2024). https://doi.org/10.1140/epjc/s10052-024-12422-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1140/epjc/s10052-024-12422-4