1 Introduction

Electromagnetic (EM) form factor (FF) [1, 2] describes the interaction strength of momentum redistribution in a hadron when it is hinted by an energetic photon whereas not breaks up. It plays an indispensable role in the QCD study, such as the development of factorization theorem, and also the investigation of hadron structure.

Pion EM form factor have attracted various QCD-based studies. For examples, the lattice QCD (LQCD) recently have improved their evaluation ability in the region \(- 1 \, \textrm{GeV}^2 \le q^2 \le 0\) [3], the evaluation from Dyson–Schwinger equation (DSE) is applicable in the low momentum transfers \(-5 \, \textrm{GeV}^2 \le q^2 \le -1 \,\textrm{GeV}^2\) [4, 5], the Light-cone sum rules (LCSRs) calculation based on operator production expansion is valid in the low and intermediate momentum transfers \(-10 \, \textrm{GeV}^2 \le q^2 \le -1 \, \textrm{GeV}^2\) [6, 7], and the perturbative QCD (pQCD) applies to the process with large momentum transfer \(\vert q^2 \vert , q^2 \gtrsim 10 \, \textrm{GeV}^2\) [8, 9]. In this work, we focus on the pQCD study based on \(k_T\) factorization theorem [10], in which the transversal momentum is picked up to regularize the end-point divergence and the resummation techniques are embodied to suppress the large logarithms generated by infrared gluon radiations, so that the processes with large momentum transfers are dominant conducted by the hard scattering and hence calculable in perturbative theorem. An advantage of pQCD is that both the spacelike and timelike form factors are calculable when the momentum transfer/invariant mass is large.

The theoretical-self uncertainty in the pQCD calculations mainly comes from the input parameters of hadron distribution amplitudes and the renormalization scheme. To be specific, first, the skewed distribution of sub-leading twist light-cone distribution amplitudes (LCDAs) results in the apparent chiral enhancement effect, saying the contribution from sub-leading twist LCDAs is larger than it from the leading twist one in the non-ultraviolet region. These enhancement terms are proportional to the chiral mass \(m_0^\pi = m_\pi ^2/(m_u+m_d)\), here \(m_\pi \), \(m_u\) and \(m_d\) being the pion, u and d quark masses, respectively. In the previous pQCD calculations, \(m_0^\pi \) is usually chosen at a fixed value in the boundary \(1.74^{+0.67}_{-0.38}\) [11] as shown in Table 1, and hence the corresponding large uncertainty is formerly disregarded. Second, the choice of lowest Gegenbauer moment \(a_2^\pi \) in the leading twist LCDAs engender sizable uncertainty to the form factor at low and intermediate momentum transfers. Last but not least, the factorization and renormalization scales, saying \(\mu _f\) and \(\mu _R\), is conventionally set at the largest virtuality in the hard scattering \(\mu _t = \textrm{Max}(x_iQ^2, 1/b_i)\), here \(x_i, b_i\) denote the longitudinal momentum fraction carried by a parton and the conjugate extent to transversal momentum in a meson, respectively. In the pion EM radiative process, the typical scales are \(x_i Q^2 \pm k_{iT}^2\) in the spacelike/timelike transitions, the other choices between the extreme alternatives \(\mu = Q^2 \pm k_{iT}^2\) and \(\mu = k_{iT}^2\) are all reasonable.

Table 1 Input of \(m_0^\pi \) in the previous pQCD calculations
Full size table

A direct way to extract \(m_0^\pi \) and \(a_2^\pi \) is to compare the precise pQCD calculation with the experiment measurements. From the experiment side, the spacelike form factor is usually measured via the electron-nucleon elastic scattering [20] and the electron produced pion meson process \(^1H(e,e'\pi ^+)n\) [21, 22]. The precise measurement has been obtained in the small momentum transfers \(-2.50 \, \textrm{GeV}^2 \le q^2 \le -0.25 \,\textrm{GeV}^2\), but not in the intermediate and large momentum transfer regions. Meanwhile, the timelike form factor have been measured at B factories. For examples, the isospin-vector form factor is measured via the \(\tau \) decays in the momentum transfer region \(4m_\pi ^2 \le q^2 \le 3.125 \,\textrm{GeV}^2\) by the Belle collaboration [23], and via the \(e^+e^-\) annihilation process in the region \(4 m_\pi ^2 \le q^2 \lesssim 8.7 \, \textrm{GeV}^2\) by the BABAR collaboration [24] with high accuracy, it is also measured by the BESIII collaboration in the low momentum transfer \(0.6 \,\textrm{GeV}^2 \le q^2 \le 0.9 \,\textrm{GeV}^2\) based on the initial state radiation (ISR) method [25]. We can see that so far the precise measurements are carried out in the resonant region, while the powerful pQCD calculations are valid in the region with large momentum transfers. This mismatch destroys the programme of comparing the measurements directly with the pQCD calculations.

In order to overcome this mismatch and do the extraction of this nonperturbative parameters, in this paper we employ the dispersion relation proposed in [7], in which the spacelike form factor is written as the integration of the module square of the timelike one. We take the BABAR data to describe the timelike form factor in the resonant regions, and use the pQCD approach to calculate the timelike and spacelike form factors with the large momentum transfer/invariant mass. Our pQCD calculation takes into account all the current known next-to-leading-order (NLO) corrections [12,13,14,15], and the accuracy of power expansion is up to twist four both for two-particle and three-particle LCDAs [9, 26, 27]. Besides the errors from the BABAR measurements, we examine the influence from the scale choice in the pQCD calculation, and consider the renormalization evolution of the nonperturbative parameters whose effect are usually ignored in the previous pQCD calculations.

The paper is arranged as follow. In the next section, the dispersion relations are introduced in the standard and modified formalisms, In Sect. 3, the pQCD formalism is demonstrated for the pion electromagnetic form factor. We then do the fit between the spacelike form factor obtained from the dispersion relation and the direct pQCD calculation in Sect. 4. The summary is given in Sect. 5.

Fig. 1
figure 1

Feynman diagrams of spacelike (left) and timelike (right) pion form factors at leading-order

Full size image

2 Dispersion relations

With considering the analytical properties follow from the causality and Cauchy’s theorem, the real and imaginary parts of scattering amplitudes are related to each other by the dispersion relation. In light of this, the full pion EM form factor with \(q^2 < 4m_\pi ^2\) can be written as an integral over its imaginary parts in the physical region without subtraction [28, 29]

$$\begin{aligned} \mathcal{F}_\pi (q^2) = \frac{1}{\pi } \int _{4m_\pi ^2}^\infty ds \frac{\textrm{Im}\mathcal{F}_\pi (s)}{s - q^2 - i \epsilon }. \end{aligned}$$
(1)

In the power limit at \(q^2 \rightarrow \infty \), this relation reproduces the QCD asymptotics \(\mathcal{F}_\pi (q^2) \sim 1/q^2\).

The measurement of timelike form factor in the right hand side (RHS) of Eq. (1) is carried out for the modulus square \(\vert \mathcal{F}_\pi (s) \vert ^2\), and the information of imaginary part is usually obtained by a parameterization of \(\mathcal{F}_\pi (s)\), such as the Gounaris–Sakurai (GS) representation [30] and the Kühn-Santamaria (KS) representation [31]. In this sense, the standard dispersion relation in Eq. (1) brings an inevitable model dependence which results in an additional uncertainty to the spacelike form factor on the left hand side (LHS). To get rid off the model dependence, the modulus squared dispersion integral is proposed [7]

$$\begin{aligned} {\mathcal {F}}^{\textrm{DR}}_\pi (q^2)= & {} \exp \left[ \frac{q^2 \sqrt{s_0 - q^2}}{2 \pi } \int \limits _{4m_\pi ^2}^\infty d s \frac{ \ln \vert {\mathcal {F}}_\pi (s) \vert ^2}{s\,\sqrt{s - s_0} \, (s -q^2)} \right] . \nonumber \\ \end{aligned}$$
(2)

The modulus square can be written in terms of heavy theta functions

$$\begin{aligned} \vert {\mathcal {F}}_{\pi }(s) \vert ^2= & {} \Theta (s_{\textrm{max}} - s) \vert {\mathcal {F}}^{\textrm{data}}_{\pi }(s) \vert ^2 \nonumber \\{} & {} + \Theta (s - s_{\textrm{max}}) \vert {\mathcal {F}}_{\pi }^{\textrm{tail}} (s) \vert ^2 , \end{aligned}$$
(3)

where the data is now available in the region \([4m_\pi ^2, s_{\textrm{max}} \simeq 8.7 \, \textrm{GeV}^2]\) and the high energy tail are left to theoretical calculation.

The modified formalism in Eq. (2) improves the accuracy of dispersion relation by skipping the reconstruction of imaginary part of timelike form factor, while the special expressions of \(\vert {\mathcal {F}}_{\pi }(s) \vert ^2\) in Eq. (3) could bring additional model dependence. For example, in the LCSRs work [7], the piece of data was parameterized in the GS model by taking in to account the contributions from \(\rho , \rho ^\prime , \rho ^{\prime \prime }\) and \(\omega \) [24], and the high energy tail beyond the experiment availability was written in the duality resonant model (DRM) [32, 33]. In this work, we study the modified dispersion relation from the view of pQCD approach. An unique advantage here is that the high energy tail can be directly calculated by perturbative theorem. For the measurement [24], the data sample densities are roughly \(\sim 0.01 \, \textrm{GeV}\), \(\sim 0.002 \, \textrm{GeV}\) and \( 0.1 \, \textrm{GeV}\) in the near resonances, resonances located and away resonances regions, respectively. We take the data by interpolating with evenly distribution under the interval \(0.01 \, \textrm{GeV}\). Equation (3) is then converted to

$$\begin{aligned} |{\mathcal {F}}_{\pi }(s)|^2= & {} \Theta (s_{\textrm{max}} - s) \, \vert {\mathcal {F}}^{\textrm{data}}_{\pi , \mathrm{Inter.}}(s) \vert ^2 \nonumber \\{} & {} + \Theta (s - s_{\textrm{max}}) \, \vert {\mathcal {F}}_{\pi }^{\textrm{pQCD}}(s) \vert ^2. \end{aligned}$$
(4)

3 Perturbative QCD formulism

Figure 1 shows the feynman diagrams of pion EM form factors at leading order (LO), in which the EM vertexes are denoted by \(\otimes \) and the possible attachments of internal hard gluons are denoted by \(\times \). \(p_1 = (Q/\sqrt{2}, 0, \textbf{0}), p_2 = (0,Q/\sqrt{2}, \textbf{0})\) are momenta of external mesons, \(k_1 = (xQ/\sqrt{2}, 0, \textbf{k}_{\textrm{1T}})\) and \(k_2 = (0,yQ/\sqrt{2}, \textbf{k}_{\textrm{2T}})\) are the momentum carried by the quark lines in the external mesons. Here x, y and \(\textbf{k}_{\textrm{1T}}, \textbf{k}_{\textrm{2T}}\) denote the longitudinal momentum fractions and transversal momentum, respectively. The momentum transfer squared is \(q^2 = (p_1-p_2)^2\) in the spacelike form factor, whereas it reads as \(q^2 = (p_1+p_2)^2\) in the timelike case. The sum of the two diagrams in Fig. 1 gives the invariant amplitudes of transitions with EM current.

$$\begin{aligned} j_\mu ^{\textrm{em}} = \left( {\bar{u}} \gamma _\mu u - {\bar{d}} \gamma _\mu d \right) . \end{aligned}$$
(5)

We take the spacelike pion form factor to explain the basic idea and formulae of pQCD approach. It can be defined by the nonlocal matrix element

$$\begin{aligned} \langle \pi ^-(p_2) \vert j_{\mu , q}^{\textrm{em}} \vert \pi ^-(p_1) \rangle \equiv e_q (p_1 + p_2) \mathcal{F}_\pi (Q^2) \, \end{aligned}$$
(6)

with \(j_{\mu , q}^{\textrm{em}}\) being the q-flavor component in the EM current. With separating the contributions from short and long-distance interactions, the matrix element is written in the factorizable formulism as

$$\begin{aligned}{} & {} \langle \pi ^-(p_2) \vert J_\mu ^{\mathrm {e.m.}} \vert \pi ^-(p_1) \rangle = \oint dz_1 dz_2 \, H_{\gamma \beta \alpha \delta }^{ijkl}(z_2,z_1) \nonumber \\ {}{} & {} \bigg \langle \pi ^-(p_2) \big \vert \big \{ \overline{d}_\gamma (z_2) \textrm{exp} \left( ig_s \int _{0}^{z_2} d\sigma _{\nu ^\prime } A_{\nu ^\prime }(\sigma ) \right) u_\beta (0) \big \}_{kj} \big \vert 0 \bigg \rangle _{\mu _t} \, \nonumber \\ {}{} & {} \bigg \langle 0 \big \vert \left\{ \overline{u}_\alpha (0) \, \textrm{exp} \left( i g_s \int _{z_1}^{0} d\sigma _\nu A_\nu (\sigma ) \right) d_\delta (z_1) \right\} _{il} \big \vert \pi ^-(p_1) \bigg \rangle _{\mu _t}. \nonumber \\ \end{aligned}$$
(7)

Here \(\gamma , \beta , \alpha , \delta \) are the spinor indices, i, j, k, l are the color indicators, and \(\mu _t\) is the factorizable scale. The large momentum transfer square \(\vert q^2 \vert \) ensures the smallness of relative interaction distance \(\overline{z}_1 - \overline{z}_2\), in this case \( \left( \overline{z}_1 - \overline{z}_2 \right) \cdot \overline{q} \sim 1\) and the expansion parameter for a given operator is the twist.

The matrix elements on the RHS indicate the amplitudes of mesons breaking-up into partons, or inversely of the hadronization. It is can be rewritten in terms of contributions from different Gamma matrices via the Fierz identity

$$\begin{aligned}{} & {} \bigg \langle 0 \vert \left\{ \overline{u}_\alpha (0) \, \textrm{exp} \left( i g_s \int _{z_1}^{0} d\sigma _\nu A_\nu (\sigma ) \right) d_\delta (z_1) \right\} _{il} \vert \pi ^-(p_1) \bigg \rangle _{\mu _t} \nonumber \\ {}{} & {} \quad = \frac{\delta _{il}}{3} \bigg \{ \frac{1}{4} \left( \gamma _5 \gamma ^\rho \right) _{\delta \alpha } \big \langle 0 \big \vert \overline{u}(0) \, \textrm{exp} \left( i g_s \int _{z_1}^{0} \, d\sigma _\nu A_\nu (\sigma ) \right) \nonumber \\ {}{} & {} \qquad \times \left( \gamma _\rho \gamma _5 \right) d(z_1) \big \vert \pi ^-(p_1) \big \rangle _{\mu _t} + \cdots \bigg \}. \end{aligned}$$
(8)

The remaining nonlocal matrix elements in Eq. (8) with certain Gamma matrixes are usually expanded by the LCDAs at different twists, not only for the assignment of a pair of soft quarks (two particle configuration), but also for the three soft partons with an additional soft gluon (three particle configuration) [34].

In Eq. (7), H is the perturbative hard kernel. We here only show explicitly the general expression accompanied with two particle configuration

$$\begin{aligned} H_{\gamma \beta \alpha \delta }^{ijkl} (z_1,z_2)= & {} (-1) \left[ i g_s \gamma _m \right] _{\alpha \beta } T^{ij} \left[ -i D_{mn}^0(z_1-z_2) \right] \nonumber \\ {}{} & {} \times \left[ (i e_q \gamma _\mu ) S_0(0-z_1) (i g_s \gamma _n) \right] _{\gamma \delta } T^{kl} , \end{aligned}$$
(9)

the free propagators read as

$$\begin{aligned} S_0(z) = \frac{i}{2\pi }\frac{/\!\!\!{z}}{z^4} , \,\,\,\,\,\, D_{mn}^0(z) = \frac{1}{4\pi }\frac{g_{mn}}{z^2}. \end{aligned}$$
(10)

The accuracy of pQCD prediction of pion EM form factor is now up to twist four of both two-particle (2p) and three-particle (3p) pion LCDAs and to NLO of QCD strong coupling of 2p-to-2p scattering [9]. The spacelike form factor accompanied with the 2p-to-2p and 3p-to-3p scattering are separately quoted here as

$$\begin{aligned} \mathcal{F}^{\textrm{2p}}_\pi (Q^2)= & {} \frac{8}{9} \alpha _s \pi f_\pi ^2 Q^2 \int _0^1 dx \int _0^1 dy \, \int _0^{\frac{1}{\Lambda }} b_1 db_1 b'_1 db'_1 \, \nonumber \\{} & {} \times e^{-S_{\textrm{2p}}} \Bigg \{ \bar{y} \varphi _\pi (x) \varphi _\pi (y) \, \Big [1+ F_{t2}^{(1)} (x, y, t, Q^2)\Big ] \, {\mathcal {H}} \nonumber \\{} & {} + \frac{2m_0^2}{Q^2} S_t(\bar{y}) \Big [- y \varphi _\pi ^p(x) \varphi _\pi ^p(y) \left[ 1+F_{t3}^{(1)} (x, y, t, Q^2)\right] \, {\mathcal {H}} \nonumber \\{} & {} {+} \frac{1}{6} \varphi _\pi ^p(x) \varphi _\pi ^\sigma (y) \left[ {-}y Q^2\, {\mathcal {H}}_1 {-}(\bar{x}{-}\bar{y}{+}2\bar{y}{-}x\bar{y})Q^2 \, {\mathcal {H}}_2 {-} {\mathcal {H}}\right] \Big ] \,\nonumber \\{} & {} {+} 2 S_t(\bar{y}) \Big [ g_{2\pi }(x) \varphi _\pi (y) \, \bar{x}\bar{y} {\mathcal {H}}_2 {+} \varphi _\pi (x) g_{2\pi }(y) \, \bar{y}^2 \left[ {\mathcal {H}}_1 {+} {\mathcal {H}}_2 \right] \nonumber \\{} & {} + \big [ \varphi _\pi (x) g_{1\pi }(y) - \varphi _\pi (x) g_{2\pi }^{\dag }(y) \big ] \nonumber \\{} & {} \cdot \left[ 2 \bar{y} ({\mathcal {H}}_1 + {\mathcal {H}}_2 + \bar{y}(2-x) {\mathcal {H}}_3) \right] \Big ] \Big \} , \end{aligned}$$
(11)
$$\begin{aligned} \mathcal{F}^{\textrm{3p}}_\pi (Q^2)= & {} \frac{16}{3} \alpha _s \pi f_{\pi }^2 Q^2 \int _0^1 {\mathcal {D}}x_i \int _0^1 {\mathcal {D}}y_i \int _0^{\frac{1}{\Lambda }} \, b_1 db_1 b'_1 db'_1 b_2^2 db_2 \, \nonumber \\{} & {} \times e^{-S_{\textrm{3p}}} \Bigg \{ \frac{f_{3\pi }^2}{f_{\pi }^2} (1-y_1) \varphi _{3\pi }(x_i) \varphi _{3\pi }(y_i) \, {\mathcal {H}}' \nonumber \\{} & {} + \frac{1}{2Q^2} \Big [ \varphi _\parallel ^\dag (x_i) \varphi _\parallel ^\dag (y_i) \big [ \left( -4 (1-y_1)+ (1-y_1)y_2 \right) Q^2 \, {\mathcal {H}}'_2 \nonumber \\{} & {} + 5(1-y_1)y_2Q^2 \,{\mathcal {H}}'_3 \big ] + \varphi _\parallel ^\dag (y_i) \varphi _\perp (x_i) \big [ 4\,{\mathcal {H}}' +(1-y_1)Q^2 \, {\mathcal {H}}'_1 \nonumber \\{} & {} - (1-x_1)(1-y_1)Q^2 \, {\mathcal {H}}'_2 + (1-x_1)(1-y_1)x_2 Q^2 \, {\mathcal {H}}'_3 \big ] \nonumber \\{} & {} + \varphi _\perp (y_i) \varphi _\parallel ^\dag (x_i) \big [ -y_1(1-y_1)Q^2\, {\mathcal {H}}'_2 + y_1 (1-y_1) y_2Q^2 \, {\mathcal {H}}'_3 \big ] \nonumber \\{} & {} + \varphi _\perp (y_i) \varphi _\perp (x_i) 5y_1 \, {\mathcal {H}}' + [\varphi \rightarrow \tilde{\varphi } ] \Big ] \Big \}. \end{aligned}$$
(12)

The relation \(Q^2 \equiv -q^2\) is implied for the spacelike form factor. Here \(\varphi _\pi \), \(\varphi _\pi ^{p/\sigma }\) and \(g_{2\pi }\) denote the twist-2, twist-3 and twit-4 LCDAs of pion meson with two particle configuration, \(\varphi _{3\pi }\) and \(\varphi _{\parallel ,\perp }, \tilde{\varphi }_{\parallel ,\perp }\) are the twist-3 and twist-4 LCDAs associated to three particle configurations, respectively, \(\varphi _{\parallel ,\perp }^\dag \) are the auxiliary DAs related to \(\varphi _{\parallel ,\perp }\). \(S_\textrm{2p}=S_{\textrm{2p}}(x,y,b,b',\mu )\) and \(S_{\textrm{3p}}=S_\textrm{3p}(x,y,b_i,b'_i,\mu )\) are the \(k_T\) Sudakov suppressed functions, \(S_t\) is the threshold Sudakov function start to be appeared at subleading twist due to the skewed distribution of \(\varphi _\pi ^{p/\sigma }\). The hard function \({\mathcal {H}}\) indicates the Fourier integral from \(\textbf{k}_{\textrm{iT}}\) to \(b_i\) which is usually written in the product of Bessel functions. \(F_{t2}^{(1)}\) and \(F_{t3}^{(1)}\) are the NLO correction functions associated to \(\textrm{2p}\) twist-2 and twist-3 LCDAs [12, 13], respectively. For the timelike form factor, we have the same decomposition as in Eq. (23) and similar expressions as in Eqs. (11, 12).

In our calculation, we take the leading twist LCDAs truncated to the second term on the gegenbauer polynomial expansion, take the \(\textrm{2p}\) twist three LCDAs up to NLO in conformal spin and the second terms in truncated conformal expansion.

$$\begin{aligned} \varphi _\pi (x, \mu )= & {} 6 x (1-x) \big [ 1 + a_2(\mu ) C_2^{3/2}(t) + a_4(\mu ) C_4^{3/2}(t) \big ] , \nonumber \\ \end{aligned}$$
(13)
$$\begin{aligned} \varphi ^{ p}_\pi (x, \mu )= & {} 1 + 3 \rho _{\pi } \Big ( 1 +6 a_2(\mu ) \Big )(1+\ln x) \nonumber \\{} & {} - \frac{\rho _{\pi }}{2} \Big ( 3 +54 a_2(\mu ) \Big ) \, C_1^{1/2}(t) \nonumber \\{} & {} + 3 \Big (10 \eta _{3 \pi } +5 a_2(\mu ) \rho _{\pi } \Big ) \, C_2^{1/2}(t)\nonumber \\{} & {} - \frac{9}{2} a_2(\mu ) \rho _{\pi } \, C_3^{1/2}(t) - 3 \eta _{3\pi } \omega _{3\pi } \, C_4^{1/2}(t) , \end{aligned}$$
(14)
$$\begin{aligned} \varphi _\pi ^\sigma (x, \mu )= & {} 6x(1-x) \Big \{ 1 + \frac{\rho _{\pi }}{2} \Big (2 + 30 a_2(\mu )\Big )\nonumber \\ {}{} & {} - \frac{15}{2}a_2(\mu ) \rho _{\pi } \, C_1^{3/2}(t) \nonumber \\ {}{} & {} + \frac{1}{2} \Big ( \eta _{3 \pi }(10-\omega _{3 \pi }) + 3\rho _{\pi } a_2(\mu ) \Big ) \, C_2^{3/2}(t)\nonumber \\ {}{} & {} + 3 \rho _{\pi }\Big ( 1 + 6 a_2(\mu ) \Big ) \, \ln x \Big \}. \end{aligned}$$
(15)

Here \(t=2x - 1\). Two particle twist three DAs \(\phi _\pi ^{p/\sigma }\) relate to both the leading twist DA \(\varphi _\pi \) and the \(\textrm{3p}\) twist three DAs, whose contributions are separated clearly in the Eqs. (14, 15). The renormalization group equation of light quark mass and gegenbauer coefficients read as

$$\begin{aligned}{} & {} m_q(\mu ) = m_q(\mu _0) \left[ \frac{\alpha _s(\mu _0)}{\alpha _s(\mu )} \right] ^{-4/b} , \nonumber \\{} & {} a_n(\mu ) = a_n(\mu _0) \left[ \frac{\alpha _s(\mu _0)}{\alpha _s(\mu )} \right] ^{-\left( \gamma _n^{(0)} + 4 \right) /b}. \end{aligned}$$
(16)

The one-loop anomalous dimension is

$$\begin{aligned} \gamma _n^{(0)}= & {} C_F \left[ 1 - \frac{2}{(n+1)(n+2)} + 4 \sum _{j=2}^{n+1} \frac{1}{j} \right] \end{aligned}$$
(17)

and \(b = (11 N_c - 2 n_f)/3, C_F = (N_c^2-1)/(2N_c)\). The parameters \(f_{3\pi }\) and \(\omega _{3 \pi }\) are defined by the matrix element of local twist-3 operators [34], \(\eta _{3\pi }=f_{3\pi }/(f_\pi m_0^\pi )\) and \(\rho _{\pi }=m_{\pi }/m_0^{\pi }\). In our calculation we take \(m_{\pi }=0.14 \, \textrm{GeV}\), \(f_{\pi }=0.13 \, \textrm{GeV}\), \(f_{3\pi }=0.0045\pm 0.0015 \, \textrm{GeV}^2\) and \(\omega _{3\pi }=-1.5\pm 0.7\). The Gegenbauer polynomials are

$$\begin{aligned}{} & {} C_1^{1/2}(t)= t, \quad C_1^{3/2}(t)= 3t , \nonumber \\{} & {} C_2^{1/2}(t)=\frac{1}{2}(3t^2-1), \quad C_2^{3/2}(t)=\frac{3}{2}(5t^2-1) , \nonumber \\{} & {} C_3^{1/2}(t)=\frac{1}{2}[5t^3-3t], \quad C_4^{1/2}(t)=\frac{1}{8}(35t^4-30t^2+3).\nonumber \\ \end{aligned}$$
(18)

Two particle twist four LCDAs appeared in the third term in Eq. (11) read as [35, 36]

$$\begin{aligned} g_{2\pi }(x)= & {} -\frac{1}{2}\int _0^x dx' \psi _{4\pi }(x') , \nonumber \\ g_{1\pi }(x)= & {} \frac{1}{16} \phi _{4\pi }(x) + \int _0^x dx' g_{2\pi }(x') \nonumber \\ \psi _{4\pi }(x)= & {} \delta _\pi ^2 \Big [ \frac{20}{3} \, C_2^{1/2}(2x-1) \Big ] , \nonumber \\ \phi _{4\pi }(x)= & {} \delta _\pi ^2 \big [ \frac{200}{3} x^2\bar{x}^2 + 21 \omega _{4\pi } \Big ( x\bar{x} (2+13x\bar{x}) \nonumber \\ {}{} & {} + [2x^3(6x^2-15x+10) \ln x] + [x \leftrightarrow \bar{x}] \Big ) \Big ]. \nonumber \\ \end{aligned}$$
(19)

We only consider the contributions from the “genuine” \(\textrm{3p}\) twist four DAs \(\varphi _\parallel (x_i), \varphi _{\perp }(x_i)\), which are characterised by the parameters \(\delta _\pi ^2\), and the contributions arose from the Wandzura–Wilczek-type mass corrections are neglected since they are proportional to \(m_\pi ^2\).

Twist three and twist four LCDAs accompanied with \(\textrm{3p}\) configuration are

$$\begin{aligned}{} & {} \varphi _{3\pi }(x_i) = 360 x_1 x_2 x_3^2 \left[ 1 + \frac{\omega _{3\pi } }{2} (7x_3-3) \right] , \nonumber \\ {}{} & {} \psi _\parallel (x_i) = 120x_1x_2x_3 \,\delta _{\pi }^2 \left[ \frac{21}{8} \omega _{4\pi } (x_1-x_2) \right] , \nonumber \\ {}{} & {} \psi _\perp (x_i) = 30x^2_3 \, \delta _\pi ^2 \left[ \frac{1}{3}(x_1-x_2) + \frac{21}{4} \omega _{4\pi }(x_1-x_2)(1-2x_3)\right] , \nonumber \\ {}{} & {} \tilde{\psi }_\parallel (x_i) = -120x_1x_2x_3 \delta _\pi ^2 \left[ \frac{1}{3} + \frac{21}{8}\omega _{4\pi }(1-3x_3) \right] , \nonumber \\ {}{} & {} \tilde{\psi }_\perp (x_i) = 30x_3^2 \, \delta _\pi ^2 \left[ \frac{1}{3}(1-x_3) +\frac{21}{4}\omega _{4\pi }(1-x_3)(1-2x_3) \right] , \end{aligned}$$
(20)

in which one more parameters \(\omega _{4 \pi }=0.20 \pm 0.10\) is introduced. With the bound conditions \(\varphi _\parallel (x_1=0/1,x_2,x_3) = 0\) and \(\varphi _\parallel (y_1,y_2=0/1,y_3)=0\), the auxiliary DAs \(\varphi _\parallel ^\dag (x_i)\) and \(\varphi _\parallel ^\dag (y_i)\) in Eq. (12) are defined by

$$\begin{aligned} \varphi _\parallel ^\dag (x_i){} & {} \equiv \int _0^{x_1} \, dx'_1 \, \varphi _\parallel (x'_1,x_2,x_3), \nonumber \\ \varphi _\parallel ^\dag (y_i){} & {} \equiv \int _0^{y_2} \, dy'_2 \, \varphi _\parallel (y_1,y'_2,y_3). \end{aligned}$$
(21)

The form factor with the contributions from 2p-to-2p and 3p-to-3p scattering, in Eqs. (11) and (12), can be rearranged as

$$\begin{aligned} \mathcal{F}_\pi ^{\textrm{pQCD}}(Q^2)= & {} \mathcal{F}_\pi ^{\textrm{2p}}(Q^2) + \mathcal{F}_\pi ^{\textrm{3p}}(Q^2) \nonumber \\ {}= & {} \mathcal{F}^{\textrm{pQCD}}_{\pi , \textrm{ t2}}(Q^2) + {\mathcal {F}}^{\textrm{pQCD}}_{\pi , \textrm{t3}}(Q^2) \nonumber \\ {}{} & {} + \mathcal{F}^{\textrm{pQCD}}_{\pi , \mathrm {t2 \otimes t4}}(Q^2) + \mathcal{F}^{\textrm{pQCD}}_{\pi , \textrm{3p}}(Q^2) , \end{aligned}$$
(22)

where the contributions arose from leading twist, twist three and twist four LCDAs associated to 2p configuration, as well as the contribution from 3p configuration of LCDAs. The dominate contributions come from 2p-to-2p scattering, and the pieces associated with leading and subleading 2p LCDAs can be decomposed in terms of the lowest Gegenbauer moment \(a_2^\pi \) and the chiral mass \(m_0^\pi \),

(23)

We note that the scale dependences of parameters \(a_2\) and \(m_0^\pi \) [34] have been absorbed into the expansion functions \(\mathcal{F}_{i=1-3}^{\textrm{t2}}\) and \(\mathcal{F}_{i=1-6}^\textrm{2p, t3}\), and the parameters in Eq. (23) are conventionally set at \(1 \, \textrm{GeV}\). We do not take into account the u, d quark masses in \(\mathcal{F}^\mathrm{2p, t2 \otimes t4}_\pi (q^2)\) and \(\mathcal{F}^{\textrm{3p}}_\pi (q^2)\), that’s why the quark mass correction terms and the \(m_0^{\pi }\) terms are not appeared in these pieces.

Fig. 2
figure 2

Left panel: Timelike form factor with the data samples [24] in \(s \in [4m_\pi ^2, s_{\textrm{max}} \simeq 8.7] \, \textrm{GeV}^2\) and the PQCD prediction up to \(\textrm{2p}\) twist three level in \([9, 30] \, \textrm{GeV}^2\). Right panel: Spacelike form factor obtained from the modified dispersion relation and from the direct pQCD calculations in \(Q^2 \in [10, 30] \, \textrm{GeV}^2\). The pQCD predictions in both plots are obtained by taking the testing choice of \(m_0^\pi (1 \, \textrm{GeV}) = 1.6 \pm 0.4 \, \textrm{GeV}\) and \(a_2^\pi (1 \, \textrm{GeV}) =0.25 \pm 0.25\), the effect from the scale evolutions are also taken in to account

Full size image

4 Result and discussion

In the left panel of Fig. 2, we depict the timelike form factor measured in the \(e^+e^-\) annihilation by BABAR collaboration, the high energy tail (magenta band) are supplemented by the pQCD prediction up to two particle twist three level \(\vert \mathcal{F}_{\pi , \mathrm{t2+t3}}^{\textrm{pQCD}}(s) \vert = \vert \mathcal{F}^\textrm{pQCD}_{\pi , \textrm{t2}}(s) + \mathcal{F}^{\textrm{pQCD}}_{\pi , \textrm{t3}}(s) \vert \). It is shown that the pQCD calculation marries with the data at the intermediate regions within uncertainty. In the right panel of Fig. 2, we depict the spacelike form factor obtained by the modified dispersion relation in Eq. (2). The full result \(\mathcal{F}_\pi ^{\textrm{DR2}}(Q^2)\) with considering the high energy tail from testing pQCD evaluation is shown by the blue band, and the partial result \(\mathcal{F}_\pi ^{\textrm{DR1}}(Q^2)\) without considering the high energy tail is shown in orange. It is found that the high energy tail gives a nonnegligible contribution, especially in the large momentum transfer regions. This could be traced to the logarithm expression of the timelike form factor in Eq. (2) which strengthens the role of high energy tail in the dispersion relation. For the sake of comparison, we also depict the direct pQCD calculations at the leading power (cyan band) and up to \(\textrm{2p}\) twist three level (magenta curve). The testing plot from one side reveals that the chiral enhancement effect from \(\textrm{2p}\) twist three LCDA is significant in pion form factor. From another side, it shows that the uncertainty of the result obtained by considering only the BABAR data is larger than the leading twist pQCD prediction \(\mathcal{F}^{\textrm{pQCD}}_{\pi , \textrm{ t2}}\). In light of this, the fit of Eq. (2) with the direct pQCD calculation of spacelike form factor can not arrive at a good result for \(a_2^\pi \) with well controlled errors. So we take the priori result \(a_2^\pi (1 \, \textrm{GeV}) = 0.25 \pm 0.25\) as a constraint to fit it and \(m_0^\pi \).

In this work we focus on the electromagnetic form factor and fit the spacelike form factor obtained from the modified dispersion relation and from the direct pQCD calculation. The minimal \(\chi ^2\) fitting is done in the large momentum transfer regions \(10 \le Q^2 \le 30 \, \textrm{GeV}^2\) where the pQCD calculation is applicable.

$$\begin{aligned} \chi ^2 = \sum _{i=1}^{11} \frac{\left[ \mathcal{F}_\pi ^{\textrm{DR2}}(Q_i^2) - \mathcal{F}_\pi ^{\textrm{pQCD}}(Q_i^2) \right] ^2}{\left[ \delta \mathcal{F}_\pi ^{\textrm{DR2}} (Q_i^2) \right] ^2}. \end{aligned}$$
(24)

The are two sources of uncertainty for \(\delta \mathcal{F}_\pi ^{\textrm{DR2}}\) in the denominator, one is the experimental data errors and the other one is the pQCD evaluation of high energy tail. We take eleven energy points staring from \(Q^2 = 10 \, \textrm{GeV}^2\) with the step width \(2 \, \textrm{GeV}^2\). The full pQCD prediction in Eq. (22) is rearranged in terms of the parameters \(m_0^\pi \) and \(a_2^\pi \) at default scale \(\mu _0 = 1 \, \textrm{GeV}\) as

$$\begin{aligned} \mathcal{F}_\pi ^{\textrm{pQCD}}(Q^2)= & {} (m_0^\pi )^2 F_1(Q^2) + m_0^\pi F_2(Q^2) + F_3(Q^2) \nonumber \\{} & {} + m_0^\pi a_2^\pi F_4(Q^2) + a_2^\pi F_5(Q^2) + (a_2^\pi )^2 F_6(Q^2) , \nonumber \\ \end{aligned}$$
(25)

in which the function \(F_3(Q^2)\) collects the contributions from the asymptotic term and partial high twists terms in Eq. (22).

Fig. 3
figure 3

The spacelike form factor obtained from the modified dispersion relation and the pQCD calculation with the new obtained parameters in scenario II

Full size image

The fitted results of \(m_0^\pi \) and \(a_2^\pi \) are listed in Table 2. We do the fit in two scenarios, the scenario \(\textrm{I}\) acquiesces the fixed scale \(1 \, \textrm{GeV}\) for the nonperturbative parameters in pion LCDAs, and in scenario \(\textrm{II}\) we consider the scale evolution of parameters in the pQCD evaluations. The factorization scale in pQCD calculation of hadron matrix element is formerly chosen at the largest internal virtuality. In order to examine the influence from the choice of factorization and renormalization scales, we vary it down and up by \(25 \%\) respecting to the conventional one in the scenario \(\textrm{II}\). The notations \(\textrm{IIA, IIB}\) indicate the fitted result obtained by taking the scales in pQCD calculation at \(3\mu _t/4\) and \(5\mu _t/4\), here \(\mu _t\) is the hard scale in the scattering and also the scales taken in scenario \(\textrm{II}\). We see that (a) the scale running of the nonperturbative parameters does not bring significant modification to the fit result, indicating that fixing them at the default scale in previous pQCD calculations is a reasonable treatment, (b) the variation of scale choice brings about \(20 \%\)-\(30 \%\) modification to the result of \(m_0^\pi \), which reveals the possible nonnegligible correction from the next-to-next-leading-order QCD correction to the form factor, (c) the fitted result of \(m_0^\pi \) is well under control with the current data accuracy, and the data-driven approach developed here to extract the nonperturbative parameters does not rely too much on the choice of factorization scale.

Table 2 Fitting results of \(m_0^\pi \) and \(a_2^\pi \) at the default scale \(1 \, \textrm{GeV}\). Scenario I (II) represents the fit with(out) considering the scale running of nonperturbative parameters in pQCD calculation, scenario IIA (IIB) indicates the fit with varying down (up) the renormalization and factorization scales by \(25\%\) in contrast to the conventional one taken in scenario II
Full size table

The data-driven approach developed here can not extract the gegenbauer coefficient \(a_2^\pi \) in the study of pion electromagnetic form factor. From the theoretical side, the first lattice endeavour by using the momentum smearing technique shows \(a_2^\pi (1 \, \textrm{GeV}) = 0.155^{+0.025}_{-0.027}\) with full control of all systematic errors [40], while the very recent lattice result by using large-momentum effective theory shows a noticeably larger value \(0.258^{+0.070}_{-0.052}\) [41]. It is also studied by the global PQCD fit at leading order (LO) with considering the well-explained hadronic two-body B decays [42], nevertheless, the result shows obvious difference with that obtained from QCD sum rules (QCDSRs) [34], dispersion derivation [43] and LQCD evaluations. We mark that the result obtained from the fit of B decays would firstly suffer large uncertainty from the inverse moment of B meson \(\lambda _B\), and secondly the fit is carried out at LO without considering the NLO corrections and power suppressed contributions [44].

We notice that the nonperturbative information on the shape of leading twist pion DA, saying \(a_n^\pi \), can be extracted from the measurement of the transition form factor where the sub-leading twist DAs do not contribute [45, 46]. In 2009, the BABAR collaboration reported their measurement of the \(Q^2 F_{\pi \gamma \gamma ^*}(Q^2)\) up to \(40 \, \textrm{GeV}^2\) [47], the result below \(Q^2 \sim 9 \, \textrm{GeV}^2\) are consistent with the those of CELLO [48] and CLEO [49], but the result above \(10 \, \textrm{GeV}^2\) are continues to grow up and deviates from the pQCD scaling behavior. This intriguing \(Q^2\) dependence created heat discussions on the “flat” shape of pion DA [50,51,52,53,54] and also the role of resonance contributions [55]. The excitement in the theory community is taken heat off until the new measurement released by Belle collaboration [37], whose result was close to the Brodsky–Lepage limit in the large \(Q^2 \in [20, 40] \, \textrm{GeV}^2\) and became to challenge the BABAR data. In our opinion, the discrepancy between BaBar and Belle result would be settle down with the foresee Belle-II [38] and BESIII measurements [39], and the transition form factor would then serves as the golden exclusive reaction to probe the shape of leading twist DA of pion meson.

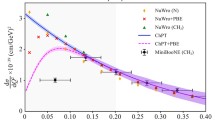
We depict in Fig. 3 the spacelike form factor obtained from the modified dispersion relation and from the pQCD calculation \(\mathcal{F}^{\textrm{pQCD}}_{\pi , \textrm{fit}}\) with the new fit parameters in the scenario II. The plot in the left panel presents the shape curves of pQCD functions associated with the parameters in our interesting, as shown in Eq. (25). The curves of functions \(m_0^\pi a_2^\pi F_{4}(Q^2)\) and \((a_2^\pi )^2 F_{6}(Q^2)\) are not presented since they are very close to zero. The plot in the right panel shows the pQCD result with the new fitted parameters, in contrast to the dispersion relation deduced result. In Fig. 4 we plot the form factor in the whole momentum transfer/invariant mass regions, where the direct measurements available in the regions \(q^2 \in [4m_\pi ^2, \simeq 8.70] \, \textrm{GeV}^2\) [24]. The dispersion relation deduced result and the pQCD prediction in the region with large \(\vert q^2 \vert \) are depicted by orange data-bar, blue and magenta bands, respectively. We can see that the new obtained pQCD prediction consists with BABAR data in the intermediate region much better than the testing pQCD calculation as shown in Fig. 2. Moreover, we present the direct measurement of spacelike form factor from \(\textrm{NA7}\) [20] and Jefferson Lab \(F_\pi \) collaborations [21, 22] in the region \(q^2 \in [-2.5, 0] \, \textrm{GeV}^2\), and also the precise LQCD evaluation (Green band) carried out in the large recoil regions \([-1, 0] \, \textrm{GeV}^2\) [3], this piece is enlarged and embodied in the top-left corner of the figure. It is shown a good agreement in the large recoiled region \(q^2 \in [-1, 0] \, \textrm{GeV}^2\) within theoretical uncertainties and experimental errors. Whereas in the region \(-2.5 \, \textrm{GeV}^2 \le q^2 \lesssim -1.0 \, \textrm{GeV}^2\), the dispersion relation deduced form factor lies slightly above the experimental points, this inconsistency is also happened in the LCSRs study where the high energy tail is parameterized by the duality resonant model [7]. The Jefferson Lab \(12 \, \textrm{GeV}\) program would say more in the intermediate momentum transfer regions.

Fig. 4
figure 4

The magnitude of pion electromagnetic form factor in the whole kinematic regions

Full size image

5 Summary

Inspired by the precise measurement of pion EM form factor in the resonant regions which marks up the missing piece without QCD-based calculation, we study the form factor with the modulus squared dispersion relation in which the line shapes in the large invariant mass/momentum transfer regions are calculated from pQCD approach. The main target of this work is to extract the chiral mass \(m_0^\pi \) and gegenbauer coefficient \(a_2^\pi \) in the LCDAs of pion meson, which are universal inputs in variable perturbative approaches. We fit the spacelike form factor obtained from the modulus squared dispersion relation with the direct pQCD calculation, and obtain the result \(m_0^\pi = \left( 1.31^{+0.27}_{-0.30} \right) \, \textrm{GeV}\) and \(a_2^\pi = 0.23 \pm 0.26\) at the default scale \(1 \, \textrm{GeV}\). In order to examine the influence from the choice of factorization and normalization scales, we vary them by \(25 \%\) respecting to the conventional one and find that the data-driven method formulated in this work is robust to extract the nonperturbative parameters. There are two directions we can strive for in this research. The first one is to increase the accuracy of measurement, especially in the region closing to \(s_{\textrm{max}}\), to reduce the uncertainty of spacelike form factor obtained from the dispersion relation, and hence improve the ability of this method to extract these parameters. Secondly, do the next-to-next-leading-order QCD correction to pion EM form factor to reduce the uncertainty from scales choice and improve the precision of pQCD calculation.