Abstract
Partons traversing the strongly interacting medium produced in heavy-ion collisions are expected to lose energy depending on their color charge and mass. We measure the nuclear modification factors for charm- and bottom-decay electrons, defined as the ratio of yields, divided by the number of binary nucleon–nucleon collisions, in \(\sqrt{s_{\textrm{NN}}}=200\) GeV Au+Au collisions to p+p collisions (\(R_{\textrm{AA}}\)), or in central to peripheral Au+Au collisions (\(R_{\textrm{CP}}\)). We find the bottom-decay electron \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\) to be significantly higher than those of charm-decay electrons. Model calculations including mass-dependent parton energy loss in a strongly coupled medium are consistent with the measured data. These observations provide evidence of mass ordering of charm and bottom quark energy loss when traversing through the strongly coupled medium created in heavy-ion collisions.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
Ultra-relativistic heavy-ion collision experiments at the Relativistic Heavy-Ion Collider (RHIC) and Large Hadron Collider (LHC) are unique in studying the strong interaction and underlying theory, Quantum Chromodynamics (QCD). Over the past decades, many experimental observations in these collisions have provided evidence that a novel QCD state of matter is created composed of de-confined quarks and gluons: the Quark-Gluon Plasma (QGP) [1,2,3,4,5]. Heavy quarks, i.e., charm and bottom quarks, in heavy-ion collisions have emerged as essential probes of the QGP because they have a rest mass much larger than the expected QGP temperature. This restricts their production to the initial hard parton scatterings in the collision, and therefore they can carry information about the entire QGP evolution [6,7,8,9].
Heavy quark energy loss in the produced medium is expected to proceed via (quasi-)elastic scatterings with the medium constituents and induced gluon radiation [10, 11]. Multiple elastic scatterings with the medium constituents also lead to a Brownian-like motion of low \(p_{\textrm{T}}\) heavy quarks. This transfers the collective motion of the expanding plasma to the heavy quark leading to large anisotropic flow. QCD predicts that heavy quarks lose less energy than light quarks due to the so-called “dead cone” effect [10, 11], i.e., gluon radiation is suppressed for heavy quarks at angles smaller than \(\theta _{c} \sim M/E\) where M and E are the quark mass and its energy, and that gluons lose more energy than light quarks due to their larger color factor. Therefore, parton energy loss (\(\Delta E\)) in the QGP is expected to follow a hierarchy ordered by parton color charge and mass, i.e., \(\Delta E(g)>\Delta E(u,d,s)>\Delta E(c)>\Delta E(b)\) with g, u, d, s, c, and b denoting gluons, up, down, strange, charm, and bottom quarks, respectively [10, 11]. Useful quantities to study parton energy loss are the nuclear modification factors, \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\). \(R_{\textrm{AA}}\) is defined as the particle yield in heavy-ion collisions divided by the respective yield in p+p collisions, scaled by the average number of binary nucleon–nucleon collisions (\(N_{\mathrm{coll.}}\)) in heavy-ion collisions:
\(R_{\textrm{CP}}\) is defined as the ratio of the yield in head-on heavy-ion (central) collisions to the yield in collisions with small nuclear geometric overlap (peripheral), scaled by a factor to account for the different \(N_{\mathrm{coll.}}\) in each case:
An observation of \(R_{\textrm{AA}}\) or \(R_{\textrm{CP}}\) that is equal to unity for heavy flavor hadrons would indicate heavy-ion collisions are an incoherent superposition of individual nucleon–nucleon collisions. It is worth noting that measurements of \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\) are affected by both the energy loss and the steepness of the spectrum shape. For given energy loss, the steeper the spectrum shape, the smaller the \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\).
At \(p_{\textrm{T}}<2\) GeV/c, a “flow hump” is seen in charm hadron \(R_{\textrm{AA}}\) due to the collective radial flow transferred to the charm quark while at \(p_{\textrm{T}}>\) 5 GeV/c, the charm hadron \(R_{\textrm{AA}}\) is much less than unity and is approximately the same as charged hadrons at sufficiently high \(p_{\textrm{T}}\) (> 8–10 GeV/c) [12,13,14,15,16,17]. The interpretation of this observation is complicated by the interplay of other effects not related to medium induced energy loss, for example differences in the fragmentation functions and \(p_{\textrm{T}}\) spectra [18,19,20,21,22]. The \(R_{\textrm{AA}}\) of bottom and charm hadrons are observed at the LHC to be similar within uncertainties for \(p_{\textrm{T}}\) larger than 7 GeV/c [16, 23]. Measurements at the LHC of bottom-decayed \(J/\psi \) and \(D^{0}\) \(R_{\textrm{AA}}\) at \(p_{\textrm{T}}<\) 20 GeV/c show a hint of energy loss mass ordering when compared to measurements of prompt \(D^{0}\) \(R_{\textrm{AA}}\) [24,25,26]. The ALICE and ATLAS collaborations report separate values for bottom- and charm-decayed lepton \(R_{AA}\) in the \(p_{\textrm{T}}\) interval [3,8] GeV/c that are consistent with the mass ordering of energy loss [27,28,29]. Models including the mass dependence of parton energy loss have predicted significantly different values of nuclear modification factors for bottom and charm hadrons and their decay leptons in heavy-ion collisions in the \(p_{\textrm{T}}\) ranges probed at RHIC [30,31,32,33]. Therefore, a comparison of charm and bottom hadron nuclear modification factors at RHIC is an excellent probe of the expected hierarchy of parton energy loss. The PHENIX experiment has reported a hint of a bottom- and charm- decayed electron \(R_{\textrm{AA}}\) separation in the \(p_{\textrm{T}}\) interval of [3,4] GeV/c [34, 35].
In this paper, we report the \(R_{\textrm{AA}}\) of electronsFootnote 1 from semileptonic decays of open charm and bottom hadrons in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}\) = 200 GeV. Additionally, we report the double-ratios of bottom- to charm-decay electron \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\). The Au+Au results presented here include the measurement of the inclusive heavy flavor hadron decayed electron (HFE) spectra and the fraction of bottom-decay electrons to the sum of bottom- and charm-decay electrons \({f_{b}^{AA}\equiv ~N( {b}\rightarrow {e})/N( {b}+ {c}\rightarrow {e})}\).
2 Experiment and data analysis
In this analysis, inclusive electrons consist of bottom- and charm-decay electrons, misidentified hadrons, photonic electrons from gamma conversions (\(\gamma \rightarrow e^+e^-\)) and Dalitz decays of \(\pi ^0\) and \(\eta \) mesons (\(\pi ^0/ \eta \rightarrow e^+e^-\gamma \)), hadron decayed electrons from prompt quarkonia, light vector mesons and kaon semi-leptonic decays (\(K_{\textrm{e3}}\)), and Drell–Yan contributions. The \(f_{b}^{AA}\) can be obtained by topologically separating the different electron sources in the inclusive electron sample by utilizing the distance of closest approach of the electron track with respect to the primary vertex [12]. The misidentified hadrons, photonic electrons, hadron decayed electrons, and Drell–Yan contribution are subtracted from the inclusive electrons in order to obtain inclusive HFE.
The data were collected by the Solenoidal Tracker At RHIC (STAR) experiment in 2014 and 2016. Minimum bias (MB) events are selected by requiring a coincidence between the Vertex Position Detectors (VPD) [36] just outside the beampipe on both ends of the STAR detector and covering the pseudorapidity (\(\eta \)) range of 4.24 \(< |\eta |<\) 5.1. An additional trigger is used to enrich the sample of high–\(p_{\textrm{T}}\) electrons by selecting events with a single Barrel ElectroMagnetic Calorimeter (BEMC) [37] tower above a transverse energy threshold \(E_{\textrm{T}}>\) 3.5 GeV (denoted high tower or HT). This data sample corresponds to an integrated luminosity of 0.2 and 1.0 nb\(^{-1}\) in 2014 and 2016 data, respectively. For the inclusive HFE measurement, the HT triggered data from the 2014 RHIC Run is utilized.
The main detectors used in the data analysis are the Time Projection Chamber (TPC) [38], Time-Of-Flight (TOF) detector [39], BEMC and Heavy Flavor Tracker (HFT) [40]. All of TPC, TOF and BEMC cover full azimuth within pseudo-rapidity range of \(|\eta | < 1\). The TPC, a gas-filled detector, provides the complete tracking of charged particles, and is used for momentum determination and particle identification (PID) via measuring ionization energy loss (dE/dx). The TOF employs Multi-gap Resistive Plate Chamber (MRPC) technology and is used for PID by measuring the flight time of charged particles. The BEMC, a lead-scintillator sampling calorimeter, can identify high-\(p_{\textrm{T}}\) electrons via the momentum to energy deposition ratio (p/E). The HFT, a four-layer high-resolution silicon vertex detector, provides a good track pointing resolution (\(\sim \) 46 \(\upmu \)m for 750 MeV/c kaons projected to the collision vertex) that enables the topological separation of charm- and bottom-decay electrons.
2.1 Fraction of bottom hadron decayed electrons
For the \(f_{b}^{AA}\) measurement, the primary vertex is required to be within 6 cm of the center of the STAR detector to ensure uniform tracking at mid-rapidity. Additionally, the difference between the TPC and VPD vertex z position is required to be less than 3 cm to reduce events with multiple collisions. After event selection there are about 1 billion and 1.1 billion MB events in the 2014 and 2016 data samples, respectively. Tracking of charged particles up to \(|\eta | < 1\) is achieved using the TPC inside a 0.5 T magnetic field. Tracks are selected by requiring a minimum of 20 hits, out of a maximum of 45, in the TPC, and the ratio of recorded over possible hits is greater than 0.52 to reduce fake combinations. To improve dE/dx resolution, the number of TPC hits used for dE/dx calculation is required to be at least 15. Additionally, a distance-of-closest approach (DCA) to the primary vertex of less than 1 cm is applied. Reconstructed tracks are then matched to hits in the HFT detector.
PID is conducted using a combination of the TPC, TOF, and the BEMC detectors. dE/dx information in the TPC is used to select electrons by requiring \(n\sigma _{e}\) to be between −1 and 3 for electrons, where \(n\sigma _{e}\) is defined as \(ln[(dE/dx)_{m}/(dE/dx)_{th}]/\sigma \) with \((dE/dx)_{m}\) and \((dE/dx)_{th}\) being the measured and expected dE/dx for electrons, respectively, and \(\sigma \) being the experimental resolution. For tracks with a \(p_{\textrm{T}}\) between 2 and 2.5 GeV/c the TOF information is used to enhance the electron purity by requiring \(|1/\beta -1|<0.025\), where \(\beta \) is a track’s velocity in units of speed of light. Finally, using the energy deposited in a single BEMC tower that is matched to the candidate electron track, we require the p/E to be between 0.3 and 1.5. Since electrons tend to deposit all of their energy this value peaks around unity for electrons and is able to discriminate against hadrons, which tend to deposit a fraction of their energy producing a p/E ratio larger than unity. Additional PID information is used in HT triggered data from the Shower Maximum Detectors (SMD) in the BEMC towers. The SMD is segmented in the \(\eta \) and \(\phi \) dimensions, and is able to distinguish between a broad electron and narrow hadron shower shape. We require the number of hits in both \(\eta \) and \(\phi \) to be greater than one.
A projective likelihood multivariate analysis (MVA) classifier, analogous to the TMVA method [41], is used to further separate electrons from hadrons, which are predominantly pions, in the MB data sample using probability distribution functions (PDF) of electrons and pions in each PID sub-detector. Control samples of electrons and pions are used to determine the PDFs, and are constructed from photonic electrons and \(K_{s}\rightarrow \pi ^{+}\pi ^{-}\) decays, respectively, using a tag-and-probe method. The likelihood is calculated as
where \(p_{i}^{e/\pi }\) are the particle probabilities, and i runs over all PID quantities. Equation 3 is further transformed as \({\mathscr {L}}\rightarrow -1/15 \cdot ln({\mathscr {L}}^{-1}-1)\) to have better discrimination between the signal and background peaks [41]. The variables used in the likelihood classifier are \(n\sigma _{e}\), p/E, \(1/\beta \) and the residuals in the \(\phi \) and z dimensions of the track projected onto the BEMC cluster center.
The likelihood distribution for electrons and pions with no PID selections are shown in Fig. 1 for 2014 data; similar distributions are observed for 2016 data. An optimization method is performed to maximize the electron purity without significantly reducing the signal efficiency, and corresponds to likelihood selections greater than 0.45 and 0.39 for 2014 and 2016 data, respectively. The hadron fractions before and after the likelihood selection are shown in Fig. 2 for the remaining MB triggered sample, after taking out electron candidates that fire the HT trigger, in 2014 and 2016 data. It is observed that the likelihood selection provides a clear improvement over the standard rectangular selections. There are significant differences between the hadron fractions in 2014 and 2016 data. These are due to the STAR trigger configurations. In 2016, the high-tower triggers were utilized during most of the data taking and had significant overlap with the minimum bias triggers. Since the high-towers were designed to trigger on electrons in the \(p_{\textrm{T}}\) range used for this measurement, and additional particle identification from the shower maximum detectors could then be utilized, most electrons in minimum bias events are re-classified into the high-tower triggers resulting in a lower electron purity for the remaining MB triggered sample. In contrast, for 2014 data this was not the case, and the high-tower trigger sample represents a small fraction of the total data set. The efficiencies for the likelihood selections after standard PID selections, determined in the electron control sample, are shown in Fig. 3 for 2014 and 2016 data, and are greater than 87% in the \(p_{\textrm{T}}\) range used in this measurement. Therefore, the additional likelihood selection significantly reduces the hadron background without compromising the statistical precision of the signal electron sample. For 2014 and 2016 HT samples, the electron purity varies from 83% to 92% and 85% to 95% at 3.5 \(<p_{\textrm{T}}<\) 8.5 GeV/c, respectively.
We additionally reject electron candidates for which we find an oppositely charged electron in the event that produces a di-electron invariant mass lower than 0.15 GeV/\(c^{2}\) to reduce photonic electron backgrounds. After electron identification requirements, the electron purity is greater than 80% across all measured electron \(p_{\textrm{T}}\). Electron candidates are defined as tracks that pass all the above criteria.
Fit to the \(\textrm{log}_{10}\)(DCA/cm) of candidate electrons with \(p_{\textrm{T}}\in \) [3.5,4.5] GeV/c in 2014 data. The solid blue line shows the full template fit, and the various other lines show the individual components. The bottom panel shows the residual distribution of the template fit scaled by the statistical uncertainties
We measure \(f_{b}^{AA}\) in intervals of electron \(p_{\textrm{T}}\) by performing a four-component-template likelihood fit to the \(\textrm{log}_{10}\)(DCA/cm) distribution of candidate electrons, where the DCA is defined as the 3D distance-of-closest approach of the track to the primary vertex. The hadron templates are taken from a sample of tracks selected with pion PID. The templates for residual photonic electrons are determined by embedding simulated detector hits of the decays of \(\pi ^{0}\), \(\eta \), and photons in real data, and applying the same reconstruction and selection as data. The charm- and bottom-decay electron templates are constructed using the data-driven fast simulation technique described in Ref. [12]. All abundant ground states are included in the simulation. The initial charm hadron \(p_{\textrm{T}}\) spectra are taken from the measured \(D^{0}\) spectra in Ref. [12], and the relative hadron fractions are from available data for \(D^{\pm }_{S}/D^{0}\) [42] and \(\Lambda ^{\pm }_{c}/D^{0}\) [43] or PYTHIA [44] for \(D^{\pm }/D^{0}\). The PYTHIA calculation for \(D^{\pm }/D^{0}\) is consistent with the ALICE measurement in Pb+Pb collisions [15]. The bottom hadron spectra are taken from Fixed Order plus Next-to-Leading Logarithms (FONLL) calculations [45, 46], and we assume equal proportions of \(B^{0}\) and \(B^{\pm }\). The relative \(B_{s}^{0}\) and \(\Lambda _{b}^{0}\) fractions are taken from Ref. [47]. We include the contributions from \(b\rightarrow c\rightarrow e\) decays in the bottom-decay electron templates. Due to the long and nearly identical bottom hadron lifetimes, we are not significantly sensitive to the bottom relative fractions. The decay-electron \(\textrm{log}_{10}\)(DCA/cm) distributions for all heavy flavor hadron species that are considered in the fast simulation are shown in Fig. 4 for an electron \(p_{\textrm{T}}\in \) [2.0,8.5] GeV/c. Potential backgrounds from Drell–Yan and prompt quarkonia are absorbed in the hadron template, as they produce electrons that point to the primary vertex. \(K_{\textrm{e3}}\) have DCA values outside the fit range considered. The only constrained normalization in the fit is the residual photonic electron template, which is estimated using a similar procedure as in Ref. [48] to calculate the residual photonic electron yield. This yield relative to the candidate electron sample ranges from 25% to 15% from low to high \(p_{\textrm{T}}\), respectively. An example fit to 2014 data using the described templates is shown in Fig. 5.
The systematic uncertainties on the measured \(f_{b}^{AA}\) fractions are: (1) the uncertainty on the simulated photonic electron cocktail composition is estimated by varying the relative proportions of electrons from photon conversions and light-meson decays by \(\sim \) 50%, and ranges from 8% at low \(p_{\textrm{T}}\) to less than 1% above 3.5 GeV/c; (2) the uncertainty on the residual photonic electron template normalization is estimated by allowing it to vary by an absolute 5%, and is about 3%; (3) the charm hadron \(p_{\textrm{T}}\) spectra uncertainty is taken from the measured \(D^{0}\) spectra, and is about 3%; (4) the \(D^{\pm }\)/\(D^{0}\) ratio uncertainty is 16% from the differences in PYTHIA 6 and 8, and is 1–3%; (5) the \(\Lambda _{c}^{\pm }\)/\(D^{0}\) ratio is varied using the different models shown in Ref. [43], and the corresponding uncertainty is less than 1%; (6) the uncertainty on the electron identification is estimated by tightening the selection, and is 2–3%; (7) the bottom hadron \(p_{\textrm{T}}\) spectrum uncertainty is estimated by applying both \(b\rightarrow e\) and \(c\rightarrow e\) \(p_{\textrm{T}}\) dependent \(R_{\textrm{AA}}\) calculated in the Duke model [31] described below, and is found to produce a maximum relative deviation of 2.5% that is assigned across all electron \(p_{\textrm{T}}\) intervals.
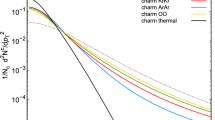
Invariant yield of the electrons from decays of prompt \(J/\psi \) (dot-dashed line), \(\Upsilon \) (dotted line), Drell–Yan (long dashdotted line) and light vector mesons (long dashed line) in 0–80% Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}\) = 200 GeV. The bands represent systematic uncertainties
2.2 Inclusive heavy flavor hadron decayed electrons
The invariant yield of inclusive HFE is measured using the same method as in Ref. [48]. Compared to the \(f_{b}^{AA}\) analysis, the notable differences are: (1) the primary vertex is required to be within 30 cm of the center of the STAR detector; (2) HFT hits are not included in track reconstruction; (3) maximum track DCA is 1.5 cm; and (4) \(n\sigma _{e}\) is chosen from −1.5 to 3.0. The inclusive electron yield is first corrected for the mis-identified hadron contamination, which is a 4% contribution at low \(p_{\textrm{T}}\) and 19% at high \(p_{\textrm{T}}\). The photonic electron background is then subtracted using a data-driven method where low-mass electron pairs with opposite charges are reconstructed in data and efficiency-corrected to estimate the photonic electron yield, in which maximum di-electron mass is 0.24 GeV/\(c^{2}\) and the minimum partner electron \(p_{\textrm{T}}\) is 0.3 GeV/c. The background-subtracted electron sample is then corrected for the tracking, PID, and trigger efficiencies which are calculated using the embedding technique. The total efficiency is 5% at low \(p_{\textrm{T}}\) and 20% at high \(p_{\textrm{T}}\).
We finally subtract the electron contributions from quarkonia, vector mesons, and Drell–Yan. The prompt \(J/\psi \) decay background is subtracted using inclusive data [49] with corrections to bottom hadron-decayed \(J/\psi \) using FONLL with Color Evaporation Model (CEM) calculations [50, 51]. EvtGen [52] is used to model the decay kinematics of quarkonium. Drell–Yan and Upsilon contributions are subtracted using \(N_{\mathrm{coll.}}\)-scaled PYTHIA and EvtGen, respectively, with the former taking no account of the nuclear and shadowing effects and the latter also incorporating the suppression model from [53]. The light-meson decays are estimated using \(m_{\textrm{T}}\)-scaling of the \(\pi ^{0}\) data [54,55,56]. PYTHIA and EvtGen are used to model the electron decay channel of \(\rho \), and \(\omega \) and \(\phi \), respectively. The \(K_{\textrm{e3}}\) also have a contribution to HFE yield, however, STAR simulation studies have shown that the \(K_{\textrm{e3}}\) contribution is less than 2% at \(p_{\textrm{T}}>\) 3 GeV/c in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}\) = 200 GeV [57] and thus can be neglected. The obtained invariant yields of these contributions in the 0–80% centrality interval of Au+Au collisions are shown in Fig. 6. These contributions amount to a \(\sim \) 20% reduction to the electron yield in the measured \(p_{\textrm{T}}\) region.
The systematic uncertainties considered in the inclusive heavy flavor electron production measurement, which in general increase with increasing \(p_{\textrm{T}}\), are the following. The electron reconstruction efficiency is evaluated by: (1) varying the required number of hits in the TPC for track reconstruction and dE/dx from 20 and 15, to 25 and 18; (2) varying the maximum track DCA from 1.5 cm to 1.0 cm; and (3) varying the p/E ratio in the BEMC between \(0.6<p{/}E<1.5\) and \(0.3<p{/}E<1.8\). The trigger efficiency uncertainty is estimated by varying the ADC trigger threshold in simulation by 3.5%. The electron purity uncertainty and the efficiency of the electron identification selection are estimated by varying the mean and width parameters from the Gaussian fit to the pure electron \(n\sigma _{e}\) distribution within one standard deviation from their central values. In addition, the latter includes an uncertainty from varying the \(n\sigma _{e}\) selections from \(-1.5<n\sigma _{e}<3.0\) to \(-1.0<n\sigma _{e}<3.0\). The uncertainty on the photonic electron yield comes from the partner electron finding efficiency. The partner finding efficiency uncertainty is determined by varying the di-electron mass window from 0.24 to 0.15 GeV/\(c^{2}\), and by varying the minimum partner electron \(p_{\textrm{T}}\) from 0.3 to 0.2 GeV/c. The uncertainties from the spectra of \(\pi ^{0}\) and \(\eta \) mesons and their branching ratios, and from the partner electron tracking efficiency are also folded into the photonic electron uncertainty. The uncertainty from the quarkonia and vector meson subtraction comes from the uncertainty on the measured spectra, from the uncertainties on the FONLL+CEM calculations used to correct the inclusive \(J/\psi \) spectra for non-prompt \(J/\psi \), and from the uncertainty of the model calculation in Ref. [53] used to correct the Upsilon spectra. The uncertainty on the Drell–Yan contribution is evaluated in the same way as in Ref. [58], taking into account the uncertainty of \(N_{\mathrm{coll.}}\).
The obtained invariant yield of inclusive HFE (\(\frac{e^++e^-}{2}\)) in 0–80% centrality of Au+Au collisions is shown in Fig. 7.
The measured fraction of bottom hadron decayed electrons, \(f_{b}^{AA}\), in intervals of electron \(p_{\textrm{T}}\) in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}=\) 200 GeV. a \(f_{b}^{AA}\) in 0–80% Au+Au collisions compared with the PHENIX measurements [34], and the corresponding fraction \(f_{b}^{pp}\) in p+p collisions at \(\sqrt{s}=\) 200 GeV [59]. b \(f_{b}^{AA}\) in three different centrality intervals. The error bars show statistical uncertainties, and the brackets show the systematic ones. The dashed line shows the central value of the FONLL prediction [45, 46]. Data points are plotted along the x-axis at their respective bin centers, except 0–20% and 40–80% centrality classes, which are offset by 75 MeV/c for clarity
3 Results
From the likelihood fit to the \(\textrm{log}_{10}\)(DCA/cm) distribution of candidate electrons shown in Fig. 5, the measured values for \(f_{b}^{AA}\) from the combined 2014 and 2016 data samples are shown in Fig. 8a along with the PHENIX measurements [34] for the 0–80% centrality class, and are compared to p+p data [59] and FONLL predictions. These STAR results in Au+Au collisions are consistent within uncertainties with published PHENIX measurements [34]. The \(f_{b}^{AA}\) fractions in the 0–20%, 20–40%, and 40–80% centrality regions are shown in Fig. 8b. Centrality is defined using the charged particle multiplicity at midrapidity [60], and is related to the impact parameter of the colliding nuclei. The 0–20% class includes nuclear collisions with the greatest spacial overlap, while 40–80% denotes peripherally colliding nuclei. A clear centrality dependence is shown for \(p_{\textrm{T}}<\) 4.5 GeV/c, with significantly enhanced \(b\rightarrow e\) fractions in 0–80% and 0–20% collisions compared to p+p data and FONLL predictions. The results in 40–80% collisions are in good agreement with the p+p data and FONLL predictions.
a The inclusive heavy flavor electron \(R_{\textrm{AA}}^{incl.}\) (green squares) and the measurement from PHENIX (open circles) [61] in intervals of electron \(p_{\textrm{T}}\) in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}=\) 200 GeV. b The measured \(R_{\textrm{AA}}\) for bottom- (blue stars) and charm-decay (red diamonds) electrons in intervals of electron \(p_{\textrm{T}}\) in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}=\) 200 GeV. The open markers indicate values calculated using the PHENIX \(R_{\textrm{AA}}^{incl.}\). In all panels the error bars show the statistical uncertainties, and the brackets show the systematic ones. The shaded boxes on the data in b show the uncertainty due to \(R_{\textrm{AA}}^{incl.}\). Additional 8(\(\oplus \)8)% and 8.1% uncertainties from the \(N_{coll.}\) calculations (p+p luminosity) for the STAR and PHENIX \(R_{\textrm{AA}}\), respectively, are not shown. The Duke [31] and PHSD [62, 63] models are shown as the various lines in b. Data points are plotted along the x-axis at their respective bin centers, except the charm-decay electron data in b, which are offset by 50 MeV/c for clarity
The HFE nuclear modification factors, \(R_{\textrm{AA}}^{incl.}\), are calculated using the HFE production yields in Au+Au collisions shown in Fig. 7 and p+p reference data from Ref. [48], and are shown in Fig. 9a. The \(R_{\textrm{AA}}^{incl.}\) is compatible with the PHENIX measurement [61]. We decompose the charm- and bottom-decay electron \(R_{\textrm{AA}}\) using the measured fractions \(f_{b}\) in Au+Au and p+p collisions: \(R_{\textrm{AA}}^{b\rightarrow e} = f_{b}^{AA}/f_{b}^{pp} \times R_{\textrm{AA}}^{incl.}\) and \(R_{\textrm{AA}}^{c\rightarrow e} = (1-f_{b}^{AA})/(1-f_{b}^{pp}) \times R_{\textrm{AA}}^{incl.}\). For the \(p_{\textrm{T}}\in \) [2.5,3.5] GeV/c, we use the PHENIX inclusive heavy flavor electron measurement. The charm- and bottom-decay electron \(R_{\textrm{AA}}\) values are shown in Fig. 9b.
The ratios of bottom- and charm-decay electron \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\) are shown in Fig. 10a, b, respectively. Note that the ratios do not depend on \(R_{\textrm{AA}}^{incl.}\). The data show that bottom-decay electron \(R_{\textrm{AA}}\) compared to charm-decay electrons are systematically larger, with a central value about 80% larger. The \(R_{\textrm{CP}}\) ratios for \(R_{\textrm{CP}}(0{-}20\%/40{-}80\%)\) and \(R_{\textrm{CP}}(0{-}20\%/20{-}40\%)\) show a more significant deviation from unity compared to \(R_{\textrm{AA}}\) because the systematic uncertainties largely cancel in the ratio.
a The \(R_{\textrm{AA}}\) ratio of bottom- to charm-decay electrons in intervals of electron \(p_{\textrm{T}}\) in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}=\) 200 GeV. b The \(R_{\textrm{CP}}\) ratios of bottom-decay electrons to that of charm-decay electrons in intervals of electron \(p_{\textrm{T}}\) in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}}=\) 200 GeV. The red diamonds show the ratios of \(R_{\textrm{CP}}(0{-}20\%/40{-}80\%)\), and the blue circles show the ratios of \(R_{\textrm{CP}}(0{-}20\%/20{-}40\%)\). In all panels the error bars and the brackets show statistical and systematic uncertainties, respectively. The Duke [31] and PHSD [62, 63] models are shown as the various lines. The null hypothesis calculations are shown as the shaded bands (see text for details). \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}(0{-}20\%/40{-}80\%)\) points are plotted along the x-axis at their respective bin centers and \(R_{\textrm{CP}}(0{-}20\%/20{-}40\%)\) is shifted by 75 MeV/c for clarity
The kinematic shift of semileptonic decays, heavy quark production spectra, and the heavy flavor hadrochemistry may cause the decay-electron double ratios of \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\) to differ from unity in the case that the bottom and charm hadron \(R_{\textrm{AA}}\)/\(R_{\textrm{CP}}\) are the same. We incorporate these effects in a null hypothesis for the ratios of bottom- and charm-decay electron nuclear modification factors using simulated charm and bottom hadron decays with the same \(p_{\textrm{T}}\) dependent \(R_{\textrm{AA}}\) or \(R_{\textrm{CP}}\), which have initial spectra from perturbative QCD [45, 46]. The abundances of \(\Lambda _{c/b}\) are matched to data in Refs. [47, 64]. We then multiply the hadron spectra for both charm and bottom hadrons by the \(p_{\textrm{T}}\) dependent Duke model \(R_{\textrm{AA}}\) or \(R_{\textrm{CP}}\) values for D mesons shown in [12], and propagate to the final state electrons. We then take the ratios of bottom- and charm-decay electron nuclear modification factors. In Fig. 10, these are shown as the shaded bands labeled “Null Hyp.”. We performed systematic variations by changing the \(D(B)_{s}\) and \(\Lambda _{c(b)}\) fractions by 50% in both p+p and Au+Au, and the Duke model \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\) values by a relative 25% in each case. The most significant effect comes from the charm baryon fractions in p+p and Au+Au collisions.
We perform a null hypothesis t-test, including data and null hypothesis model uncertainties, and find the bottom-to-charm \(R_{\textrm{AA}}\) ratios are consistent with the null hypothesis in the \(p_{\textrm{T}}\in \) [2.5,4.5] GeV/c range. The bottom-to-charm \(R_{\textrm{CP}}\)(0–20%/40–80%) and \(R_{\textrm{CP}}\)(0–20%/20–40%) ratios in the \(p_{\textrm{T}}\in \) [2.0,4.5] GeV/c range reject the null hypothesis at 4.2 and 3.3 standard deviations, respectively. In all t-tests, bin-by-bin correlations of systematic uncertainties in data are included. The PHENIX experiment also measured the \(R_{\textrm{AA}}\) of bottom- and charm-decay electrons for different centrality bins in Au+Au collisions at \(\sqrt{s_{\textrm{NN}}} = 200\,\,\mathrm GeV\) [34, 35], and found that charm-decay electrons are more suppressed than bottom-decay electrons with a significance of at least one standard deviation for 0–40% central collisions. Compared to PHENIX \(R_{\textrm{AA}}\) results, our \(R_{\textrm{CP}}\) measurements with a significance larger than 3 standard deviations have a much improved precision.
We compare our results of charm- and bottom-decay electron nuclear modification factors to theoretical models describing the heavy-quark dynamics in the de-confined medium in Figs. 9 and 10. The curves denoted “PHSD” show the Parton-Hadron-String-Dynamics model [62, 63] and the “Duke” curves show a modified Langevin transport model [31]. Both models include heavy quark diffusion in the QGP medium, heavy quark hadronization through coalescence and fragmentation, and mass-dependent energy loss mechanisms. In our measured electron \(p_{\textrm{T}}\) region, the corresponding parent heavy flavor hadrons \(\langle p_{\textrm{T}}\rangle \) covers about \(4-8\) GeV/c, where the contribution from heavy quark collectivity to the measured \(R_{\textrm{AA}}/R_{\textrm{CP}}\) is negligible [12]. Additionally, the modification in heavy flavor hadrochemistry in Au+Au collisions does not play a significant role to account for the observed \(R_{\textrm{AA}}/R_{\textrm{CP}}\) suppression. This is because the coalescence contribution is expected to be small in the measured \(p_{\textrm{T}}\) region [31, 65], and the contribution from heavy flavor strange mesons and heavy flavor baryons to the electrons is small [66]. We find that both models can reproduce the bottom- and charm-decay electron \(R_{\textrm{AA}}\) and ratios of \(R_{\textrm{CP}}\), suggesting the mass ordering of parton energy loss in the QGP medium.
4 Summary
We have measured \(R_{\textrm{AA}}\) of inclusive heavy flavor-decay electrons, and separately for bottom- and charm-decay electrons in the \(p_{\textrm{T}}\) range of 2 to 8.5 GeV/c in \(\sqrt{s_{\textrm{NN}}}\) = 200 GeV Au+Au collisions. In addition, we have measured the double ratios of bottom- and charm-decay electron \(R_{\textrm{AA}}\) and \(R_{\textrm{CP}}\). We find the measured values of bottom-decay nuclear modification factors are systematically larger than those of charm-decay electrons after accounting for effects not related to parton energy loss. The significance of this observation, in the \(p_{\textrm{T}}\) range of 2 to 4.5 GeV/c, is 4.2 and 3.3 standard deviations for the double ratios of \(R_{\textrm{CP}}(0{-}20\%/40{-}80\%)\) and \(R_{\textrm{CP}}(0{-}20\%/20{-}40\%)\), respectively. Compared to the data, the Duke and PHSD models are compatible within experimental uncertainties. These observations represent a significant comparison of bottom and charm hadron energy loss in heavy-ion collisions at RHIC, and provide evidence of mass ordering of charm and bottom quark energy loss when traversing through the strongly coupled medium created in heavy-ion collisions.
Data Availability
This manuscript has associated data in a data repository. [Authors’ comment: https://www.hepdata.net/record/ins1978766].
Change history
01 June 2023
An Erratum to this paper has been published: https://doi.org/10.1140/epjc/s10052-023-11425-x
Notes
Unless specified otherwise, electrons referred here include both electrons and positrons.
References
J. Adams et al., Nucl. Phys. A 757(1), 102 (2005). https://doi.org/10.1016/j.nuclphysa.2005.03.085
I. Arsene et al., Nucl. Phys. A 757(1–2), 1 (2005). https://doi.org/10.1016/j.nuclphysa.2005.02.130
K. Adcox et al., Nucl. Phys. A 757(1–2), 184 (2005). https://doi.org/10.1016/j.nuclphysa.2005.03.086
B. Back et al., Nucl. Phys. A 757(1), 28 (2005). https://doi.org/10.1016/j.nuclphysa.2005.03.084
S.A. Bass, M. Gyulassy, H. Stöcker, W. Greiner, J. Phys. G Nucl. Part. Phys. 25(3), R1 (1999). https://doi.org/10.1088/0954-3899/25/3/013
Z. Lin, M. Gyulassy, Phys. Rev. C 51, 2177 (1995). https://doi.org/10.1103/PhysRevC.51.2177
M. Cacciari, P. Nason, R. Vogt, Phys. Rev. Lett. 95, 122001 (2005). https://doi.org/10.1103/PhysRevLett.95.122001
R. Rapp, H. van Hees, Quark-Gluon Plasma 4, 111–206 (2010). https://doi.org/10.1142/9789814293297_0003
X. Dong, Y.J. Lee, R. Rapp, Annu. Rev. Nucl. Part. Sci. 69, 417 (2019). https://doi.org/10.1146/annurev-nucl-101918-023806
Y. Dokshitzer, D. Kharzeev, Phys. Lett. B 519(3), 199 (2001). https://doi.org/10.1016/S0370-2693(01)01130-3
R. Abir et al., Phys. Lett. B 715(1), 183 (2012). https://doi.org/10.1016/j.physletb.2012.07.044
J. Adam et al., Phys. Rev. C 99, 034908 (2019). https://doi.org/10.1103/PhysRevC.99.034908
B. Abelev et al., JHEP 2012(9), 112 (2012). https://doi.org/10.1007/JHEP09(2012)112
J. Adam et al., JHEP 2016(3), 81 (2016). https://doi.org/10.1007/JHEP03(2016)081
S. Acharya et al., JHEP 2018(10), 174 (2018). https://doi.org/10.1007/JHEP10(2018)174
A. Sirunyan et al., Phys. Lett. B 782, 474 (2018). https://doi.org/10.1016/j.physletb.2018.05.074
S. Acharya et al., JHEP 2022(1), 174 (2022). https://doi.org/10.1007/JHEP01(2022)174
R. Rapp et al., Nucl. Phys. A 979, 21 (2018). https://doi.org/10.1016/j.nuclphysa.2018.09.002
Z.B. Tang, W.M. Zha, Y.F. Zhang, Nucl. Sci. Technol. 31(8), 81 (2020). https://doi.org/10.1007/s41365-020-00785-8
A. Andronic et al., Eur. Phys. J. C 76(3), 107 (2016). https://doi.org/10.1140/epjc/s10052-015-3819-5
X. Dong, Y.J. Lee, R. Rapp, Annu. Rev. Nucl. Part. Sci. 69(1), 417 (2019). https://doi.org/10.1146/annurev-nucl-101918-023806
M. Djordjevic, Phys. Rev. Lett. 112, 042302 (2014). https://doi.org/10.1103/PhysRevLett.112.042302
A.M. Sirunyan et al., Phys. Rev. Lett. 119, 152301 (2017). https://doi.org/10.1103/PhysRevLett.119.152301
A.M. Sirunyan et al., Eur. Phys. J. C 78(6), 509 (2018). https://doi.org/10.1140/epjc/s10052-018-5950-6
A.M. Sirunyan et al., Phys. Rev. Lett. 123, 022001 (2019). https://doi.org/10.1103/PhysRevLett.123.022001
M. Aaboud et al., Eur. Phys. J. C 78(9), 762 (2018). https://doi.org/10.1140/epjc/s10052-018-6219-9
J. Adam et al., JHEP 07, 052 (2017). https://doi.org/10.1007/JHEP07(2017)052
G. Aad et al., Phys. Lett. B 829, 137077 (2022). https://doi.org/10.1016/j.physletb.2022.137077
S. Acharya et al., Phys. Lett. B 820, 136558 (2021). https://doi.org/10.1016/j.physletb.2021.136558
H. van Hees, M. Mannarelli, V. Greco, R. Rapp, Phys. Rev. Lett. 100, 192301 (2008). https://doi.org/10.1103/PhysRevLett.100.192301
S. Cao, G.Y. Qin, S.A. Bass, Phys. Rev. C 92, 024907 (2015). https://doi.org/10.1103/PhysRevC.92.024907
P.B. Gossiaux, J. Aichelin, Phys. Rev. C 78, 014904 (2008). https://doi.org/10.1103/PhysRevC.78.014904
M. Djordjevic, M. Djordjevic, Phys. Rev. C 90, 034910 (2014). https://doi.org/10.1103/PhysRevC.90.034910
A. Adare et al., Phys. Rev. C 93, 034904 (2016). https://doi.org/10.1103/PhysRevC.93.034904
U. Acharya et al., (2022). https://doi.org/10.48550/arXiv.2203.17058. arXiv:2203.17058
W. Llope et al., Nucl. Instrum. Meth. A 522(3), 252 (2004). https://doi.org/10.1016/j.nima.2003.11.414
M. Beddo et al., Nucl. Instrum. Meth. A 499(2), 725 (2003). https://doi.org/10.1016/S0168-9002(02)01970-8
M. Anderson et al., Nucl. Instrum. Meth. A 499(2), 659 (2003). https://doi.org/10.1016/S0168-9002(02)01964-2
W. Llope, Nucl. Instrum. Meth. A 661, S110 (2012). https://doi.org/10.1016/j.nima.2010.07.086
G. Contin et al., Nucl. Instrum. Meth. A 907, 60 (2018). https://doi.org/10.1016/j.nima.2018.03.003
A. Hocker et al., (2007). https://doi.org/10.48550/arXiv.physics/0703039. arXiv:physics/0703039
J. Adam et al., Phys. Rev. Lett. 127, 092301 (2021). https://doi.org/10.1103/PhysRevLett.127.092301
J. Adam et al., Phys. Rev. Lett. 124, 172301 (2020). https://doi.org/10.1103/PhysRevLett.124.172301
T. Sjöstrand, S. Mrenna, P. Skands, Comput. Phys. Commun. 178(11), 852 (2008). https://doi.org/10.1016/j.cpc.2008.01.036
M. Cacciari, S. Frixione, N. Houdeau, M.L. Mangano, P. Nason, G. Ridolfi, JHEP 2012(10), 137 (2012). https://doi.org/10.1007/JHEP10(2012)137
M. Cacciari, M.L. Mangano, P. Nason, Eur. Phys. J. C 75(12), 610 (2015). https://doi.org/10.1140/epjc/s10052-015-3814-x
R. Aaij et al., Phys. Rev. D 100, 031102 (2019). https://doi.org/10.1103/PhysRevD.100.031102
M.S. Abdallah et al., Phys. Rev. D 105, 032007 (2022). https://doi.org/10.1103/PhysRevD.105.032007
J. Adam et al., Phys. Lett. B 797, 134917 (2019). https://doi.org/10.1016/j.physletb.2019.134917
A. Frawley, T. Ullrich, R. Vogt, Phys. Rep. 462(4), 125 (2008). https://doi.org/10.1016/j.physrep.2008.04.002
M. Cacciari, S. Frixione, P. Nason, JHEP 2001(03), 006 (2001). https://doi.org/10.1088/1126-6708/2001/03/006
D.J. Lange, BEAUTY2000, Proceedings of the 7th Int. Conf. on B-Physics at Hadron Machines (2001). https://doi.org/10.1016/S0168-9002(01)00089-4
B. Krouppa, A. Rothkopf, M. Strickland, Phys. Rev. D 97, 016017 (2018). https://doi.org/10.1103/PhysRevD.97.016017
B.I. Abelev et al., Phys. Rev. Lett. 97, 152301 (2006). https://doi.org/10.1103/PhysRevLett.97.152301
A. Adare et al., Phys. Rev. Lett. 101, 232301 (2008). https://doi.org/10.1103/PhysRevLett.101.232301
A. Adare et al., Phys. Rev. C 87, 034911 (2013). https://doi.org/10.1103/PhysRevC.87.034911
L. Adamczyk et al., Phys. Rev. C 95, 034907 (2017). https://doi.org/10.1103/PhysRevC.95.034907
C. Aidala et al., Phys. Rev. D 99, 072003 (2019). https://doi.org/10.1103/PhysRevD.99.072003
M.M. Aggarwal et al., Phys. Rev. Lett. 105, 202301 (2010). https://doi.org/10.1103/PhysRevLett.105.202301
B.I. Abelev et al., Phys. Rev. C 79, 034909 (2009). https://doi.org/10.1103/PhysRevC.79.034909
A. Adare et al., Phys. Rev. C 84, 044905 (2011). https://doi.org/10.1103/PhysRevC.84.044905
W. Cassing, E. Bratkovskaya, Phys. Rev. C 78, 034919 (2008). https://doi.org/10.1103/PhysRevC.78.034919
W. Cassing, E. Bratkovskaya, Nucl. Phys. A 831, 215 (2009). https://doi.org/10.1016/j.nuclphysa.2009.09.007
S. Acharya et al., JHEP 2018(4), 108 (2018). https://doi.org/10.1007/JHEP04(2018)108
S. Plumari, V. Minissale, S.K. Das, G. Coci, V. Greco, Eur. Phys. J. C 78(4), 348 (2018). https://doi.org/10.1140/epjc/s10052-018-5828-7
F. Si, X.L. Chen, L. Zhou, Y.F. Zhang, S.H. Zhang, X.Y. Ju, X.J. Li, X. Dong, N. Xu, Phys. Lett. B 805, 135465 (2020). https://doi.org/10.1016/j.physletb.2020.135465
Acknowledgements
We thank the RHIC Operations Group and RCF at BNL, the NERSC Center at LBNL, and the Open Science Grid consortium for providing resources and support. This work was supported in part by the Office of Nuclear Physics within the U.S. DOE Office of Science, the U.S. National Science Foundation, National Natural Science Foundation of China, Chinese Academy of Science, the Ministry of Science and Technology of China and the Chinese Ministry of Education, the Higher Education Sprout Project by Ministry of Education at NCKU, the National Research Foundation of Korea, Czech Science Foundation and Ministry of Education, Youth and Sports of the Czech Republic, Hungarian National Research, Development and Innovation Office, New National Excellency Programme of the Hungarian Ministry of Human Capacities, Department of Atomic Energy and Department of Science and Technology of the Government of India, the National Science Centre of Poland, the Ministry of Science, Education and Sports of the Republic of Croatia, German Bundesministerium für Bildung, Wissenschaft, Forschung and Technologie (BMBF), Helmholtz Association, Ministry of Education, Culture, Sports, Science, and Technology (MEXT) and Japan Society for the Promotion of Science (JSPS).
Author information
Authors and Affiliations
Consortia
Corresponding author
Additional information
The original online version of this article was revised: Three authors were missing. X. Bai, University of Science and Technology of China, https://en.ustc.edu.cn/. Y. Cheng and X. Wu, University of California, https://www.pa.ucla.edu/And. Also, the wrong institute is used for author Y. H. Leung. It should be: Y. H. Leung, University of Heidelberg, https://www.uni-heidelberg.de/en.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3. SCOAP3 supports the goals of the International Year of Basic Sciences for Sustainable Development.
About this article
Cite this article
Abdallah, M.S., Aboona, B.E., Adam, J. et al. Evidence of Mass Ordering of Charm and Bottom Quark Energy Loss in Au+Au Collisions at RHIC. Eur. Phys. J. C 82, 1150 (2022). https://doi.org/10.1140/epjc/s10052-022-11003-7
Received:
Accepted:
Published:
DOI: https://doi.org/10.1140/epjc/s10052-022-11003-7