Longitudinal structure function from the parton parameterization

Abstract

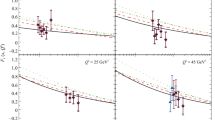
We present a certain theoretical model to describe data based on the DGLAP evolution equations at low values of x. This model is based on a hard-pomeron exchange in the next-to-next-to-leading order of the perturbative theory. The behavior of the DIS cross section ratio $R(x,Q^{2})$ and $F_{L}(x,Q^{2})/F_{2}(x,Q^{2})$ is studied and compared with the experimental data. These behaviors are controlled by the color dipole model bound. These results show good agreement with the DIS experimental data throughout the low values of x. The results can be applied to the LHeC region for analyses of ultra-high-energy processes.

Appendices

Appendix A

The coefficient functions for $F_{L}$ have the following forms [7]:

At LO:

$$\begin{aligned} c^{1}_{L,g}= & {} 8n_{f}z(1-z),\nonumber \\ c^{1}_{L,q}= & {} 4C_{F}z. \end{aligned}$$

(28)

At NLO:

$$\begin{aligned} c^{2}_{L,g}= & {} n_{f}\{(94.74-49.20z)z_{1}L_{1}^2+864.8z_{1}L_{1}\nonumber \\&+1161zL_{1}L_{0}+60.06zL_{0}^2+39.66z_{1}L_{0}\nonumber \\&-5.333(1/z-1)\},\nonumber \\ c^{2}_{L,q}= & {} 128/9zL_{1}^2-46.50zL_{1}-84.094L_{0}L_{1}-37.338\nonumber \\&+89.53z+33.82z^2+zL_{0}(32.90+18.41L_{0})\nonumber \\&-128/9L_{0}-0.012\delta (z_{1})+16/27n_{f}(6zL_{1}\nonumber \\&-12zL_{0}-25z+6)\nonumber \\&+n_{f}\{(15.94-5.212z)z_{1}^2L_{1}+(0.421+1.520z)L_{0}^2\nonumber \\&+28.09z_{1}L_{0}-(2.371/z-19.27)z_{1}^3\}. \end{aligned}$$

(29)

At NNLO:

$$\begin{aligned} c^{3}_{L,g}= & {} n_{f}\{(144L_{1}^4-47024/27L_{1}^3+6319L_{1}^2+53160L_{1})z_{1}\nonumber \\&+72549L_{0}L_{1}+88238L_{0}^2L_{1}+(3709-33514z\nonumber \\&-9533z^2)z_{1}+66773zL_{0}^2-1117L_{0}+45.37L_{0}^2\nonumber \\&-5360/27L_{0}^3-(2044.70z_{1}+409.506L_{0})1/z\}\nonumber \\&+n_{f}^2\{(32/3L_{1}^3-1216/9L_{1}^2-592.3L_{1}\nonumber \\&+1511zL_{1})z_{1}+311.3L_{0}L_{1}+14.24L_{0}^2L_{1}\nonumber \\&+(577.3-729z)z_{1}+30.78zL_{0}^3+366L_{0}+1000/9L_{0}^2\nonumber \\&+160/9L_{0}^3+88.50371/zz_{1}\}\nonumber \\&+fl_{11}^{g}n_{f}^2\{(-0.0105L_{1}^3+1.550L_{1}^2+19.72zL_{1}\nonumber \\&-66.745z+0.615z^2)z_{1}+20/27zL_{0}^4+(280/81\nonumber \\&+2.260z)zL_{0}^3-(15.40-2.201z)zL_{0}^2+2.260z)zL_{0}^3\nonumber \\&-(71.66-0.121z)zL_{0}\},\nonumber \\ c^{3}_{L,q}= & {} 512/27L_{1}^4-177.40L_{1}^3+650.6L_{1}^2-2729L_{1}\nonumber \\&-2220.5-7884z+4168z^2-(844.7L_{0}\nonumber \\&+517.3L_{1})L_{0}L_{1}+(195.6L_{1}-125.3)z_{1}L_{1}^3\nonumber \\&+208.3zL_{0}^3-1355.7L_{0}-7456/27L_{0}^2 -1280/81L_{0}^3\nonumber \\&+0.113\delta (z_{1})+n_{f}\{1024/81L_{1}^3-112.35L_{1}^2+344.1L_{1}\nonumber \\&+408.4-9.345z -919.3z^2+(239.7+20.63L_{1})z_{1}L_{1}^2\nonumber \\&+(887.3+294.5L_{0}-59.14L_{1})L_{0}L_{1}-1792/81zL_{0}^3\nonumber \\&+200.73L_{0}+64/3L_{0}^2+0.006\delta (z_{1})\}+n_{f}^2\{3zL_{1}^2\nonumber \\&+(6-25z)L_{1}-19+(317/6-12\zeta _{2})z -6zL_{0}L_{1}\nonumber \\&+6zLi_{2}(x))+9zL_{0}^2-(6-50z)L_{0}\}64/81\nonumber \\&+fl_{11}^{ns}n_{f}\{(107+321.05z-54.62z^2)z_{1}-26.717\nonumber \\&+9.773L_{0}+(363.8+68.32L_{0})zL_{0}\nonumber \\&-320/81L_{0}^2(2+L_{0})\}z\nonumber \\&+n_{f}\{(1568/27L_{1}^3-3968/9L_{1}^2+5124L_{1})z_{1}^2\nonumber \\&+(2184L_{0}+6059z_{1})L_{0}L_{1} -(795.6+1036z)z_{1}^2\nonumber \\&-143.6z_{1}L_{0}+2848/9L_{0}^2-1600/27L_{0}^3\nonumber \\&-(885.53z_{1}+182L_{0})1/zz_{1}\} +n_{f}^2\{(-32/9L_{1}^2\nonumber \\&+29.52L_{1})z_{1}^2+(35.18L_{0}+73.06z_{1})L_{0}L_{1}\nonumber \\&-35.24zL_{0}^2-(14.16-69.84z)z_{1}^2 -69.41z_{1}L_{0}\nonumber \\&-128/9L_{0}^2+40.2391/zz_{1}^2\}+fl_{11}^{ps}n_{f}\{(107\nonumber \\&+321.05z-54.62z^2)z_{1}-26.717+9.773L_{0}+(363.8\nonumber \\&+68.32L_{0})zL_{0}-320/81L_{0}^2(2+L_{0})\}z. \end{aligned}$$

(30)

In these equations we have used the abbreviations $z_{1}=1-z,~L_{0}=\ln {z}$ and $L_{1}=\ln {z_{1}}$. For the SU(N) gauge group, we have $C_{A}=N$, $C_{F}=(N^{2}-1)/2N$, $T_{F}=n_{f}T_{R}$, and $T_{R}=1/2$ where $C_{F}$ and $C_{A}$ are the color Cassimir operators in QCD. Also the new charge factors are defined by $fl_{11}^{ns}=3<e>$, $fl_{11}^{g}=<e>^{2}/<e^{2}>$ and $fl_{11}^{ps}=fl_{11}^{g}-fl_{11}^{ns}$.

Appendix B

The LO up to NNLO splitting functions for singlet and gluon distribution functions are as follows [52, 53]. At LO:

$$\begin{aligned}&P^{\mathrm{LO}}_{qq}(z)=C_{F}\left[ \frac{1+z^{2}}{(1-z)_{+}}+\frac{3}{2}\delta (1-z)\right] .\nonumber \\&P^{\mathrm{LO}}_{qg}(z)=\frac{1}{2}(z^{2}+(1-z)^{2}).\nonumber \\&P^{\mathrm{LO}}_{gq}(z)=C_{F}\frac{1+(1-z)^2}{z}.\nonumber \\&P^{\mathrm{LO}}_{gg}(z)=2C_{A}\left( \frac{z}{(1-z)_{+}}+\frac{(1-z)}{z}+z(1-z)\right) \nonumber \\&+\delta (1-z)\frac{(11C_{A}-4n_{f}T_{R})}{6}. \end{aligned}$$

(31)

The convolution integrals which contain a plus prescription, $()_{+}$, can be easily calculated by

$$\begin{aligned} \int _{x}^{1}\frac{dy}{y}f\left( \frac{x}{y}\right) _{+}g(y)= & {} \int _{x}^{1}\frac{dy}{y}f\left( \frac{x}{y}\right) \left[ g(y)-\frac{x}{y}g(x)\right] \nonumber \\&-g(x)\int _{0}^{x}f(y)dy. \end{aligned}$$

(32)

At NLO:

$$\begin{aligned} P_{qq}^{\mathrm{NLO}}= & {} (C_F)^2(-1+z+(1/2-3/2z)\ln (z)\nonumber \\&-1/2(1+z)\ln (z)^{2}-(3/2\ln (z)\nonumber \\&+2\ln (z)\ln (1-z))p_{qq}(z)\nonumber \\&+2p_{qq}(-z)S_2(z))+C_F C_A(14/3(1-z)\nonumber \\&+(11/6\ln (z)+1/2\ln (z)^{2}\nonumber \\&+67/18-\pi ^2/6)p_{qq}(z)-p_{qq}(-z)S_2(z))\nonumber \\&+C_FT_F(-16/3+40/3z+(10z+16/3z^2+2)\nonumber \\&\ln (z)-112/9z^2+40/(9z)-2(1+z)\ln (z)^{2}\nonumber \\&-(10/9+2/3\ln (z))p_{qq}(z)),\nonumber \\ P_{qg}^{\mathrm{NLO}}= & {} C_FT_F(4-9z-(1-4z)\ln (z)-(1-2z) \ln (z)^{2}\nonumber \\&+4\ln (1-z)+(2\ln ((1-z)/z)^{2}-4ln((1-z)/z)\nonumber \\&-2/3\pi ^2+10)P_{qg}(z))\nonumber \\&+C_AT_F(182/9+14/9z+40/(9z)\nonumber \\&+(136/3z-38/3)\ln (z)-4\ln (1-z)\nonumber \\&-(2+8z)\ln (z)^{2}+2P_{qg}(-z)S_2(z)\nonumber \\&+(-\ln (z)^{2}+44/3\ln (z)-2\ln (1-z)^{2}\nonumber \\&+4\ln (1-z)+\pi ^2/3-218/9)P_{qg}(z)),\nonumber \\ P_{gq}^{\mathrm{NLO}}= & {} C_F^2(-5/2-7z/2+(2+7/2z)\ln (z)\nonumber \\&-(1-z/2)ln(z)^{2} -2z\ln (1-z)-(3\ln (1-z)\nonumber \\&+\ln (1-z)^{2})P_{gq}(z))+C_FC_A(28/9+65/18z\nonumber \\&+44/9z^2 -(12+5z+8/3z^2)\ln (z)+(4+z)\ln (z)^{2}\nonumber \\&+2z\ln (1-z)+S_2(z)P_{gq}(-z)+(1/2-2\ln (z)\nonumber \\&\ln (1-z)+1/2\ln (z)^{2}+11/3\ln (1-z)\nonumber \\&+\ln (1-z)^{2}-\pi ^2/6)P_{gq}(z))\nonumber \\&+C_FT_F(-4/3z-(20/9+4/3\ln (1-z))P_{gq}(z)),\nonumber \\ P_{gg}^{\mathrm{NLO}}= & {} C_FT_F(-16+8z+20/3z^2+4/(3z)\nonumber \\&-(6+10z)\ln (z)-(2+2z)\nonumber \\&\ln (z)^{2})+C_AT_F(2-2z\nonumber \\&+26/9(z^2-1/z)-4/3(1+z)\ln (z)-20/9P_{gg}(z))\nonumber \\&+C_A^2(27/2(1-z)+26/9(z^2-1/z)\nonumber \\&-(25/3-11/3z+44/3z^2)\ln (z)+4(1+z)\nonumber \\&\ln (z)^{2}+2P_{gg}(-z)S_2(z)\nonumber \\&+(67/9-4\ln (z)\ln (1-z)\nonumber \\&+\ln (z)^{2}-\pi ^2/3)P_{gg}(z)), \end{aligned}$$

(33)

where

$$\begin{aligned}&p_{qq}(z)=2/(1-z)-1-z,\nonumber \\&p_{qq}(-z)=2/(1+z)-1+z,\nonumber \\&P_{qg}(z)=z^2+(1-z)^2,\nonumber \\&P_{qg}(-z)=z^2+(1+z)^2,\nonumber \\&P_{gq}(z)=(1+(1-z)^2)/z,\nonumber \\&P_{gq}(-z)=-(1+(1+z)^2)/z,\nonumber \\&P_{gg}(z)=1/(1-z)+1/z-2+z(1-z),\nonumber \\&P_{gg}(-z)=1/(1+z)-1/z-2-z(1+z),\nonumber \\&S_2(z)=\int _{1/(1+z)}^{z/(1+z)}1/y\ln ((1-y)/y)dy. \end{aligned}$$

(34)

At NNLO:

$$\begin{aligned} P_{qq}^{\mathrm{NNLO}}= & {} (n_f(-5.926L_{1}^3-9.751L_{1}^2-72.11L_{1} +177.4\nonumber \\&+392.9z-101.4z^2-57.04L_{0}L_{1}-661.6L_{0}\nonumber \\&+131.4L_{0}^2-400/9L_{0}^3+160/27L_{0}^4\nonumber \\&-506/z-3584/271/zL_{0})\nonumber \\&+n_f^2(1.778L_{1}^2+5.944L_{1}\nonumber \\&+100.1-125.2z+49.26z^2-12.59z^3\nonumber \\&-1.889L_{0}L_{1}+61.75L_{0}\nonumber \\&+17.89L_{0}^2+32/27L_{0}^3\nonumber \\&+256/811/z))(1-z).\nonumber \\ P_{qg}^{\mathrm{NNLO}}= & {} n_f(100/27L_{1}^4-70/9L_{1}^3-120.5L_{1}^2+104.42L_{1}\nonumber \\&+2522-3316z+2126z^2\nonumber \\&+L_{0}L_{1}(1823-25.22L_{0})-252.5zL_{0}^3\nonumber \\&+424.9L_{0} +881.5L_{0}^2-44/3L_{0}^3 +536/27L_{0}^4\nonumber \\&-1268.31/z-896/31/zL_{0})+n_f^2(20/27L_{1}^3\nonumber \\&+200/27L_{1}^2-5.496L_{1}-252+158z+145.4z^2\nonumber \\&-139.28z^3-L_{0}L_{1}(53.09+80.616L_{0})\nonumber \\&-98.07zL_{0}^2 +11.70zL_{0}^3-254L_{0}-98.80L_{0}^2\nonumber \\&-376/27L_{0}^3-16/9L_{0}^4+1112/2431/z).\nonumber \\ P_{gq}^{\mathrm{NNLO}}= & {} 400/81L_{1}^4+2200/27L_{1}^3+606.3L_{1}^2\nonumber \\&+2193L_{1}-4307\nonumber \\&+489.3z+1452z^2+146z^3-447.3L_{0}^2L_{1}\nonumber \\&-972.9zL_{0}^2+4033L_{0}-1794L_{0}^2+1568/9L_{0}^3\nonumber \\&-4288/81L_{0}^4+6163.11/z+1189.31/zL_{0}\nonumber \\&+n_f(-400/81L_{1}^3-68.069L_{1}^2-296.7L_{1}\nonumber \\&-183.8 +33.35z-277.9z^2+108.6zL_{0}^2\nonumber \\&-49.68L_{0}L_{1}+174.8L_{0}+20.39L_{0}^2+704/81L_{0}^3\nonumber \\&+128/27L_{0}^4-46.411/z+71.0821/zL_{0})\nonumber \\&+n_f^2(96/27L_{1}^2(1/z-1+1/2z)\nonumber \\&+320/27L_{1}(1/z-1+4/5z)\nonumber \\&-64/27(1/z-1-2z)), \nonumber \\ P_{gg}^{\mathrm{NNLO}}= & {} 2643.524D_{0}+4425.894\delta (1-z)\nonumber \\&+3589L_{1}-20852\nonumber \\&+3968z-3363z^2+4848z^3\nonumber \\&+L_{0}L_{1}(7305+8757L_{0})+274.4L_{0}-7471L_{0}^2\nonumber \\&+72L_{0}^3-144L_{0}^4+142141/z+2675.81/zL_{0}\nonumber \\&+n_f(-412.142D_{0}-528.723\delta (1-z)-320L_{1}\nonumber \\&-350.2+755.7z-713.8z^2+559.3z^3\nonumber \\&+L_{0}L_{1}(26.15-808.7L_{0})+1541L_{0}\nonumber \\&+491.3L_{0}^2+832/9L_{0}^3\nonumber \\&+512/27L_{0}^4+182.961/z\nonumber \\&+157.271/zL_{0})+n_f^2(-16/9D_{0}\nonumber \\&+6.4630\delta (1-z)-13.878\nonumber \\&+153.4z-187.7z^2+52.75z^3\nonumber \\&-L_{0}L_{1}(115.6-85.25z+63.23L_{0})-3.422L_{0}\nonumber \\&+9.680L_{0}^2-32/27L_{0}^3-680/2431/z), \end{aligned}$$

(35)

where $D_{0}=1/(1-z)$.

Appendix C

We present here the kernels for the quark and gluon sectors, denoted by $\Phi$ and $\Theta$, respectively, at LO up to NNLO which we used in Eqs. (19–21). We have

$$\begin{aligned} \Theta _{gq}(x,Q^{2})= & {} P_{gq}(x,\alpha _{s}){\odot } x^{\lambda _{s}},\nonumber \\ \Theta _{qg}(x,Q^{2})= & {} P_{qg}(x,\alpha _{s}){\odot } x^{\lambda _{g}},\nonumber \\ \Phi _{gg}(x,Q^{2})= & {} P_{gg}(x,\alpha _{s}){\odot } x^{\lambda _{g}},\nonumber \\ \Phi _{qq}(x,Q^{2})= & {} P_{qq}(x,\alpha _{s}){\odot } x^{\lambda _{s}}, \end{aligned}$$

(36)

where the splitting functions expanded into one, two and three loops correction in accordance with Appendix B. The required leading-order approximation of the singlet kernels are written

$$\begin{aligned} \Phi _{qq}(x,Q^{2})= & {} \frac{\alpha _{s}}{4\pi }\left\{ 4+\frac{16}{3}\ln \left( \frac{1-x}{x}\right) \right. \nonumber \\&\left. +\frac{16}{3}\int _{x}^{1}{\frac{z^{\lambda _{s}}-{z}^{-1}}{1-z}dz}\right. \nonumber \\&\left. -\frac{8}{3}\int _{x}^{1}{(1+z)z^{\lambda _{s}}dz}\right\} ,\nonumber \\ \Theta _{qg}(x,Q^{2})= & {} \frac{\alpha _{s}}{4\pi }\frac{20}{9}\int _{x}^{1}{(z^2+(1-z)^2)z^{\lambda _{g}}dz}. \end{aligned}$$

(37)

The longitudinal kernels at the low-x limit are given by

$$\begin{aligned} I_{L,q}(x,Q^{2})= & {} C_{L,q}(x,\alpha _{s}){\odot } x^{\lambda _{s}},\nonumber \\ I_{L,g}(x,Q^{2})= & {} C_{L,g}(x,\alpha _{s}){\odot } x^{\lambda _{g}}. \end{aligned}$$

(38)

Here the longitudinal coefficient functions $C_{L,a}(x,\alpha _{s}),~a=q,~g$ are the singlet and gluon functions known perturbatively up to the first few orders in the running coupling constant $\alpha _{s}$ and can be written as

$$\begin{aligned} C_{L,a}(x,\alpha _{s})= & {} \frac{\alpha _{s}}{4\pi }c^{1}_{L,a}(x)+\left( \frac{\alpha _{s}}{4\pi }\right) ^{2} c^{2}_{L,a}(x)\nonumber \\&\quad +\left( \frac{\alpha _{s}}{4\pi }\right) ^{3} c^{3}_{L,a}(x), \end{aligned}$$

(39)

where $c_{L,a}(x)$ are given in Appendix A. The required leading order approximation of the longitudinal singlet and gluon coefficient functions are written as

$$\begin{aligned} I_{L,q}(x,Q^{2})= & {} \frac{\alpha _{s}}{4\pi }\int _{x}^{1}8n_{f}(1-z)z^{\lambda _{s}+1}dz,\nonumber \\ I_{L,g}(x,Q^{2})= & {} \frac{\alpha _{s}}{4\pi }\int _{x}^{1}4C_{F}z^{\lambda _{g}+1}dz. \end{aligned}$$

(40)

Appendix D

The proton structure function parameterized with a global fit function [66] to the HERA combined data for $F^{\gamma p}_{ 2} (x,Q^{2})$ for $0.85< Q^{2} < 3000~ GeV^{2}$ and $x< 0.1$, which ensures that the saturated Froissart $\ln ^{2}(1/x)$ behavior dominates at low x. This global fit takes the form

$$\begin{aligned} F^{\gamma p}_{ 2} (x,Q^{2})= & {} (1- x)\left[ \frac{F_{P}}{1 -x_{P}} + A(Q^{2})\ln \left( \frac{x_{P}}{ x}\frac{1 - x}{ 1 - x_{P}}\right) \right. \nonumber \\&\left. +B(Q^{2}) \ln ^{2}\left( \frac{x_{P}}{ x}\frac{1 - x}{ 1 - x_{P}}\right) \right] , \end{aligned}$$

(41)

where

$$\begin{aligned} A(Q^{2}) = a_{0} + a_{1} {\ln }Q^{2} + a_{2} {\ln }^{2} Q^{2} \end{aligned}$$

and

$$\begin{aligned} B(Q^{2}) = b_{0} + b_{1} {\ln }Q^{2} + b_{2} {\ln }^{2} Q^{2}.\nonumber \end{aligned}$$

The fitted parameters are tabulated in Table 1. At low x (or large $\nu = \ln (1/x)$), the global fit becomes a quadratic polynomial in $\nu$, ${\widehat{F}}^{\gamma p}_{ 2} (\nu ,Q^{2}){\rightarrow } C_{0f} (Q^{2}) + C_{1f} (Q^{2})\nu + C_{2f} (Q^{2})\nu ^{2} + {\widehat{O}}(\nu )$, where the coefficient functions are defined in Ref. [66].

Table 1 Parameters of Eq. (41), resulting from a global fit to the HERA combined data

This form for $F_{2}^{{\gamma }p}$ (i.e. Eq. (41)) describes the HERA data well, but the model does not have the properties necessary for the $\gamma ^{*}-p$ reduced cross section to extend smoothly to the $Q^{2}=0$ limit. The authors in Ref. [20] provide good fits to the HERA data at low x and large $Q^{2}$ values. With respect to the Block–Halzen [67] fit, the explicit expression for the proton structure function in a range of the kinematical variables x and $Q^{2}$, $x<0.001$ and $0.15~\mathrm {GeV}^{2}<Q^{2}<3000~\mathrm {GeV}^{2}$, is defined by the following form:

$$\begin{aligned} F^{\gamma p}_{ 2}(x,Q^{2})= & {} D(Q^{2})(1- x)^{n}\left[ C(Q^{2})+A(Q^{2})\ln \left( \frac{1}{x}\frac{Q^{2}}{Q^{2}+\mu ^{2}}\right) \right. \nonumber \\&\left. +B(Q^{2})\ln ^{2}\left( \frac{1}{x}\frac{Q^{2}}{Q^{2}+\mu ^{2}}\right) \right] , \end{aligned}$$

(42)

where

$$\begin{aligned} A(Q^{2})= & {} a_{0} + a_{1} {\ln }\left( 1+\frac{Q^{2}}{\mu ^{2}}\right) + a_{2}{\ln }^{2}\left( 1+\frac{Q^{2}}{\mu ^{2}}\right) ,\nonumber \\ B(Q^{2})= & {} b_{0} + b_{1} {\ln }\left( 1+\frac{Q^{2}}{\mu ^{2}}\right) + b_{2}{\ln }^{2}\left( 1+\frac{Q^{2}}{\mu ^{2}}\right) ,\nonumber \\ C(Q^{2})= & {} c_{0} + c_{1} {\ln }\left( 1+\frac{Q^{2}}{\mu ^{2}}\right) ,\nonumber \\ D(Q^{2})= & {} \frac{Q^{2}\left( Q^{2}+\lambda M^{2}\right) }{(Q^{2}+M^{2})^2}. \end{aligned}$$

(43)

Here M is the effective mass and $\mu ^{2}$ is a scale factor. The additional parameters with their statistical errors are given in Table 2.

Table 2 The effective parameters at low x for $0.15~\mathrm {GeV}^{2}<Q^{2}<3000~\mathrm {GeV}^{2}$ provided by the following values. The fixed parameters are defined by the Block–Halzen fit to the real photon–proton cross section as $M^{2}=0.753 \pm 0.068~ \mathrm {GeV}^{2}$, $\mu ^2 = 2.82 \pm 0.290~ \mathrm {GeV}^{2}$ and $c_{0} = 0.255 \pm 0.016$ [20]