Skip to main content
SpringerLink
Log in

Self-Induced Suction of Fluid in a Turbulent Boundary Layer on a Permeable Surface

  • MATHEMATICAL PHYSICS
  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

Abstract

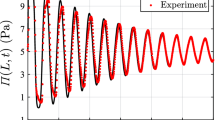
A turbulent boundary layer of a viscous incompressible fluid developing past a flat plate at finite distances from the laminar–turbulent transition zone is studied. It is assumed that the characteristic Reynolds number of the flow is large, while the thickness of the boundary layer is small. The problem is analyzed using the asymptotic method of multiple scales, which is used to find solutions of the Navier–Stokes equations. The velocity and pressure in the turbulent boundary layer are represented in the form of a sum of steady and perturbed terms, instead of traditional sums of time-averaged quantities and their fluctuations. It turns out that such a steady flow (called secondary) inside the turbulent boundary layer is determined by classical ideas and results of Reynolds and Kolmogorov without using any closure hypothesis. In terms of physics, this steady solution represents self-induced suction of the fluid from the outer flow to the turbulent boundary layer, which ensures kinetic energy transfer from the maximum-velocity zone to the basic part of the boundary layer. The found solution explains the concept of turbulent viscosity, since the solution is applicable on the scale of the boundary layer thickness. In the basic approximation, the zones of generation and dissipation of Kolmogorov vortices do not affect this steady solution. The features of solutions in the cases when the fluid flows into and out of the turbulent boundary layer through a permeable surface are studied. The obtained solutions are compared with available experimental data.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.
Fig. 5.
Fig. 6.

Similar content being viewed by others

REFERENCES

  1. V. B. Zametaev, A. R. Gorbushin, and I. I. Lipatov, “Steady secondary flow in a turbulent mixing layer,” Int. J. Heat Mass Transfer 132, 655–661 (2019).

    Article  MATH  Google Scholar 

  2. V. B. Zametaev and I. I. Lipatov, “Energy exchange in a compressible turbulent mixing layer,” J. Turbul. 22 (1), 48–77 (2021).

    Article  MathSciNet  Google Scholar 

  3. A. R. Gorbushin, V. B. Zametaev, and I. I. Lipatov, “Steady secondary flow in a plane turbulent free jet,” Fluid Dyn. 54 (2), 244–256 (2019).

    Article  MathSciNet  MATH  Google Scholar 

  4. V. B. Zametaev, “Modeling of the turbulent Poiseuille–Couette flow in a flat channel by asymptotic methods,” Comput. Math. Math. Phys. 60 (9), 1528–1538 (2020).

    Article  MathSciNet  MATH  Google Scholar 

  5. H. Reichardt, “Messungen turbulenter Schwankungen,” Naturwissenschaften 26, 404–408 (1938).

    Article  Google Scholar 

  6. G. B. Schubauer and P. S. Klebanoff, “Contributions on the mechanics of boundary layer transition,” NACA Tech. Rep., No. 1289 (1955).

  7. P. S. Klebanoff and Z. W. Diehl, “Some features of artificially thickened fully developed turbulent boundary layers with zero pressure gradient,” NACA Tech. Rep., No. 1110 (1952).

  8. P. S. Klebanoff, “Characteristics of turbulence in a boundary layer with zero pressure gradient,” NACA Tech. Rep., No. 1247 (1955).

  9. A. Gorbushin, S. Osipova, and V. Zametaev, “Mean parameters of an incompressible turbulent boundary layer on the wind tunnel wall at very high Reynolds numbers,” Flow Turbul. Combust. 107 (1), 31–50 (2021).

    Article  Google Scholar 

  10. O. Reynolds, “An experimental investigation of the circumstances which determine whether the motion of water shall be direct or sinuous, and of the law of resistance in parallel channels,” Phil. Trans. R. Soc. 174, 935–982 (1883).

    Article  MATH  Google Scholar 

  11. O. Reynolds, “On the dynamic theory of incompressible viscous fluids and the determination of the criterion,” Phil. Trans. R. Soc. A 186, 123 (1895).

    MATH  Google Scholar 

  12. L. Prandtl, “Bericht über Untersuchungen zur ausgebildeten Turbulenz,” Z. Angew. Math. Mech. 5, 136–139 (1925).

    Article  MATH  Google Scholar 

  13. T. von Kármán, “Mechanische Ahnlichkeit und Turbulenz,” Nachr. Ges. Wiss. Göttigen, Math.-Phys. 1, 58–76 (1930).

    MATH  Google Scholar 

  14. M. Hansen, “Die Geschwindigkeitsverteilung in der Grenzschicht an einer eigentauchten Platte,” Z. Angew. Math. Mech. 8 (2), 185–199 (1928).

    Article  Google Scholar 

  15. E. U. Repik and Yu. P. Sosedko, Turbulent Boundary Layer (Fizmatgiz, Moscow, 2007) [in Russian].

    MATH  Google Scholar 

  16. G. I. Taylor, “Statistical theory of turbulence I,” Proc. R. Soc. London A 151, 421–444 (1935).

    Article  MATH  Google Scholar 

  17. A. N. Kolmogorov, “The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers,” Dokl. Akad. Nauk SSSR 30 (4), 299–303 (1941).

    MathSciNet  Google Scholar 

  18. A. M. Obukhov, “On the spectral energy distribution in a turbulent flow,” Izv. Akad. Nauk SSSR, Ser. Geogr. Geofiz. 5, 453–466 (1941).

    Google Scholar 

  19. L. Prandtl, “Neuere Ergebnisse der Turbulenzforschung,” Z. Ver. Dtsch. Ing. 77, 105–114 (1933).

    MATH  Google Scholar 

  20. A. A. Izakson, “On the velocity distribution formulas near a wall,” Zh. Eksp. Teor. Fiz. 7, 919–924 (1939).

    Google Scholar 

  21. C. B. Millikan, “A critical discussion of turbulent flows in channels and circular tubes,” Proceedings of the 5th International Congress on Applied Mechanics (Wiley, New York, 1939), pp. 386–392.

  22. R. H. Kraichnan, “Inertial ranges in two-dimensional turbulence,” Phys. Fluids 10, 1417 (1967).

    Article  Google Scholar 

  23. S. I. Chernyshenko and M. F. Baig, “The mechanism of streak formation in near-wall turbulence,” J. Fluid Mech. 544, 99–131 (2005).

    Article  MathSciNet  MATH  Google Scholar 

  24. J. Jiménez, “Near-wall turbulence,” Phys. Fluids 25, 101302 (2013).

  25. P. Hall, “Vortex-wave interaction arrays: a sustaining mechanism for the log layer?” J. Fluid Mech. 850, 46–82 (2018).

    Article  MathSciNet  MATH  Google Scholar 

  26. A. S. Iyer, F. D. Witherden, S. I. Chernyshenko, and P. E. Vincent, “Identifying eigenmodes of averaged small-amplitude perturbations to turbulent channel flow,” J. Fluid Mech. 875, 758–780 (2019).

    Article  MathSciNet  MATH  Google Scholar 

  27. V. V. Sychev and Vik. V. Sychev, “On turbulent boundary layer structure,” J. Appl. Math. Mech. 51 (4), 462–467 (1988).

    Article  Google Scholar 

  28. P. A. Durbin and S. E. Belcher, “Scaling of adverse-pressure-gradient turbulent boundary layers,” J. Fluid Mech. 238, 699–722 (1992).

    Article  MATH  Google Scholar 

  29. T. S. Lundgren, “Asymptotic analysis of the constant pressure turbulent boundary layer,” Phys. Fluids 19, 055105 (2007).

  30. F. T. Smith, B. Scheichl, and A. Kluwick, “On turbulent separation,” J. Eng. Math. 68 (3), 373–400 (2010).

    Article  MathSciNet  MATH  Google Scholar 

  31. B. Scheichl, “Time-mean turbulent shear flows: Classical modelling—asymptotic analysis—new perspectives,” in Fluid and Solid Mechanics, Ed. by S. Bullett, T. Fearn, and F. Smith (World Scientific, Singapore, 2016), pp. 71–108.

    Google Scholar 

  32. I. I. Vigdorovich, “Turbulent thermal boundary layer on a plate: Reynolds analogy and heat transfer law over the entire range of Prandtl numbers,” Fluid Dyn. 52 (5), 631–645 (2017).

    Article  MathSciNet  MATH  Google Scholar 

  33. B. Scheichl and A. Kluwick, “Non-unique turbulent boundary layer flows having a moderately large velocity defect: A rational extension of the classical asymptotic theory,” Theor. Comput. Fluid Dyn. 27 (6), 735–766 (2013).

    Article  Google Scholar 

  34. J. C. R. Hunt, N. D. Sandham, J. C. Vassilicos, B. E. Launder, P. A. Monkewitz, and G. F. Hewitt, “Developments in turbulence research: A review based on the 1999 Program of the Isaac Newton Institute, Cambridge,” J. Fluid Mech. 436, 353–391 (2001).

    Article  MathSciNet  MATH  Google Scholar 

  35. Closure Strategies for Turbulent and Transitional Flows, Ed. by B. Launder and N. Sandham (Cambridge Univ. Press, Cambridge, 2002).

    MATH  Google Scholar 

  36. M. H. Buschmann and M. Gad-el-hak, “Recent developments in scaling of wall-bounded flows,” Prog. Aerospace Sci. 42, 419–467 (2007).

    Article  Google Scholar 

  37. I. V. Egorov, V. G. Sudakov, and A. V. Fedorov, “Numerical modeling of the perturbation propagation in a supersonic boundary layer,” Fluid Dyn. 39 (6), 874–884 (2004).

    Article  MATH  Google Scholar 

  38. Y. Duguet, P. Schlatter, and D. S. Henningson, “Formation of turbulent patterns near the onset of transition in plane Couette flow,” J. Fluid Mech. 650, 119–129 (2010).

    Article  MATH  Google Scholar 

  39. V. I. Borodulin, V. R. Gaponenko, Y. S. Kachanov, D. G. W. Meyer, U. Rist, Q. X. Lian, and C. B. Lee, “Late-stage transitional boundary-layer structures: Direct numerical simulation and experiment,” Theor. Comput. Fluid Dyn. 15, 317 (2002).

    Article  MATH  Google Scholar 

  40. H. Schlichting and K. Gersten, Boundary Layer Theory, 9th ed. (Springer, New York, 2017).

    Book  MATH  Google Scholar 

  41. M. R. Head, “Entrainment in the turbulent boundary layer,” Aeronaut. Res. Counc. Rep. Memo., No. 3152 (1960).

  42. V. B. Zametaev and A. R. Gorbushin, “Evolution of vortices in 2D boundary layer and in the Couette flow,” AIP Conf. Proc. 1770, 030044 (2016).

  43. A. R. Gorbushin and V. B. Zametaev, “Asymptotic analysis of viscous fluctuations in turbulent boundary layers,” Fluid Dyn. 53 (1), 9–20 (2018).

    Article  MathSciNet  MATH  Google Scholar 

  44. H. Schlichting, “Berechnung ebener periodischer Grenzschichtströmungen,” Phys. Z. 33, 327–335 (1932).

    MATH  Google Scholar 

  45. M. van Dyke, An Album of Fluid Motion (Parabolic, Stanford, California, 1982).

    Book  Google Scholar 

  46. C. C. Lin, “Motion in the boundary layer with a rapidly oscillating external flow,” Proceedings of the 9th International Congress on Applied Mechanics (Brussels, 1957), Vol. 4, pp. 155–167.

  47. T. J. Pedley, “Two-dimensional boundary layers in a free stream which oscillates without reversing,” J. Fluid Mech. 55, 359–383 (1972).

    Article  MATH  Google Scholar 

  48. V. I. Yudovich, “Example of the secondary steady flow in a laminar flow,” Prikl. Mat Mech. 29 (3), 453–467 (1965).

    Google Scholar 

  49. K. Stewartson, “The theory of unsteady laminar boundary layers,” Adv. Appl. Mech. 6, 1–37 (1960).

    Article  MathSciNet  MATH  Google Scholar 

  50. N. Riley, “Unsteady laminar boundary layers,” SIAM Rev. 17, 274–297 (1975).

    Article  MATH  Google Scholar 

  51. V. I. Kornilov and Yu. A. Litvinenko, “Skin friction measurements in an incompressible turbulent boundary layer: Part 1. Adverse pressure gradient,” Thermophys. Aeromech. 4, 475–491 (2001).

    Google Scholar 

  52. G. F. Oweis, E. S. Winkel, J. M. Cutbrith, S. L. Ceccio, M. Perlin, and D. R. Dowling, “The mean velocity profile of a smooth-flat-plate turbulent boundary layer at high Reynolds number,” J. Fluid Mech. 665, 357–381 (2010).

    Article  MATH  Google Scholar 

  53. S. A. Glazkov, A. R. Gorbushin, S. L. Osipova, and A. V. Semenov, “Influence of movable test section elements configuration on its drag and flow field uniformity at transonic speeds, AIP Conf. Proc. 1770, 030008 (2016).

  54. S. A. Glazkov, A. R. Gorbushin, V. B. Zametaev, S. L. Osipova, and A. V. Semenov, “Investigation of boundary layer on the walls of test section of the transonic wind tunnel T-128,” Proceedings of the 13th Russian Conference of Young Scientists on Problems in Mechanics: Theory, Experiment, and New Technologies, Novosibirsk-Sheregesh, Russia, March 15–22, 2019.

  55. V. Biryukov, S. Glazkov, A. Gorbushin, A. Ivanov, and A. Semenov, “Experimental investigation of the effect of nozzle shape and test section perforation on the steady and non-steady characteristics of flow field in the large transonic TsAGI T-128 wind tunnel,” Aeronaut. J. 109 (1092), 75–82 (2005).

    Article  Google Scholar 

  56. Lord Rayleigh, “On the stability, or instability, of certain fluid motions,” Sci. Papers Cambridge Univ. Press 1, 474–487 (1880).

    MATH  Google Scholar 

  57. P. G. Drazin and W. H. Reid, Hydrodynamic Stability, 2nd ed. (Cambridge Univ. Press, Cambridge, 2004).

    Book  MATH  Google Scholar 

  58. Yih Chia-Shun, “Surface waves in flowing water,” J. Fluid Mech. 51 (2), 209–220 (1972).

    Article  MATH  Google Scholar 

Download references

Funding

This work was performed at the Moscow Institute of Physics and Technology and was supported by the Russian Science Foundation, project no. 20-11-20006. Computer support was provided by the Central Aerohydrodynamic Institute and the Federal Research Center “Computer Science and Control” of the Russian Academy of Sciences.

Author information

Authors and Affiliations

  1. Moscow Institute of Physics and Technology (National Research University), 141701, Dolgoprudnyi, Moscow oblast, Russia

    A. R. Gorbushin, V. B. Zametaev, I. I. Lipatov, M. A. Fedotov & A. A. Khokhlov

  2. Federal Research Center “Computer Science and Control,” Russian Academy of Sciences, 119333, Moscow, Russia

    V. B. Zametaev

  3. Central Aerohydrodynamic Institute (TsAGI), National Research Center “Zhukovsky Institute”, 140180, Zhukovskii, Moscow oblast, Russia

    A. R. Gorbushin, V. B. Zametaev & I. I. Lipatov

Authors
  1. A. R. Gorbushin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. V. B. Zametaev
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. I. I. Lipatov
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. M. A. Fedotov
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. A. A. Khokhlov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. B. Zametaev.

Ethics declarations

The authors declare that they have no conflicts of interest.

Additional information

Translated by I. Ruzanova

APPENDIX A

APPENDIX A

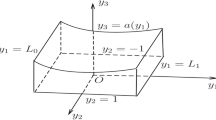
Substituting asymptotic expansions (2.5) into (2.1)–(2.4) and sequentially extracting the leading terms from the equations, we obtain the following problems for the first-order perturbations \({{v}_{1}},\,\,{{p}_{1}}\) and \({{w}_{1}},\,\,{{u}_{1}}\):

$$\begin{gathered} O\left( {\frac{{{{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{{{{\delta }^{2}}}}} \right)\,:\quad {{\nabla }^{2}}{{p}_{1}} + 2\frac{{\partial {{u}_{0}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{1}}}}{{\partial {{x}_{1}}}} = 0, \\ O\left( {\frac{{{{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{{{{\delta }^{2}}}}} \right)\,:\quad \frac{{\partial {{v}_{1}}}}{{\partial {{t}_{1}}}} + {{u}_{0}}\frac{{\partial {{v}_{1}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{p}_{1}}}}{{\partial {{y}_{1}}}} = 0, \\ {{y}_{1}} = 0\,:\quad {{v}_{1}} = 0,\quad \frac{{\partial {{p}_{1}}}}{{\partial {{y}_{1}}}} = 0;\quad y_{1}^{2} + x_{1}^{2} + z_{1}^{2} \to \infty \,:\quad {{p}_{1}} = O(1), \\ \end{gathered} $$
(A1a)
$$\begin{gathered} O\left( {\frac{{{{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{{{{\delta }^{2}}}}} \right)\,:\quad \frac{{\partial {{w}_{1}}}}{{\partial {{t}_{1}}}} + {{u}_{0}}\frac{{\partial {{w}_{1}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{p}_{1}}}}{{\partial {{z}_{1}}}} = 0, \\ O\left( {\frac{{{{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{{{{\delta }^{2}}}}} \right)\,:\quad \frac{{\partial {{u}_{1}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{v}_{1}}}}{{\partial {{y}_{1}}}} + \frac{{\partial {{w}_{1}}}}{{\partial {{z}_{1}}}} = 0. \\ \end{gathered} $$
(A1b)

As a result, we derive the inviscid system of equations introduced in [56] for describing the evolution of small perturbations. In the case of a two-dimensional basic velocity \({{u}_{0}}(x,{{y}_{1}})\), the equations for \({{v}_{1}},\,\,{{p}_{1}}\) decouple. Given \({{p}_{1}}\), the transverse fluctuations can be calculated using the corresponding momentum equation, while the streamwise fluctuations can be determined from the continuity equation.

Traditionally, solutions of the inviscid linear problem (A1) are sought in the form of normal modes \({{v}_{1}}\sim \exp (i\alpha ({{x}_{1}} - c{{t}_{1}}))\) with real \(\alpha \) (they will be referred to as solutions of type 1). If the basic velocity profile \({{u}_{0}}\) has no inflection point, then the spectrum of solutions of system (A1) is continuous, there are no growing discrete modes, and the phase velocity is real (see [57, 58]). Such solutions are bounded near the plate surface, in contrast to turbulent fluctuations, which increase as the wall is approached.

Additionally, another solution was found in [42]. It behaves singularly when tending to some point on the wall (referred to as a solution of type 2) and cannot be represented in the form of normal modes. Qualitatively, it resembles the behavior of turbulent fluctuations.

System (A1) is linear and contains derivatives only with respect to the fast variables \({{x}_{1}},\,\,{{y}_{1}},\,\,{{z}_{1}},\,\,{{t}_{1}}\). In the general case, the perturbations \({{v}_{1}},\,\,{{p}_{1}},\,\,{{u}_{1}}\) have to be sums of two-dimensional steady terms depending on the slow variable \(x\) and unsteady fast fluctuations (such steady solutions will be called solutions of type 3):

$$\begin{gathered} {{v}_{1}} = {{v}_{{10}}}(x,{{y}_{1}}) + {{v}_{{11}}}({{x}_{1}},{{y}_{1}},{{z}_{1}},{{t}_{1}},x),\quad {{p}_{1}} = {{p}_{{10}}}(x) + {{p}_{{11}}}({{x}_{1}},{{y}_{1}},{{z}_{1}},{{t}_{1}},x), \\ {{w}_{1}} = {{w}_{{11}}}({{x}_{1}},{{y}_{1}},{{z}_{1}},{{t}_{1}},x),\quad {{u}_{1}} = {{u}_{{10}}}(x,{{y}_{1}}) + {{u}_{{11}}}({{x}_{1}},{{y}_{1}},{{z}_{1}},{{t}_{1}},x). \\ \end{gathered} $$
(A2)

For the fluctuation \({{w}_{1}}\), if there are no physical causes of a steady transverse velocity appearing in the flow, then there is no such term. Substituting formulas (A2) into system (A1) shows that the slow functions with indices containing zero are eliminated in the differentiation and do not influence the solution of system (A1). Specifically, system (A1) holds for the fluctuations \({{v}_{{11}}},\;{{p}_{{11}}}\), while the slow functions \({{v}_{{10}}},\;{{p}_{{10}}}\) remain arbitrary.

In this paper, we study a steady solution of the third type, which can be found by considering the next approximations.

The equations for the second approximation can be represented as follows:

$$O\left( {\frac{\delta }{{{{\delta }^{2}}}}} \right)\,:\quad {{\nabla }^{2}}{{p}_{2}} + 2\frac{{\partial {{u}_{0}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{2}}}}{{\partial {{x}_{1}}}} = - 2\frac{{\partial {{u}_{1}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{1}}}}{{\partial {{x}_{1}}}} - 2{{\left( {\frac{{\partial {{v}_{1}}}}{{\partial {{y}_{1}}}}} \right)}^{2}} - 2\frac{{\partial {{v}_{1}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{w}_{1}}}}{{\partial {{z}_{1}}}} - 2{{\left( {\frac{{\partial {{w}_{1}}}}{{\partial {{z}_{1}}}}} \right)}^{2}} - 2\frac{{\partial {{u}_{1}}}}{{\partial {{z}_{1}}}}\frac{{\partial {{w}_{1}}}}{{\partial {{x}_{1}}}} - 2\frac{{\partial {{w}_{1}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{1}}}}{{\partial {{z}_{1}}}},$$
$$\begin{gathered} O\left( {\frac{\delta }{\delta }} \right)\,:\quad \frac{{\partial {{v}_{2}}}}{{\partial {{t}_{1}}}} + {{u}_{0}}\frac{{\partial {{v}_{2}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{p}_{2}}}}{{\partial {{y}_{1}}}} = - {{u}_{1}}\frac{{\partial {{v}_{1}}}}{{\partial {{x}_{1}}}} - {{v}_{1}}\frac{{\partial {{v}_{1}}}}{{\partial {{y}_{1}}}} - {{w}_{1}}\frac{{\partial {{v}_{1}}}}{{\partial {{z}_{1}}}} + \frac{1}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}{{\nabla }^{2}}{{v}_{1}}, \\ O\left( {\frac{\delta }{\delta }} \right)\,:\quad \frac{{\partial {{w}_{2}}}}{{\partial {{t}_{1}}}} + {{u}_{0}}\frac{{\partial {{w}_{2}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{p}_{2}}}}{{\partial {{z}_{1}}}} = - {{u}_{1}}\frac{{\partial {{w}_{1}}}}{{\partial {{x}_{1}}}} - {{v}_{1}}\frac{{\partial {{w}_{1}}}}{{\partial {{y}_{1}}}} - {{w}_{1}}\frac{{\partial {{w}_{1}}}}{{\partial {{z}_{1}}}} + \frac{1}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}{{\nabla }^{2}}{{w}_{1}}, \\ \end{gathered} $$
(A3)
$$O\left( {\frac{\delta }{\delta }} \right)\,:\quad \frac{{\partial {{u}_{2}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{u}_{0}}}}{{\partial x}} + \frac{{\partial {{v}_{2}}}}{{\partial {{y}_{1}}}} + \frac{{\partial {{w}_{2}}}}{{\partial {{z}_{1}}}} = 0.$$

In Eqs. (A3) for second-order perturbations, the linear operators coincide with the operators in (A1), but there appear inhomogeneous quadratic terms. Additionally, viscous terms are retained in (A3) according to the results of [43], where it was shown that the parameter \({{Z}_{i}} = \operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}\) runs over a countable set of eigenvalues ranging from finite values to infinitely large ones. The values of this parameter at finite distances from the laminar–turbulent transition zone can be estimated using the experimental data of [7], namely, \({{Z}_{i}}\sim {{10}^{3}}\). In contrast to the traditional method of multiple scales, derivatives with respect to the slow variable \(x\) do not appear in system (A3), except for the continuity equation. This is explained by the special choice of the original system (2.1)–(2.4) and by the fact that the sought functions are expanded in powers of \({{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}\), while the slow derivatives decrease as powers of \(\delta \). Accordingly, differential equations for the perturbation amplitudes can be obtained only from the third approximation, while system (A3) is used to determine the slow steady secondary perturbation \({{v}_{{10}}}\).

After substituting expressions (A2) into system (A3), the right-hand sides of (A3) can be decomposed into sums of steady and unsteady terms. As a result, the system of equations for the second-order perturbations \({{v}_{2}} = {{v}_{{20}}} + {{v}_{{21}}},\) \({{p}_{2}} = {{p}_{{20}}} + {{p}_{{21}}}\) splits into two subsystems: the steady one (A4a) for \({{v}_{{20}}}({{x}_{1}},{{y}_{1}},x),\) \({{p}_{{20}}}({{y}_{1}},x)\) and the unsteady one (A4b) for \({{v}_{{21}}}({{x}_{1}},{{y}_{1}},{{z}_{1}},{{t}_{1}},x),\) \({{p}_{{21}}}({{x}_{1}},{{y}_{1}},{{z}_{1}},{{t}_{1}},x)\):

$$\begin{gathered} \frac{{{{\partial }^{2}}{{p}_{{20}}}}}{{\partial {{y}_{1}}^{2}}} + 2\frac{{\partial {{u}_{0}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{{20}}}}}{{\partial {{x}_{1}}}} = - 2{{\left( {\frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}}} \right)}^{2}} = G, \\ {{u}_{0}}\frac{{\partial {{v}_{{20}}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{p}_{{20}}}}}{{\partial {{y}_{1}}}} = - {{v}_{{10}}}\frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}} + \frac{1}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}\frac{{{{\partial }^{2}}{{v}_{{10}}}}}{{\partial {{y}_{1}}^{2}}} = E, \\ \end{gathered} $$
(A4a)
$$\begin{gathered} {{\nabla }^{2}}{{p}_{{21}}} + 2\frac{{\partial {{u}_{0}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{{21}}}}}{{\partial {{x}_{1}}}} = - 2\frac{{\partial {{u}_{1}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{{11}}}}}{{\partial {{x}_{1}}}} - 2\left( {2\frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{{11}}}}}{{\partial {{y}_{1}}}} + {{{\left( {\frac{{\partial {{v}_{{11}}}}}{{\partial {{y}_{1}}}}} \right)}}^{2}}} \right) \\ \, - 2\frac{{\partial {{v}_{1}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{w}_{1}}}}{{\partial {{z}_{1}}}} - 2{{\left( {\frac{{\partial {{w}_{1}}}}{{\partial {{z}_{1}}}}} \right)}^{2}} - 2\frac{{\partial {{u}_{1}}}}{{\partial {{z}_{1}}}}\frac{{\partial {{w}_{1}}}}{{\partial {{x}_{1}}}} - 2\frac{{\partial {{w}_{1}}}}{{\partial {{y}_{1}}}}\frac{{\partial {{v}_{1}}}}{{\partial {{z}_{1}}}}, \\ \frac{{\partial {{v}_{{21}}}}}{{\partial {{t}_{1}}}} + {{u}_{0}}\frac{{\partial {{v}_{{21}}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{p}_{{21}}}}}{{\partial {{y}_{1}}}} = - {{u}_{1}}\frac{{\partial {{v}_{{11}}}}}{{\partial {{x}_{1}}}} - ({{v}_{{10}}} + {{v}_{{11}}})\frac{{\partial {{v}_{{11}}}}}{{\partial {{y}_{1}}}} - {{v}_{{11}}}\frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}} - {{w}_{1}}\frac{{\partial {{v}_{1}}}}{{\partial {{z}_{1}}}} + \frac{1}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}{{\nabla }^{2}}{{v}_{{11}}}. \\ \end{gathered} $$
(A4b)

The contribution to the steady inhomogeneous terms is expressed in terms of the velocity \({{v}_{1}}\); therefore, only this variable is decomposed when necessary into components in (A4a), (A4b). First, we need to solve the steady problem (A4a). For this purpose, it is possible to eliminate \({{\partial {{v}_{{20}}}} \mathord{\left/ {\vphantom {{\partial {{v}_{{20}}}} {\partial {{x}_{1}}}}} \right. \kern-0em} {\partial {{x}_{1}}}}\) from the first equation to obtain an ordinary differential equation in \({{y}_{1}}\) for \({{p}_{{20}}}\). As a result, the solution of problem (A4a) for a steady inhomogeneity has the form

$$\begin{gathered} {{v}_{{20}}} = {{x}_{1}}{{V}_{m}}({{y}_{1}}),\quad {{p}_{{20}}} = {{p}_{{20}}}({{y}_{1}}), \\ {{V}_{m}} = \frac{{E - p_{{20}}^{'}}}{{{{u}_{0}}}},\quad p_{{20}}^{'} = - u_{0}^{2}\int\limits_{{{y}_{1}}}^{ + \infty } {\left( {\frac{G}{{u_{0}^{2}}} - \frac{{2u_{0}^{'}}}{{u_{0}^{3}}}E} \right)} d\eta . \\ \end{gathered} $$
(A5)

If the functions G and E, i.e., the steady right-hand sides of the equations are arbitrary, then formulas (A5) imply that the vertical velocity \({{v}_{{20}}}\) has a secular term linearly increasing with growing \({{x}_{1}}\), which leads to the violation of the original asymptotic expansions (2.5). To avoid the spurious growing solu-tions (A5), we set \({{V}_{m}}({{y}_{1}})\) to zero, which yields a necessary condition for the absence of secular terms:

$$\frac{1}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}{v}_{{10}}^{{'''}} - {{{v}}_{{10}}}{v}_{{10}}^{{''}} + {{({v}_{{10}}^{'})}^{2}} = 0,\quad {{{v}}_{{10}}}(0) = 0.$$
(A6)

This condition is a nonlinear ordinary differential equation of the third order for the secondary vertical steady velocity from the first approximation. The only boundary condition to be set for it is the impermeability condition on the wall. A detailed analysis of the unsteady system (A4b) can be conducted only if we know the velocities \({{v}_{{10}}},\,\,{{u}_{0}}\).

The next stage is to analyze the secondary steady streamwise velocity \({{u}_{0}}\) in the boundary layer, which is possible only if the vertical fluid velocity \({{v}_{{10}}}\) is known. From the continuity equation in the first approximation, we can find the fast perturbation of the horizontal velocity \({{u}_{{11}}}\):

$$O\left( {\frac{{{{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{\delta }} \right)\,:\quad \frac{{\partial {{u}_{{11}}}}}{{\partial {{x}_{1}}}} + \frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}} + \frac{{\partial {{v}_{{11}}}}}{{\partial {{y}_{1}}}} + \frac{{\partial {{w}_{{11}}}}}{{\partial {{z}_{1}}}} = 0 \to {{u}_{{11}}} = - \frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}}{{x}_{1}} + {{u}_{{12}}}.$$
(A7)

It contains a steady \({{x}_{1}}\)-linear term, which, in contrast to the vertical velocity, cannot be eliminated explicitly. Therefore, we need to verify the validity of the asymptotic expansion for the streamwise velocity on scales of \({{x}_{1}}\sim {{\delta }^{{{{ - 1} \mathord{\left/ {\vphantom {{ - 1} 2}} \right. \kern-0em} 2}}}}X,\) \(X = O(1)\). Substituting \({{v}_{{10}}}\) from (2.9) into \({{u}_{{11}}}\) and into the asymptotic expansion of the streamwise velocity yields estimates for the terms on the indicated scales:

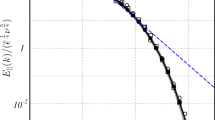
$$\begin{gathered} u = {{u}_{0}}({{y}_{1}}) + {{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}({{u}_{{10}}} + {{u}_{{11}}}) + \ldots ,\quad {{v}_{{10}}} = \frac{{{{V}_{e}}}}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}\left( {1 - \exp ({{V}_{e}}{{y}_{1}})} \right), \\ u = {{u}_{0}} + {{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}({{u}_{{10}}} - \frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}}{{\delta }^{{{{ - 1} \mathord{\left/ {\vphantom {{ - 1} 2}} \right. \kern-0em} 2}}}}X + {{u}_{{12}}}) + \ldots \Rightarrow u = {{u}_{0}} + {{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}\left[ {{{\delta }^{{{{ - 1} \mathord{\left/ {\vphantom {{ - 1} 2}} \right. \kern-0em} 2}}}}X\frac{{{{V}_{e}}^{2}}}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}\exp ({{V}_{e}}{{y}_{1}}) + {{u}_{{10}}} + {{u}_{{12}}}} \right] + \ldots . \\ \end{gathered} $$

If the parameter \({{Z}_{i}} = \operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}\) has a finite value, then the asymptotic expansion for u is obviously violated. However, an analytical study [43] has shown that the parameter \({{Z}_{i}} = \operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}\) can take the value of a countable number of eigenvalues increasing infinitely with growing index i and the asymptotic expansion is valid in the limit. The experimental data from [7] showed that the characteristic value of \({{Z}_{i}}\) is on the order of \({{10}^{3}}\) and grows with increasing coordinate of the considered point on the plate. Moreover, problem (A4a) does not involve a linearly increasing perturbation of the streamwise velocity, so it cannot affect the derivation of Eq. (A6).

To determine the basic profile of the velocity \({{u}_{0}}\), we consider the standard equation for the streamwise momentum in the Navier–Stokes system. It is not given in system (2.1)–(2.4), but has the well-known form

$$\frac{{\partial u}}{{\partial t}} + u\frac{{\partial u}}{{\partial x}} + v\frac{{\partial u}}{{\partial y}} + w\frac{{\partial u}}{{\partial z}} = - \frac{{\partial p}}{{\partial x}} + \frac{1}{{\operatorname{Re} }}{{\nabla }^{2}}u.$$
(A8)

Substituting the asymptotic expansions (2.5) and sequentially extracting the leading terms from the equation, we derive the following equation for first-order perturbations:

$$O\left( {\frac{{{{\delta }^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{\delta }} \right)\,:\quad \frac{{\partial {{u}_{{12}}}}}{{\partial {{t}_{1}}}} + {{u}_{0}}\frac{{\partial {{u}_{{12}}}}}{{\partial {{x}_{1}}}} = {{\left( {{{u}_{0}}\frac{{\partial {{v}_{{10}}}}}{{\partial {{y}_{1}}}} - {{v}_{{10}}}\frac{{\partial {{u}_{0}}}}{{\partial {{y}_{1}}}} + \frac{1}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}\frac{{{{\partial }^{2}}{{u}_{0}}}}{{\partial {{y}_{1}}^{2}}}} \right)}_{{{\text{slow}}}}} - \;\,{{\left( {{{v}_{{11}}}\frac{{\partial {{u}_{0}}}}{{\partial {{y}_{1}}}} + \frac{{\partial {{p}_{{11}}}}}{{\partial {{x}_{1}}}}} \right)}_{{{\text{fast}}}}}.$$
(A9)

As in the equations for vertical velocities, the left-hand side represents an operator depending on the fast variables. The right-hand side contains steady slow and unsteady fast inhomogeneous terms. The perturbation of the streamwise velocity should not contain a steady linear term (since it was extracted in (A7)), which is possible only if the slow right-hand side of (A9) is set to zero. In other words, a kind of consistency condition has to be satisfied for the streamwise momentum equation, since it was differentiated once to derive the pressure equation (2.1). As a result, we obtain an equation for the steady streamwise velocity in TBL, which is viscous as for the vertical velocity:

$$\frac{1}{{\operatorname{Re} {{\delta }^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-0em} 2}}}}}}u_{0}^{{''}} - {{v}_{{10}}}u_{0}^{'} + {v}_{{10}}^{'}{{u}_{0}} = 0,\quad {{u}_{0}}(0) = 0,\quad {{u}_{0}}( + \infty ) = 1.$$
(A10)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gorbushin, A.R., Zametaev, V.B., Lipatov, I.I. et al. Self-Induced Suction of Fluid in a Turbulent Boundary Layer on a Permeable Surface. Comput. Math. and Math. Phys. 62, 1691–1706 (2022). https://doi.org/10.1134/S0965542522100050

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542522100050

Keywords:

Search

Navigation