Skip to main content
SpringerLink
Log in

Numerical Simulation of the Variable Order Fractional Integro-Differential Equation via Chebyshev Polynomials

  • Research Articles
  • Published:
Mathematical Notes Aims and scope Submit manuscript

Abstract

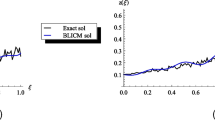
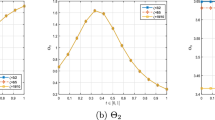
In this paper, the Chebyshev polynomials method is applied to solve a space-time variable fractional order integro-differential equation. Using operational matrices of Chebyshev polynomials furnished from the Caputo–Prabhakar sense and also suitable collocation points, the variable fractional order integro-differential equation would be converted to the system of algebraic equations. The main aim of the Chebyshev polynomials method is to derive four kinds of operational matrices of Chebyshev polynomials. With such operational matrices, an equation is transformed into the products of several dependent matrices, which can also be viewed as the system of linear equations after dispersing the variables. An error bound is proved for the approximate solution obtained by the proposed method. Finally, some numerical examples are presented to demonstrate the accuracy of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.
Fig. 5.
Fig. 6.

Similar content being viewed by others

References

  1. Z. M. Odibat, “A study on the convergence of variational iteration method,” Math. Comput. Modelling 51 (9-10), 1181–1192 (2010).

    Article  MathSciNet  Google Scholar 

  2. I. L. El-Kalla, “Convergence of the Adomian method applied to a class of nonlinear integral equations,” Appl. Math. Lett. 21 (4), 372–376 (2008).

    Article  MathSciNet  Google Scholar 

  3. M. M. Hosseini, “Adomian decomposition method for solution of nonlinear differential algebraic equations,” Appl. Math. Comput. 181 (2), 1737–1744 (2006).

    MathSciNet  MATH  Google Scholar 

  4. S. Momani, Z. Odibat, and V. S. Erturk, “Generalized differential transform method for solving a space and time fractional diffusion wave equation,” Phys. Lett. A 370 (5–6), 379–387 (2007).

    Article  MathSciNet  Google Scholar 

  5. Y. Chen, M. Yi, and C. Yu, “Error analysis for numerical solution of fractional differential equation by Haar wavelets method,” J. Comput. Sci. 3 (5), 367–373 (2012).

    Article  Google Scholar 

  6. Y. Xu and V. Ertürk, “A finite difference technique for solving variable order fractional integro–differential equations,” Bull. Iranian Math. Soc. 40 (3), 699–712 (2014).

    MathSciNet  MATH  Google Scholar 

  7. M. Zayernouri and G. E. Karniadakis, “Fractional spectral collocation methods for linear and nonlinear variable order FPDEs,” J. Comput. Phys. 293, 312–338 (2015).

    Article  MathSciNet  Google Scholar 

  8. A. Mohebbi and M. Saffarian, “Implicit RBF Meshless method for the solution of two-dimensional variable order fractional cable equation,” J. Appl. Comput. Mech. 6 (2), 235–247 (2020).

    Google Scholar 

  9. H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, “Exact solutions for the fractional differential equations by using the first integral method,” Nonlinear Engineering 4 (1), 15–22 (2015).

    Article  Google Scholar 

  10. M. Mashoof and A. R. Sheikhani, “Numerical solution of fractional control system by Haar-wavelet operational matrix method,” Int. J. Industrial Math. 8 (3), 303–312 (2016).

    Google Scholar 

  11. M. Mashoof, A. R. Sheikhani, and H. S. Najafi, “Stability analysis of distributed-order Hilfer–Prabhakar systems based on inertia theory,” Math. Notes 104 (1), 74–85 (2018).

    Article  MathSciNet  Google Scholar 

  12. M. Mashoof, A. R. Sheikhani, and H. S. Naja, “Stability analysis of distributed order Hilfer-Prabhakar differential equations,” Hacet. J. Math. Stat. 47 (2), 299–315 (2018).

    MathSciNet  MATH  Google Scholar 

  13. H. S. Najafi, S. A. Edalatpanah, and A. R. Sheikhani, “Convergence analysis of modified iterative methods to solve linear systems,” Mediterr. J. Math. 11 (3), 1019–1032 (2014).

    Article  MathSciNet  Google Scholar 

  14. F. Shariffar, A. Refahi Sheikhani, and HS. Najafi, “An efficient chebyshev semi-iterative method for the solution of large systems,” Politehn. Univ. Bucharest Sci. Bull. Ser. A Appl. Math. Phys. 80 (4), 239–252 (2018).

    MathSciNet  MATH  Google Scholar 

  15. R. Garra, R. Gorenflo, F. Polito, and Ž. Tomovski, “Hilfer–Prabhakar derivatives and some applications,” Appl. Math. Comput. 242, 576–589 (2014).

    MathSciNet  MATH  Google Scholar 

  16. T. R. Prabhakar, “A singular integral equation with a generalized Mittag Leffler function in the kernel,” Yokohama Math. J. 19, 7–15 (1971).

    MathSciNet  MATH  Google Scholar 

  17. M. Ichise, Y. Nagayanagi, and T. Kojima, “An analog simulation of non-integer order transfer functions for analysis of electrode processes,” J. Electroanal. Chem. and Interfacial Electrochem. 33 (2), 253–265 (1971).

    Article  Google Scholar 

  18. S. Khubalkar, A. Junghare, M. Aware, and S. Das, “Unique fractional calculus engineering laboratory for learning and research,” Int. J. Electrical Engineering Education (2018).

    Google Scholar 

  19. J. Lai, S. Mao, J. Qiu, H. Fan, Q. Zhang, Z. Hu, and J. Chen, “Investigation progress and applications of fractional derivative model in geotechnical engineering,” Math. Problems in Eng. 2016, Art. ID 9183296 (2016).

    Google Scholar 

  20. M. D. Ortigueira, Fractional Calculus for Scientists and Engineers, in Lect. Notes Electr. Eng. (Springer, Dordrecht, 2011), Vol. 84.

    Book  Google Scholar 

  21. F. Shariffar and R. Sheikhani, “A new two-stage iterative method for linear systems and its application in solving Poissons equation,” Int. J. Industrial Math. 11 (4), 283–291 (2019).

    Google Scholar 

  22. A. H. R. Sheikhani and M. Mashoof, “A Collocation Method for Solving Fractional Order Linear System,” J. Indones. Math. Soc. 23 (1), 27–42 (2017).

    Article  MathSciNet  Google Scholar 

  23. A. A. A. Kilbas, H. M. Srivastava, and J. J. Trujillo, Theory and Applications of Fractional Differential Equations, in North-Holland Math. Stud. (Elsevier Sci., Amsterdam, 2006), Vol. 204.

    Book  Google Scholar 

  24. R. Garra and R. Garrappa, “The Prabhakar or three parameters Mittag-Leffler function: theory and application,” Commun. Nonlinear Sci. Numer. Simul. 56, 314–329 (2018).

    Article  MathSciNet  Google Scholar 

  25. R. Garrappa, “Grünwald–Letnikov operators for fractional relaxation in Havri\=liak-Negamimodels,” Commun. Nonlinear Sci. Numer. Simul. 38, 178–191 (2016).

    Article  MathSciNet  Google Scholar 

  26. A. Giusti, I. Colombaro, R. Garra, R. Garrappa, F. Polito, M. Popolizio, and F. Mainardi, “A practical guide to Prabhakar fractional calculus,” Fract. Calc. Appl. Anal. 23 (1), 9–54 (2020).

    Article  MathSciNet  Google Scholar 

  27. F. Mainardi and R. Garrappa, “On complete monotonicity of the Prabhakar function and non-Debye relaxation in dielectrics,” J. Comput. Phys. 293, 70–80 (2015).

    Article  MathSciNet  Google Scholar 

  28. S. C. Pandey, “The Lorenzo–Hartley’s function for fractional calculus and its applications pertaining to fractional order modelling of anomalous relaxation in dielectrics,” Comput. Appl. Math. 37 (3), 2648–2666 (2018).

    Article  MathSciNet  Google Scholar 

  29. R. K. Gupta, B. S. Shaktawat, and D. Kumar, “Certain relation of generalized fractional calculus associated with the generalized Mittag-Leffler function,” J. Rajasthan Acad. Phys. Sci. 15 (3), 117–126 (2016).

    MathSciNet  MATH  Google Scholar 

  30. A. Giusti and I. Colombaro, “Prabhakar-like fractional viscoelasticity,” Commun. Nonlinear Sci. Numer. Simul. 56, 138–143 (2018).

    Article  MathSciNet  Google Scholar 

  31. R. Agarwal, S. O. N. A. L. Jain, and R. P. Agarwal, “Analytic solution of generalized space-time fractional reaction-diffusion equation,” Fract. Differ. Calc. 7, 169–84 (2017).

    Article  MathSciNet  Google Scholar 

  32. E. H. Doha, M. A. Abdelkawy, A. Z. M. Amin, and A. M. Lopes, “On spectral methods for solving variable-order fractional integro-differential equations,” Comput. Appl. Math. 37 (3), 3937–3950 (2018).

    Article  MathSciNet  Google Scholar 

  33. M. A. Snyder, Chebyshev Methods in Numerical Approximation (Prentice-Hall, Englewood Cliffs, NJ, 1966).

    MATH  Google Scholar 

  34. K. Sun and M. Zhu, “Numerical algorithm to solve a class of variable order fractional integral-differential equation based on Chebyshev polynomials,” Math. Probl. Eng. 2015, Art. ID 902161 (2015).

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Lahijan Branch, Islamic Azad University, Lahijan, 1616, Iran

    B. Bagherzadeh Tavasani & A. H. Refahi Sheikhani

  2. University of Guilan, Rasht, 41938, Iran

    H. Aminikhah

Authors
  1. B. Bagherzadeh Tavasani
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. H. Refahi Sheikhani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. H. Aminikhah
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Translated from Matematicheskie Zametki, 2022, Vol. 111, pp. 676–691 https://doi.org/10.4213/mzm13511.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bagherzadeh Tavasani, B., Refahi Sheikhani, A.H. & Aminikhah, H. Numerical Simulation of the Variable Order Fractional Integro-Differential Equation via Chebyshev Polynomials. Math Notes 111, 688–700 (2022). https://doi.org/10.1134/S0001434622050030

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0001434622050030

Keywords

Search

Navigation