Influences of elastic foundation on bending analysis of multidirectional porous functionally graded plate under industrial used loading: a meshfree approach

Abstract

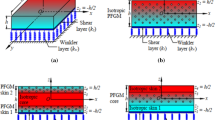
In the present work, the bending analysis of a multi-directional porous functionally graded plate (MDPFG) resting on an elastic foundation involves determining the deflection and stress distribution of the plate under I, T and L loads. The material properties of MDPFG plates are to be graded in the thickness and length directions according to modified power-law distribution considering uniformly and nonuniformly types of porosity distributions. The energy principle produces the plate’s governing differential equations (GDEs). The strong form formulation is discretized using Wendland radial basis function (WRBF) is used to solve GDEs and determine the deflection and stress distribution of the plate under applied loading. To generate the numerical results, a code has been computed in MATLAB (2019). The normalized deflection and stresses of MDPFG plates are investigated with respect to the grading index, span-to-thickness ratio, aspect ratio, transverse loading type, porosity fraction, and porosity distribution. Future investigations of MDPFG plates may use new numerical results as standards.

Appendix-I

The first part of GDEs (13) $\delta u_{0}:\frac{{\partial N_{xx} }}{\partial x} + \frac{{\partial N_{xy} }}{\partial y} = 0$ are discretized using WRBF and are expressed as

$$[K^{l}_{1u} ]_{(NI,N)} = \sum\limits_{i = 1}^{NI} {\sum\limits_{j = 1}^{N} {\left( \begin{gathered} A_{11} \frac{{\partial^{2} g_{(i,j)} }}{{\partial x^{2} }} + A_{66} \frac{{\partial^{2} g_{(i,j)} }}{{\partial y^{2} }} \hfill \\ + 2A_{16} \frac{{\partial^{2} g_{(i,j)} }}{\partial x\partial y} \hfill \\ \end{gathered} \right)} }$$

(30)

$$[K^{l}_{1v} ]_{(NI,N)} = \sum\limits_{i = 1}^{NI} {\sum\limits_{j = 1}^{N} {\left( \begin{gathered} A_{12} \frac{{\partial^{2} g_{(i,j)} }}{\partial x\partial y} + A_{16} \frac{{\partial^{2} g_{(i,j)} }}{{\partial x^{2} }} \hfill \\ + A_{66} \frac{{\partial^{2} g_{(i,j)} }}{\partial x\partial y} + A_{26} \frac{{\partial^{2} g_{(i,j)} }}{{\partial y^{2} }} \hfill \\ \end{gathered} \right)} }$$

(31)

$$[K^{l}_{1w} ]_{(NI,N)} \, = \sum\limits_{i = 1}^{NI} {\sum\limits_{j = 1}^{N} {\left( \begin{gathered} - B_{11} \frac{{\partial^{3} g_{(i,j)} }}{{\partial x^{3} }} - B_{12} \frac{{\partial^{3} g_{(i,j)} }}{{\partial x\partial y^{2} }} \hfill \\ - 3B_{16} \frac{{\partial^{3} g_{(i,j)} }}{{\partial x^{2} \partial y}} - 2B_{66} \frac{{\partial^{3} g_{(i,j)} }}{{\partial x\partial y^{2} }} \hfill \\ - B_{26} \frac{{\partial^{3} g_{(i,j)} }}{{\partial y^{3} }} \hfill \\ \end{gathered} \right)} }$$

(32)

$$[K^{l}_{{1\phi_{x} }} ]_{(NI,N)} = \sum\limits_{i = 1}^{NI} {\sum\limits_{j = 1}^{N} {\left( \begin{gathered} E_{11} \frac{{\partial^{2} g_{(i,j)} }}{{\partial x^{2} }} + 2E_{16} \frac{{\partial^{2} g_{(i,j)} }}{\partial x\partial y} \hfill \\ + E_{66} \frac{{\partial^{2} g_{(i,j)} }}{{\partial y^{2} }} \hfill \\ \end{gathered} \right)} }$$

(33)

$$[K^{l}_{{1\phi_{y} }} ]_{(NI,N)} \, = \sum\limits_{i = 1}^{NI} {\sum\limits_{j = 1}^{N} {\left( \begin{gathered} E_{12} \frac{{\partial^{2} g_{(i,j)} }}{\partial x\partial y} + E_{16} \frac{{\partial^{2} g_{(i,j)} }}{{\partial x^{2} }} \hfill \\ + E_{26} \frac{{\partial^{2} g_{(i,j)} }}{{\partial y^{2} }} + E_{66} \frac{{\partial^{2} g_{(i,j)} }}{\partial x\partial y} \hfill \\ \end{gathered} \right)} }$$

(34)

Similarly, other parts of GDEs are discretized.

The boundary conditions can be discretized in similar fashion. For, e.g., simply supported boundary condition at the edge x = 0 (Eq. 22) is discretized and finally expressed as

$$[K]_{b,x = 0} \left\{ \delta \right\} = \left\{ 0 \right\}$$

(35)

$$\left[ K \right]_{b,x = 0} = \left[ {\begin{array}{*{20}c} {[K^{lb}_{1u} ]} & {[K^{lb}_{1v} ]} & {[K^{lb}_{1w} ]} & {[K^{lb}_{{1\phi_{x} }} ]} & {[K^{lb}_{{1\phi_{y} }} ]} \\ {[0]_{{nbx_{0} \times N}} } & {[K^{{{\text{l}} b}}_{2v} ]} & {[0]_{{nbx_{0} \times N}} } & {[0]_{{nbx_{0} \times N}} } & {[0]_{{nbx_{0} \times N}} } \\ {[0]_{{nbx_{0} \times N}} } & {[K^{{{\text{l}} b}}_{2v} ]} & {[K^{lb}_{3w} ]} & {[0]_{{nbx_{0} \times N}} } & {[0]_{{nbx_{0} \times N}} } \\ {[K^{lb}_{4u} ]} & {[K^{lb}_{4v} ]} & {[K^{lb}_{4w} ]} & {[K^{lb}_{{4\phi_{x} }} ]} & {[K^{lb}_{{4\phi_{y} }} ]} \\ {[0]_{{nbx_{0} \times N}} } & {[K^{{{\text{l}} b}}_{2v} ]} & {[0]_{{nbx_{0} \times N}} } & {[0]_{{nbx_{0} \times N}} } & {[K^{lb}_{{5\phi_{y} }} ]} \\ \end{array} } \right]$$

(36)

nbx₀ = number of nodes on the boundary x = 0.

$$[K^{lb}_{1u} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {A_{11} \frac{{\partial g_{(i,j)} }}{\partial x} + A_{16} \frac{{\partial g_{(i,j)} }}{\partial y}} \right)} }$$

(37)

$$[K^{lb}_{1v} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {A_{12} \frac{{\partial g_{(i,j)} }}{\partial x} + A_{16} \frac{{\partial g_{(i,j)} }}{\partial y}} \right)} }$$

(38)

$$[K^{lb}_{{1\phi_{x} }} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( \begin{gathered} - B_{11} \frac{{\partial^{2} g_{(i,j)} }}{{\partial x^{2} }} - B_{12} \frac{{\partial^{2} g_{(i,j)} }}{{\partial y^{2} }} \hfill \\ - 2B_{16} \frac{{\partial^{2} g_{(i,j)} }}{\partial y\partial x} \hfill \\ \end{gathered} \right)} }$$

(39)

$$[K^{l}_{{1\phi_{x} }} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {E_{12} \frac{{\partial g_{(i,j)} }}{\partial y} + E_{16} \frac{{\partial g_{(i,j)} }}{\partial x}} \right)} }$$

(40)

$$\,[K^{lb}_{{1\phi_{y} }} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {E_{11} \frac{{\partial g_{(i,j)} }}{\partial x} + E_{16} \frac{{\partial g_{(i,j)} }}{\partial y}} \right)} }$$

(41)

$$[K^{lb}_{2v} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {g_{(i,j)} } }$$

(42)

$$[K^{lb}_{3w} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {g_{(i,j)} } }$$

(43)

$$[K^{lb}_{4u} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {B_{11} \frac{{\partial g_{(i,j)} }}{\partial x} + B_{16} \frac{{\partial g_{(i,j)} }}{\partial y}} \right)} }$$

(44)

$$[K^{lb}_{4v} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {B_{12} \frac{{\partial g_{(i,j)} }}{\partial x} + B_{16} \frac{{\partial g_{(i,j)} }}{\partial y}} \right)} }$$

(45)

$$[K^{lb}_{4w} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( \begin{gathered} - D_{11} \frac{{\partial^{2} g_{(i,j)} }}{{\partial x^{2} }} - D_{12} \frac{{\partial^{2} g_{(i,j)} }}{{\partial y^{2} }} \hfill \\ - 2D_{16} \frac{{\partial^{2} g_{(i,j)} }}{\partial y\partial x} \hfill \\ \end{gathered} \right)} }$$

(46)

$$[K^{l}_{{4\phi_{x} }} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {F_{12} \frac{{\partial g_{(i,j)} }}{\partial y} + F_{16} \frac{{\partial g_{(i,j)} }}{\partial x}} \right)} }$$

(47)

$$[K^{lb}_{{4\phi_{y} }} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {\left( {F_{11} \frac{{\partial g_{(i,j)} }}{\partial x} + F_{16} \frac{{\partial g_{(i,j)} }}{\partial y}} \right)} }$$

(48)

$$[K^{lb}_{{5\phi_{y} }} ] = \sum\limits_{i = NI + 1}^{{NI + 1 + nbx_{0} }} {\sum\limits_{j = 1}^{N} {g_{(i,j)} } }$$

(49)

Similarly, other boundary conditions at the edges x = a, y = 0 and y = b are discretized. The resulting equation is written as matrix form as

$$[K]_{B} \left\{ \delta \right\} = \left\{ 0 \right\}$$

(50)

where

$$\left[ K \right]_{B} = \left[ {\left[ {\begin{array}{*{20}c} {[K]_{b,y = 0} } & {[K]_{b,x = a} } & {[K]_{b,y = b} } & {[K]_{b,x = 0} } \\ \end{array} } \right]_{{(5 \times N_{B} ,5 \times N)}} } \right]^{T}$$

(51)