Dust and powder in fusion plasmas: recent developments in theory, modeling, and experiments

Abstract

In this paper, we present a brief historic overview of the research on dust in fusion devices with carbon plasma-facing components and then highlight the most recent developments in the post-carbon era of the field. In particular, we consider how the metallic dust form, mobilize, and interact with fusion plasmas and plasma facing components. Achievements in wall conditioning and associated anomalous plasma transport modification, including ELM suppression, with the powder injection technique is another focus of the paper. Capabilities of the state-of-art simulation tools to describe different aspects of dust in fusion devices are exemplified and new directions for future dust studies are brought forward.

1 Introduction

The documented history of the study of dust-related phenomena in magnetic fusion devices has started about 45 years ago with the paper by Ohkawa (1977). In Ohkawa (1977), the author has assessed the role of dust in core plasma pollution with impurities originated as a result of the vaporization of some “particulates that might have been produces by blistering or abrasion of wall in previous discharges”. The results found in Ohkawa (1977) did not contradict to available, at that time, rather sparse data on impurity fraction in core plasma and it was concluded that “It is important to test the above hypothesis by further experimental observations”. Nearly four decades later, there is still no consensus on the role of dust in the contamination of plasma with impurity in magnetic fusion devices.

Soon after Ohkawa’s paper, “high speed cine photography” was introduced in many magnetic fusion devices to study different processes ranging from plasma interactions with material surfaces surrounding plasma to the “UFO” observations (“UFO” being the “nick-name” for glowing dust particles heated up by plasma). Inspection of the plasma facing components (PFCs) during ventilation events also revealed the presence of a large amount of dust particles of different sizes, shapes, and composition. This marked the beginning of systematic investigation of dust in magnetic fusion devices; in the TEXTOR tokamak (Winter 1998) followed by studies from the JET (Peacock et al. 1999), TFTR, DIII-D, Alcator C-Mod (Carmack et al. 2000), Tore-Supra (Chappuis et al. 2001), and ASDEX-Upgrade (Sharpe 2001; Rohde et al. 2009) tokamaks. The results of such "postmortem" analysis of dust showed a whole "zoo" of dust particles with sizes ranging from sub-mm to 100’s of nm, see Fig. 1. Survey of the postmortem analysis of dust particle collected in different magnetic fusion devices can be found in the review papers (Federici et al. 2001; Rubel et al. 2001; Sharpe et al. 2002; Winter 2004; Krasheninnikov et al. 2011), and the Chapter 5 of the book (Krasheninnikov et al. 2020).

Low sensitivity of the “high speed cine photography” and limitations of postmortem analysis to yield information on the dust number density, size distribution, and dynamics in plasma volume called for development of other experimental techniques. It turned out that the signal from the Rayleigh channel of the lasers, typically used in tokamaks for Thomson scattering measurements of plasma density and electron temperature, is a very useful tool for in-situ measurements of both dust particle density and size distribution. First in-situ detection of dust particles with the laser was reported for JIPPT-IIU tokamak (Narihara et al. 1997). This was followed by rather detailed studies of dust density and size distribution in both mid-plane and divertor regions of the DIII-D tokamak (West et al. 2006; West and Bray 2007), see Fig. 2. Later on the laser diagnostic was employed for dust studies in other magnetic fusion devices (Krasheninnikov et al. 2020).

Analysis of data of laser dust detection in DIII-D tokamak with the Mie scattering theory, accounting for the dust grain ablation under intense laser radiation, has shown size distribution close to the power-law, with the exponent $\alpha \approx$ 2.6–2.7 (Smirnov et al. 2007a). Interestingly, similar power-law distribution with $\alpha \approx 2.5$ was found in the LHD stellarator (Koga et al. 2009) as a result of the analysis of dust particles in the range $10 \div {10}^4$ nm collected from the PFCs.

Fig. 1

Reproduced with the permission from Winter (1998), $\copyright$ IOP Publishing

Dust particles collected in TEXTOR tokamak (Winter 1998).

Fig. 2

Reprinted from West and Bray (2007), with the permission from Elsevier

Dust particle density in a low divertor of the DIII-D tokamak.

In-situ dust observations with fast framing CCD cameras, which in the beginning of twenty-first century started to be widely used for different applications in fusion devices, played a pivotal role in the development of both theoretical models and numerical codes focused on deeper understanding of the role of dust in fusion plasmas. In particular, tracking of dust with multiple cameras facilitated reconstruction of 3D dust trajectories (Roquemore et al. 2007), which were used for the benchmarking of dust transport codes. Some trajectories appear to be very peculiar (e.g. see Fig. 3), indicating a complex grain shapes and composition affecting dust dynamics. In addition, fast cameras were used for study of dust creation events, see e.g. Hong et al. (2010). Detailed analysis of imaging diagnostics with fast cameras (Smirnov et al. 2009) has shown that the sensitivity of the cameras does not allow to detect dust grains with the size below $\sim 1$ $\upmu$m. The description of other diagnostics used for dust studies in fusion plasmas, such as electrostatic and microbalance detectors, dust capturing with aerogel, and others can be found in the reviews (Ratynskaia et al. 2008; Rudakov et al. 2008; Krasheninnikov et al. 2011) and the references therein.

Fig. 3

Reproduced with the permission from Rudakov et al. (2009), $\copyright$ IAEA 2009

Spiral trajectory of an object recorded in the DIII-D tokamak.

Analysis of available experimental data and the need for assessment of dust impact in both contemporary and future devices has prompted progress of theoretical understanding of dust–plasma interaction in fusion plasmas. Although the initial modelling development has greatly benefited from a significant body of work in the field of dusty- or complex-plasmas (e.g. see Tsytovich 1997; Shukla and Mamun 2002; Fortov et al. 2005; Fortov and Morfill 2009), dust–plasma interaction in fusion devices is characterized by several distinct processes which had to be yet addressed. Dusty-plasma experiments deal with low-temperature weakly ionized plasmas seeded with well-defined population of spherical dust particles, typically composed of a single material. In such plasmas the heat flux to the particles is insignificant, thus three is essentially no ablation of the dust. Moreover, dust particle number density is usually high so that a significant fraction of the total charge is carried by the dust, altering the overall quasineutrality condition. Finally, with exception of recent laboratory set-ups (Melzer et al. 2021), the magnetic field in typical dusty-plasma experiments, if any, is weak.

The situation in fusion experiments is very different. (i) Fusion plasma is highly magnetized, the Larmor radii of plasma particles, electrons in particular, could be significantly smaller than the size of dust grains. (ii) Dust shapes can be far from spherical (see Fig. 1). (iii) Hot and dense plasma causes strong evaporation and ablation of dust particles. (iv) The clouds of ablated material can significantly alter plasma–grain interactions by “shielding” the dust particle and mitigating the incoming heat flux. Such shielding effects are of particular importance for relatively large grain size (Krasheninnikov and Smirnov 2009; Brown et al. 2014) [similar effects are observed in the course of the injection of large pellets in fusion plasmas for both fueling and discharge termination (Parks et al. 1977; Rozhansky and Senichenkov 2005)] and for the case where a large amount of powder particles is injected into fusion plasma for both an active impact on edge plasma parameters and in-situ conditioning of the first wall (Mansfield et al. 2010; Osborne et al. 2015; Lunsford et al. 2019, 2021; Sun et al. 2019; Bortolon et al. 2019a, 2020; Maingi et al. 2020; Gilson et al. 2021). (v) One of the main dust sources in fusion devices with carbon-based PFC is delamination of the co-deposited layers formed on PFCs due to erosion processes (Federici et al. 2001; Rubel et al. 2001; Sharpe et al. 2002; Winter 2004; Krasheninnikov et al. 2011). These layers consist of materials with different physical properties, which can cause inhomogeneity of evaporation rate over the particle surface and result in substantial “rocket” force exerted on the grain. Although a rather general theory of the interactions of a complex shape dust particles with plasma was developed (Krasheninnikov et al. 2020), it is difficult to use it in practice because both shape and composition of intrinsic dust in fusion devices are not known a priori. Nonetheless, an indirect evidence of an impact of such effects on dust dynamics was deduced from dust imaging with fast cameras. Examples include observations of rapid change of flight direction and jitter-like deviations from an average trajectory direction (Smirnov et al. 2011) as well as a spiral dust trajectory (Rudakov et al. 2009) (shown in Fig. 3), which could be attributed to the motion of a flake-like dust particle and/or “rocket-force” effect. It is worth noting that dynamics of large objects, sometimes classified as ’debris’, such as depicted in Fig. 3, might significantly differ from that of smaller (100’s $\upmu$m and below) dust particles.

With the ITER coming to the scene, tritium uptake by the first wall material has forced scientists and engineers to move from the carbon-based to full-metal wall devices (Girard et al. 2008; Roth et al. 2009). In ITER, tungsten (W) will be used for divertor tiles, whereas beryllium (Be) for the main chamber walls. The need to gain experience of operation with metal PFCs has prompted upgrading and building machines with full metal vessel or with metal-coated PFCs. While overall experience demonstrated a drastic reduction of dust production (see Sect. 2.1), recalling that even a small fraction ($\sim 10^{-4}$) of W in the core of fusion reactor could be “deadly” for the reactor performance (Pütterich et al. 2019), it raises concern about an impact of dust on the reactor grade plasma parameters and possibility to trigger disruptions of discharges. In the reactor-scale devices, such as ITER, disruptions could cause a significant damage of the PFCs. Moreover, as a licensed nuclear facility, ITER must meet limits imposed on the in-vessel dust inventory, with specified maximum amount of mobilizable dust in the vessel as well as quantities of dust lying on hot PFC surfaces (Rosanvallon et al. 2009; Shimada et al. 2013). Possible impact of dust on in-vessel diagnostics systems is also a matter of concern (Costley et al. 2005). Hence, reliable assessment of the formation, mobilization and transport of metallic dust in fusion plasmas is crucial for future reactors.

There are also cases, which attracted attention recently, where dust can play a positive role in magnetic fusion devices. One of such examples is related to establishing of so-called divertor plasma detachment, which is considered to be a primary operation regime in ITER, see e.g. Krasheninnikov and Kukushkin (2017) and the references therein. In early fusion devices with carbon-based first wall armor, natural carbon erosion provided enough carbon impurity to cool down plasma in front of divertor targets insuring the transition into detachment. However, full metal PFCs choice resulted in the reduction of both hydrogen retention and impurity/dust production so that high auxiliary plasma heating divertor plasma detachment only could be obtained by carefully controlled impurity injection (Telesca et al. 2017). Therefore, one can envisage use of dust particles as an impurity carrier, which can penetrate deeper into plasma and cause stronger plasma cooling in comparison with the same amount of impurity injected in the form of a gas.

Another frontier application is dust/powder injection into magnetic fusion devices for in-situ wall conditioning (Mansfield et al. 2010; Osborne et al. 2015; Lunsford et al. 2019; Sun et al. 2019; Bortolon et al. 2019a, 2020; Maingi et al. 2020; Lunsford et al. 2021; Gilson et al. 2021; Bodner et al. 2022). Moreover, it appears that such injection suppresses violent bursts of Edge Localized Modes (ELMs), which are considered to be particularly dangerous for PFCs in future tokamak reactors. The mechanism of ELM suppression with powder injection is not yet understood and is a topic of ongoing research (Maingi et al. 2020). It is plausible that in future reactors the powder injection also could be used for in-situ deposition of a sacrificial layer on the main chamber walls to avoid unacceptable erosion of the wall material by both plasma and charge-exchange neutrals.

We note that many theoretical and experimental aspects of dust/powder particle interactions with plasma in fusion devices were discussed in review papers (Ratynskaia et al. 2008; Rudakov et al. 2008; Krasheninnikov et al. 2011) and the book (Krasheninnikov et al. 2020). Therefore, this paper is focused only on recent developments in the field of dust interactions with fusion plasmas and dust applications in future reactors. In particular, in Sect. 2 we consider the peculiarities of metallic dust formation, mobilization, and interactions with plasma and vessel. Experimental results on wall conditioning and ELM suppression via dust/powder injection are highlighted in Sect. 3. Discussion of available tools for numerical modeling of dust in fusion plasmas and selected applications for predictive modeling is provided in Sect. 4. Finally, Sect. 5 summarizes current status of the studies of dust in fusion plasmas and outlines potential directions for future investigations.

2 Metallic dust formation, mobilization, and interactions with plasmas and vessel wall

To address issues of practical concern in full-metal fusion reactors (dust inventory evolution, in-vessel accumulation sites, and impurity production), the sequence of all involved physical processes should be considered, as outlined in Fig. 4. Only some of these processes concern dust–plasma interaction, while others involve fluid dynamics and contact mechanics (Ratynskaia et al. 2022). The dust formation and release mechanisms have a direct impact on dust dynamics by dictating both the initial conditions and the regimes of plasma particle collection by the dust. In particular, dust and droplets in metal machines feature large sizes implying inhibition of electron collection due to the presence of strong magnetic fields and, owing to significant release speeds, an inertia-dominated motion.

Fig. 4

Fluid dynamics and contact mechanics problems are highlighted in red and blue, respectively. Reproduced from Ratynskaia et al. (2022) with the permission of IOP Publishing

Processes involved in dust inventory evolution, in-vessel accumulation sites and impurity production in fusion devices.

2.1 Formation

The collection of particles and loosely adhered matter from PFC surfaces in full metal machines or partly metal machines with metal-coated CFC PFCs reveal a rather consistent picture (Fortuna-Zaleśna et al. 2017a, b, 2021; Rubel et al. 2018; Flanagan et al. 2015; Baron-Wiechec et al. 2015; Balden et al. 2014; Arnas et al. 2017; Peillon et al. 2020; De Angeli et al. 2015a, b, 2021a, b); (i) much less dust is produced compared to machines with carbon PFCs (ii) the collected particulate shapes can be mainly categorized as spheroids or flakes (iii) the presence of ferromagnetic or strongly paramagnetic dust.

The suppression of dust production in the ASDEX-Upgrade (AUG) tokamak after the transition from the C first wall to the W first wall was reported in Ref. Balden et al. (2014) and was confirmed by video analysis of dust events recorded in the full W AUG over seven campaigns (Brochard et al. 2017). Quantitative estimates from dedicated JET studies yield a two orders of magnitude reduction between JET-C and JET-ILW over about 20 h of plasma operation (Rubel et al. 2018). The flakes, as also found previously, originate from the delamination of co-deposited layers of eroded materials. The spherical particles signify a novel mechanism of dust formation in metal machines—droplet ejection from unstable liquid metal pools. The appreciable size variations of observed splashes and spherical solid particles, which are classified as re-solidified droplets, are related to two factors; the characteristics of the normal or off-normal event that led to the formation and splashing of the liquid pool as well as the geometric correlation between the original ejection and collection sites. Melting of metallic PFCs occurs under intense heat loads which can be realized in a number of scenarios, the most typical ones include energetic transient events such as edge-localized modes (ELMs), vertical displacement events (VDEs) or major disruptions (MDs), unipolar arcing and interaction with runaway electrons (REs). In a number of investigations, it was possible to trace the origin of the observed droplets and splashes back to one of such energetic events. A few representative examples include the MD-induced melting of edges of divertor tiles in Alcator C-mod (Arnas et al. 2017) and of divertor monoblocks in EAST (Lei et al. 2021); the VDE-induced melting of upper damp plates (UDPs) in JET Jepu et al. (2019); the RE-induced melting of antenna protector limiter in WEST (Peillon et al. 2020), of UDPs in JET (Fortuna-Zaleśna et al. 2017a; Jepu et al. 2019) and of poloidal limiter in FTU (De Angeli et al. 2015a); arcing in AUG (Balden et al. 2014; Rohde et al. 2021), FTU (De Angeli et al. 2015a) and WEST (Peillon et al. 2020). The morphology of particles collected in contemporary devices is illustrated in Fig. 5, the spherical shape and large size are to be contrasted to the dust in carbon-era machines discussed in the Introduction and depicted in Fig. 1

Fig. 5

Reproduced from Fazinić et al. (2020) with the permission of IAEA Publishing. Reproduced from Arnas et al. (2017), $\copyright$2017 The Authors of Arnas et al. (2017). Reproduced from De Angeli et al. (2021b), $\copyright$2021 The Authors of De Angeli et al. (2021b)

Particle morphology in contemporary fusion devices. Upper raw: W-rich agglomerate composed of flakes detached from the W-coated divertor tiles and a Be droplet collected in JET ILW (Fazinić et al. 2020). Middle raw: Mo, B, W particles collected in Alcator C-Mod (Arnas et al. 2017). Lower raw: SS droplet collected in FTU and SS-based particle collected in COMPASS (De Angeli et al. 2021b). Compare to carbon dust in Fig. 1.

.

Depending on the scenario and location of the collection/observation site, the measured size and overall mass might not necessary correspond to those of the original droplets. For example, the time scale for the development of a large enough oscillation amplitude to induce material ejection from the edge of the molten JET UDP (Vignitchouk et al. 2022b) is comparable to duration of a typical VDE (responsible for the melting) (Jepu et al. 2019; Ratynskaia et al. 2020), so that droplets are ejected essentially into a vacuum and do not lose mass due to interaction with the plasma prior to impacting on the wall. Yet, in the case that droplets encounter dense and/or hot plasma during their trajectory towards the wall, the final solid population might not be a faithful representation of the original droplet distribution. A quantitative example can be found in Ref. Vignitchouk et al. (2018c) where simulated Be droplets, that were generated during the thermal quench, interact with the disrupting ITER plasma. A very low conversion rate was found [Table 3 of Ref. Vignitchouk et al. (2018c)]; the mass of solid dust never exceeded 5$\%$ of the initial mass in the MD scenario, and 2$\%$ in the VDE scenario. This is due to the fact that solid dust production is most efficient for droplets with low speeds and a certain intermediate size, since they must survive their passage through the plasma but must also resolidify prior to their first encounter with the wall.

The analysis of dust particles collected in JET-ILW (Fortuna-Zaleśna et al. 2017a, b, 2021; Rubel et al. 2018; Flanagan et al. 2015; Baron-Wiechec et al. 2015; Fazinić et al. 2020; Ashikawa et al. 2020) reveals that they consist mainly of beryllium and tungsten with the contributions of the Inconel components from the vacuum vessel wall (such as Ni, Cr, Fe) and carbon from tungsten-coated tiles. Formation of W-rich spherical particles has been attributed to agglomeration of flakes originating from W-coated CFC tiles. Some small fractions of Cu, Mo, B, Mg, Al, Si, and O were also found. In addition, nitrogen (from molecular nitrogen puffing used in the studies of divertor detachment physics) and deuterium (in particular in C-rich dust particles) were present. The tungsten dust particles with the Inconel components (Ni, Cr, Fe) were also found in the WEST tokamak Peillon et al. (2020). The majority of dust collected the AUG tokamak was represented by W-dominated spheroids consisting of a solid W core, identified as resolified droplets, as well as W-, C-, and B-dominated irregularly shaped particles, presumably produced by flaking of deposited films (Rohde et al. 2009; Balden et al. 2014).

The presence of a significant magnetic component in the dust inventory is another peculiarity of metal devices. Ferromagnetic and strongly paramagnetic dust has been collected in several devices that feature different PFCs and operating magnetic fields; Textor (15 wt$\%$) (Winter 1998), Globus-M (10 wt$\%$) (Novokhatsky et al. 2011), FTU (30 wt$\%$) (De Angeli et al. 2015a), Alcator C-Mod (27 wt$\%$) (De Angeli et al. 2021a), COMPASS (18 wt$\%$) (De Angeli et al. 2021a) and DIII-D (De Angeli et al. 2021a). Dedicated cross-machine investigations (De Angeli et al. 2021a, b) concluded that the main mechanism of in-situ ferromagnetic dust formation is the change of iron crystalline phase from austenitic to ferritic during the re-solidification of stainless steel droplets. The significance of this magnetic dust population is elucidated in Sect. 2.2.2.

2.2 Mobilization

In the light of above, it is necessary to differentiate between the release of freshly generated dust or droplets and the remobilization of dust produced in previous discharges. In the former case, particle speeds and sizes naturally depend on the specific production mechanism. In the latter case, particle speeds and sizes are determined by nature of the dominant mobilizing force (plasma induced, vessel induced, airflow), heat exchange with the plasma and contact mechanics aspects. Below we briefly summarize both.

It it important to note that, with the exception of dedicated dust injection or remobilization experiments where cameras can observe the specific dust population introduced to the machine, the monitoring of dust events in a significant plasma volume does not allow an unambiguous discrimination between freshly produced and remobilized particle populations (Brochard et al. 2017, 2019). However, studies, that have been carried out in the full W AUG over several campaigns and are based on extensive statistics from nearly 2500 discharges (Brochard et al. 2017, 2019), can provide crucial insights into the correlation of enhanced dust rates with off-normal plasma events, such as MDs and VDEs.

2.2.1 Release of freshly generated dust and droplets.

Unstable molten layers formed on PFC surfaces under intense heat flux loads can cause droplet ejection. Evidence from dedicated melting experiments (Krieger et al. 2018; Coenen et al. 2015; Jepu et al. 2019; Corre et al. 2021) rarely reveal disintegrated or splashed pools. Indeed, postmortem deformation profiles typically demonstrate a predominant direction of motion for the frozen melt flow. The prominent exception is material ejection due to the presence of geometrical obstacles, such as PFC edges. Characteristic examples of controlled transient melting (in designed experiments) include splashing of an ELM-induced molten W layer from the leading tile edge in AUG (Krieger et al. 2018) and the splashing of a VDE-induced Be melt from the UDP edge in JET (Jepu et al. 2019). Significant droplet ejection was also reported from accidentally formed leading edges of divertor tiles in Alcator C-Mod (Lipschultz et al. 2012) and divertor monoblocks in EAST (Lei et al. 2021), where it is plausible that material ejection is caused by geometry-driven instabilities (tile corner or sloping bathymetry due to the modification of the surface profile under multiple exposures), although camera observations are not detailed enough to identify the exact dynamics. In Alcator C-Mod, a recent dust collection study has concluded that melting events were responsible for the large, compared to other machines, amount of metal dust and droplets collected in the vessel (Arnas et al. 2017).

In transient melting experiments, the melt layer thickness and melt life time, typically 100 $\upmu$m to several 100’s $\upmu$m and no more than several ten’s of ms (Ratynskaia et al. 2020, 2021), are smaller than those anticipated for ITER transient melting, where predictive modelling reports melt layers in excess of 1 mm for the duration of few 100’s of ms (Coburn et al. 2020, 2021, 2022). From a practical perspective, the unstable modes of interest for melt splashing are those whose wavelength is smaller than the melt pool depth and whose growth time is smaller than the duration of the melt-inducing energetic transient or than the time required for the melt to cross the edge (Bazylev et al. 2008; Pitts et al. 2015; Ratynskaia et al. 2022; Vignitchouk et al. 2022b). Droplet sizes are of the order of the most unstable wave length (Lefebvre 1989; Maroteaux et al. 2002; Brailovsky et al. 1995; Bazylev et al. 2008; Pitts et al. 2015; Ratynskaia et al. 2022), thus the extended thin molten layers created by energetic events with large plasma wetted areas are susceptible to splash into large, fraction of melt thickness, droplets. In Alcator C-Mod, droplets above 100 $\upmu$m were collected (most probable size 50 $\upmu$m with the largest being 450 $\upmu$m) (Arnas et al. 2017), while the melts that spilled from the edge of AUG W tile (Krieger et al. 2018) and the Be UDP in JET (Jepu et al. 2019) formed splats with dimensions up to several millimeters. When the information regarding the splash traces consists exclusively of photographs (i.e. cross-sectional areas), translation of splat sizes to ejected droplet volumes requires knowledge of the maximum droplet spread (Pasandideh-Fard et al. 1998; Liu 1999). In the case of the JET Be splashes on the vacuum vessel wall, two opposite limits yield the sizes of several 10’s of $\upmu$m and several 100’s of $\upmu$m (Jepu et al. 2019; Vignitchouk et al. 2022b).

The splashing of Be flow from the JET UDP edge has been modelled in Ref. Vignitchouk et al. (2022b) and representative dynamics is depicted in Fig. 6. The dimensions of the ligaments are overestimated, but they signify that the ejecta size is comparable to the melt thickness in such a geometry. The characteristic ejection speeds are of the order of the melt layer velocity in both the jetting (a) and intermediate (b) regimes. For analytical ejection speed estimates, a droplet breakaway condition can be employed that is based on a comparison between the kinetic and the cohesive energies of the perturbed free surface (Brailovsky et al. 1995; Bazylev et al. 2008).

Fig. 6

Reproduced from Ratynskaia et al. (2022) with the permission of IOP Publishing

Simulations of liquid Be flows over a straight edge, displaying two different ejection regimes: quasi-steady jetting (left) and the so-called intermediate regime accompanied by material detachment (right) (Vignitchouk et al. 2022b). The melt front is plotted as the dashed line; the injected melt depth h and velocity v are indicated.

It is worth noting that shallow transient melt layers are often stabilized by re-solidification which takes places during contact with the colder surrounding solid surface (Ratynskaia et al. 2020; Vignitchouk et al. 2022b). Other scenarios, where sustained and/or deep melt pools are developed, might display quite different dynamics. For example, pools formed in the TEXTOR melting experiment, that featured a thermally insulated 2 mm thick W plate exposed to plasma over several seconds, exhibited fine $\sim 4\,\upmu$m spraying in addition to the ejection of large droplets 20–100 $\upmu$m (Coenen et al. 2011).

Our discussion has been so far limited to evidence from contemporary fusion devices. A large number of experiments were also carried out in Quasi-Steady-State Plasma Accelerators (QSPAs), multiple mirror magnetic confinement and linear machines aiming to elucidate metal PCF behaviour under high transient heat flux loads  (Tereshin et al. 2003; Klimov et al. 2009, 2011, 2017; Shoshin et al. 2011; Kupriyanov et al. 2015; Arzhannikov et al. 2013; Morgan et al. 2014; De Temmerman et al. 2013; Makhlaj et al. 2013; Garkusha et al. 2007, 2009, 2014, 2015; Makhlai et al. 2019, 2020). Such experiments yield valuable data for the validation and benchmarking of models as they are often accompanied by direct observation of splashing events, providing information on the initial velocities and sizes of ejected droplets. Caution must be exercised when extrapolating these results to other scenarios, since the heat loads might be comparable to those expected in future reactors, but the plasma conditions and specifics of plasma production are often not relevant to tokamaks. The current closure through the end plate in linear machines (providing a ${\varvec{J}}\times {\varvec{B}}$ volume force rotating the melt) De Temmerman et al. (2013), the high—in excess of MPa—plasma pressures in QSPAs (driving the molten layer unstable) Makhlai et al. (2020) and the magnetic field inclination angle are a few characteristic examples.

Unipolar arcs produce localized molten pools of several $\upmu$m extent where the plasma-pressure driven liquid is pushed out and the resulting metallic jets might break into small, micrometer-sized, droplets (Mesyats and Uimanov 2015; Kaufmann et al. 2017; Vignitchouk et al. 2020; Zhang et al. 2019; Wang et al. 2021). The representative droplet ejection speeds are in the range of tens m/s, as revealed by direct laboratory observations (Laux et al. 2013, 2014; Siemroth et al. 2019) and confirmed by numerical modelling (Kaufmann et al. 2017; Vignitchouk et al. 2020). Both laboratory tests and numerical modelling indicate that surface tangential droplet ejection is favored (Siemroth et al. 2019; Kaufmann et al. 2017; Vignitchouk et al. 2020; Zhang et al. 2019; Wang et al. 2021). Multiple evidence of PFC damage by unipolar arcing in contemporary machines confirm the production of dust with characteristic sizes in the micrometer range (Balden et al. 2014; Rohde et al. 2021; Bykov et al. 2017a; Rohde et al. 2013, 2016; Rudakov et al. 2013). The experimental works provide estimates of the total mass loss in arcing processes which exhibits a strong dependence on the composition as well as the conditions of the eroded surfaces. In this light, relative measures of dust production are useful figures of merit; a study in AUG has reported 15$\%$ of the tungsten eroded by arcs was found as dust particles (Rohde et al. 2013).

Runaway electrons constitute another known source of substantial PFC damage (Federici et al. 2001; Peillon et al. 2020; Jepu et al. 2019; Fortuna-Zaleśna et al. 2017a). The specifics of the interaction depend on the electron deposition profile; the depth range of MeV electrons is of the order of millimeters implying volumetric heating. Observations in present day tokamaks include evidence of melt pools and ejection of droplets of the order of 10 $\upmu$m in JET & WEST (Peillon et al. 2020; Jepu et al. 2019; Fortuna-Zaleśna et al. 2017a) but also the release of high speed $\sim 500\,$m/s, 50 $\upmu$m large solid dust in FTU. Modelling has been so far limited to the thermal response (Sizyuk and Hassanein 2009; Bazylev et al. 2013; Chen et al. 2021) (sensitive to the RE incident energy and angles), while there have been no simulations that include RE-specific processes associated to compressive flows and shocks.

Finally, the release of solid dust is mostly associated with delamination of co-deposited layers and cracking. In the former case, information available primary stems from post-mortem analysis in tokamaks, see the discussion above, as well as from QSPA studies (Klimov et al. 2017). Cracking, owing to the thermal stress present in the molten and re-solidified near-surface layers, has been identified as source of solid dust (Pestchanyi et al. 2010, 2011). Modelling and experimental evidence from QSPAs both suggest the production of micron size dust (Pestchanyi et al. 2014).

2.2.2 Remobilization of pre-existing dust

Adhered dust particles are remobilized when the external forces acting on them are able to overcome the contact forces—adhesion and static friction. Below we summarize evidence of dust remobilization by steady-state and transient plasmas as well of prior- or post- discharge dust remobilization by air-flows, vessel vibration and magnetic fields. The discussion concerns solid particles—either permanently solid or droplets that resolidified prior to their contact with the vessel. Liquid drops that impact on the cold(er) vessel wall result in the formation of splats, whose topology is determined by the competition between spreading dynamics (inertia and wetting) and damping due to viscosity and re-solidification  (Pasandideh-Fard et al. 1998; Liu 1999). The rather extensive experience with the thermal and plasma spraying of W and Be coatings  (Castro et al. 1995; Matejicek et al. 2007; Ruset et al. 2011) has confirmed that such solidified splats are unlikely to remobilize given their extended contact area and strong metallic bonding  (Ruzbarsky and Panda 2017).

In steady-state plasmas, when the dust temperature is well below the material melting point, the contact area can be assumed to be unaltered by energy exchange with the plasma (Ratynskaia et al. 2017). Pull-off force is the minimum normal force required to separate two bodies in intimate contact and, in the framework of contact mechanics models for elastic-adhesive contacts, has the general form

$$\begin{aligned} \mid F_\mathrm{a} \mid =\mid F_\mathrm{po} \mid = \xi _\mathrm{a} \Gamma R_\mathrm{d}. \end{aligned}$$

(1)

In the above, $R_\mathrm{d}$ is particle radius, $\Gamma$ denotes the interface energy per unit area with the dimensionless numerical factor being minimum, $\xi _\mathrm{a}=3 /2$, in the Johnson–Kendall–Roberts (JKR) (Johnson et al. 1971) and maximum, $\xi _\mathrm{a}=2$, in the Derjaguin–Muller–Toporov (DMT) model (Derjaguin et al. 1975). Since the pull-off force scales with the contact area that is proportional to the dust size $\propto R_\mathrm{d}$, while all the plasma-induced forces scale with the dust surface $\propto R_\mathrm{d}^2$ and gravitational, inertial, magnetic forces scale with the dust volume $\propto R_\mathrm{d}^3$, it is evident that larger dust (or agglomerates) should remobilize much easier. An illustrative example here is gravity, which becomes comparable to the adhesion only for sizes exceeding one millimeter. For plasma parameters relevant to the ITER divertor and $5\,\upmu$m radius W dust, one finds

$$\begin{aligned} F_\mathrm{po} \sim 10^2 F_\mathrm{id}^\mathrm{sc} \sim 10^3 F_\mathrm{id}^\mathrm{abs} \sim 10^3 F_\mathrm{E} \sim 10^6 F_\mathrm{g}, \end{aligned}$$

(2)

indicating that it is impossible to detach such particles (Tolias et al. 2016). Here $F_\mathrm{id}^\mathrm{sc}$ and $F_\mathrm{id}^\mathrm{abs}$ are the orbital and absorption contributions to the ion drag, $F_\mathrm{E}$ is the electrostatic force¹ and $F_\mathrm{g}$ is the gravitational force. The standard expressions for the ion drag (Hutchinson 2006) and the electrostatic force (Lebedev and Skalskaya 1962) are not valid due to the strong plasma inhomogeneity within the sheath as well as the effect of the boundary in binary collisions (on $F_\mathrm{id}^\mathrm{sc}$), but they can serve as upper limit estimates (Tolias et al. 2016).

Steady state dust remobilization was the subject of an extensive cross-machine study in the Pilot-PSI linear device, the EXTRAP-T2R reverse field pinch and the TEXTOR tokamak (Tolias et al. 2016; Ratynskaia et al. 2017). An important conclusion has been drawn based on the empirical evidence; if a condition for remobilization for a given ‘dust-PFC contact’ is not fulfilled in the beginning of the plasma exposure, then the dust shall remain adhered as long as the plasma conditions are stationary. Despite strong variation of the plasma parameters, consistent results have been obtained: (i) On average, large, $\ge 10$ $\upmu$m, dust and agglomerates remobilize, as expected from the simple scalings, implying that smaller dust is expected to reside longer on PFCs. (ii) The overall dust remobilization rate is higher than aforementioned normal pull-off estimates.

Possible reasons for the enhanced remobilization can be understood with the aid of the force diagram for a spherical dust grain adhered to a smooth planar PFC, as sketched in Fig. 7. Remobilization conditions are obtained from force balance in the normal and the tangential directions and from torque balance around the contact area’s edge (Hubbe 1984; Burdick et al. 2001). Among these, the sliding condition is problematic to evaluate due to involvement of the coefficient of static friction. The rolling condition should be easily realized owing to the small deformation due to adhesion and thus could be responsible for the observed remobilization rates (Tolias et al. 2016). Another possible reason is that in the direct lift-up condition, adhesion is weaker than the JKR/DMT model predictions. This indeed has been established in recent measurements (Riva et al. 2017; Peillon et al. 2019) revealing that contact mechanics models (JKR, DMT) overestimate the adhesion force by about two orders of magnitude, while the van der Waals expression agrees well with experiments. Contact mechanics models implicitly assume that extremely short range strong metallic forces generate adhesion while van der Waals models explicitly assume that long range weak induced multipole interactions generate adhesion. Metallic bonding results from electron exchange interactions characterized by extremely short range and is negligible beyond one nanometer. Thus, omnipresent surface roughness of the order of a few nanometers suffices to switch the dominant contact force from metallic bonding to van der Waals attraction, leading to a dramatic decrease of the surface energy (Tolias 2018; Tolias et al. 2020). Taking into account these experimental and theoretical results, for practical estimates, in the scenario of weakest adhesion that corresponds to rough contaminated surfaces and very low dust impact speed, we recommend

$$\begin{aligned} F_\mathrm{a} = \frac{A}{6 z_0^2} R_\mathrm{d}, \end{aligned}$$

(3)

with A the material-dependent non-retarded Hamaker constant and with $z_0=0.4\,$ nm the distance of closest approach. The room temperature Hamaker constants for different fusion relevant dust-wall combinations have been recently computed within the non-retarded version of the full Lifshitz theory of van der Waals forces  (Tolias 2018, 2020).

Fig. 7

Reproduced from Tolias et al. (2016) with the permission of IOP Publishing

Force diagram of a dust grain in contact with the PFC.

In accumulation sites that feature dust multi-layers or dust agglomerates, particles residing in the upper layers will establish contact with their neighbouring particle(s) rather than with the underlying substrate surface. In the case of particle-particle contact, both contact mechanics and van der Waals models predict weaker adhesion. In contact mechanics models, $R_d$ in Eq. (1) should be substituted with the relative curvature of sphere-sphere contact, $R_{\mathrm{d},1} R_{\mathrm{d},2}/(R_{\mathrm{d},1} +R_{\mathrm{d},2})$, which is smaller than any of $R_{\mathrm{d},1}, R_{\mathrm{d},2}$. In van der Waals models, $R_\mathrm{d}$ in Eq.(3) should again be substituted with the effective radius $R_{\mathrm{d},1} R_{\mathrm{d},2}/(R_{\mathrm{d},1}+ R_{\mathrm{d},2})$ within the Derjaguin approximation (also known as proximity force approximation)  (Derjaguin and Abrikosova 1957; Fosco et al. 2011; Tolias 2022). Overall, the weaker contact strength compared to isolated particles on flat surface and the much larger surface/volume should result to the easier remobilization of agglomerates—as has been confirmed in observations  (Ratynskaia et al. 2017; Tolias et al. 2016).

Systematic adhesion measurements have also been performed aiming to elucidate the influence of different tokamak-relevant non-ideal effects (presence of impurity films, atmospheric contaminants, surface roughness variations) on W-on-W adhesion  (Tolias et al. 2018, 2019). Moreover, an experiment reproducing the scenario of dust adhered on a hot divertor surface has revealed that prolonged tokamak relevant heat treatments even below the W recrystallization range lead to an increase of adhesion up to two orders of magnitude making it comparable to contact mechanics values (Tolias et al. 2020).

The discussion above is limited to dust remobilization from planar surfaces. Dedicated experiments on W dust remobilization from castellated plasma-facing components have been performed in Pilot-PSI (De Angeli et al. 2017) and AUG (Ratynskaia et al. 2018a) to investigate whether gaps can constitute a preferred dust accumulation site. The results indicate that dust is efficiently trapped inside the gaps with important implications for monitoring but also removal.

Empirical data on remobilization velocities are limited to camera observations in Pilot-PSI reporting speeds of 1–2 m/s for 5–25 $\upmu$m W dust (Tolias et al. 2016). Extrapolations to other combinations of size and materials can be carried out with additional assumptions (Ratynskaia et al. 2022).

In transient plasmas, cross-machine (Pilot-PSI, DIII-D, COMPASS, AUG) studies (Ratynskaia et al. 2016, 2017, 2018a, b; Bykov et al. 2017b) have yielded consistent evidence that energy exchange with high energy transients increases the dust bulk temperature and strengthens the dust-PFC contact due to microscopic diffusion and nano-roughness filling. In agglomerates, the top–bottom dust wetting is promoted owing to the essential thermal insulation of the top grains that thus melt easier than the bottom or the isolated grains which are in direct contact with the PFC, as also verified in dedicated heat transfer simulations (Ratynskaia et al. 2017, 2018a). The formation of large spherical grains through wetting-induced coagulation, where wetting and spreading is arrested by resolidification owing to the comparatively short (few ms) ELM duration, was reported in Refs. Ratynskaia et al. (2016, , 2018a, b), Bykov et al. (2017b). Despite their large size, the newly formed grains, that are composed of several to more than a hundred dust monomers (see Fig. 8), exhibit a low remobilization rate due to the strengthening of the contact.

Fig. 8

Formation of large spherical grains through wetting-induced coagulation (Ratynskaia et al. 2017). Dimensions of the original dust population, adhered to the substrate prior to the exposure, can be appreciated from the lower corner images

The possibility that magnetic dust can be mobilized straight into the main vessel volume during the rising of the toroidal magnetic field and impede plasma breakdown was first considered more than two decades ago (Winter 1998). Evidence of dust mobilization at least 300 ms prior to the discharge was obtained from Thomson scattering diagnostic at DIII-D (Rudakov et al. 2009). The first systematic investigations were recently carried out in FTU and provided multiple independent evidence in support of the occurrence of the pre-plasma remobilization of ferromagnetic dust (De Angeli et al. 2019, 2021b). Analytical estimates revealed that the magnetic moment force is sufficient to overcome the adhesion of not only ferromagnetic but also strongly paramagnetic particles prior to tokamak start-up (De Angeli et al. 2019, 2021b).

Dust adhered on a vibrating wall will experience an inertial force that might lead to its remobilization. Vibrational detachment has been considered in small particle removal, see Refs. Ziskind et al. (2000), Ziskind (2006), but also in connection to tokamak dust (Martynenko and Nagel 2012; Brochard et al. 2017; De Angeli et al. 2019). In tokamaks, the vessel motion is typically monitored allowing for an estimation of the acceleration amplitude $A_v \omega _v^2$ with empirical input for the amplitude $A_v$ and the frequency of normal-to-wall harmonic oscillations $\omega _v$. In FTU, typical values for this acceleration are comparable to gravity, thus vessel vibrations are unable to remobilize dust of sizes below 1 mm [see the discussion after Eq. (1)]   De Angeli et al. (2019). Similar conclusions were also reached in AUG, where camera observations combined with strain measurements revealed no correlation between the amount of dust observed and the instances of maximum strain  (Brochard et al. 2017).

Finally, during loss-of-vacuum accidents (LOVAs), vessel isolation from the external ambient is compromised leading to the ingress of atmospheric air which can remobilize particles that could even escape to the environment. Hydrodynamic mechanisms leading to particle removal in different flow regimes and effects of surface roughness have been reviewed in   (Hubbe 1984; Burdick et al. 2001; Ziskind 2006). Dust of relevance to tokamaks and LOVA regimes has been studied in laboratory set-ups and compared to modelling predictions  (Nakaharai et al. 2003; Rondeau et al. 2015a, b; Rossi et al. 2018; Ciparisse et al. 2018).

2.3 Dust–plasmas and dust–wall interaction

2.3.1 Interaction with plasmas

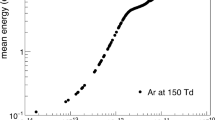
Owing to the large sizes and mass densities of metallic dust and droplets, inertia and initial conditions play a central role in the dust dynamics. Multiple camera observations of intrinsic and injected populations have reported dust trajectories that closely resemble straight lines (Shalpegin et al. 2015a; Autricque et al. 2017a; Brochard et al. 2019), thus indicating that plasma forces within the observation volume are not significant compared to the inertia, see Fig. 9 for examples. Since intrinsic and often even injected dust populations feature size distributions, the fitting of such trajectories yields a limited insight on the dust dynamics and basically no information concerning the validity of the dust heating models (Shalpegin et al. 2015a; Autricque et al. 2017a; Brochard et al. 2019). In fact, given the freedom on the choice of the initial conditions, the simulated trajectories can easily match observations in contrast to the simulated dust life times; overestimated dust sizes or shielding factors had to be employed as a compensation (Shalpegin et al. 2015a; Autricque et al. 2017a; Brochard et al. 2019; De Temmerman et al. 2010; Smirnov et al. 2011). From a practical perspective, heat balance is crucial, since the dust ablation rate determines not only the impurity production but also the solid dust inventory in machines (owing to the fact that the dust size and temperature dictate the final location of dust in the vessel and whether if can be remobilized in subsequent discharges Ratynskaia et al. 2022), see also Fig. 4. The direct validation of heating models is challenging experimentally as, ideally, both the dust radius and temperature must be known or measured, but also interpretation-wise due to insensitivity of the dust temperature to the plasma parameters in the strong vaporization regime (Skovorodin et al. 2016; Vignitchouk et al. 2018a). The dust temperature has been inferred from thermal radiation, for instance in TEXTOR experiments with injected C dust (Kantor et al. 2013), in Pilot-PSI experiments with controlled injected monodisperse W dust (Vignitchouk et al. 2018a) and in QSPA experiments with droplets splashed from a molten W layer (Klimov et al. 2009).

Fig. 9

Reproduced from Shalpegin et al. (2015a) with the permission of IOP Publishing. Reproduced from Brochard et al. (2019), $\copyright$2019 The Authors of Brochard et al. (2019). Reproduced from Autricque et al. (2017a), $\copyright$2017 The Authors of Autricque et al. (2017a)

Camera observations of W dust trajectories in the TEXTOR (Shalpegin et al. 2015a), AUG (Brochard et al. 2019), KSTAR (Autricque et al. 2017a) tokamaks.

The increased role of inertia for heavy and large dust implies a stronger effect of the initial conditions. Dynamics-wise the main forces and scalings outlined in earlier reviews remain relevant (Krasheninnikov et al. 2011, 2020), while the release conditions have been discussed in the preceding subsections and in Ref. Ratynskaia et al. (2022). Thus, below we focus on dust and droplet life time as controlled by surface processes but also by effects resulting from vapor shielding and rotational dynamics.

Numerous surface processes determine the dust surface potential and the heating power $Q_{\mathrm {tot}}$. Dust charging is due to collection of electrons, denoted by a subscript ’e’, and ions, denoted by a subscript ’i’ (index ’j’ running over all ionic species) as well as due to electron- and ion-induced electron emission (EIEE and IIEE), and thermionic emission (TE). The dust potential is determined by the floating condition

$$\begin{aligned} I_{\mathrm {e}}+\sum _j\,I_{i,j}+I_{\mathrm {EIEE}}+I_{\mathrm {IIEE}}+I_{\mathrm {TE}}=0\,. \end{aligned}$$

(4)

EIEE becomes important at high electron temperatures (Tolias 2014a, b), the potential contribution of IIEE becomes important in the presence of noble gas or multiple charged ions (Vignitchouk et al. 2018c) and TE becomes important for heated W dust or droplets. The total heating power includes contributions from the same processes but also from ion backscattering (subscript ’bs’), thermal radiation (subscript ’rad’) and vaporization (subscript ’vap’).

$$\begin{aligned} Q_{\mathrm {tot}}&=Q_{\mathrm {e}}+\sum _j(Q_{i,j}+Q_{\mathrm {i},j}^{\mathrm {bs}})+Q_{\mathrm {EIEE}}+Q_{\mathrm {IEEE}} \\&+Q_{\mathrm {TE}}+Q_{\mathrm {rad}}+Q_{\mathrm {vap}}. \end{aligned}$$

(5)

Recall that, similar to arcing modelling (Benilov and Benilova 2010; Kaufmann et al. 2017) and to plasma–wall interaction modelling (Komm et al. 2017a; Ratynskaia et al. 2021), the electron $Q_\mathrm{e}$ and ion $Q_i$ heating terms do not only stand for the kinetic (dust potential dependent due to the sheath acceleration) contributions but also include chemical contributions. Denoting the collected particle fluxes by $\Gamma _{\mathrm{e},i}$, for the collected electrons, we have $Q_\mathrm{e}^\mathrm{chem}= A_\mathrm{d} \Gamma _\mathrm{e} W_\mathrm{f}$, with the work function being $W_\mathrm{f} \sim$ 4–5 eV for most metals, that can be the dominant contribution in cold $T_\mathrm{e} \sim 1\,$ eV detached divertor plasmas, as confirmed in Ref. Vignitchouk et al. (2018a). For the collected ions, $Q_i^\mathrm{chem}= A_\mathrm{d} \Gamma _i (U_{iz}- W_f + U_\mathrm{sb})$, with the main contribution from the stored ionization energy $U_{iz}$ released upon neutralization. Surface neutralization heating becomes significant in the presence of multiply charged ions, see an example of heating rates for specific ITER disruption profiles in Ref. Vignitchouk et al. (2018c).

It is important to decompose electron-induced electron emission in the processes of secondary electron emission, inelastic electron backscattering and quasi-elastic electron reflection (Bronshtein and Fraiman 1969). Owing to the different underlying physics, each process features different energy distributions of the emitted electrons² and thus leads to varying contributions to the dust cooling per ejected electron (Tolias 2014a, b; Vignitchouk et al. 2018c). There are also indirect effects, such as the suppression of the thermionic emission flux $\Gamma _\mathrm{TE}$ due to the formation of a potential well (Delzanno and Tang 2014a; Vignitchouk et al. 2018b) or due to prompt re-deposition (Autricque et al. 2017b), that can lead to more negative dust potential values and thus to reduced incident electron fluxes (compared to the unimpeded emission case). The corresponding reduction in cooling due to thermionic emission, $Q_\mathrm{TE} \simeq A_\mathrm{d} W_\mathrm{f} \Gamma _\mathrm{TE}$ is not crucial as, courtesy of the ambipolarity condition for a floating body and provided that thermionic emission is the dominant emission processes, the $W_\mathrm{f}$ contribution to the energy balance cancels out (Vignitchouk et al. 2018c; Ratynskaia et al. 2021).

The heating power is dictated by the functional form of the collected particle fluxes which is determined by the hierarchy of plasma scale lengths and the characteristic dust size. The typical scenarios of interest for dust transport in contemporary tokamaks as well as in ITER disruption and ramp-up scenarios fall under the electron temperature range $T_{\mathrm {e}}$ =1–100 eV, the plasma density range $n_{\mathrm {e}}= 10^{18}$—$10^{21}~\text {m}^{-3}$ and the magnetic field range B = 2–5.3 T. With $T_\mathrm{e} \sim T_i$ and dust sizes as discussed above, the majority of dust and droplet populations follow the scale ordering of ${R}_{\mathrm {Li}} > R_{\mathrm {d}}\gg {R}_{\mathrm {Le}},L_{\mathrm {De}}$. Here ${R}_{\mathrm {Le},i}$ and $L_{\mathrm {De}}$ denote electron/ion Larmor radius and Debye length, respectively. Since the ions are unmagnetized, the thin sheath correction to the Orbital Motion Limited (OML) approach (Willis et al. 2012) or the Orbital Motion (OM) theory (Laframboise 1966; Allen 1992; Delzanno et al. 2005) can be used. On the other hand, for the magnetized electrons, there are major modifications in the collected fluxes.

In such regime, the electron transport is collisional, even though the dust size is much smaller than the electron mean free path $\lambda _\mathrm{e}$, owing to the extension of the collection area along the magnetic field ($R_\mathrm{d} \lambda _e / R_{Le} \gg \lambda _\mathrm{e}$). The solution for attracted electrons is known as the Bohm saturation current  (Bohm et al. 1949; Rozhanskiĭ and Tsendin 2001). The Bohm current scaling of $\varpropto n_\mathrm{e} R_\mathrm{d} T_\mathrm{e} /B$ essentially implies a $R_{Le}/R_\mathrm{d}$ suppression of the thermal current. The kinetic solution of the problem by Sanmartin (1970) revealed that for dust potentials close to the plasma potential, a potential overshoot or ’hill’ is formed due to the strong reduction of the saturation current compared to the thermal electron flux. Fluid models for the electron current have been constructed that remarkably match the kinetic solution of Sanmartin (Rozhanskiĭ and Ushakov 1998; Stangeby 1982). The presence of the potential hill can be incorporated (Vignitchouk et al. 2017) in the thin sheath ion collection models of Lam (1965) and Laframboise (1966).

The strongly inhibited electron collection naturally increases dust life time (Vignitchouk et al. 2017). Another important consequence in such collection regime is the reduced sensitivity of the estimated incoming heat flux to the dust floating potential. The reason is the absence of the exponential behavior inherent to OML currents for repulsive potentials. Indeed, the stiff coupling between charging and heating in the OML framework gives rise to the well-known thermal instability due to thermionic emission (Smirnov et al. 2007b), whereupon an increase of thermionic emission leads to a shift of the dust potential towards positive values, which in turn enhances the incoming heat flux, further increasing the temperature and the emission. In the magnetized collection, flattening of the electron current at small and modest repulsive potentials results in electron emission becoming a less important factor in determining the evolution of the dust temperature (Vignitchouk et al. 2017).

It should be emphasized that the large scale separation, $R_{\mathrm {d}}\gg {R}_{\mathrm {Le}},L_{\mathrm {De}}$, allows to take advantage of relevant results derived for planar surfaces, such as those concerning emission in magnetized sheaths (Tskhakaya and Kuhn 2000; Igitkhanov and Janeschitz 2001; Takamura et al. 2004; Komm et al. 2017a, b, 2020). For example, in the absence of electrostatic fields, the attenuation factor of the emitted flux due to prompt re-deposition can be analytically found to be $\sin \alpha$ (Gunn et al. 2017; Komm et al. 2020), where $\alpha$ is the magnetic field inclination angle. In such a case, integration over angles for spherical geometry yields an overall reduction of electron emission by the factor of 1/2 compared to the unimpeded value.

Another crucial aspect of heat balance is shielding, associated with evaporated dust material surrounding the dust particle, that is important not only for the reduction of the incident heat flux (Parks et al. 1977; Rozhansky and Senichenkov 2005; Marenkov and Krasheninnikov 2014), but also for other features of dust–plasma interactions including forces and torques, which the plasma exerts on the particles.

We note that the rotational dynamics can also affect the dust and droplet lifetime. Different plasma mechanisms have been suggested over the years that result in a torque that is exerted on “bare” (unshielded) grains in fusion devices. These mechanisms are related to the gyro-motion of plasma particles, electric fields associated with plasma instabilities, electric currents, etc. (Krasheninnikov et al. 2020). The spinning of a droplet leads to a centrifugal force $F_\mathrm{c}$, which can only be balanced by surface tension force $F_\mathrm{tens}$, so that, when angular velocities $\Omega _\mathrm{d}$ exceed a critical value $\Omega _\mathrm{d}^\mathrm{crit}$, the droplet will be ripped apart. Crude estimates can be obtained by comparing $F_\mathrm{c}\sim R_\mathrm{d}^3 \rho R_\mathrm{d} \Omega _\mathrm{d}^2$ with $F_\mathrm{tens}\sim \sigma R_\mathrm{d}$, yielding $\Omega _\mathrm{d}^\mathrm{crit}\sim \sqrt{\sigma /(R_\mathrm{d}^3 \rho )}$. Here $\rho$ is the mass density and $\sigma$ the surface tension of the material. This estimate differes from more refined calculations of $\Omega _d^\mathrm{crit}$ triggering instabilities and droplet break-up (Chandrasekhar 1965) only by a constant of the order of unity. Employing $\rho = 18 \times 10^3\,$ kg/m$^3$, $\sigma =2.5\,$ N/m (Tolias 2017) for a W droplet of $R_\mathrm{d}=10\,\upmu$m, one finds $\Omega _\mathrm{d}^\mathrm{crit} \sim 10^6$ s$^{-1}$, a value that can be reached in the tokamak plasmas. Estimates for the dust spinning angular velocity due to the collection of a single species have been made in the original work of Ref. Krasheninnikov (2006) and were later applied to explain experimental observations in JET (Holgate et al. 2019). The net torque, consisting of competing electron and ion contributions which can also feature a different dust radius dependence of the collected particle flux, depends non-monotonically on $R_\mathrm{d}$ and crosses zero for an intermediate $R_\mathrm{Le}<R_\mathrm{d}<R_\mathrm{Li}$ (Ratynskaia et al. 2022). For collisionless plasmas, Monte-Carlo simulations of the net torque, also including contributions due to asymmetric current collection, have been carried out (Simons and Long 2021). It is also worth noting that for an appreciable effect on dust lifetime, the characteristic spin-up must be sufficiently small to allow multiple splitting events as the per event $R_\mathrm{d}$ reduction is merely by the factor of $(1/2)^{1/3}$.

Similarly, a strong centrifugal force could also lead to the rotational break-up of solid dust particles if the angular velocity exceeds $\sim \sqrt{\sigma _{y}/(R_\mathrm{d}^2 \rho )}$. Since for metals the yield strength $\sigma _{y}$ is typically above 100 MPa ($\sim 400\,$ MPa for Be and $\sim 600\,$ MPa for W at room temperature), $\Omega _\mathrm{d}^\mathrm{crit}$ is larger than for droplet break -up and such effect is only possible for rather high values of $\Omega _\mathrm{d}$. However, it is plausible that some relatively loosely bounded conglomerate particles, having a much lower effective yield strength, could be disintegrated due to spinning. Moreover, the yield strength features strong temperature and size dependence, in particularly $\sigma _{y}$ decreases at high temperatures while it increases with smaller sizes of particles. Coupling of both effects has not been investigated experimentally, thus only combinations of known temperature and size dependencies are viable, for example by analogy with the Hall-Petch grain-boundary strengthening phenomenon, see Vignitchouk et al. (2014) for a detailed discussion.

2.3.2 Interaction with the vessel

Elastic-perfectly plastic adhesive dust collisions Impacts of dust on the vessel walls and PFCs are inherent part of dust dynamics (Krasheninnikov et al. 2011; Rudakov et al. 2008; Ratynskaia et al. 2008, 2011), enabling long-distance migration (Ratynskaia et al. 2013). For dust speeds of relevance to fusion machines (a few to few 100’s m/s), the collisions are characterized by energy losses due to adhesive work, plastic deformation and frictional dissipation, with the outcome strongly dependent on the projectile’s size and velocity along with the material properties of the projectile and the surface (Johnson 1985; Stronge 2000).

Mechanical impacts involve short-range forces and are instantaneous compared to time-scales relevant for dust motion. Due to the impulsive nature of the interactions, they can be quantified with restitution coefficients, thus enabling the application of impact mechanics models. The normal velocity component can be analyzed treating dust as a elastic-perfectly plastic adhesive sphere within the Thornton & Ning model (Thornton and Ning 1998), while the tangential velocity component can be treated by rigid body theory for frictional contacts (Gorham and Kharaz 2000). Given the lack of reliable experimental values for the friction coefficient and consistency issues concerning rotational dust dynamics, the simplification of a frictionless contact can be employed (Ratynskaia et al. 2013; Tolias et al. 2017). Such model predictions have been validated in plasma environments in dedicated experiments with controlled dust injection (Ratynskaia et al. 2015; Shalpegin et al. 2015b; Tolias et al. 2017). Dust trajectories recorded in Pilot-PSI with unprecedented resolution (Shalpegin et al. 2015b) provided visualization of basic processes predicted by the contact mechanics models, for instance bouncing from the wall is accompanied by a gradual reduction of the normal velocity component until it is reduced to the adhesive velocity value. The adhesive velocity $v_\mathrm{s}^\mathrm{adh}$ is the maximum velocity resulting in sticking during elastic impacts, depends on the material properties and is nearly inversely proportional to the dust radius

$$\begin{aligned} v_\text {s}^\text {adh}=\frac{\sqrt{3}}{2}\pi ^{1/3}\sqrt{\frac{1+6\times 2^{2/3}}{5}}\left( \frac{\Gamma ^5}{\rho _\text {d}^3E^{*2}R_\text {d}^5}\right) ^{1/6} ~, \end{aligned}$$

(6)

where $E^{*}$ is the reduced Young modulus and $\Gamma$ the interface energy (Thornton and Ning 1998). The $v_\mathrm{s}^\mathrm{adh}$ scaling with $R_\mathrm{d}$ is responsible for selectivity in migration (Ratynskaia et al. 2013); larger dust bounces easier and tends to accumulate at the bottom of the machine, while smaller dust tends to get stuck on surfaces, independent of their orientation with respect to gravity, upon its first encounter with the wall. Figure 10 presents impact outcomes corresponding to small (left, straight trajectory) and to substantial (right, bouncing trajectory) losses of the normal kinetic energy. The latter type of impact is characteristic of elevated dust temperatures, where losses due to plastic deformation are enhanced by the decreased yield strength. Note that purely contact-mechanical models cannot account for the large near-wall sheath electric fields which can significantly reduce the normal velocity or even reflect the particle prior to the actual impact. The effect, however, is only relevant for $R_\mathrm{d} \ll L_\mathrm{De}$, otherwise the dust grain is too large to be approximated by a point charge at the sheath scale.

Fig. 10

W dust—W surface collisions recorded in a Pilot-PSi experiment with controlled dust injection (Shalpegin et al. 2015b); impact outcomes corresponding to small (left, straight trajectory) and to substantial (right, bouncing trajectory) losses of the normal kinetic energy

Droplet collisions The above discussion refers exclusively to solid dust. If metal droplets collide with a surface, considering the temperature difference with the much colder wall, the outcome is determined by the competition between spreading dynamics, due to inertia and wetting, and damping, due to viscosity and re-solidification (Pasandideh-Fard et al. 1998; Liu 1999). These processes depend on the impinging droplet size and velocity but also on the respective temperatures and thermo-physical properties of both materials in contact. Estimates for Be splashes on the JET wall can be found in Ref. (Vignitchouk et al. 2022b).

Hypervelocity collisions Dust-wall impacts fall into the hypervelocity (HV) regime when the impact speed approaches or exceeds the compressional wave speed in both materials. Pure HV impacts are accompanied by complete dust vaporization and wall crater formation with the excavated volume possibly far exceeding that of the dust. Thus, HV impacts constitute a potential unidentified source of gross erosion. Postmortem evidence of HV impacts in FTU was first reported more than a decade ago (Castaldo et al. 2007; Ratynskaia et al. 2008a). However, physical mechanisms capable of accelerating dust to such high speeds in tokamak relevant scenarios and geometry had not been yet identified [though in laboratory experiments acceleration by the ion drag to dust velocities of $\sim 4$ km/s has been achieved  Ticos et al. (2008)]. Recent postmortem evidence from FTU (2013 shutdown, 2019 decommissioning) not only further supported the realization of HV impacts but also revealed a strong correlation with RE-induced damage (De Angeli et al. 2022). To be more specific, it has been suggested that RE dissipation on the limiter leads to an explosive damage event which leads to the ejection of $\sim 1$ km/s solid dust. Currently, no numerical tools have been developed that are capable of accounting for solid–solid and solid–fluid interactions realized in such explosive regimes (Ratynskaia et al. 2022). Two-stage light gas gun experiments that have been performed with the relevant projectile sizes and speeds have been shown to closely reproduce the HV impact damage observed on the FTU PFCs (Tolias et al. 2022).

3 Powder injection for fusion applications

In this section, we review recent experiments where dust or powder have been introduced in fusion plasmas intentionally, as a research tool for fusion, with applications that go well beyond the study of dust dynamics. We will limit our focus to experiments in tokamak and stellarator devices.

In this context, the term powder refers to materials in form of fine solid particulates of size much smaller than 1 mm, produced externally to the fusion device. The distinction between powder and dust relies on the fact that powders have predefined morphology and composition, whereas for dust these are the result of plasma material interaction and migration processes and, therefore, uncontrolled.

The earliest use of injection of particulate in plasmas to assist the operation of fusion plasmas stemmed from the seminal work of Mansfield in the TFTR tokamak, where Li aerosol produced by laser ablation was utilized to mitigate plasma–wall interaction and specifically to reduce fuel recycling from the carbon PFCs (Mansfield et al. 1996; Labik et al. 1997). Follow-up experiments explored injection of Li powder, in the form of stabilized micro-spheres, relying on ablation of the particulate by the SOL plasma (Osborne et al. 2015). More recently, the development flexible powder dropper systems capable of handling a variety of materials in powder form (Nagy et al. 2018, 2019) has spurred a research path leveraging powder injection to facilitate the operation and improve the performance of a fusion device under a variety of conditions. These systems have been implemented and utilized in a number of fusion devices, tokamak and stellarators alike, and presently, impurity powder injection is an area of active experimental investigation in DIII-D (Bortolon et al. 2020), ASDEX-Upgrade (Bortolon et al. 2019a; Lunsford et al. 2019), KSTAR (Gilson et al. 2021), EAST (Maingi et al. 2020; Sun et al. 2021), WEST (Bodner et al. 2022), LHD Nespoli et al. (2020) and W7-X (Lunsford et al. 2021).

Typically the powder injector system consists in one or more reservoirs coupled to vibratory feeders, which provide controlled delivery of powder to the plasma. Most implementations utilize gravitational injection, through vertical drift tubes leading to the vacuum vessel. Reservoirs and feeders the powder is agitated by virtue of piezoelectric actuators allowing to adjust the delivery to previously calibrated mass rates.

Table 1 Characteristics of powders utilized in tokamak and stellarator experiments, for different applications: wall conditioning (WC), pedestal control (PC), heat exhaust (HE), and energy confinement (EC)

The impurity injection location for powders does not have the flexibility provided by gas injection system manifolds. In powder injection experiments, the role of injection location with regard to the plasma shape is explored by varying the magnetic configuration of the target plasma. While in most cases the powder is dropped into the plasma crown [see for instance Ref. Bortolon et al. (2018); Gilson et al. (2021)] this approach allowed to study injection in divertor proximity or directly at the strike point the target (Effenberg et al. 2021). In LHD, the transport of powder in SOL flows with varying degrees of ergodicity has been studied (Nespoli et al. 2020; Shoji et al. 2020).

The ability of injecting solid materials in powder form greatly extend the range of impurities that can be injected in the plasma, to include low-Z (Li, Be, B, C) and weakly recycling materials. Table 1 provides a summary of powder materials that have been used experimentally and with potential for use in the future.

It is important to note that powder injection for fusion applications typically requires injection rates that are substantially higher than in dust dynamics studies, aiming to deliberately affect the main plasma behaviour and/or the PMI. The mass rates depend on the mass density of the material. For light elements such as Li and B the mass rate ranges from 1 to 100 mg/s, which corresponds to $10^{19-21}$ atoms/s, i.e. several orders of magnitude larger than the sputtering sources but in the order of magnitude of the gas injection rates for radiative divertor applications.

The gravitational injection is associated with small injection speeds which typically are in the range of 5–10 m/s, depending on spatial separation between the feeder device and the plasma edge. At these low speeds, the powder particles are typically ablated by the SOL plasma in the vicinity of the injection location. Due to the restrictions associated with safety and handling, Be and Be compounds have not been exploited to date. Nevertheless the Be-rich materials have potential applications for machines with Be wall, such as JET and ITER. The size of the powder can be tailored depending on the application. Particle size $<10~\upmu$m tend to ablate in the scrape of layer, producing atomic impurity source external to the confined plasma. Conversely, particles of 50–200 $~\upmu$m can transit through the SOL to generate a source of impurities inside the magnetic separatrix. The penetration depth is strongly influenced by the particle size and material sublimation energy. For instance, in Ref. Osborne et al. (2015) 40 $\upmu$m Li micro-spheres due to the low sublimation energy ablate primarily in the far SOL. Ablation from supra-thermal ions populating the SOL, such as those generated by injection of heating neutral beams in high density plasmas can also contribute to the ablation of particles in the SOL (Bortolon et al. 2019b).

Strong toroidal localization of the plasma powder interaction was reported from B and BN powder injection in AUG (Bortolon et al. 2019a; Lunsford et al. 2019). Figure 11 shows emission from B from SOL as well as particles that are dragged with parallel SOL flows. The location of injection with regard to the magnetic configuration has a strong effect on the distribution of the injected material. In the common case of single-null tokamak configurations parallel SOL flows exhibit a stagnation point near the plasma crown. For injection inboard of the stagnation point the ablated and ionized impurity will be transported towards the inboard target, as shown in Fig. 11b. Substantial fluxes to the outer target are still possible indirectly via the confined plasma, which can be reached by the injected impurity by deep penetration of powder particles or perpendicular transport.

Fig. 11

Reproduced from Bortolon et al. (2019a) with permission from Elsevier

True color camera image from B powder injection in AUG. Emission from B ionis follows the field lines, wrapping around the plasma towards the inner target.

Powder injection has been typically utilized for applications in general areas: (i) real-time wall conditioning, (ii) pedestal control, (iii) heat exhaust and (iv) improvement of energy confinement. In the following we review experimental results for these areas separately, introducing examples of experimental results obtained in different tokamak and stellarator devices. The intent of this section is to provide an overview of the experimental results in this relatively young research domain, emphasizing the diversity of applications where powder injection can find a role.

3.1 Real-time wall conditioning

It has long been established that the conditions of the PFC affect directly the performance of fusion plasmas. It has also been recognized that deposition of low-Z coatings on the vessel wall can have beneficial effects in terms of reducing recycling and wall fueling as well as minimizing impurities. Wall conditioning techniques such as glow discharge boronization (GDB) (Winter 1996) or Li evaporation (Skinner et al. 2011; Sugai et al. 1995) are used routinely to enable robust high performance operation. Recent experiments have shown that the beneficial effects of these techniques can be obtained also by injection of Li and B powder directly onto the confined plasmas. In this case, injected material is typically ablated within the SOL and transported to the PFC, resulting in deposition of protective, low-Z coatings. In AUG, injection of B and BN powder was explored experimentally with the goal of coating primarily the ICRH antenna limiters, which represent a major source of W sputtering, preventing operation at low plasma density without a fresh GDB. Cumulative injection of 200 mg of B in a series of high-density discharges with co-formal wall resulted in lower W sputtering from the limiters and appeared to enable operation of low density scenarios, such as ELM-suppressed plasmas, without prior GDB (Bortolon et al. 2018). Similar experiments are reported from the DIII-D tokamak, where a series of plasma discharges with B powder injection were executed after vessel vent in air and before GDB. Injection of B powder in quantities  2–10 mg was sufficient to enable density control in the initial plasma current ramp in L-mode, suggesting a strong reduction of plasma fueling from wall desorption and D recycling. Figure 12 shows that after the first shot with B injection a clear increase of wall retention was estimated. Concomitant with the effect on D retention, a reduction of intrinsic impurities, in particular C, O, and N were documented. Notably, in both AUG and DIII-D experiments, the observed improvements in wall condition figures of merit were obtained with injection of cumulative quantities $\approx 200$ mg, i.e. much smaller than the amount deposited on the vessel walls by a typical GDB boronization cycle $\sim$ 10–20 g.

In short pulse machines such as AUG and DIII-D, for the relatively short pulse length $\sim$ 4–10 s, the improvement of wall condition observable is typically evaluated by shot-by-shot evolution. However, improvements in wall conditions within the same plasma discharge, i.e. in real-time, have been documented in long pulse devices. In the stellarator LHD, injection of B powder during long plasmas caused a reduction of oxygen radiation, which was maintained at low levels after the end of powder injection phase (Nespoli et al. 2020). Wall conditioning effects have been well documented with Li powder injection in EAST Sun et al. (2021).

Fig. 12

Reproduced from Bortolon et al. (2020) with the permission of IAEA Publishing

Increase of deuterium retention rate estimated from particle balance over a series of DIII-D plasma discharges without (176705–176707) and with B powder injection (176708–176711).

The growth of a B rich layer has been demonstrated exposing sample substrates to plasma fluxes with B powder injection. Figure 13 shows a graphite sample from the Divertor Materials Evaluation System (DiMES) (Wong et al. 1998) after exposure to DIII-D plasmas with cumulative injection of 170 mg of boron (Bortolon et al. 2020). The sample was exposed in the divertor in the far SOL region of the outer target. Elemental concentration measurements by X-ray Photon Spectroscopy (XPS) found a surface layer of 2 nm thickness with B and C in equal concentrations, consistent with observations from GDB in graphite wall devices.

The use of isotopically enriched B allowed to distinguish B from the injected powder from residual natural B from the diborane gas used in GDB. In this case the isotopic ratio matched that of the injected material demonstrated. The results indicate B-rich film growth rate $\approx 0.1$ nm/mg at this location of net deposition (Bortolon et al. 2020). Similar experiments in AUG have found similar deposition rates in the outer target far SOL. Much larger deposition rates have been reported in the private flux region (Bortolon et al. 2019a) near the outer strike point, resulting in growth of a macroscopic film of thickness $\le 1~\upmu$m after 100 mg of injected B. The mechanisms conducive to deposition the private flux region are still under investigation: this could be associated with cross field transport at the x-point and along the divertor leg, or alternatively, radial migration of re-eroded material at the strike point.

Fig. 13

Reproduced from Bortolon et al. (2020) with the permission of IAEA Publishing

Surface analysis of the DiMES sample exposed to outer target SOL plasma flux during B powder injection indicates deposition of B:C film. a DiMES sample after exposure to 12 plasma discharges. Map of intensity of B$^{11}$ b isotopic ration measured with Laser Ablation Mass Spectroscopy. The relative concentration of B$^{11}$ c indicates an isotope abundance $\sim$98.5% consistent with the nominal specification of the injected B powder. d Relative abundance profile of C, B, and O by x-ray photoelectron spectroscopy (XPS).

3.2 Pedestal control

Low-Z powder injection in tokamaks has also been observed to have a strong effect on the structure of the H-mode pedestal. A number of experiments have shown substantial improvements during powder injection, in terms of promoting a wider and higher pedestal pressure. This is often accompanied by the presence of benign plasma instabilities, localized in the pedestal region, which enhances particle transport. In certain conditions, ELM suppression was also observed. This behaviour is well represented by DIII-D experiments where Li injection in H-mode plasmas with relatively low NB heating of 3 MW induced ELM-free phases (Fig. 14) with pedestal expanding radially to reach pressures more than double compared to the reference ELMy phases without Li (Osborne et al. 2015). A bursting-chirping instability was observed, localized at the pedestal, causing an increase of particle transport, which, in turn, effectively steers the pedestal growth away from the ELM stability boundary. At sufficiently high pedestal pressure threshold, the ELMs return.

Fig. 14

Reproduced from Osborne et al. (2015) with the permission of IAEA Publishing

Right: True color picture of DIII-D plasma with Li powder injection. Left (a–f): evolution of selected plasma and pedestal parameters. The shaded time intervals correspond to ELM-free phases with wider and higher pedestal and enhanced confinement.

Boron powder injection in EAST has been observed to robustly induce a ELM-free scenario, with stored energy similar or higher than the reference ELMy scenarios and a substantial reduction of W concentration in the the core (Sun et al. 2019). This scenario has been obtained and maintained under a wide range of auxiliary heating power (1–4 MW, RF and/or NB). In this case, harmonic oscillations have been observed in conjunction with the ELM suppressed regime (Fig. 15), which appear to be localized in the pedestal region (Sun et al. 2021). These were ascribed to a radially localized increase particle transport, counteracting and reducing W accumulation to the core.

Fig. 15

Reproduced from Sun et al. (2021), with the permission of AIP Publishing

Evolution of selected plasma parameters for a EAST plasma with B powder injection: plasma current (a), electron density (b), auxiliary power (c), stored energy (d), tungsten, boron and deuterium line emission (e, f, g), magnetic fluctuations (h) and spectrograms of XUV emission from the plasma edge (i, j) During B injection, a long ELM-suppressed scenario is obtained, with lower recycling (g), higher stored energy (d) and lower W concentration (e). The scenario is characterized by harmonic instabilities in the pedestal, as shown in the spectrogram of XUV line emission from the plasma edge (j).

Long lived ELM suppressed regimes without loss of confinement have been reported from KSTAR, with BN injection in H-mode plasmas (Gilson et al. 2021). ELM free phases of 1–2 s, were observed injection in response to pulses of 1–2 mg of BN powder. This ELM free pedestal was not correlated with appearance of pedestal instabilities suggesting a change to pedestal structure associated with dilution or reduction of neutral fueling.

3.3 Energy confinement

Several experiments have also demonstrated that low-Z powders can promote improvements of energy confinement, with a number of physics mechanisms proposed to explain the observed effects.

Reduction of edge cooling by reduction of recycling has been identified as the leading mechanism for improvement of confinement by Li aerosol injection in TFTR (Mansfield et al. 1996) (L-mode plasmas in limiter configuration), resulting in substantial increase in ion temperature, leading to higher fusion neutron production rates.

Modifications of the interplay between SOL and pedestal can also lead to improvement of plasma performance. This is the case for the continuous injection of BN powder in AUG W-wall (Lunsford et al. 2019) leading to simultaneous increase of stored energy and radiative losses from the edge. The underlying mechanism is traced to a change of pedestal stability associated with the cooling of inboard SOL plasma, which is the main contributor to pedestal fueling (Dunne et al. 2017; Wolfrum et al. 2017), causing an outward shift of the electron density pedestal.

Reduced energy transport in the core plasma has also been identified as a possible cause of improvement of confinement associated with low-Z powder injection. This can arise from a change of the dominant type of micro-instabilities, per effect of the increase of the dilution (Ennever et al. 2015) and collisionality (Mordijck et al. 2020) associated with a finite concentration of low-Z impurities in the plasma. These effects are maximized near the plasma edge or in the H-mode pedestal, where the ablation can lead to significant increases of the plasma effective charge $Z_\mathrm{eff}$ (Osborne et al. 2015; Lunsford et al. 2019).

Increase of confinement in the WEST tokamak have been reported from recent experiments with B powder injection in L-mode plasmas on full W wall (Bodner et al. 2022). Along with the improved wall conditions, WEST plasmas with B powder injection displayed significant increases of stored energy, neutron rate, and electron temperature (up to 25% for stored energy and 200% for the neutron rate) at constant input power. These increases in confinement scaled up with the powder drop rate and were initially attributed to suppression of ion temperature gradient (ITG) turbulence from changes in and/or modifications to the electron density profile.

Similar observations of improvement of confinement have also been reported from stellarator devices. Transient improvements of energy confinement has been reported in W7-X upon B$_4$C powder injection (Lunsford et al. 2021). There, 50 ms injections at increasing amounts from 1 to 30 mg/pulse, at 2.5 Hz, were applied from the outer equatorial plane using an injector installed on a manipulator probe (Nagy et al. 2019). Once the injected boron carbide was fully absorbed, the density rise transitioned to the core while the stored energy increased above the previous baseline level by an amount linearly correlated with the injection quantity. During the injection phase, the ion temperature gradient steepened with peak core ion temperatures observed to increase from a nominal 1.7 keV to over 2.6 keV for the largest injection amounts. Enhanced performance was accompanied by a reversal of the radial electric field in the core, indicating that the core transport has switched to the ion root associated with changes to ion root transport and increase of electron-ion coupling by increase of collisionality.

Recent results in LHD reported consistent observations of increased ion temperature during B powder injection (Nespoli et al. 2022), in a variety of configurations and auxiliary heating schemes. The increase was sustained for time windows of multiple seconds, during B injection at flow rates of 10–50 mg/s. Analysis of core turbulence diagnostics showed a reduction of turbulence in the range of frequency of ion temperature gradient modes. As a result, the core energy confinement, inferred from power balance, was observed to increase by up to 20% in the range of plasma conditions explored (Fig. 16).

Fig. 16

Reproduced from Nespoli et al. (2022), $\copyright$2022 The Authors of Nespoli et al. (2022)

a–e Evolution of selected parameters for two plasma discharges from the LHD stellarator. In the plasma with continuous B injection (red) higher electron and ion temperatures are observed compared to a reference discharge without B (blue). The high confinement regime with B injection is characterized by reduction of density fluctuations due to turbulence (f-g). The scenario showed improvement of confinement over a wide range of density (h).

3.4 Power exhaust

Finally, injection of low-Z materials in powder form has found applications in the problem of power exhaust, in particular expanding the range of impurity radiators beyond the typical gases commonly used for this application, N$_2$ and Ne. In particular, the relevance of low-Z radiators has emerged as a potential solution for coupling an radiative divertor with high performance core. For instance, Pigarov Pigarov (2017) has shown through systematic modeling that, for a mid-scale tokamak, B has optimal properties in terms of maximizing divertor radiative power exhaust, while limiting the increment of core $Z_\mathrm{eff}$ to acceptable values.

The potential of low-Z powders as near-target radiators has been explored with dedicated DIII-D experiments, where Li, B and BN powders have been injected in the Small Angle Slot (SAS) divertor (Guo et al. 2017) during H-mode plasmas (Effenberg et al. 2021). There, the unique experimental configuration, with powder injection port vertically aligned with the SAS, allowed powder deposition directly at the outer strike point. The data set demonstrated enhanced divertor dissipation with a rapid reduction of divertor electron temperature and increase of neutral pressure for the three injected species, to a variable degree scaling with the powder injection rate, with negligible or tolerable effect on the global plasma energy confinement. The most significant effect was observed with injection of BN at rates larger than 60 mg/s, which resulted in substantial reduction of heat flux (from $\approx 40$ to $<2$ MW/m$^2$) and more than one order of magnitude increase in neutral compression (ration between strike-point and main chamber pressure) indicating an transition to detachment, robustly maintained over the duration of powder injection (Effenberg et al. 2021, 2022).

The aforementioned injection of BN in AUG, H-mode plasmas, in addition to improving the pedestal stability, showed significant increase of radiative losses, up to 80% of the injected heating power (Bortolon et al. 2018). The injection of the radiator into the plasma crown leads to significant differences in the spatial structure of the radiative losses compared with the typical N$_2$ injection in the divertor region Lunsford et al. (2019), with a less pronounced increase of divertor radiation (up to 30%) combined with a radiative mantle surrounding the main plasma boundary. Interestingly, the performance improvement was obtain with a 10-50 fold lower production of ammonia, compared to reference cases with N$_2$ injection. While the processes leading to this result remains unclear, a lower ammonia production is attractive for next-step fusion devices because of the need to minimize the amounts of tritiated ammonia formed in N$_2$ ratiative divertors (Drenik et al. 2019).

4 Available simulation tools and some results of their applications

Given the complex multidisciplinary physics associated with dust in fusion devices, an accurate description of dust–plasma and dust–vessel interactions or a comparison with experimental data is only viable numerically. Dust dynamics codes (Smirnov et al. 2007b; Martin et al. 2008; Vignitchouk et al. 2014; Gervasini et al. 2017; Autricque et al. 2017a; Delzanno and Tang 2014b; Brochard et al. 2019) solve a set of coupled ordinary differential equations that describe charging, heating, mass loss and momentum exchange. A dust grain or a number of independent isolated dust grains, treated as spherical point particles without an internal structure, are injected into a known plasma background with an initial speed and size selected from given distributions. The plasma profiles are constructed from experimental input or obtained with the aid of edge plasma transport codes such as the UEDGE (Rognlien et al. 1992), the EDGE2D (Simonini et al. 1994), the EMC3 Feng et al. (2002) and different versions of the SOLPS code (Wiesen et al. 2015) as well as the DINA code (Khayrutdinov and Lukash 1993) for disruption scenarios. Since dust dynamics codes solve essentially the same system of equations, the sole and major differences lie in the physics models employed for calculations of their source terms—particle and heat fluxes as well as forces. As pointed earlier (Ratynskaia et al. 2022), this fact limits the value of direct comparisons between codes and a better assessment of the impact of each physical process can be obtained by varying expressions for selected source term within a single code (Vignitchouk et al. 2017, 2018a; Ratynskaia et al. 2013).

Early dust–plasma interaction description, adopted in all codes starting from the pioneering DUSTT (Smirnov et al. 2007b), was based on the OML framework that is suitable for small, $R_{\mathrm {d}}\ll L_{\mathrm {De}}, R_{\mathrm {Le}}$ particles. As discussed in Sect. 2, for the majority of dust/droplet populations, the regime of relevance is characterized by the opposite ordering, thus invalidating the OML application. To account for larger sizes, different ion current descriptions have been introduced in MIGRAINe (Vignitchouk et al. 2017) and DTOKS (Simons et al. 2021). Note that for W particles close to the melting point, the thermionic electron flux dominates the ion flux, thus minimizing the impact of the ion current on the dust life time. The magnetized nature of electron collection is a more crucial aspect of OML violation, see Sect. 2, and has been addressed in two codes. MIGRAINe employs analytical electron collection models for the $R_{\mathrm {d}}\gg R_{\mathrm {Le}}, L_{\mathrm {De}}$ regime discussed in Section 2, where electron magnetization leads to drastic suppression of the electron particle and heat flux (Vignitchouk et al. 2017, 2018c). DTOKS has been coupled to the Monte-Carlo code DiMPl Simons and Coppins (2020) which tracks plasma particles in a prescribed electric potential (i.e. neglecting space-charge effects), an approach valid for $R_{\mathrm {d}} < L_{\mathrm {De}}$ and thus only for very small dust given the ${T_\mathrm{e}, n_\mathrm{e}}$ ranges of interest. Another important aspect of heat balance, the effect of the ablation cloud, has been incorporated in DUSTT (Smirnov and Krasheninnikov 2020) through tabulating predictions of the models of Refs. Marenkov and Krasheninnikov (2014); Krasheninnikov and Marenkov (2015).

Being focused on dust studies in tokamaks, DUSTT, DTOKS, and MIGRAINE assume a toroidal symmetry of the plasma parameters, which are needed for the description of the dust–plasma interactions. However, the DUSTT code was also adopted for the stellarator configuration, to study transport of dust (Shoji et al. 2015) and more recently of B powder injected for wall conditioning (Shoji et al. 2020).

The core objectives of predictive reactor studies concern the identification of dust accumulation sites as well as their evolution during machine operation and the impact of ablating dust onto plasma profiles. Below we exemplify ITER-relevant studies as carried out by the codes specifically developed for such purposes.

Droplet-to-dust conversion and evolution of dust inventory The MIGRAINe code development has been guided by the need to simulate droplet survival during transient events, droplet conversion rates to solid dust and dust inventory evolution in the course of normal discharges. In this light, the prime focus of the MIGRAINe model has been surface processes and particle collection in environments with high-Z charged ions, accounting for electron current suppression by strong magnetic fields as well as the description of dust-wall collisions (Vignitchouk et al. 2017, 2018c). The cooling of Be droplets that are produced by first wall surface melting after ITER MDs and VDEs with delayed mitigation were simulated by the MIGRAINe code in Refs. (Vignitchouk et al. 2017, 2018c), providing detailed estimates of droplet solidification into dust and their subsequent deposition around the vessel. Droplets were injected from the first wall location receiving the highest heat load during the thermal quench into time-dependent plasma profiles evaluated by the DINA code. The results showed that only a few percent of the initially injected mass is converted into solid dust, while the remaining mass either vaporizes or forms liquid splashes on the wall. For the surviving dust, simulated in-vessel spatial distributions and accumulation in the diagnostics ports provide guidance for planned dust diagnostic, retrieval and clean-up systems on ITER. As an example, the spatial distribution of the average radius of solid dust over the ITER poloidal cross-section after the MD and VDE scenarios (Vignitchouk et al. 2018c) is shown in Fig. 17. The dust size and final location sites reflect the discussion on mechanical impacts of Sect. 2.3.2.

Fig. 17

Reproduced from Vignitchouk et al. (2018c) with the permission of IAEA Publishing

Spatial distribution of the average radius of solid dust over the ITER poloidal cross-section after MD and VDE scenarios simulated in Vignitchouk et al. (2018c). The key features of the distribution are labelled with capital letters and highlighted on the cross-section shown in the insert.

The dust survival rates and the characteristic decay times of in-vessel inventory in ITER relevant ramp-up and steady-state plasmas have also been recently assessed (Vignitchouk et al. 2022a). MIGRAINe simulations have been performed for ITER with low-power SOLPS-ITER profiles as a proxy for plasma ramp-up or early discharges. The selection of sizes and speeds of dust mobilized from sites in the divertor region (inner leg, outer leg and dome) has been guided by semi-empirical scalings discussed in Sect. 2.2.2. Starting from an initial particle population, MIGRAINe simulation results were iterated to yield an estimation of dust inventory evolution over multiple discharges in the absence of new dust production. The analysis shows that the in-vessel inventory follows simple exponential models which are useful for quick estimates and eventual integration into more advanced models that include dust production.

Dust—plasma profile feedback Aiming for a self-consistent modeling of dust impact on edge plasma parameters and the corresponding feedback, DUSTT has been coupled to the UEDGE plasma transport code. The DUSTT modeling possibilities include both steady-state and time-dependent dust injection into tokamak plasmas (Smirnov et al. 2011; Smirnov and Krasheninnikov 2020). The simulations of steady-state injection facilitate the assessment of important reactor issues such as divertor plasma detachment (Smirnov et al. 2011) and in-situ wall conditioning with the dust/powder (see Sect. 3). The time-dependent injection into tokamak plasma allows to follow the evolution of dust and its ablation associated impurities as well as evolution of all edge plasma parameters.

The results of the simulations of a sudden release of W dust into the outer divertor of an ITER-like plasma reveal that the main impact is caused by the amount of the injected dust, whereas the dust size, overall, does not play a significant role (Smirnov and Krasheninnikov 2020). However, simulations demonstrate a complex and sometimes counter-intuitive time evolution of plasma parameters and divertor heat loads on the outer and inner divertor targets. As seen from Fig. 18, the evolution of plasma parameters, following the injection of W dust, results in opposite behavior of the peak heat loads on outer and inner targets. Moreover, the peak heat loads on the targets can reach values well above the nominal ones. Such evolution is due to plasma density redistribution between inner and outer divertors.

Fig. 18

Reproduced from Smirnov and Krasheninnikov (2020), with the permission of AIP Publishing

Time evolution of a peak heat loads in outer (left) and inner (right) divertors for different amounts and different sizes of injected dust.

Simple estimates show that an increase of the peak load to $\sim 30$ MW/m$^2$ for $\sim 0.1$ s causes the rise of surface temperature of W divertor target by $\sim 600$ K. As a result, it can lead to the recrystallization W layers adjacent to the surface. In addition, the estimates, based on the results of Ref. Smirnov and Krasheninnikov (2020), show that the W fraction in the core plasma could exceed $\sim 10^{-4}$ for injected (in the vicinity of the outer strike point) W mass above 10 mg, which might result in a catastrophic cooling of the core plasma (Pütterich et al. 2019). We note that 10 mg of tungsten corresponds to a spherical dust grain with the radius of 1 mm.

We recall that all the coupled dust-edge plasma simulations still assume toroidal symmetry for all plasma and dust parameters. In practice, accidental dust injection is localized in the poloidal and the toroidal directions similar to the powder injection shown in Fig. 11. Therefore, for realistic dust injection, one should anticipate a rather complex 3D variation of plasma parameters, resembling the structure of plasma parameters following the pellet injection triggering ELMs, see Refs. (Pégourié 2007; Lang et al. 2014; Futatani et al. 2014) and the references therein. As an example, Fig. 19 presents the 3D structure of plasma pressure found from the numerical modeling of pellet injection into the DIII-D tokamak (Futatani et al. 2014). We point out that the formation of 3D structures during pellet injection was envisioned well before such complex nonlinear simulations become possible, see Pégourié (2007) and the references therein. It is well understood that toroidal symmetry breaking results in plasma polarization accompanied by the complex $E \times B$ plasma flows. Therefore, the results of dust injection into the tokamak plasma with the DUSTT-UEDGE package, performed with the assumption of toroidal symmetry, can be considered only as an approximate assessment of the impact of dust on overall plasma performance.

Fig. 19

3D structure of plasma pressure formed after pellet injection. Reproduced with permission from Futatani et al. (2014), $\copyright$ IAEA 2014

5 Discussion and outlook

The transition to all-metal first wall devices led to a large reduction of the amount of dust in magnetic fusion experiments. However, since high-Z tungsten is now used as the material of PFCs, the possible impact of tungsten dust on the performance of the whole fusion device could be still devastating. Moreover, in-vessel dust inventory, subject to nuclear licensing in future reactors, would need to be assessed in the conditions of reactor plasmas. As a result, the main foci of post-carbon era dust studies have shifted toward questions of how metallic dust is formed, released into the plasma volume and remobilized from material PFCs in the subsequent discharges. Furthermore, the self-consistent modeling of the impact of metallic dust on the performance of fusion devices with coupled 2D edge plasma transport and dust transport codes has been achieved. In addition, in-situ wall conditioning, ELM suppression, and the stimulation of divertor plasma detachment with the dust (powder) injection have been initiated essentially on all major magnetic fusion devices.

Capitalizing on recent advances in the field, one can aim to obtain quantitative predictions of the key figures of merit related to operational safety concern to the ITER project and conceptual designs of fusion power plants. For the assessment of the dust inventory evolution, release conditions are a primary concern. There is some confidence in the sizes of freshly released droplets, that are related to the melt thickness, as well as the remobilization size-thresholds for pre-existing dust, but information on the release velocities associated with these events is much more limited. The release of dust due to delamination and cracking is also never observed directly in tokamaks and no speed data are available. Furthermore, as far as the production of dust and droplets from PFC interactions with runaway electrons is concerned, there are scarce empirical data while no numerical tools exist as of yet that are capable of modelling RE-induced compressive flows and shocks. Another aspect that is crucial for the predictive power of modelling of the dust inventory in reactors is the correlation between the chosen dust release mechanism and plasma profiles. Droplets are formed due to PFC melting under transients and thus are to be injected in MD or VDE plasmas - in contrast to remobilization of pre-existing solid dust which should be lifted up during discharge start-up. While transient melt events potentially causing droplet ejection, such as ELMs or disruptions, eventually will be controlled and/or suppressed, arcing represents a dust source which cannot be mitigated. It is not feasible to predict the number of arcing events per discharge in a whole reactor realistically and, therefore, to accurately assess the amount of dust produced through this channel. On the other hand, once can anticipate that arcing-related micrometer dust will essentially be non-remobilizable due to very strong adhesion in such size range. If droplets released from cathode-spot melt pools are ejected towards the plasma, their contribution to impurities should be less significant compared to other dust due to their small dimensions. In addition, in view of the future use of stainless steel in ITER  (Pitts et al. 2015) and DEMO  (Roth et al. 2014) and the expectation of significant stainless steel dust production in ITER, the issue of magnetic dust, which is remobilized by the magnetic fields and can interfere with the plasma break-down, deserves further attention.

The application of state-of-the-art experimental techniques and simulation tools is a necessary step to advance the science of powder injection for fusion applications. The recent implementation of powder dropper systems in a variety of present fusion devices has yielded promising results in several key areas for the safe and robust operation of a fusion reactor, such as wall conditioning, ELM suppression and the stimulation of divertor plasma detachment. However, open questions remain on the scalability of these techniques to larger devices, in particular with regard to the required throughput of injected powder vis-à-vis the amounts of dust tolerable in a fusion device. This motivates an urgent need for model validation of present day experiments. In this context, 3D edge plasma codes, coupled to modified dust tracking codes, which are no more relying on the toroidal symmetry of plasma parameters, should replace current 2D plasma-dust packages as, e.g., those discussed in Sect. 4. Such development is needed to address an important issue of the breaking of toroidal symmetry in the course of both poloidally and toroidally localized dust injection. Plausible approach would be the adaptation of available MHD or edge plasma turbulence codes and their coupling with dust codes.