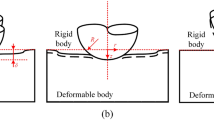
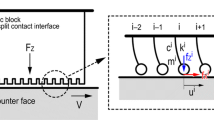
Abstract
Adhesion is one of essences with respect to rubber friction because the magnitude of the friction force is closely related to the magnitude of adhesion on a real contact area. However, the real contact area during sliding depends on the state and history of the contact surface. Therefore, the friction force occasionally exhibits rate-, state-, and pressure dependency. In this study, to rationally describe friction and simulate boundary value problems, a rate-, state-, and pressure-dependent friction model based on the elastoplastic theory was formulated. First, the evolution law for the friction coefficient was prescribed. Next, a nonlinear sliding surface (frictional criterion) was adopted, and several other evolution laws for internal state variables were prescribed. Subsequently, the typical response characteristics of the proposed friction model were demonstrated, and its validity was verified by comparing the obtained results with those of experiments conducted considering the contact surface between a rough rubber hemisphere and smooth acrylic plate.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Bowden F P, Tabor D. The Friction and Lubrication of Solids. Oxford (UK): Oxford University Press, 1950.
Oden J T, Martins J A C. Models and computational methods for dynamic friction phenomena. Comput Meths Appl Mech Eng52(1–3): 527–634 (1986)
Scholz C H. The Mechanics of Earthquakes and Faulting. 2nd ed. Cambridge (UK): Cambridge University Press, 2002.
Baumberger T, Heslot F, Perrin B. Crossover from creep to inertial motion in friction dynamics. Nature367(6463): 544–546 (1994)
Rubinstein S M, Cohen G, Fineberg J. Detachment fronts and the onset of dynamic friction. Nature430(7003): 1005–1009 (2004)
Baumberger T, Caroli C. Solid friction from stick-slip down to pinning and aging. Adv Phys55(3-4): 279–348 (2006)
Ben-David O, Fineberg J. Static friction coefficient is not a material constant. Phys Rev Lett106(25) 254301 (2011
Maegawa S, Suzuki A, Nakano K. Precursors of global slip in a longitudinal line contact under non-uniform normal loading. Tribol Lett38(3): 313–323 (2010)
Ozaki S, Inanobe C, Nakano K. Finite element analysis of precursors to macroscopic stick-slip motion in elastic materials: Analysis of friction test as a boundary value problem. Tribol Lett55(1): 151–163 (2014)
Dieterich J H. Time-dependent friction in rocks. J Geophys Res77(20): 3690–3697 (1972)
Dieterich J H. Modeling of rock friction: 1. Experimental results and constitutive equations. J Geophys Res Solid Earth84(B5): 2161–2168 (1979)
Ruina A. Slip instability and state variable friction laws. J Geophys Res Solid Earth88(B12): 10359–10370 (1983)
Rice J R, Ruina A L. Stability of steady frictional slipping. J Appl Mech50(2): 343–349 (1983)
Dieterich J H, Kilgore B D. Direct observation of frictional contacts: New insights for state-dependent properties. Pure Appl Geophys143(1-3): 283–302 (1994)
Popov V L, Grzemba B, Starcevic J, Popov M. Rate and state dependent friction laws and the prediction of earthquakes: What can we learn from laboratory models? Tectonophysics532-d535: 291–300 (2012)
Grzemba B. New experimental investigations on the Dieterich-Ruina friction law. Facta Univ, Ser: Mech Eng13(1): 11–20 (2015)
Kikuchi N, Oden J T. Contact Problems in Elasticity: A Study of Variational Inequalities and Finite Element Methods. Philadelphia (USA): SIAM, 1988.
Laursen T A. Computational Contact and Impact Mechanics: Fundamentals of Modeling Interfacial Phenomena in Nonlinear Finite Element Analysis. New York (USA): Springer-Verlag Berlin Heidelberg, 2003.
Wriggers P. Computational Contact Mechanics. Hoboken (USA): John Wiley, 2002.
Hashiguchi K, Ozaki S. Constitutive equation for friction with transition from static to kinetic friction and recovery of static friction. Int J Plast24(11): 2102–2124 (2008)
Ozaki S, Hashiguchi, K. Numerical analysis of stick-slip instability by a rate-dependent elastoplastic formulation for friction. Tribol Int43(11): 2120–2133 (2010)
Ozaki S, Ito C, Hashiguchi K. Experimental verification of rate-dependent elastoplastic analogy friction model and its application to FE analysis. Tribol Int64: 164–177 (2013)
Ozaki S, Hikida K, Hashiguchi K. Elastoplastic formulation for friction with orthotropic anisotropy and rotational hardening. Int J Solids Struct49(3-4): 648–657 (2012)
Johnson K L, Greenwood J A, Higginson J G. The contact of elastic regular wavy surfaces. Int J Mech Sci27(6): 383–396 (1985)
Manners W. Pressure required to flatten an elastic random rough profile. Int J Mech Sci42(12): 2321–2336 (2000)
Hyun S, Pei L, Molinari J F, Robbins M O. Finite-element analysis of contact between elastic self-affine surfaces. Phys Rev E70(2): 026117 (2004)
Persson P N J, Bucher F, Chiaia B. Elastic contact between randomly rough surfaces: Comparison of theory with numerical results. Phys Rev B65(18) 184106 (2002)
Yang C, Persson P N J. Contact mechanics: Contact area and interfacial separation from small contact to full contact. J Phys: Condens Matter20(21-21) 5214 (2008)
Maegawa S, Itoigawa F, Nakamura T. Effect of normal load on friction coefficient for sliding contact between rough rubber surface and rigid smooth plane. Tribol Int92: 335–343 (2015)
Ostermeyer G P. On the dynamics of the friction coefficient. Wear254(9): 852–858 (2003)
Lorenz B, Persson B N J, Dieluweit S, Tada T. Rubber friction: Comparison of theory with experiment. Eur Phys J E34(12) 129 (2011)
Hashiguchi K. Foundations of Elastoplasticity: Subloading Surface Model. Cham (Germany): Springer, 2017.
Popov V L, Heß M. Method of Dimensionality Reduction in Contact Mechanics and Friction. Berlin (Germany): Springer, 2015.
Teidelt E. Oscillating contact: Friction induced motion and control of friction. Ph.D. Thesis. Berlin (Germany): Technical University of Berlin, 2015.
Nguyen H X, Teidelt E, Popov V L, Fatikow S. Modeling and waveform optimization of stick-slip micro-drives using the method of dimensionality reduction. Arch Appl Mech86(10): 1771–1785 (2016)
Bureau L, Baumberger T, Caroli C. Rheological aging and rejuvenation in solid friction contacts. Eur Phys J E8(3): 331–337 (2002)
Johnson K L. Contact Mechanics. Cambridge (UK): Cambridge University Press, 2003.
Maegawa S, Nakano K. Dynamic behaviors of contact surfaces in the sliding friction of a soft material. J Adv Mech Des, Syst, Manuf1(4): 553–561 (2007)
Mark J E. Polymer Data Handbook. Oxford (UK): Oxford University Press, 1999.
Author information
Authors and Affiliations
Corresponding author
Additional information
Shingo Ozaki. He received his Ph.D. degree from Kyushu University, Japan, in 2005. After that he worked as an assistant professor at Department of Mechanical Engineering in Tokyo University of Science, Japan. His current position is an associate professor at Department of Mechanical Engineering in Yokohama National University, Japan. His research area covers the tribology and computational mechanics of solids.
Satoru Maegawa. He received his Ph.D. degree in mechanical engineering from Yokohama National University, Japan, in 2012. His current position is an associate professor at Department of Electrical and Mechanical Engineering, Nagoya Institute of Technology, Japan. His research area covers the tribology of soft materials, the control of friction-induced vibration, and laser-manufacturing technologies.
Rights and permissions
Open Access: This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made.
The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder.
To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Ozaki, S., Matsuura, T. & Maegawa, S. Rate-, state-, and pressure-dependent friction model based on the elastoplastic theory. Friction 8, 768–783 (2020). https://doi.org/10.1007/s40544-019-0321-3
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40544-019-0321-3