Skip to main content
SpringerLink
Log in

A Legendre spectral-finite difference method for Caputo–Fabrizio time-fractional distributed-order diffusion equation

  • Original Research
  • Published:
Mathematical Sciences Aims and scope Submit manuscript

Abstract

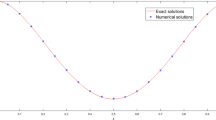
In this paper, we introduce a hybrid method based on a finite difference method and a spectral method for solving the multi-term time-fractional diffusion equations (TFDEs) based on Caputo–Fabrizio fractional operator. We apply a finite difference scheme for discretizing the time derivatives and consider a Legendre-spectral approximation in space discretization to semi-discrete problem. It is known that the spectral method has been an efficient tool for computing numerical solutions of differential equations because of its high-order accuracy. We discuss the convergence of the proposed method in discrete \(L^{2}\)-norm. Furthermore, we extend the multi-term TFDE to the distributed order and analyze the method for the considered equation. In the end, we confirm the proven theoretical results with the help of some numerical examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Machado, J.A.T., Kiryakova, V.: Recent history of the fractional calculus: data and statistics. Basic Theory 1–22 (2019)

  2. Hifer, R.: Application of Fractional Calculus in Physics. World Scientific, Singapore (2000)

    Book  Google Scholar 

  3. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam (2006)

    MATH  Google Scholar 

  4. Diethelm, K.: The Analysis of Fractional Differential Equations. Springer, Berlin (2010)

    Book  MATH  Google Scholar 

  5. Ortigueira, M.D.: Fractional Calculus for Scientists and Engineer: Lecture Notes in Electrical Engineering. Springer, Berlin (2011)

    Book  Google Scholar 

  6. Oldham, K.B., Spanier, J.: The Fractional Calculus. Academic Press, New York (1974)

    MATH  Google Scholar 

  7. Podlubny, I.: Fractional Differential Equations. Academic Press, San Diego (1999)

    MATH  Google Scholar 

  8. Metzler, R., Klafter, J.: The random walks guide to anomalous diffusion: a fractional dynamics approach. Phys. Rep. 339(1), 1–77 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  9. Hao, Z., Cao, W.: An improved algorithm based on finite difference schemes for fractional boundary value problems with nonsmooth solution. J. Sci. Comput. 73(1), 395–415 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  10. Hao, Z., Sun, Z., Cao, W.: A fourth-order approximation of fractional derivatives with its applications. J. Comput. Phys. 281, 787–805 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  11. Meerschaert, M.M., Tadjeran, C.: Finite difference approximations for fractional advection-dispersion flow equations. J. Comput. Appl. Math. 172(1), 65–77 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  12. Tian, W., Zhou, H., Deng, W.: A class of second order difference approximations for solving space fractional diffusion equations. Math. Comput. 84(294), 1703–1727 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  13. Wang, H., Basu, T.S.: A fast finite difference method for two-dimensional space-fractional diffusion equations. SIAM J. Sci. Comput. 34(5), A2444–A2458 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  14. Zhao, J., Fang, Z., Li, H., Liu, Y.: A Crank-Nicolson finite volume element method for time fractional Sobolev equations on triangular grids. Mathematics 8(9), 1591 (2020)

    Article  Google Scholar 

  15. Zhao, Z., Zheng, Y., Guo, P.: A Galerkin finite element method for a class of time-space fractional differential equation with nonsmooth data. J. Sci. Comput. 70(1), 386–406 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  16. Yin, B., Liu, Y., Li, H.: A class of shifted high-order numerical methods for the fractional mobile/immobile transport equations. Appl. Math. Comput. 368, 124799 124799 (2020)

    MathSciNet  MATH  Google Scholar 

  17. Hao, Z., Park, M., Lin, G., Cai, Z.: Finite element method for two-sided fractional differential equations with variable coefficients: Galerkin approach. J. Sci. Comput. 79(2), 700–717 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  18. Wang, H., Yang, D.: Wellposedness of variable-coefficient conservative fractional elliptic differential equations. SIAM J. Numer. Anal. 51(2), 1088–1107 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  19. Wen, C., Liu, Y., Yin, B., Li, H., Wang, J.: Fast second-order time two-mesh mixed finite element method for a nonlinear distributed-order sub-diffusion model. Numer. Algorithms (2021). https://doi.org/10.1007/s11075-020-01048-8

    Article  MathSciNet  MATH  Google Scholar 

  20. Zeng, F., Mao, Z., Karniadakis, G.E.: A generalized spectral collocation method with tunable accuracy for fractional differential equations with end-point singularities. SIAM J. Sci. Comput. 39(1), A360 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  21. Zhang, Z., Zeng, F., Karniadakis, G.E.: Optimal error estimates of spectral Petrov-Galerkin and collocation methods for initial value problems of fractional differential equations. SIAM J. Numer. Anal. 53(4), 2074–2096 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  22. Ervin, V.J., Heuer, N., Roop, J.P.: Regularity of the solution to 1-D fractional order diffusion equations. Math. Comput. 87(313), 2273–2294 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  23. Huang, C., Jiao, Y., Wang, L., Zhang, Z.: Optimal fractional integration preconditioning and error analysis of fractional collocation method using nodal generalized Jacobi functions. SIAM J. Numer. Anal. 54(6), 3357–3387 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  24. Xu, X.L.: Existence and uniqueness of the weak solution of the space-time fractional diffusion equation and a spectral method approximation. Commun. Comput. Phys. 8(5), 1016–1051 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  25. Mao, Z., Chen, S., Shen, J.: Efficient and accurate spectral method using generalized Jacobi functions for solving Riesz fractional differential equations. Appl. Numer. Math. 106, 165–181 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  26. Xu, Q., Hesthaven, J.S.: Discontinuous Galerkin method for fractional convection–diffusion equations. SIAM J. Numer. Anal. 52(1), 405–423 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  27. Simmons, A., Yang, Q., Moroney, T.: A finite volume method for two-sided fractional diffusion equations on non-uniform meshes. J. Comput. Phys. 335, 747–759 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  28. Zhao, J., Fang, Z., Li, H., Liu, Y.: Finite volume element method with the WSGD formula for nonlinear fractional mobile/immobile transport equations. Adv. Differ. Equ. 2020(1), 1–20 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  29. Caputo, M.: Distributed order differential equations modelling dielectric induction and diffusion. Fract. Calc. Appl. Anal. 4, 421–442 (2001)

    MathSciNet  MATH  Google Scholar 

  30. Jiao, Z., Chen, Y., Podlubny, I.: Distributed-Order Dynamic Systems. Springer, Berlin (2012)

    Book  MATH  Google Scholar 

  31. Chechkin, A.V., Gorenflo, R., Sokolov, I.M.: Retarding subdiffusion and accelerating superdiffusion governed by distributed-order fractional diffusion equations. Phys. Rev. E 66, 046129 (2002)

    Article  Google Scholar 

  32. Kochubei, A.: Distributed order calculus and equations of ultraslow diffusion. J. Math. Anal. Appl. 340, 252–281 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  33. Mark, M., Nane, E.: Distributed-order fractional diffusions on bounded domains. J. Math. Anal. Appl. 379, 216–228 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  34. Eab, C.H., Lim, S.C.: Fractional Langevin equations of distributed order. Phys. Rev. E 83, 031136 (2011)

    Article  MathSciNet  Google Scholar 

  35. Katsikadelis, J.T.: Numerical solution of distributed order fractional differential equations. J. Comput. Phys. 259, 11–22 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  36. Ye, H., Liu, F., Anh, V., Turner, I.: Numerical analysis for the time distributed-order and Riesz space fractional diffusions on bounded domains. Int. J. Appl. Math. 80, 825–838 (2015)

    MathSciNet  MATH  Google Scholar 

  37. Morgado, M.L., Rebelo, M.: Numerical approximation of distributed order reaction–diffusion equations. J. Comput. Appl. Math. 275, 216–227 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  38. Gao, G.H., Sun, Z.Z.: Two unconditionally stable and convergent difference schemes with the extrapolation method for the one-dimensional distributed-order differential equations. Numer. Methods Partial Differ. Equ. 32, 591–615 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  39. Gao, G.H., Sun, H.W., Sun, Z.Z.: Some high-order difference schemes for the distributed-order differential equations. J. Comput. Phys. 298(1), 337–359 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  40. Bu, W., Xiao, A., Zeng, W.: Finite difference/finite element methods for distributed-order time fractional diffusion equations. J. Sci. Comput. 72(1), 422 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  41. Li, X., Wu, B.: A numerical method for solving distributed order diffusion equations. Appl. Math. Lett. 53, 92–99 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  42. Yin, B., Liu, Y., Li, H., Zhang, Z.: Approximation methods for the distributed order calculus using the convolution quadrature. Discrete Contin. Dyn. Syst. B 26(3), 1447–1468 (2021)

    MathSciNet  MATH  Google Scholar 

  43. Lin, Y., Li, X., Xu, C.: Finite difference/spectral approximations for the fractional cable equation. Math. Comput. 80(275), 1369–1396 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  44. Lin, Y., Xu, C.: Finite difference/spectral approximations for the time-fractional diffusion equation. J. Comput. Phys. 225(2), 1533–1552 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  45. Caputo, M., Fabrizio, M.: A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 1(2), 1–13 (2015)

    Google Scholar 

  46. Losada, J., Nieto, J.J.: Properties of a new fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 1(2), 87–92 (2015)

    Google Scholar 

  47. Caputo, M., Fabrizio, M.: Applications of new time and spatial fractional derivatives with exponential kernels. Prog. Fract. Differ. Appl. 2(1), 1–11 (2016)

    Article  Google Scholar 

  48. Alkahtani, B., Atangana, A.: Controlling the wave movement on the surface of shallow water with the Caputo–Fabrizio derivative with fractional order. Chaos Solitons Fractals 89, 539–546 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  49. Singh, J., Kumar, D., Qurashi, M.A., Baleanu, D.: A new fractional model for giving up smoking dynamics. Adv. Differ. Equ. 2017(1), 1–16 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  50. Gómez-Aguilar, J., López-López, M., Alvarado-Martínez, V., Baleanu, D., Khan, H.: Chaos in a cancer model via fractional derivatives with exponential decay and Mittag–Leffler law. Entropy 19(12), 681 (2017)

    Article  MathSciNet  Google Scholar 

  51. Singh, J., Kumar, D., Hammouch, Z., Atangana, A.: A fractional epidemiological model for computer viruses pertaining to a new fractional derivative. Appl. Math. Comput. 316, 504–515 (2018)

    MathSciNet  MATH  Google Scholar 

  52. Al-Khedhairi, A.: Dynamical analysis and chaos synchronization of a fractional-order novel financial model based on Caputo–Fabrizio derivative. Eur. Phys. J. Plus 134(10), 532 (2019)

    Article  Google Scholar 

  53. Dokuyucu, M.A., Celik, E., Bulut, H., Baskonus, H.M.: Cancer treatment model with the Caputo–Fabrizio fractional derivative. Eur. Phys. J. Plus 133(3), 1–6 (2018)

    Google Scholar 

  54. Bushnaq, S., Khan, S.A., Shah, K., Zaman, G.: Mathematical analysis of HIV/AIDS infection model with Caputo–Fabrizio fractional derivative. Cogent Math. Stat. 5(1), 1432521 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  55. Dubey, R., Baleanu, D., Mishra, M., Goswami, P.: Solution of modified Bergmans minimal blood glucose insulin model using Caputo–Fabrizio fractional derivative. https://doi.org/10.22541/au.159446913.31343500 (2020)

  56. Baleanu, D., Jajarmi, A., Mohammadi, H., Rezapour, S.: A new study on the mathematical modelling of human liver with Caputo–Fabrizio fractional derivative. Chaos Solitons Fractals 134, 109705 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  57. Qureshi, S., Yusuf, A.: Fractional derivatives applied to MSEIR problems: comparative study with real world data. Eur. Phys. J. Plus 134(4), 171 (2019)

    Article  Google Scholar 

  58. Atanackovic, T.M.: A generalized model for the uniaxial isothermal deformation of a viscoelastic body. Acta Mech. 159(1–4), 77–86 (2002)

    Article  MATH  Google Scholar 

  59. Atanackovic, T.M., Budincevic, M., Pilipovic, S.: On a fractional distributed-order oscillator. J. Phys. A Math. Gen. 38(30), 6703–6713 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  60. Atanackovic, T.M., Pilipovic, S., Zorica, D.: Time distributed-order diffusion-wave equation. II. Applications of Laplace and Fourier transformations. Proc. R. Soc. A Math. Phys. Eng. Sci. 465(2106), 1893–1917 (2009)

    MathSciNet  MATH  Google Scholar 

  61. Atanackovic, T.M., Pilipovic, S., Zorica, D.: Distributed-order fractional wave equation on a finite domain: creep and forced oscillations of a rod. Contin. Mech. Thermodyn. 23(4), 305–318 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  62. Diethelm, K., Ford, N.J.: Numerical analysis for distributed-order differential equations. J. Comput. Appl. Math. 225(1), 96–104 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  63. Atangana, A., Nieto, J.J.: Numerical solution for the model of RLC circuit via the fractional derivative without singular kernel. Adv. Mech. Eng. 7(10), 1687814015613758 (2015)

    Article  Google Scholar 

  64. Qureshi, S., Yusuf, A., Shaikh, A.A., Inc, M., Baleanu, D.: Fractional modeling of blood ethanol concentration system with real data application. Chaos Interdiscip. J. Nonlinear Sci. 29(1), 013143 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  65. Qureshi, S., Atangana, A.: Mathematical analysis of dengue fever outbreak by novel fractional operators with field data. Phys. A Stat. Mech. Appl. 526, 121127 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  66. Atangana, A., Qureshi, S.: Modeling attractors of chaotic dynamical systems with fractal-fractional operators. Chaos Solitons Fractals 123, 320–337 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  67. Qureshi, S., Yusuf, A.: Modeling chickenpox disease with fractional derivatives: from caputo to atangana-baleanu. Chaos Solitons Fractals 122, 111–118 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  68. Liu, Z.G., Cheng, A.J., Li, X.L.: A second finite difference scheme for quasilinear time fractional parabolic equation based on new fractional derivative. Int. J. Comput. Math. 95, 396–411 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  69. Bernardi, C., Maday, Y.: Approximations spectrales de problems aux limites elliptiques. Springer, Berlin (1992)

    MATH  Google Scholar 

  70. Tomovski, Ž, Sandev, T.: Distributed-order wave equations with composite time fractional derivative. Int. J. Comput. Math. 95(6–7), 1100–1113 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  71. Luchko, Y.: Boundary value problems for the generalized time-fractional diffusion equation of distributed order. Fract. Calc. Appl. Anal. 12(4), 409–422 (2009)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, Faculty of Mathematical Science, Shahrekord University, P. O. Box 115, Shahrekord, Iran

    M. Fardi & J. Alidousti

Authors
  1. M. Fardi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. J. Alidousti
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. Fardi.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fardi, M., Alidousti, J. A Legendre spectral-finite difference method for Caputo–Fabrizio time-fractional distributed-order diffusion equation. Math Sci 16, 417–430 (2022). https://doi.org/10.1007/s40096-021-00430-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40096-021-00430-4

Keywords

Search

Navigation