A note about rational surfaces as unions of affine planes

Abstract

We prove that any smooth rational projective surface over the field of complex numbers has an open covering consisting of 3 subsets isomorphic to affine planes.

Since all smooth rational curves are isomorphic to ${\mathbb {P}}^1$, they can be seen as the union of two affine lines. In dimension two, as a consequence of the structure Theorem 1.3 below, all rational surfaces admit a covering of open subsets isomorphic to the affine plane. However, up to the authors’ knowledge, no general results are known on the minimal number of open subsets of such a covering, while some advances are known by computer algebrists in terms of surjectivity of parametrizations [1, 5, 6, 8]. In this short note we prove that all projective smooth rational surfaces behave like the projective plane in this aspect.

1 Main result

Theorem 1.1

Let X be a projective smooth rational surface over the complex field. Then, there are three open subsets $U_0,U_1,U_2\subset X$ such that:

• (1) $U_0\cup U_1\cup U_2 =X$.

• (2) For all $i=0,1,2$, $U_i$ is isomorphic to the affine plane.

Remark 1.2

Note that the bound of three subsets in the covering is sharp. If the projective surface $X\subset {\mathbb {P}}^n$ is the union of two affine planes $U_0$ and $U_1$, then $Z=X-U_0$ is closed in X, so projective, and it is contained in $U_1\simeq {\mathbb {A}}^2$, so it must be finite. Since Z is finite, there is a hyperplane $H\subset {\mathbb {P}}^n-Z$. Then the section $H\cap X$ is a projective curve contained in $X-Z=U_0\simeq {\mathbb {A}}^2$. Since ${\mathbb {A}}^2$ does not contain projective varieties of positive dimension, this is a contradiction.

To prove Theorem 1.1, we will use the following well-known result:

Theorem 1.3

(see e.g. [2, Theorem V.10]) Every non-singular rational surface can be obtained by repeatedly blowing up either ${\mathbb {P}}^2$ or the projective bundle ${\mathbb {P}}(\mathcal {O}_{{\mathbb {P}}^1}\oplus \mathcal {O}_{{\mathbb {P}}^1}(-n))$ (the Hirzebruch surface $\Sigma _n$), for $n\ne 1$.

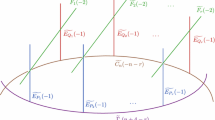
By Theorem 1.3, there exists a chain of morphisms $\pi =\pi _1\circ \cdots \circ \pi _r:X\rightarrow M$ such that M is either ${\mathbb {P}}^2$ or a Hirzebruch surface and $\pi _i:X_i\rightarrow X_{i-1}$ is the blowup of a smooth surface at a single point. Let E be the exceptional divisor of $\pi$ and $E_i$ the exceptional divisor of $\pi _i$. Then, $\pi (E)\subset M$ is a finite set of closed points and $\pi _i(E_i)$ is one closed point. Moreover, $E_i\simeq {\mathbb {P}}^1$ and E is a finite union of smooth rational curves (in fact, $E_1$ and the proper transforms of all the $E_2,...,E_r$). We begin by proving Theorem 1.1 for $X=M$ with care for the centers of the blowups:

Lemma 1.4

In the above conditions, there exist three open subsets $U_0^0$, $U_1^0$, $U_2^0$ such that:

• (1) $U_0^0\cup U_1^0\cup U_2^0 =M$.

• (2) For all $i=0,1,2$, $U_i$ is isomorphic to the affine plane.

• (3) $\pi (E)\subset U_0^0\cap U_1^0\cap U_2^0$.

Proof

The case $M={\mathbb {P}}^2$ is well-known. Since $\pi (E)$ is finite and we work over an infinite field, one can choose three different projective lines $L_1$, $L_2$ and $L_3$ in ${\mathbb {P}}^2$ such that $\pi (E)\cap (L_1\cup L_2\cup L_3)=\emptyset =L_1\cap L_2\cap L_3$.

If M is a Hirzebruch surface, then it is the projective bundle of a rank two vector bundle ${\mathcal {O}}_{{\mathbb {P}}^1}\oplus {\mathcal {O}}_{{\mathbb {P}}^1}(-m)$ over ${\mathbb {P}}^1$. This means that there is a surjective morphism $p:M\rightarrow {\mathbb {P}}^1$ such that, for any point $P\in {\mathbb {P}}^1$, $p^{-1}({\mathbb {P}}^1-\{P\})\simeq ({\mathbb {P}}^1-\{P\})\times {\mathbb {P}}^1$. Then, since we work over an infinite field, one can choose a closed point $P_0\in {\mathbb {P}}^1-p(\pi (E))$ with its isomorphism $q_0:p^{-1}({\mathbb {P}}^1-\{P_0\})\rightarrow {\mathbb {A}}^1\times {\mathbb {P}}^1$. Then, we choose a line $L_0={\mathbb {A}}^1\times \{Q_0\}$, such that $q_0(\pi (E))\cap L_0$ is empty. With this choice, $U_0^0=q_0^{-1}({\mathbb {A}}^1\times {\mathbb {P}}^1-L_0)$ is isomorphic to ${\mathbb {A}}^2$ and contains $\pi (E)$.

Then, $M-U_0^0$ is the union of two rational curves $C_1:=p^{-1}(P_0)$ and $C_2:=\overline{q_0^{-1}(L_0)}$. Choosing $P_1\in {\mathbb {P}}^1-(p(\pi (E))\cup \{P_0\})$ (again, the complement of a finite set), together with the isomorphism $q_1:p^{-1}({\mathbb {P}}^1-\{P_1\})\rightarrow {\mathbb {A}}^1\times {\mathbb {P}}^1$, we have that $p^{-1}({\mathbb {P}}^1-\{P_1\})$ contains $C_1$ and $C_2$ with the exception of the point $R_1:=C_2\cap p^{-1}(P_1)$ (the intersection of a section ${\mathbb {A}}^1\rightarrow {\mathbb {A}}^1\times {\mathbb {P}}^1$ with a fiber). We now choose a line $L_1={\mathbb {A}}^1\times \{Q_1\}$ such that:

• $Q_1\in {\mathbb {P}}^1$ is not in the second projection of $q_1(\pi (E))\in {\mathbb {A}}^1\times P^1$; and

• $L_1\ne q_1(C_2)$ (i.e. we are asking a constant section not to coincide with a given one, which is an open condition for $Q_1$), so the intersection of the two curves is finite.

Then, $U_1^0=q_1^{-1}({\mathbb {A}}^1\times {\mathbb {P}}^1-L_1)$ is isomorphic to ${\mathbb {A}}^2$ and contains $\pi (E)$.

Now, $M-(U_0^0\cup U_1^0)=(C_1\cup C_2)-U_1^0$ is the finite set $A:=\{R_1\}\cup q_1^{-1}(L_1\cap q_1(C_2))$. Finally, we have again the complement of a finite set to choose $P_2\in {\mathbb {P}}^1-(p(\pi (E))\cup p(A)\cup \{P_0,P_1\})$ with the isomorphism $q_2:p^{-1}({\mathbb {P}}^1-\{P_2\})\rightarrow {\mathbb {A}}^1\times {\mathbb {P}}^1$, so we have $A\subset p^{-1}({\mathbb {P}}^1-\{P_2\})$. We now choose $L_2={\mathbb {A}}^1\times \{Q_2\}$ such that $Q_2\in {\mathbb {P}}^1$ is not in the second projection of the finite set $q_2(A\cup \pi (E))\subset {\mathbb {A}}^1\times {\mathbb {P}}^1$, and we define $U_2^0=q_2^{-1}({\mathbb {A}}^1\times {\mathbb {P}}^1-L_2)\simeq {\mathbb {A}}^2$. Then $\pi (E)\subset U_2^0$ and, since $A\subset U_2^0$, we have that $U_0^0\cup U_1^0\cup U_2^0 =M$. $\square$

Remark 1.5

Let Bl$_P({\mathbb {A}}^2)$ be the blowup of the affine plane at a point P. Consider a line l passing through P and define $U_l$ as the complement in Bl$_P({\mathbb {A}}^2)$ of the proper transform of l. Just by changing coordinates, one has that all $U_l$ are isomorphic to each other. Since the case for l being the vertical axis is well known to be isomorphic to ${\mathbb {A}}^2$, by restricting the defining projection $\pi :$Bl$_P({\mathbb {A}}^2)\rightarrow {\mathbb {A}}^2$, we have morphisms $\pi _l:U_l\simeq {\mathbb {A}}^2\mapsto {\mathbb {A}}^2$. Moreover:

• (1) for $l_1\ne l_2$, $U_{l_1}\cup U_{l_2}=$Bl$_P({\mathbb {A}}^2)$.

• (2) if $E_{{\mathbb {A}}^2}$ is the exceptional divisor of Bl$_P({\mathbb {A}}^2)$, for any line l passing through P, $E_{{\mathbb {A}}^2}-U_{l}$ consists in one point, given by the isomorphism between $E_{{\mathbb {A}}^2}$ and the ${\mathbb {P}}^1$ of all lines through P.

• (3) the restriction $\pi _l|_{U_l-E_{{\mathbb {A}}^2}}$ is an isomorphism between $U_l-E_{{\mathbb {A}}^2}$ and ${\mathbb {A}}^2-l$.

Lemma 1.6

Let X be a smooth rational surface such that there exist three open subsets $U_0,U_1,U_2\subset X$ with

• (1) $U_0\cup U_1\cup U_2 =X$.

• (2) For all $i=0,1,2$, $U_i$ is isomorphic to the affine plane.

Consider a finite set $A_1\subset U_0\cap U_1\cap U_2$. Let $P\in (U_0\cap U_1\cap U_2)-A_1$ be a point and consider $\pi :Y\rightarrow X$ to be the blowup of X at P. Consider also a finite set $A_2$ in the exceptional divisor $E=\pi ^{-1}(P)\subset Y$. Then, there are three open subsets $U_0',U_1',U_2'\subset Y$ such that

• (1) $U_0'\cup U_1'\cup U_2' =Y$.

• (2) For all $i=0,1,2$, $U_i'$ is isomorphic to the affine plane.

• (3) Both $A_2$ and the proper transform of $A_1$ are contained in $U_0'\cap U_1'\cap U_2'$

Remark 1.7

In the conditions of Lemma 1.6, note that for any $i,j=0,1,2$, $i\ne j$, $X-(U_i\cup U_j)$ is a Zariski closed subset of a projective surface which is contained in $U_k\simeq {\mathbb {A}}^2$, with $i\ne k\ne j$. Since it is a projective scheme in an affine space, it must be finite.

Proof

Taking into account Remark 1.5, consider a line $l_0\subset U_0\simeq {\mathbb {A}}^2$ through P such that

• The intersection of $l_0$ with the finite set $X-(U_1\cup U_2)$ (see Remark 1.7) is empty.

• $A_1\cap l_0=\emptyset$.

• The intersection point of the proper transform of $l_0$ with the exceptional divisor is not in $A_2$.

Then, we define $U_0'$ to be the open subset $U_{l_0}$ of the blowup of $U_0$ at P. $U_0$ is isomorphic to the affine plane, as said in Remark 1.5, and $Y-U_0'$ consists in the proper transform of $l_0\cup (X-U_0)$. Therefore, it is one-dimensional.

Now, we choose a line $l_1\subset U_1\simeq {\mathbb {A}}^2$ such that the following open conditions are satisfied:

• (1) The intersection of $l_1$ with the finite set $X-(U_0\cup U_2)$ (see Remark 1.7) is empty.

• (2) the intersection multiplicity of $l_1$ and $l_0$ at P is 1 (note that $l_1$ is smooth at P, so we are asking that $l_1$ is not the tangent line at P to $l_0$, when we see them in $U_1$).

• (3) $l_1$ does not contain any point in $l_0\cap (X-U_2)$ (note that $l_0$ is irreducible and $P\subset l_0\cap U_2$, so such intersection is finite).

• (4) $A_1\cap l_1=\emptyset$.

• (5) The intersection point of the proper transform of $l_1$ with the exceptional divisor is not in $A_2$.

Since we work over an infinite field, these conditions define a nonempty Zariski open subset to choose $l_1$ from. Now, we define $U_1'$ to be $U_{l_1}\simeq {\mathbb {A}}^2$. The whole exceptional divisor is in $U_0'\cup U_1'$. Then, $Y-(U_0'\cup U_1')$ is the proper transform of the finite sets $B_1=[l_0\cup (X-U_0)]\cap l_1$ and $B_2=X-(U_0\cup U_1)$.

Note that $P\not \in B_1\cup B_2\subset U_2$, so we choose a last line $l_2\subset U_2\simeq {\mathbb {A}}^2$ such that

• the intersection of $l_2$ with the finite set $X-(U_0\cup U_1)$ (see Remark 1.7) is empty,

• $(A_1\cup B_1\cup B_2)\cap l_2=\emptyset$, and

• the intersection point of the proper transform of $l_2$ with the exceptional divisor is not in $A_2$.

Defining $U_2'=U_{l_2}$, one concludes the proof. $\square$

Remark 1.8

It is likely that a generalisation of Lemma 1.6 to higher dimension is possible. However, it is not yet known if all rational varieties of dimension greater than 2 are covered by open subsets isomorphic to open subsets of ${\mathbb {A}}^n$ (see [7] for the original question). These varieties are known as plain [3] or uniformly rational [4] and it is possible that the main result can be extended to higher dimension for this type of varieties.

Proof of Theorem 1.1

By Lemma 1.4, we have that $M=X_0=U_0^0\cup U_1^0\cup U_2^0$ with $U_i^0\simeq {\mathbb {A}}^2$ and $\pi (E)\subset U_0^0\cap U_1^0\cap U_2^0$. Now we apply Lemma 1.6 to $\pi _i:X_i\rightarrow X_{i-1}$, choosing

$$\begin{aligned} A_1=\left[ \pi _{i}\circ \pi _{i+1}(E_{i+1})\cup \cdots \cup \pi _i\circ \cdots \circ \pi _r(E_r)\right] -\{P_i\} \end{aligned}$$

(i.e. the points to be the center of future blowups outside $\{P_i\}$) and

$$\begin{aligned} A_2=\left[ \pi _{i+1}(E_{i+1})\cup \cdots \cup \pi _{i+1}\circ \cdots \circ \pi _r(E_r)\right] \cap E_i \end{aligned}$$

(i.e. the points to be center of future blowups in $E_i$). Note that any curve contracted by $\pi _{i+1}\circ \cdots \circ \pi _i$ is contracted to a point in $\pi _i^{-1}(A_1)\cup A_2$. We then get $U_0^i, U_1^i, U_2^i$ from $U_0^{i-1},U_1^{i-1},U_2^{i-1}$ all isomorphic to ${\mathbb {A}}^2$ and covering $X_i$, with all centers of future blowups in the intersection of the three open subsets. Then $U_0^r$, $U_1^r$ and $U_2^r$ are the three open subsets in the statement. $\square$