Fiscal centralization: a remedy for corruption?

Abstract

The empirical evidence on the relationship between political decentralization and corruption is far from clear. We develope a model that analizes the relationship between decentralization and corruption and show how cost–benefit calculations can lead public administrations to tolerate a certain degree of corruption. In the model economy, the centralized provision of public goods might be more efficient than their decentralized provision, but under very restrictive conditions. Decentralization, on the other hand, is more efficient where there exists heterogeneity across jurisdictions. In this scenario, the descentralized provision of public goods would be more efficient because regional governments would dedicate optimal amounts of funding to fighting corruption, while their centralized provision might lead to the over or under-allocation of resources.

1 Introduction

Corruption in the public administration is perceived differently, depending on the social and cultural characteristics of each country. According to a special Eurobarometer report on corruption, half of all Europeans (49%) do not think corruption in their country is more widespread than in other EU Member States.¹ Opinion is very diverse across the EU with respondents in Greece (80%) and Romania (78%) the most likely, and those in Denmark, Sweden and the Netherlands (all 2%) the least likely to think that corruption is more widespread in their countries.

This means that an activity that is seen as corrupt in some countries might be regarded as acceptable in others. Different countries and possibly different regions within the same country will therefore have varying degrees of tolerance for corruption. These different perceptions will also lead to differences in the initiatives that are taken to combat corruption and in the demand for political accountability. Nevertheless, in spite of these discrepancies, most western countries seem to be moving toward a common understanding of what corruption in the public administration constitutes.

There is an extensive literature that analyzes the effects of corruption (see for instance, Mauro 1995, 1998; Tanzi and Davoodi 1997; Gupta et al. 1998; Alesina and Weder 1999, among others). Nonetheless, fewer studies analyze the causes of corruption (see Ades and Di Tella 1995, 1997; Leite and Weidmann 1999; Tanzi 1998 among others), particularly in terms of the relationship between decentralization and corruption in the public administration.

Very often decentralization is pointed to as one of the main causes of corruption in in literature. It is argued that decentralization broadens the potential for corruption because: (i) the public administrations are multiplied, which means that there are more public resources available for rent-seeking; (ii) local officials live in close proximity with the citizens they serve and so local elites can make a greater impact on the decision process; (iii) there may be a shortage of highly skilled public workers to meet the increase in the demand for public officials and politicians, and efforts by local bureaucrats to combat corruption may be less rigorous as a consequence; and, (iv) it creates barriers to change the status quo. From an empirical perspective, Fan et al. (2009) find strong evidence that the danger of uncoordinated rent-seeking increases as government structures become more complex and Treisman (2000) also shows that states with more tiers of government tend to have higher perceived levels of corruption. These results are corroborated by Nelson (2013).

On the other hand, some authors (see Shleifer and Vishny 1993; Huther and Shah 1998) find empirical evidence that increased decentralization reduces corruption (see Shah 2006 for a comprehensive literature review). In addition to these authors, Tanzi 1994 argues that personal links between bureaucrats and the people they serve reduce the probability of corruption, which suggests that centralized states will have more corruption. In fact, the World Bank suggests that decentralization is an appropriate way of fighting corruption in developing countries (see Gatti and Fisman 2000; Ivanya and Shah 2010). Freille et al. (2010) argue that there are different definitions of decentralization and that while fiscal decentralization is associated with lower levels of corruption, some forms of political decentralization worsen the positive effect of constitutional centralization on corruption. Lessmann and Markwardt (2010) find that decentralization counteracts corruption in countries where the press has a high degree of freedom, whereas countries with no effective monitoring by the press suffer under a decentralized system of government. Finally, Fiorino et al. (2015) find that there is a negative relationship between corruption and decentralization, with this relationship taking 3–5 years to develop.

The inconclusiveness of current empirical work on the subject is partly due to the lack of comprehensive and comparable indicators for measuring decentralization and corruption across countries. There are also very few theoretical models that can help us to understand such data, the exceptions being, as far as we know, Arikan (2004) and Albornoz and Cabrales (2013). Arikan (2004) developed a model to analyze corruption based on a tax-competition model, finding that fiscal decentralization leads to a lower level of corruption. On the other hand, Albornoz and Cabrales (2013) argue that the relationship between decentralization and corruption depends on the degree of political competition and they find that decentralization is associated with lower (higher) levels of corruption when there is high (low) political competition.

In this paper, we develop a theoretical model that analyzes the relationship between political and fiscal decentralization and corruption. In particular, we model central provision of public goods with multiple territorial units in a novel way. In the existing literature it is often assumed that the centralized provision of public goods is decided on by politicians who are not swayed by local taxpayers, and that their aim is to maximize the nation’s welfare. Central governments are also often assumed to recruit public workers with no personal or professional links with local agents, thus supposedly limiting the influence of any local elites. However, this is far from realistic, because in numerous fields of central government authority, this is not the system for the provision of public goods that can actually be found. There is a more realistic intermediate scenario, not traditionally taken into account in theoretical models, midway between centralization and decentralization.² In this alternative scenario, responsibilities are delegated by the central administration to hierarchically dependent units created on some kind of spatial basis, a system we refer to as administrative deconcentration.³ This regime is what we find in most centralized countries, and it is the one that characterizes the organization of administrative activity of most federal governments.

In this alternative scenario, although the central authorities decide the rules to be implemented nation-wide, they are implemented through its offices in each jurisdiction. This means that the public workers who have to implement these rules and provide these public services—such as judges, prosecutors, local attorneys, the central administration’s local representatives, federal policemen, National Park employees or coastline inspectors—do have strong links with local agents, and very often they are local agents themselves. It might be argued that in countries with centralized systems, these public workers are selected through competitive recruitment exams open to all the nation’s citizens and that where they are posted will depend on their qualifications, preferences and the central administration’s needs in each jurisdiction. However, in countries where citizens are reluctant to move or countries whose jurisdictions have some kind of peculiarities, such as a specific culture, language, civil law, geographical location, etc., public workers in these regions are very likely to have been born and raised in the region where they finally work.

In this paper, we deal with different systems for the provision of public goods, based on three spatial forms of political organization, and we analyze their impact on corruption at an aggregate level. First, we take the scenario of a “traditional” centralized system of government; second, we analyze a centralized system of government that provides public services through public agencies created on a hierarchically organized territorial basis, that we refer to as deconcentration; and finally, we study the provision of public goods by self-governing regional governments. At this point, it is very important to stress that the distinction between these three regimes is based on a fiscal federalism approach. We mention that because from a formal point of view, based on the solutions to the theoretical model, the reader might think that deconcentration is a special case of decentralization. However, although the solutions to decentralization and concentration are rather similar, from a fiscal federalism approach, deconcentration is a special case of centralization

We analyze the three different systems individually, assuming that they do not coexist. In addition, we incorporate the possibility that central and regional governments might be committed to combating corruption to different degrees, which is corroborated by the European Quality of Governance Index.⁴

Our results are as follows: First, the level of commitment to fight corruption is an economic decision and under certain conditions, public administrations will tolerate certain degrees of corruption. Second, the centralized provision of public goods might be more efficient than their decentralized provision, but under very restrictive conditions. These conditions are homogenous initial levels of corruption across regions, efficient recruiting procedures of staff by the central government and rent-seeking opportunities being lower in a centralized framework. Third, decentralization is more efficient than centralization when heterogeneous jurisdictions are considered. In this scenario, the decentralized provision of public goods would be more efficient because regional governments would dedicate optimal amounts of funding to fighting corruption, while their centralized provision might lead to the over or under-allocation of resources (which would represent a waste of public funding in the first case and a tolerance of corruption in the second). This result must be qualified when the central administration takes into account regional heterogeneity and the specificities of its regions in the provision of public goods.

In the next section, we refer what we understand from corruption. In Sect. 3, a presentation is given of our model and, in Sect. 4, we present the main findings. Finally, our conclusions are outlined in Sect. 5.

2 Corruption

In this paper, corruption is defined as practices in the public sector that lead to the misuse of public funding (see Shah 2006 for a detailed outline of corrupt practices). Not only does this include illegal activities but also ones that cause extra costs or else entail the under-provision of public goods or a loss of government revenue (other than losses merely associated with inefficient policies or behaviors). There are many examples of such practices, such as awarding a contract to a bidder even though their tender was not the best or to a bidder that deliberately under priced it with a view to pushing up the budget later. Other possible examples are awarding a concession based on unrealistic demand forecasts so that the public authorities finally have to pay de difference (e.g. forecast for the number of cars in highways, etc.), or changing zoning regulations that regulate land use to favor certain interest groups.

We focus our analysis on the role of public workers, grouped together to include politicians, higher officials and civil servants. We argue that it is not possible for politicians to be corrupt without the collaboration of civil servants and vice versa. For instance, according to the Eurobarometer European citizens think that politicians, particularly national politicians (57%), and officials awarding public tenders (47%) are the ones most likely involved in corruption.⁵

We incorporate corruption in our model by assuming that a certain percentage of public workers devote part of their time to fostering their own interests instead of working for the administration. The higher this percentage is, the more corruption there will be in that public authority.

We measure the cost of corrupt practices through the effect on the aggregate net income after public government action. The higher the percentage of potentially corrupt public workers, the lower the net income in that economy, because a certain amount of taxes will not be transformed into public goods.

As for the mechanisms used to combat corruption, we consider two of them. First, the government uses selection procedures to limit the number of potentially corrupt public workers who might be hired. Although these precautionary procedures are inexpensive, we think they are not very effective, because they do not prevent an honest public worker from being corrupted in a few years’ time. That is why these selection procedures will not eradicate corruption completely. We argue that differences in the procedures used by each of the political systems under consideration explain the initial differences in the level of corruption found in each one, although we do not go deep into their analysis.

Second, the public administration may decide to reduce its existing corruption. Bureaucratic requirements might be tightened to prevent decisions from being taken by one single person, stipulating that several public workers must be involved in them. Alternatively, the government might decide to monitor its workers, discouraging them from corrupt practices through the threat of dismissal. The main problem with these mechanisms is that they are very costly to put into practice, and thus ultimately the fight to combat corruption will be based on an endogenous economic decision. We focus our analysis on these mechanisms and their effects on combating corruption.

3 The model

We develop a model economy in which public workers decide whether or not to engage in corruption, while the public administrations decide how much resources to spend to fight corruption as well as whether or not to provide public goods in a centralized or decentralized way. In this model economy, corruption provides private benefits for workers but lowers the aggregate output.

3.1 Corrupt workers

We work in a framework where public workers work in an administration j that can take the form of a Central Government, an administrative territorial unit of a Central Government or a Regional Government. The number of Regional Governments or administrative territorial units is the same as the number of regions ($j=\ldots R$).

We assume that a public worker that works for administration j has the goal of maximizing his expected income ($EI_{j}$), which is represented by

$$\begin{aligned} EI_{j}=p_{j}(wT+s_{j}\ tc_{j}\gamma _{j}), \end{aligned}$$

where w is the fixed salary per hour received and it is considered as exogenous and uniform across administrations; $tc_{j}$ represents a fixed level of effort (in terms of time) devoted to corrupt activities and we assume that all corrupt workers will devote the same effort to corruption; T is total effort that should be devoted to work for the administration; $p_{j}$ is the probability that the administration cannot prove that the worker is corrupt and dismisses him; in this case he obtains positive income in spite of his low effort. Alternatively, with probability ($1-p_{j}$) he will be dismissed and we will get zero income. This probability is taken as given by public workers.

Finally, $s_{j}$ denotes extra income obtained from corrupt activities in that administration, heterogeneity might be explained by differences in rent-seeking opportunities, productive structure, etc. In addition to that, $\gamma _{j}$ denotes his ability to participate in corrupt activities. With respect to $\gamma _{j}$ we assume that there are two types of individuals, those with ability zero ($\gamma _{j}=0$) and those that present some ability in corruption, $\gamma _{j}\ne 0$. We assume that among N public workers, there is an exogenous fraction $\phi _{j}$ of workers that will engage in corruption if they have the economic incentive. We assume that all these individuals have the same ability, which means $\gamma _{j}=\gamma \ne 0$ and that the economy offer the same possibilities of rent-seeking to all individuals ($s_{j}>0$). For simplicity, given that there is no heterogeneity across individuals, we will deal with $s_{j}$ and $\gamma _{j}$ through a unique parameter $\delta _{j}$, which is positively related to both rent-seeking reward and rent-seeking abilities.

All public workers have the same time constraint

$$\begin{aligned} T=tf_{j}+tc_{j}, \end{aligned}$$

where $tf_{j}$ represents the effort that is effectively devoted to work for the administration.

If public administrations do not monitor public workers’ efforts, then the optimal decision of each type of public worker will be:

• (1) Individuals with $\delta _{j}=0$ would work for the public administration exclusively, $tf=T$

• (2) Individuals with $\delta _{j}\ne 0$ would devote $T-tc$ efforts to public activity. These individuals would receive their salary (Tw) plus rents obtained from corruption, with probability $p_{j}$.

As a consequence, if the public administration wants to prevent that politicians and civil servants participate in corrupt activities they should implement some procedures aimed at reducing $\delta _{j}$ or $p_{j}$.

3.2 Public administration

In our economy, aggregate income at jurisdiction j is represented by

$$\begin{aligned} Y_{j}=AC_{j}^{\alpha }F_{j}^{\beta }. \end{aligned}$$

We assume that the economy is endowed with fixed quantities of capital, denoted $C_{j}$. This means that $AC_{j}^{\alpha }$ can be treated as exogenous. For simplicity we will work with the parameter ${\hat{A}} =AC_{j}^{\alpha }$. $F_{j}$ denotes the public good provided by the public administration (we assume that $0<\beta <1$), which is measured through the number, and effort, of public workers, such that

$$\begin{aligned} F_{j}=(1-\phi _{j})N_{j}T+\phi _{j}N_{j}tf, \end{aligned}$$

which can be written as

$$\begin{aligned} F_{j}=N_{j}(T-tc\phi _{j}). \end{aligned}$$

Hence a fraction $\phi _{j}$ of workers engage in corruption and only work tf hours for the public administrations while ($1-\phi _{j}$) workers are not corrupt and work full time.

Let $B_{j}$ denote the budget that is devoted to hiring and monitoring public workers (where $B_{j}=\tau Y_{j}$), given by:

$$\begin{aligned} B_{j}=wN_{j}T\left[ 1-(1-p_{j})\phi _{j}\right] +M_{j,}. \end{aligned}$$

where $M_{j}$ refers to the costs associated to fighting corruption. The term $(1-p_{j})\phi _{j}$ enters in the budget constraint with a negative sign because with probability ($1-p_{j}$) corrupt public workers will be dismissed and they will not receive any salary.

We assume that public administration’s goal is to hire the optimal number of public workers ($N_{j}$) and how much to spend on fighting against corruption ($M_{j}$) to achieve $\phi _{j}=0$.

There is a final assumption that relates $M_{j}$ and $1-p_{j}$. We assume that there is a positive relationship between budget devoted to monitoring ($M_{j}$) and the probability to expose corrupt workers ($p_{j}$). In particular, this relationship follows

$$\begin{aligned} p_{j}=\frac{1}{1+M_{j}}, \end{aligned}$$

which means that the larger the efforts to fight corruption is, the lower is the probability of a corrupt public worker to succeed.

At this point, it is important to emphasize that $\phi _{j}$, the share of corrupt workers, is an endogenous object and will depend on $M_{j}$ and other model parameters. We assume that in each jurisdiction there is an exogenously given level of corrupt workers, denoted by $\overset{-}{\phi _{j} }$. The government can change initial levels of $\overset{-}{\phi _{j}}$ by improving the procedures addressed to monitor public workers and to reduce their probability to succeed ($p_{j}$). Therefore, decisions on $M_{j}$ affect $p_{j}$ and the decision to be taken by corrupt workers consequently, which affects $\phi _{j}$. We denote this dependence by $\phi _{j}(M)$

These procedures have some cost and they will be implemented or not depending on their effects on $Y_{j}-B_{j}$. Thus, the problem to be solved by the public administration j is

$$\begin{aligned}&\quad Max_{\{N_{j},M_{j}\}}\,\,{\hat{A}}_{j}\left[ N_{j}(T-tc\phi _{j}(M))\right] _{j}^{\beta }-B_{j}\\&\text {subject to }B_{j}=wN_{j}T\left[ 1-(1-p_{j})\phi _{j}(M)\right] +M_{j},\\&\qquad \qquad \text {and }p_{j}=\frac{1}{1+M_{j}}. \end{aligned}$$

It is important to note that we do not introduce any a-priory differences concerning the tolerance of corruption across different regimes of public good provision. Decisions on this matter are endogenous and derive from a maximization problem solved by each administration.

Before we present our results, four remarks should be made concerning the role of public administrations: First, given that we do not consider any specific role attributed to public workers, corrupts workers could be in charge of auditing effective efforts of public workers, which bring us to assume that resources devoted to avoid corruption are managed by non corrupt public workers. Second, the administration cannot identify corrupt workers individually. It may know that there are some workers that could be corrupt, but it does not know who they are, unless it applies a monitoring program.

Third, we will consider three different regimes of public goods provision and we will show that each regime has different effects on the final degree of corruption, and hence on the final level of regional income. First, we consider a centralized regime (denoted as C) that consists of a unique level of government that provides the public good. In this regime public workers contribute to national income regardless where they are located and this, according to some authors, implies that they are not subject to local tax payers influence. We present this regime as a benchmark. Second, we analyze a specific type of centralized provision which we refer to as “deconcentrated” (denoted by D). It is specific as far as the central administration takes the main decisions and public goods are provided through ($j=1,\ldots ,R$) administrative territorial units and public activity is executed through public workers distributed across regions. Therefore, centralized and deconcentrated regimes are treated as two different regimes in order to avoid confusion. Lastly, we consider a third regime that consists in R regional governments that are autonomous and that decide on the provision of public goods in their jurisdiction and they can hire their own workers (denoted by DC). We will differentiate each of the regimes by using subindexes (C, DC, an D) in the variables N, M, Y, B, $\delta$ and $\phi$. In addition to that, we will use subindex $j=1,\ldots ,R$ in case that we work with administrative territorial units or regional governments.

Unfortunately, there is no empirical evidence concerning which regime implements more efficient procedures aimed at selecting the lowest number of corrupt workers. As a consequence, no a-priory assumptions concerning potential differences among the distribution of corrupt public workers depending on the regime of provision of public goods are made. On the contrary, some evidence exist to what concerns difference in corruption levels across regions.⁶

4 Results

Results are presented as follows. First we analyze the inefficiency caused in case of corruption and the need to monitor public workers in a centralized regime, which is considered as a benchmark. Second we analyse under which conditions centralized provision is more efficient compared to a deconcentrated provision of public goods. Finally, we compare decentralized versus deconcentrated provisions of public goods.

4.1 Centralized provision of public goods

Two different scenarios are contemplated, depending on whether the government monitors its public workers or not. We eliminate the subscript j because only one jurisdiction is considered.

4.1.1 Non-monitoring of public workers

If central government decided $N_{C}$, disregarding the possibility that some of its public workers might be corrupt and not monitoring their work ($M=0$), then it would seek to solve:

$$\begin{aligned} Max_{\{N_{C}\}}\,\,{\hat{A}}_{C}(N_{C}T)^{\beta }-(wN_{C}T), \end{aligned}$$

(1)

where the First Order Conditions are given by:

$$\begin{aligned} \frac{\partial (Y_{C}-B_{C})}{\partial N_{C}}=0;\text {or }\beta {\hat{A}} _{C}(N_{C}T)^{\beta -1}=w, \end{aligned}$$

(2)

which results in the following optimal size of public employees,

$$\begin{aligned} N_{C}^{*}=\left( \frac{{\hat{A}}_{C}\beta }{w}\right) ^{\frac{1}{1-\beta }}\frac{1}{T}. \end{aligned}$$

(3)

Central Government would hire $N_{C}^{*}$ workers expecting their contribution to the aggregate income to be

$$\begin{aligned} Y_{C}={\hat{A}}_{C}(N_{C}^{*}T)^{\beta }. \end{aligned}$$

However, if there is no monitoring, $p=1$ and a fraction $\overset{-}{\phi _{C}}$ of public workers with $\delta _{j}\ne 0$ will engage in corruption, and the real impact of the $N_{C}^{*}$ public workers would instead be:

$$\begin{aligned} Y_{C}={\hat{A}}_{C}\left[ N_{C}^{*}(T-\overset{-}{\phi _{C}}t_{c})\right] ^{\beta }, \end{aligned}$$

because $\overset{-}{\phi _{C}}N_{C}^{*}$ public workers would contribute with $T-t_{c}$ efforts to aggregate income.

Thus, under a centralized political system where public workers are not monitored, the net income would be:

$$\begin{aligned} (Y_{C}-B_{C})={\hat{A}}_{C}\left[ N_{C}^{*}(T-\overset{-}{\phi _{C}} t_{c})\right] ^{\beta }-(wN_{C}^{*}T), \end{aligned}$$

(4)

which is below

$$\begin{aligned} {\hat{A}}_{C}(N_{C}^{*}T)^{\beta }-(wN_{C}^{*}T). \end{aligned}$$

4.1.2 Monitoring of public workers

If central government were aware that some of its public workers might be corrupt and it decided to monitor them, the problem would be:

$$\begin{aligned} Max_{\{N_{C},M_{C}\}}\,\,{\hat{A}}_{C}\left[ N_{C}(T-\phi (M_{C})t_{c})\right] ^{\beta }-wN_{C}T\left[ 1-(1-p(M_{C}))\phi (M_{C})\right] -M_{C}). \end{aligned}$$

(5)

The First Order Conditions for $N_{C}$ are given by:

$$\begin{aligned} \frac{\partial (Y_{C}-B_{C})}{\partial N_{C}}=0,\ \end{aligned}$$

(6)

which can be written as

$$\begin{aligned} \beta {\hat{A}}_{C}N_{C}^{\beta -1}(T-\phi (M_{C})t_{c})^{\beta }=wT[1-(1-p(M_{C}))\phi (M_{C})], \end{aligned}$$

and for $M_{C}$ are given by:

$$\begin{aligned} \frac{\partial (Y_{C}-B_{C})}{\partial M_{C}}=0, \end{aligned}$$

(7)

which can be written as

$$\begin{aligned} \beta {\hat{A}}_{C}[(T-\phi (M_{C})t_{c})]^{\beta -1}N_{C}^{\beta }\phi ^{\prime }(M_{C})t_{c}=wN_{C}T[(1-p(M_{C}))\phi ^{\prime }(M_{C})-\phi (M)p^{^{\prime }}(M_{C})]-1. \end{aligned}$$

At this point, we need to understand that a potential corrupt worker would be swayed when the expected financial benefits that they could reap from corrupt practices would exceed their real income. This decision therefore depends on whether:

$$\begin{aligned} EI=p(wT+\delta _{C}\ t_{c})>wT. \end{aligned}$$

The public administration knows that $p=\frac{1}{1+M}$, so it knows that if

$$\begin{aligned} M_{C}{}^{*}\subset \left( 0,\frac{\delta _{C}\ tc}{wT}\right] , \end{aligned}$$

it will pay to be corrupt and $\overset{-}{\phi _{C}}$ fraction of workers will still engage in corruption. In contrast, if

$$\begin{aligned} M_{C}{}^{*}\subset \left( \frac{\delta _{C}\ tc}{wT},\infty \right) , \end{aligned}$$

then there would not be corrupt workers. As expected, in a scenario of workers with a certain flair for corruption, the higher the potential rewards from corruption (that means $\delta _{C}\ne 0$) and tc efforts devoted to such practices, the more expenditure that would be needed by the public administration to avoid it, i.e.,

$$\begin{aligned} \frac{\partial M_{C}^{*}}{\partial \delta _{C}}>0,\frac{\partial M_{C}^{*}}{\partial tc}>0. \end{aligned}$$

It must be noted that the effect of spending $M_{C}=\frac{\delta _{C}tc}{wT} +\varepsilon$ ($\varepsilon \simeq 0$) would be $\phi _{C}=0$ and that larger amounts of $M_{C}$ would not improve the results (larger values would prove to be inefficient). It must also be noted that spending $M_{C}\le \frac{\delta _{C}\ tc}{wT}$ would not lead to changes in $\overset{-}{\phi _{C}}$, which implies that it is more efficient to spend $M_{C}=0$ than $M_{C}=\frac{\delta _{C}\ tc}{wT}$.

The question now is to quantify the optimal amount of expenditure that is needed to combat corruption ($M_{C}^{*}$).

Proposition 1

Tolerating corruption might be more efficient than spending larger amounts of money on combating it.

In order to prove it, we must consider that if the public administration decided to spend $M_{C}=\frac{\delta _{C}tc}{wT}+\varepsilon$, then $\phi _{C}=0$ and

$$\begin{aligned} N_{C}^{*} \mid _{M_{C}=\frac{\delta _{C}\ tc}{wT}+\varepsilon }= & {} \left( \frac{ {\hat{A}}_{C}\beta }{w}\right) ^{\frac{1}{1-\beta }}\frac{1}{T},\nonumber \\ (Y_{C}-B_{C})^{*} \mid _{M_{C} = \frac{\delta _{C}\ tc}{wT}+\varepsilon }\,= & {} \,\left[ \frac{{\hat{A}}_{C}\beta ^{\beta }}{w^{\beta }}\right] ^{\frac{1}{1-\beta }}(1-\beta )-\frac{\delta _{c}\ tc}{wT}-\varepsilon . \end{aligned}$$

(8)

On the contrary, if the decision was to spend $M_{C}=0$ (for which $p=1$) then results for $N_{C}^{*}$ and would be $(Y_{C}-B_{C})^{*}$

$$\begin{aligned} N_{C}^{*} \mid _{M_{C}=0}= & {} \left[ \frac{{\hat{A}}_{C}\beta \left( T-\overset{-}{\phi _{C}}t_{c}\right) }{wT}\right] ^{\frac{1}{1-\beta }}, \nonumber \\ (Y_{C}-B_{C})^{*} \mid _{M_{C}=0}= & {} \left[ \frac{\left( T-\overset{-}{\phi _{C}} t_{c}\right) ^{\beta }}{(wT)^{\beta }}\right] ^{\frac{1}{1-\beta }}{\hat{A}}_{C}^{\frac{1}{ 1-\beta }}\beta ^{\frac{\beta }{1-\beta }}(1-\beta ). \end{aligned}$$

(9)

Comparing Eqs. (10) and (11) we obtain that if

$$\begin{aligned} (Y_{C}-B_{C})\mid _{M_{C}=\frac{\delta _{C}\ tc}{wT}+\varepsilon }>(Y_{C}-B_{C})\mid _{M_{C}=0}^{*}, \end{aligned}$$

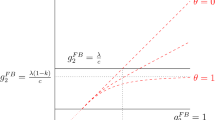
then the public administration would be interested in combating corruption. If the opposite were true, the public administration would tolerate corruption because the costs of having corrupt workers would be lower than the expenditure needed to dissuade them. More specifically, we can compute the threshold value for $\delta _{C}$ above which the government will not be interested in investing in monitoring because although public workers would be more efficient (hence increasing Y), the government should devote excessive resources to monitor them (increasing B) and it could hire a lower number of workers. This occurs for

$$\begin{aligned} \delta _{C}> \left[ \left[ \frac{\beta ^{\frac{1}{1-\beta }}}{w^{1-\beta }}\right] \hat{ A}_{C}^{\frac{1}{1-\beta }}(1-\beta )\left[ 1-\left[ \frac{(T-\overset{-}{\phi _{C}}t_{c})}{T}\right] ^{\frac{\beta }{1-\beta }} \right] -\varepsilon \right] \frac{wT}{t_{c}}. \end{aligned}$$

(10)

For a given $\overset{-}{\phi _{C}}$, the larger the rent-seeking opportunities ($\delta _{C}$) the more likely the government will tolerate corruption because the positive direct effects on income would be compensated by the negative effects on income due to the resources needed to dissuade corrupt workers.

4.2 Deconcentrated provision of public goods

With this scenario, central government has to decide how many workers to hire for each of R jurisdictions and how much it is willing to spend on monitoring them, $M_{Dj}$ (where D stands for deconcentration).

For the sake of simplicity, we assume that ${\hat{A}}_{R}=A(\frac{C}{R} )^{\alpha }$ for any j, which means that regional heterogeneity is contained in $\delta _{Dj}$ and $\overset{-}{\phi _{Dj}}$. This heterogeneity stems from the fact that even though the same procedure is used by central government to select workers, some regional characteristics—the culture, tradition, morality—might cause it to have differing effects in different regions. What is more, differences in $\delta _{Dj}$ might be explained by regional disparities in rent-seeking opportunities, the production fabric, etc.

The problem to be solved by the central government for each region is

$$\begin{aligned}&Max_{\{N_{Dj},M_{Dj}\}}{\hat{A}}_{R}[N_{Dj}(T-\phi _{j}(M_{Dj})t_{c}]^{\beta }-wN_{Dj}T[1-(1-p_{j}(M_{Dj}))\phi _{j}(M_{Dj})]\\&\quad -M_{Dj}, \text { for }j =1,\ldots ,R.\\ \end{aligned}$$

The First Order Condition for $N_{Dj}$ is:

$$\begin{aligned} \frac{\partial (Y_{Dj}-B_{Dj})}{\partial N_{Dj}}=0,\ \end{aligned}$$

(11)

which can be written as

$$\begin{aligned} \beta {\hat{A}}_{R}N_{Dj}^{-\alpha }(T-\phi _{j}(M_{Dj})t_{c})^{1-\alpha }=wT,\ j=\ 1,\ldots ,R, \end{aligned}$$

while for $M_{Dj}$ are

$$\begin{aligned} \frac{\partial (Y_{Dj}-B_{Dj})}{\partial M_{Dj}}=0, \end{aligned}$$

which can be written as

$$\begin{aligned} \beta {\hat{A}}_{R}\left[ (T-\phi _{j}(M_{Dj})t_{c})\right] ^{\beta -1}N_{Dj}^{\beta }\phi _{j}(M_{Dj})^{\prime }t_{c}= & {} \end{aligned}$$

(12)

$$\begin{aligned} wN_{Dj}T\left[ (1-p_{j}(M_{Dj}))\phi _{j}(M_{Dj})^{\prime }-\phi _{j}(M_{Dj})p_{j}(M_{Dj})^{\prime }\right] -1,\ j= & {} 1,\ldots ,R.\nonumber \\ \end{aligned}$$

(13)

With this scenario, the public administration knows that any public worker in region j would engage in corrupt activities as long as

$$\begin{aligned} M_{Dj}^{*}\subset \left( 0,\frac{\delta _{Dj}\ tc}{wT}\right] , \end{aligned}$$

where

$$\begin{aligned} \frac{\partial M_{Dj}^{*}}{\partial \delta _{Dj}}>0. \end{aligned}$$

Government’s decisions on $M_{Dj}^{*}$ must be taken considering its effects on the percentage of corrupt public workers ($\phi _{Dj}$) and on the regional net income. Thus, the decision with regard to $M_{Dj}^{*}$ will be based on a comparison of

$$\begin{aligned} (Y_{Dj}-B_{Dj})\mid _{M_{Dj}^{*}=\frac{\delta _{Dj}\ tc}{wT}+\varepsilon }\text {to }(Y_{Dj}-B_{Dj})\mid _{_{M_{j}^{*}=0}}\text {for each }j=1,\ldots ,R \end{aligned}$$

As long as $\delta _{Dj}\ne \delta _{D}$, $M_{Dj}^{*}$ must be computed for each region. Therefore, the government may decide $M_{Dj}^{*}=0$ for some regions (signifying that there would be some tolerance of corruption in there) and $M_{Dj}^{*}=\frac{\delta _{Dj}\ tc}{wT}+\varepsilon$ in the case of certain other regions.

At this point, it is interesting to analyze the effects on the aggregate net income if the government were to consider the uniform provision of $M_{Dj}^{*}$. This is the same as assuming the centralized provision with $\overset{-}{\phi _{Dj}}=\overset{-}{\phi _{D}}$ and $\delta _{Dj}=\delta _{D}$ for any j (see the previous section). In this case, several situations should be analyzed.

Proposition 2

If there were no regional heterogeneity with $\overset{-}{\phi _{Dj}}=\overset{-}{\phi _{D}}$ and $\delta _{Dj}=\delta _{D}$ for any j and it happened that $\overset{-}{\phi _{Dj}}=\overset{-}{ \phi _{C}}$ and $\delta _{jD}=\delta _{C}$ for any j, then there would be no difference between the centralized and the deconcentrated solutions; there would be no loss of efficiency by centralizing the provision of public workers and the finance spent on fighting corruption.

This means that if we substitute $\overset{-}{\phi _{C}}$ and $\delta _{C}$ for $\overset{-}{\phi _{D}}$ and $\delta _{D}$ in Eqs. (10) and (11), we would obtain

$$\begin{aligned} (Y_{C}-B_{C})\mid _{_{MC^{*}}}=\sum \limits _{j=1}^{R}(Y_{Dj}-B_{Dj})\mid _{_{M_{D}^{*}}}\text {.} \end{aligned}$$

(14)

Under this assumption, corruption would not be tolerated under deconcentration if

$$\begin{aligned} (Y_{Dj}-B_{Dj})\mid _{M_{D}^{*}=\frac{\delta _{D}\ tc}{wT}+\varepsilon }>(Y_{Dj}-B_{Dj})\mid _{_{M_{D}^{*}=0}}\text {for each }j=1,\ldots ,R, \end{aligned}$$

(15)

which occurs for

$$\begin{aligned} \delta _{D}<\left[ \left[ \frac{{\hat{A}}_{R}\beta ^{\beta }}{w^{\beta }}\right] ^{\frac{ 1}{1-\beta }}(1-\beta )\left[ 1-\left[ \frac{\left( T-\overset{-}{\phi _{D}}t_{c}\right) }{T}\right] ^{\frac{\beta }{1-\beta }}\right] -\varepsilon \right] \frac{wT}{t_{c}} \ \text {for each }j=1,\ldots ,R, \end{aligned}$$

which is the same condition as for the centralized case.

If the opposite were true, both political systems would tolerate corruption.

Proposition 3

If there were regional heterogeneity $(\overset{-}{ \phi _{Dm}}\ne \overset{-}{\phi _{Dl}}$ and/or $\delta _{mD}\ne \delta _{lD}$ for any pair of m and l) or if there were no heterogeneity but it happened that $\overset{-}{\phi _{Dj}}\ne \overset{-}{\phi _{C}}$ and/or $\delta _{jD}\ne \delta _{C}$, then a central government decision regarding uniform $M_{Dj}$, based on $\overset{-}{\phi _{C}}$ and $\delta _{C}$, might be inefficient meaning

$$\begin{aligned} (Y_{C}-B_{C})\mid _{_{M_{C}^{*}}}<\sum \limits _{j=1}^{R}(Y_{Dj}-B_{Dj})\mid _{_{M_{Dj}^{*}}.} \end{aligned}$$

This is due to the fact that there would be a shortage in the number of public workers and resources used to combat corruption in some regions (the resources invested in fighting corruption would have no impact) and a surplus in other regions.

For instance, in a region with $\overset{-}{\phi _{Dj}}=0\ne \overset{-}{ \phi _{C}}$ even with $\delta _{Dj}=\delta _{C}$, optimal $M_{Dj}^{*}$ should be zero, although the government might decide $M_{D}^{*}>0$ [which would occur for large $\overset{-}{\phi _{C}}$ and low $\delta _{C}$ according to Eq. (12)]. The same applies for $\overset{-}{\phi _{Dj}}= \overset{-}{\phi _{C}}$ with $\delta _{Dj}=0\ne \delta _{C}$, where again $M_{Dj}^{*}$ should be zero and $M_{D}^{*}>0$.

However, for another situations where the less heterogeneous the regions, the lower the distance between $\overset{-}{\phi _{Dj}}$ and $\overset{-}{ \phi _{C}}$ and the lower the differences between $\delta _{Dj}$ and $\delta _{C}$, the less inefficiency that there would be.

Proposition 4

The centralized provision would be more efficient if $\delta _{C}<\delta _{Dj}$ for all $j=1,\ldots ,R$ and $\overset{-}{\phi _{Dj}}> \overset{-}{\phi _{C}}$ for all $j=1,\ldots ,R$.

In order to prove this proposition, let us assume that $\delta _{C}=0$ and $\overset{-}{\phi _{C}}=0$ (which means that there would be no inefficiencies associated to corruption and that there would be no need for monitoring public workers) and that deconcentration implied that $\delta _{Dj}$ $\ne 0$ and $\overset{-}{\phi _{Dj}}\ne 0$ for some j, then the optimal solution on M in a deconcentrated regime, compared to a centralized one, would imply:⁷ (i) lower income levels due to the presence of corrupt workers ($\overset{-}{\phi _{Dj}}\ne 0$), even though it was not optimal to spend on monitoring them, (ii) lower income levels in case that it was optimal to spend resources in monitoring public workers, because these resources could not be spent in hiring public workers.

This result is important since it contradicts a long established result in the literature since Oates (1972) according to which in the presence of regional heterogeneity a decentralized provision is more efficient than a centralized (and uniform) provision. The result is due to the fact that decentralization/deconcentration might increase initial levels of corrupt workers and might provide higher rent-seeking opportunities compared to a centralized regime. The inefficiency due to a uniform provision of public goods in a scenario with regional heterogeneity is more than compensated by the increase rent-seeking opportunities in a decentralized regime.

4.3 Decentralized versus deconcentrated provision of public goods

In this section, we compare the deconcentrated provision of public goods by central government with the decentralized provision by R regional governments. Deconcentrated and decentralized administrations face the same problem, which means that a comparison of the centralized solution and decentralized solution is equivalent to comparing the central provision with the deconcentrated provision of public goods. This means that Propositions 2–4 still apply, substituting $\phi _{Dj}$ for $\phi _{DCj}$ and $\delta _{Dj}$ for $\delta _{DCj}$(DC stands for decentralized)

As mentioned previously, in the literature it is argued that $\overset{-}{ \phi _{C}}<\overset{-}{\phi _{DCj}}$ for any j. However, we argue that if public workers are hired by central government and regional characteristics are taken into account, even if there is no regional heterogeneity, this may result in $\overset{-}{\phi _{C}}<\overset{-}{\phi _{D}}$. Nevertheless, it possible that $\overset{-}{\phi _{DC}}>\overset{-}{\phi _{D}}$ or $\delta _{Dj}\lessgtr \delta _{DCj}$.

It must be emphasized that the deconcentrated provision of public goods is a particular type of centralized provision, meaning that under the previous assumptions, decentralization would be more efficient that centralization.

Again, in this scenario if there were heterogeneity across regions and regional specificities were not taken into account in the deconcentrated provision of public goods, then this regime would be inefficient compared with the decentralized provision of such goods. The final effects of corruption on the aggregate income would depend on the degree of heterogeneity across regions. The greater the heterogeneity in parameters $\overset{-}{\phi }_{DCj}$ and $\delta _{DCj}$, the less efficient a centralized (and uniform) provision of these goods would be.

In addition to that, we should compare a decentralized provision to a deconcentrated one that differentiate among regions. However, in this scenario results are inconclusive because when the decentralized provision and the non-uniform deconcentrated provision of public goods is compared, in case of heterogeneity across regions, differences in the net income would depend on the extent to which the procedures used by central government to recruit public workers in each region limited corruption in relation to methods used by regional governments. Hence the results would depend on the relationship between $\overset{-}{\phi }_{DCj}\gtrless \overset{-}{\phi } _{Dj}$ for all $j=1,\ldots ,R$ and $\delta _{Dj}\gtrless \delta _{DCj}$ for all $j=1,\ldots ,R$). In particular for the case that $\overset{-}{\phi } _{DCj}>\overset{-}{\phi }_{Dj}$ and $\delta _{Dj}>\delta _{DCj}$ for any $j=1,\ldots ,R$, then deconcentrated provision would be more efficient than decentralization, and viceversa.

All in all, when all the solutions for each of the political systems under consideration are compared, it can be concluded that differences in the level of corruption depend on: (i) whether it is optimal or not to invest in monitoring public workers, (ii) central government’s ability to decide N and M efficiently; that is, taking into account the characteristics of each region (in the case of heterogeneity across regions) and finally, (iii) the heterogeneity of the parameters that represent the initial percentage of corrupt public workers (more specifically, the parameter that relates to the selection procedure for recruiting public workers) and the potential that each region offers for revenues to be obtained from rent-seeking ($s_{j}$).

5 Conclusions

Whether there is a relationship between fiscal decentralization and corruption in the public administration is far from clear because empirical literature on the subject gives conflicting results. In addition to that, little attention has been paid in literature to theoretical studies of fiscal federalism and corruption. In this paper, we try to fill this gap by presenting a theoretical model that takes into account the provision of public goods under three different political systems. First, we analyze the level of corruption within the framework of a central administration that provides public goods, assuming that the location of the public workers is centralized and that only pure public goods are supplied. This scenario is included for comparative purposes only, because, for many of a central administration’s scopes of authority, public goods are provided in each jurisdiction (i.e. judges, federal policemen, regulatory agencies, etc.), particularly when there are regional specificities in terms of language, civil codes, geographical characteristics, etc. Second, we analyze an alternative political system in which the central administration provides public goods through public agencies created on a territorial basis. Finally, we consider a third scenario, characterized by the existence of self-governing regional governments strongly influenced by local tax payers.

Our results indicate that, first of all, the decision whether or not to combat corruption is an economic one, meaning that under certain conditions, public administrations will tolerate certain levels of corruption. Second, the results suggest that, compared with the decentralized provision of public goods, the centralized provision might be more efficient under very restrictive conditions, such as when there are uniform initial levels of corruption across regions, and also assuming that the central government’s staff recruitment procedures are efficient in weeding out potential corrupt public workers. Third, the results seem to show that decentralization is more efficient, compared to centralization, when heterogeneity among jurisdictional units is taken into account. In such a scenario, the decentralized provision of public goods would be more efficient because regional governments would spend optimal amounts of finance on fighting corruption while the centralized provision of public goods might lead to over-spending on combating corruption (denoting a waste of public resources) or under-spending (which would imply a tolerance of corruption). Lastly, this result is not robust in the event of regional heterogeneity when the central administration that provides public goods takes into account regional specificities, which means that a deconcentrated provision of public goods might be more efficient that a decentralized one.