Smart lost circulation materials for productive zones

Mansour, Ahmed; Dahi Taleghani, Arash; Salehi, Saeed; Li, Guoqiang; Ezeakacha, C.

doi:10.1007/s13202-018-0458-z

Original Paper - Production Engineering
Open Access
Published: 02 May 2018

Smart lost circulation materials for productive zones

Ahmed Mansour³,
Arash Dahi Taleghani¹,
Saeed Salehi²,
Guoqiang Li³ &
…
C. Ezeakacha²

Journal of Petroleum Exploration and Production Technology volume 9, pages 281–296 (2019)Cite this article

6858 Accesses
40 Citations
Metrics details

Abstract

Lost circulation has been a serious problem while drilling that may lead to heavy financial costs in the form of lost rig time and lost mud fluid. In severe cases, it can lead to well blowout with serious environmental hazards and safety consequences. Despite extensive advances in the last couple of decades, lost circulation materials used today still have disadvantages such as damaging production zones, failing to seal large fractures or plugging drilling tools. Here, we propose a new class of smart expandable lost circulation material (LCM) to remotely control the expanding force and functionality of the injected LCM. Our smart LCM is made out of shape memory polymers that become activated by formation’s natural heat. Once activated, these particles can effectively seal fractures’ width without damaging pores in the production zone or plugging drilling tools. The activation temperature of the proposed LCM can be adjusted based on the formation’s temperature. We conducted a series of experiments to measure the sealing efficiency of these smart LCMs as a proof of concept study. Various slot disk sizes were used to mimic different size fractures in the formation. The API RP 13B-1 and 13B-2 have been followed as standard testing methods to evaluate this product.

Introduction

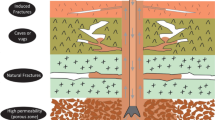
One of the most important decisions that need to be made in drilling operations is choosing the right type and density of drilling fluids. Drilling fluids are used in drilling operations to provide a pressure overbalance in the bottomhole and prevent the wellbore from collapsing. They are also used to cool down the drilling bits and transport cuttings to the surface. However, very often not all the drilling fluid is circulated back to the surface. Drilling fluid can be lost to the formation through fractures. Such incidents are called lost circulation events. Lost circulation is a costly problem due to creating non-productive time (NPT) while drilling, and if not controlled, it can cause serious environmental risks such as blowouts (Arshad et al. 2014). Table 1 shows the lower-end costs of lost circulation based on today’s market prices. It can be seen that controlling a severe lost circulation incident can take from 3 to 7 days. It becomes more expensive if the lost circulation is on an offshore rig than on an onshore rig. It was seen that most lost circulation incidents occur in highly permeable, karsted or naturally fractured formations (Al-Saba et al. 2014a, b). Given that 26% of the wells around the world experience such problems, a solution to such problems would be very beneficial especially in low oil price time were marginal costs should be minimized by operators.

Table 1 Cost of lost circulation

Full size table

There are two general approaches to prevent or minimize lost circulation problems. The first approach is called the preventive approach. This method can be used when the drill engineer anticipates that a lost circulation event may occur in a specific zone, but has not yet occurred. In this approach, lost circulation can be prevented by adding materials to the mud that will seal fractures and strengthen the wellbore, therefore, preventing a fracture from further propagation or worsening the fluid loss. The second approach is called corrective lost circulation treatment and can be achieved by adding materials known as lost circulation materials. When mixed with the mud, lost circulation materials have the ability to plug the fracture and seal it if their particle size is big enough to do so. The type, shape, composition, size distribution and strength of the lost circulation materials (LCMs) can also be important to design an efficient solution treatment to address lost circulation (White 1956). In this paper, we propose a smart expandable material made out of shape memory polymers that can seal the fracture and strengthen the wellbore.

Shape memory polymers have the ability to recover stress when inserted in a confined environment. The stress recovered is supposed to increase the circumferential compressional stress around the wellbore and may strengthen the wellbore by expanding the mud weight window.

To be able to understand how mud loss to the formation may occur during drilling and how to strengthen and stabilize the wellbore, we need to look at the stress distribution around the wellbore as drilling mud is primarily circulated in the bottomhole to keep the hole open and prevent the well from collapsing. Let’s assume a vertical well, the effective hoop stress can be defined as

$$ \sigma_{{\theta^{\prime } }} = \sigma_{\text{H}} + \sigma_{\text{h}} - p_{\text{p}} - p_{\text{m}} - \sigma_{\text{T}} - 2\left( {\sigma_{\text{H}} - \sigma_{\text{h}} } \right)\cos 2\theta $$

(1)

By assuming that the tensile strength of rock is negligible, wellbore starts bleeding mud when hoop stress becomes tensile. The only parameter that the drilling engineer can control in the above equation is the mud weight ($ p_{\text{m}} $). Wellbore strengthening is achieved here by the compression induced by the expansion of LCM materials filling the fracture. Increasing the compressional hoop stress will prevent the fracture from propagating. Alberty and Mclean (2004) presented a stress cage model to explain that the hoop stress around the wellbore can be enhanced by sealing the fracture mouth (isolating them). Salehi (2012) tested the wellbore hoop stress enhancement theory using a 3D poro-elastic, finite element model and obtained the same results. Here, we extend strengthening process by actually increasing compressional hoop stress.

Lost circulation materials

Wellbore instability has been estimated to cause economic losses of about 8 billion US dollars per year (Cook et al. 2012). From this 8 billion US dollars, it was estimated that lost circulation alone accounted for $2–$4 billion annual costs due to lost time (Cook et al. 2012). In the Gulf of Mexico, lost circulation, stuck pipe, sloughing shales and wellbore collapse account for 44% of the total non-productive time (Cook et al. 2012). The more non-productive time the well experiences will increase costs higher. Lost circulation does not only cause economic losses due to an increase in non-productive time. Uncontrolled loss of fluid can also damage the formation’s productivity and lead to further economic losses. The use of synthetic-based muds that range from $100 to $200 per barrel makes losing these fluids extremely costly.

LCMs work in such a way that particles in the mud increase in size in order to plug the pores and cracks that mud alone cannot seal (White 1956). Materials should have certain characteristics to be used as a LCM, which are briefly discussed in API standards 13B-1 and 13B-2. For instance, if the size of the particles is too small for the given fracture width, the particles will flow through the fractures and particle bridging may never occur. Similarly, if the size of the particles is too big, the particles will not be able to enter the fracture, and therefore, sealing may not take place. Therefore, the size of the material particles and their distribution are very crucial for a fast and proper sealing of fractures. The materials should also be able to adapt to a wide range of environments, temperatures and pressures. The seal formed by the LCMs should be able to withstand mechanical forces that come from drilling mud, erosional forces that come from the moving particles in the mud as well as hydrodynamic forces that come from swab and surge (Cook et al. 2012). Lost circulation materials can be classified as fibrous, flaky, granular types or a mixture of the three types. The LCM can be effective if the bridge it forms inside the fracture can withstand all the forces mentioned earlier. In order to make sure that the LCM seals the fracture effectively, researchers have come up with theories to maximize the chance of bridging. Bridging of particles is defined as the buildup of solids that reduces inflow. Various jamming or bridging theories are available, and their main goal is to predict bridging time. Abrams (1977) proposed two rules to minimize formation damage due to lost circulation and mud invasion. The first rule suggests that the average particle size (D50) of the bridging materials or LCMs should be equal or slightly larger than a third of the average formation pore size. The second rule suggests that the LCM concentration should not be less than 5% by volume of the total solids in the mud formation. Whitfill (2008) proposed an alternative approach to optimize bridging considering fracture width instead of pore throat sizes. The average particle size of the LCM should be equal to the fracture width to make sure that the fracture is effectively plugged.

Despite continuous efforts to improve effectiveness of LCMs and understand the mechanisms involved in their placement, there are still some shortcomings. The biggest disadvantage LCMs face today is failure in sealing big fractures. According to Al-Saba et al. (2014a, b), these big fracture sizes have apertures that are equal to or larger than few millimeters wide, and LCMs fail to bridge properly to seal it. The second disadvantage is failure to work in high-pressure and high-temperature (HPHT) environments; the LCM seal is either not able to withstand the high pressures or it will melt due to high temperatures. Third, according to the private communications with field engineers, if LCMs are not soluble, they may damage production zones and therefore affect productivity, which could be a serious problem. Finally, plugging drilling tools has been seen as an issue. LCMs that are too big in size sometimes may plug the tools and impose extra cost and service to control the well.

Looking at the disadvantages mentioned above, it is very clear that we need an LCM that can go through the drilling tools without plugging it and then through activation and expansion stage to plug big fractures and strengthen the wellbore.

Shape memory polymers

The shape memory effect was first discovered by Chang and Read in 1932. Shape memory polymers (SMPs) are polymers that have the ability to be deformed and fixed into a temporary shape; they are then able to recover to their original permanent shape only when they are exposed to a specific external stimulus such as heat, light, magnetic field, moisture or pH. Not all polymers can be fixed in a temporary shape. For example, rubber can change shape whenever it’s loaded, but when the load is removed the rubber goes back instantaneously to its original shape and no fixing of the temporary loaded shape has occurred. However, when the shape memory polymers are deformed when loaded, they have the ability to trap mechanical energy as internal energy and release this energy whenever an external stimulus causes a change in the molecular relaxation rate or in material morphology (Li 2014). Shape memory polymers not only have this shape-changing advantage but they are also cheap, lightweight, nontoxic, biocompatible and biodegradable (Ratna and Karger-Kocsis 2008).

The smart LCMs proposed in this paper will have temperature as an external stimulus. Shape memory polymers with temperature as an external stimulus can be either thermoset or thermoplastic. Thermoset SMPs are physically or chemically cross-linked polymers. They are usually preferred in engineering structures due to their high stiffness, high strength, high thermostability, high-dimensional stability and high corrosion resistance as compared to thermoplastic SMPs. During the shape recovery process, thermoplastic SMPs melt and, therefore, may not be an appropriate material to use as an LCM. The smart LCMs proposed here will have characteristics that stand between the thermoset and thermoplastic SMPs, a.k.a. ionic polymers or ionomers (Lu and Li 2016). Before explaining how smart LCMs will recover, seal fractures and strengthen the wellbore, there is a process called programming that needs to be done to achieve the mentioned advantages. Programming is the process that fixes the SMP in the temporary shape. A five-step thermomechanical cycle is used here for SMP programming as shown in Fig. 1.

This preparation process is called cold programming because the programming is conducted in the glassy state (Li and Xu 2011; Li et al. 2013). From Fig. 1, the original shape of the SMP is prestressed and put under compression at a temperature below the glass transition temperature ($ T_{\text{g}} $) region. The glass transition temperature is the temperature at which the SMPs go from being in a hard and glassy state to a pliable state. The second step is called stress relaxation and it happens while keeping the strain constant but relaxing the stress. The third step is removing the load. This completes the programming. As for recovery, it has two representative modes. One is free shape recovery, as shown in the fourth step in Fig. 1. This happens whenever the SMP is heated to above $ T_{\text{g}} $. It can also show stress recovery, as illustrated in step 5 in Fig. 1. Step 5 happens when the recovery is constrained and it is called constrained stress recovery.

It is noted that in programming of the shape memory polymers, the shape-changing properties allow the smart LCM to seal big fractures without plugging drilling tools or damaging production zones. Knowing the wellbore temperature profile, the smart LCM can be utilized in such a way that it will be small in size when entering the tool and then expand at a specific temperature within the fracture due to the difference between the mud and formation temperature. Therefore, the smart LCMs will have an advantage of sealing the fracture and preventing tool plugging. Because the smart LCM will also have stress recovery due to the constrained expansion, the wellbore can be strengthened by this stress release.

Siskind and Smith (2008) developed a model to predict the overall stress recovery of the activated SMP. Equation 2 shows the overall stress recovery from the SMP. The authors explain that the SMP has an initial volume and when this volume changes due to temperature, there will be a frozen volume portion ($ \varphi_{\text{f}} $) and an active volume portion ($ \varphi_{\text{a}} $). The active volume is the sum of the strain from the entropic and thermal components, while the frozen volume is the temporarily fixed volume, which transfers to active volume during the recovery process. The strains from both components are then subtracted from the total strain ($ \epsilon $) of the SMP and multiplied by the Young’s Modulus (E) to calculate the overall stress recovery of the SMP. The total strain can be calculated using Eq. 3.

$$ \sigma_{\text{overall}} = E\left( {\epsilon - \mathop \int \limits_{0}^{{\varphi_{\text{f}} }} \epsilon_{\text{f}}^{\text{e}} \left( x \right){\text{d}}\varphi - \mathop \int \limits_{{T_{0} }}^{{T_{\text{g}} }} [\varphi_{\text{f}} \alpha_{\text{f}} + (1 - \varphi_{\text{f}} )\alpha_{\text{a}} ]{\text{d}}T} \right) $$

(2)

$$ \epsilon = \frac{1}{V}\mathop \int \limits_{0}^{{V_{\text{frz}} }} \epsilon_{\text{f}}^{\text{e}} {\text{d}}V + \left[ {\varphi_{\text{f}} \epsilon_{\text{f}}^{\text{i}} + \left( {1 - \varphi_{\text{f}} } \right)\epsilon_{\text{a}}^{\text{e}} } \right] + \left[ {\varphi_{\text{f}} \epsilon_{\text{f}}^{\text{T}} + \left( {1 - \varphi_{\text{f}} } \right)\epsilon_{\text{a}}^{\text{T}} } \right] $$

(3)

Alternatively, Liu et al. (2006) proposed another model to calculate the overall stress recovery of the SMP:

$$ \sigma_{\text{Overall}} = E \left( { \varepsilon - \varepsilon_{\text{s}} - \mathop \int \limits_{{T_{\text{h}} }}^{T} \alpha {\text{d}}T } \right) $$

(4)

where E is given by

$$ E = {1 \mathord{\left/ {\vphantom {1 {\left( {\frac{{\varphi_{\text{f}} }}{{E_{\text{i}} }} + \frac{{1 - \varphi_{\text{f}} }}{{E_{\text{e}} }}} \right)}}} \right. \kern-0pt} {\left( {\frac{{\varphi_{\text{f}} }}{{E_{\text{i}} }} + \frac{{1 - \varphi_{\text{f}} }}{{E_{\text{e}} }}} \right)}} $$

(5)

$ \varphi_{\text{f}} $ in the above equation is the volume fraction of the frozen phase, which is given as

$$ \varphi_{\text{f}} = \frac{{\varepsilon_{\text{s}} }}{{\varepsilon_{\text{pre}} }}. $$

(6)

According to Wang and Li (2015), the overall stress recovery can be divided into four components: relaxed stress, thermal stress, memorized stress and residual programming stress. The relaxed stress occurs due to the SMP particles being constrained from expansion, and it reduces the overall stress recovery. The relaxed stress is given as

$$ \sigma_{\text{Relaxed}} = \sigma_{\text{eff}} \left( {1 - \mathop \sum \limits_{i = 1}^{n} \exp \left( { - \frac{t}{{\tau_{i} }}} \right)} \right). $$

(7)

The thermal stress occurs due to temperature rising. Since this is a constrained environment and free expansion is prohibited, stress is developed. Thermal stress can increase or decrease the overall stress based on the type of programming occurred. For example, it can be seen in Fig. 1 that the stress in step 5 will peak then decrease again; this peak is because of the thermal and entropic stresses. Subtracting the thermal stress will give a constant and more reliable stress release from the SMP. The thermal stress is calculated as

$$ \sigma_{\text{Thermal}} = \mathop \int \limits_{{T_{0} }}^{{T_{\text{r}} }} E\left( T \right)\alpha \left( T \right){\text{d}}t $$

(8)

The memorized stress is the stress stored in the programming phase of the shape memory polymer. It is calculated by

$$ \sigma_{\text{memorized}} = E(T) \cdot \varepsilon_{\text{r}} \cdot \left( {1 - \varphi_{\text{f}} } \right) $$

(9)

Finally, the residual programming stress is considered to be zero whenever there is an unloading phase in the programming of the SMP. The overall recovery stress in a confined environment is given by

$$ \sigma_{\text{overall}} = \sigma_{\text{Thermal}} + \sigma_{\text{memorized}} + \sigma_{\text{residual}} - \sigma_{\text{relaxed}} $$

(10)

According to Li (2014), the relaxed stress was measured using an MTS Q-TEST 150 machine with a fully constrained recovery. Li and Xu (2011) tried to calculate the memorized stress by considering the stress relaxation effect during stress recovery. The memorized stress is the difference between the measured stress by the MTS machine and the thermal stress and stress relaxation. They used this basic relationship to calculate the relaxed stress. Figure 2 shows the relaxed stress of the pure SMP versus SMP-based syntactic foam (Li and Nettles 2010).

Both of these SMPs types were also prestressed with two different stress values. It can be seen that the more the SMP is prestressed, the higher the stabilized recovered stress. The recovery stress peaks first and then gradually reduces until stabilization, due to the combined effect of thermal stress, memorized stress release and stress relaxation at the higher recovery temperature. After 3-D confined compression programming, the SMP-based syntactic foam showed a recovery stress as high as 26 MPa (Li and Uppu 2010), meaning that it will cause compressional forces on the wellbore hoop stress. These compressional forces could help prevent the hoop stress from going into tension and could lead to wellbore strengthening. Finally, whenever a bundle of SMPs are present and are activated above $ T_{\text{g}} $, their rubbery state allows them to bridge and connect together, forming an effective strong seal that isolates the fracture from the wellbore. When activated, the shape memory polymers use their ductility property to form bridges that are extremely hard to break. Therefore, there is a stress recovery advantage and a bridging advantage for LCMs made out of SMPs. It is also important to note that the smart LCMs will float in the mud due to their lower density. Adding surfactants to reduce the surface tension of the mud and disperse the particles will prevent such floating from occurring. SMPs have also been used as proppants (Santos et al. 2016) for re-fracturing operations (Santos et al. 2017) and in cementing applications (Taleghani et al. 2016).

Static fluid loss experimental procedure

In this section, the smart LCM proposed in this paper was tested for its expansive and sealing properties using the particle plugging apparatus (PPA). The PPA is a high-pressure, high-temperature apparatus that can withstand up to 5000 psi and 500 °F. Four different types of tests conducted using this experiment are reported here. Preliminary results from Mansour et al. (2017a, b) are also presented in this paper.

The PPA consists of a hydraulic pump that pumps fluid and measures pressure to the nearest 100 psi, a PPA cell, a LCM receiver, a slot or tapered disk to represent the fracture in the formation, a thermostat to adjust the temperature, a thermometer that measures temperature to the nearest 1 °F and a measuring cylinder that measures fluid loss to the nearest 1 ml. A PPA cell schematic can be seen in Fig. 3. The PPA cell is usually filled with a mixture of drilling fluid and LCMs. Then the slot disk is inserted on top of this mixture. The PPA cell has a floating piston in it which separates the oil coming from the hydraulic pump from the mixture of mud and LCM. The LCM receiver is then tightly capped at the top of the PPA cell and has a nozzle to allow the fluid that is lost from the PPA cell to be measured. The hydraulic pump is connected to the bottom of the PPA cell, where oil pushes the floating piston and if the slot or tapered disks are sufficiently sealed, then pressure builds up; if not, then fluid is lost and collected from the nozzle of the LCM receiver at the bottom of the apparatus.

The lost circulation material used in these experiments is made out of ionic shape memory polymers. The smart LCMs used here are manufactured in the form of disks, and their activation temperature is 158 °F and a melting temperature of 248 °F. As long as the temperature they are tested with is in between both temperatures, the particles will activate regardless of what the temperature is. The activation temperature is the temperature at which the smart LCM starts to expand and become a rubbery state. Disk-shaped particles settle down in the fractures along their thickness; hence, their expansion will occur normal to the fracture plane which is required to get a better sealing and more stress release. Figure 4 shows the smart LCMs before and after activation.

The smart LCMs have a density of 59.3 lb/ft³, Poisson’s ratio of 0.4, pre-strain (the maximum strain the LCM can achieve) of 25% and Young’s modulus of 95,000, 37,710 and 350 psi at temperatures of − 9.4, 73 and 176 °F, respectively. All experiments were done with the same type of smart LCMs but either shape or size was varied. The drilling fluid was made by adding 0.084 lb of bentonite to 0.092 gallons of water. The mud had a density of 8.9 ppg, and its formulation is provided in Table 2. 170 ml of this mud was taken, and the smart LCMs were added to this mud at a concentration of 0.3 lb of LCM per gallon of mud. This concentration of LCM is equivalent to almost 50 particles in the test sample. In one of the tests, the concentration was varied to see the relationship between smart LCM concentration and fracture sealing. The PPA cell is then filled with this mixture of drilling fluid and LCMs.

Table 2 Mud formulation

Full size table

A mud rheogram was made by an Anton Paar modular compact rheometer to see the behavior of the mud (with no LCMs) with shear stress. The tests were conducted at 73 and 176 °F. Figure 5 shows the viscosity versus shear rate. Using Fig. 5, we can understand the apparent viscosity of the mud at room temperature and at the temperature from which the smart LCMs will activate. It can be seen that the higher the temperature the higher the apparent viscosity of the mud. This is also considered a shear thinning drilling fluid where the viscosity decreases with increasing shear rate.

A slot disk or tapered disk is inserted on top of this mixture and on the top of the cell. The slot and tapered disk descriptions can be seen in Table 3. These dimensions are similar to the one used by Alsaba et al. to test the sealing of big fractures by conventional LCMs. Finally, all tests were repeated three times except the volumetric strain test which was repeated two times.

Table 3 Dimensions for disks

Full size table

Sealing efficiency test

The objective of this test is to check how effective the smart LCM is by measuring fluid loss and pressure buildup once the LCM is activated with respect to time. This experiment is supposed to show the decrease in fluid loss that the drilling engineer will see once the fracture is effectively sealed. The heating jacket was preheated to 167 °F. The LCMs used in this test were a mixture of two different diameter sizes. These sizes were 3 and 5 mm from which 0.15 ppg (lb/gal) of each smart LCM size was added to 170 ml of water-based mud and inserted in the PPA cell. The PPA cell was then inserted in the heating jacket and left there for 30 min before applying pressure, to allow the heat to be transferred to the cell to simulate downhole conditions. The fluid loss and the maximum pressure the seal can hold with respect to time were recorded. The fluid loss was measured by pumping hydraulic fluid in the cell at a rate of 2 ml/s. Since the fracture is being sealed with time, fluid is being prevented from going through the fracture and the pressure is building up. This pressure build up is also recorded with respect to time.

Volumetric strain measurement test

The objective of this test is to analyze the volumetric change property of the LCM as a function of pressure. Since the wellbore is a partially constrained environment, the smart LCM will not be able to fully recover to its shape due to pressure from the bottomhole. Therefore, this experiment will help the drilling engineer understand how the smart LCMs will expand under various pressures. Two particles were picked, and their diameter, thickness and mass were measured. These two particles were then mixed with the water-based mud and put in the PPA cell. A disk with no fractures was used instead of a slot disk to allow the pressure to build up in the PPA Cell. Oil was pumped from the hydraulic pump to raise the pressure until 3000 psi, and then the temperature was raised to 176 °F and kept constant for 30 min. The pressure was then dropped gradually until it reached zero and the particles were then cooled down to room temperature, 73 °F. The particles were then taken out from the PPA cell, and their diameter, thickness and mass were measured. This experiment was repeated for pressures of 0, 1000 and 2000 psi.

Permeability plugging test

The objective of this test is to measure the sealing efficiency of the smart LCM at 176 and 73 °F with respect to time and compare the results. This experiment will help drilling engineers understand the difference between the smart LCM and a normal LCM that has no expansive properties. It is also designed to see if the smart LCMs can still seal fractures if not activated.

Concentration required for sealing test

The objective of this test is to experiment different concentrations of LCMs through the slot and tapered disk and see how much LCM is required to seal the fracture efficiently. The LCM particles used here had a shape of a thin sheet as opposed to disk shape in previous experiments. They were also much smaller in size ranging from 0.25 to 1 mm. They are made out of the same material but they have a different shape. So another objective of this experiment is to see if the shape of the material matters when trying to seal the fracture.

These smart LCMs were mixed with 170 ml of the drilling fluid at concentrations of 0.24, 0.48 and 0.96 lb/gal with the slot disk and the tapered disk. The heating jacket was set to a temperature of 185 °F, and the mixture was left there to settle for 30 min before running the test.

Dynamic fluid loss experimental procedure

The objective of the dynamic fluid loss experiments conducted in this study is to quantify the fracture-sealing efficiency of the smart LCM. Table 4 shows the experimental design where the mud blend and temperature are two independent variables. The dependent variable is the cumulative mud loss. Water-based mud (WBM) was used as the control base fluid. Table 5 shows that the control base fluid was formulated with mud additives that would have little to no impact on the fluid loss and filtration property. 5 lb/bbl of the swellable polymer LCM was used in formulating the second recipe as shown in Tables 6 and 7. Based on the fluid loss test results from the second recipe and some preliminary tests, a combination of the smart LCM and fiber LCM, 5 lb/bbl each, was used in formulating the third recipe. 120 and 212 °F were chosen as the low and high temperature levels, respectively, and 10 ppg was selected as the design mud weight.

Table 4 Design of experimental table

Full size table

Table 5 Base mud formulation

Full size table

Table 6 Smart LCM mud formulation

Full size table

Table 7 Smart/fiber LCM mud formulation

Full size table

According to Ghalambor et al. (2014), lost circulation and drilling fluids invasion are classified into losses through pore throats, losses through induced and natural fractures and losses through vugs and carvens. In this study, a 2000-μm-width fracture cylindrical slot was used because this fracture size falls within the range of typical induced fracture widths observed from Petrophysics image logs. In addition, Alsaba (2015) performed similar static condition tests using this fracture size. Figure 6 (left) shows two parts (top and bottom) of the cylindrical core slot. The bottom part shows the fracture orientation and a fracture length of 10,000 μm. The dimensions of the entire cylindrical slot are: O.D = 1.5 inches, I.D = 1.0 inches and length = 1.1 inches. Figure 6 (right) shows that the core slot is carefully secured inside a core holder such that the only fluid exit from the entire system would be through the fracture.

Furthermore, most of the experiments that have been used to quantify fracture-sealing efficiency of LCM drilling fluids have been conducted in static conductions (Alsaba 2015; Guo et al. 2014; Kumar and Savari 2011; Hettema et al. 2007; Aston et al. 2004). In real-time drilling, bottomhole conditions are often in a dynamic mode, and a greater percentage of drilling fluids invasion occur during this time because the inertia state of the mud is surpassed by the hydrodynamic condition of mud particles and the fluids shearing action (Ezeakacha et al. 2016). Figure 7 shows the various stages of setting up the machine used in characterizing dynamic drilling fluid loss, for wellbore-shaped core samples and slots. The experimental procedure was programmed to track real-time data every 5 s, and operating parameters include but not limited to fluid loss through the fracture, rotary speed, temperatures (bath and sample) and pressures (back and cell). Based on previous tests and preliminary calibrations, 50 RPM, 100 psi and 200 psi were chosen as the rotary speed, back pressure and cell pressure, respectively.

Static fluid loss results and discussions

As mentioned above, four tests were made using the static fluid apparatus to test the smart LCMs. In this section results obtained for each test will be explained.