Some examples of rank-2 Brill–Noether loci

Abstract

In this paper, we construct some examples of rank-2 Brill–Noether loci with “unexpected” properties on general curves. The key examples are in genus 6, but we also have interesting examples in genus 5 and in higher genus. We relate some of our results to the recent proof of Mercat’s conjecture in rank 2 by Bakker and Farkas.

1 Introduction

Let C be a general curve of genus g defined over the complex numbers. The main focus of this paper is to study certain rank-2 Brill–Noether loci in the case $g=6$ and, in particular, to show that B(2, 10, 4) is reducible (see below for the definitions); this is contrary to naïve expectations. We consider also similar situations in genus 5 and in higher genus and finish with some results on bundles computing the rank-2 Clifford index for low values of g. These examples are presented as a contribution to higher rank Brill–Noether theory, which is still far from fully understood even in rank 2.

We denote by M(n, d) (respectively, $\widetilde{M}(n,d)$) the moduli space of stable bundles (respectively, S-equivalence classes of semistable bundles) of rank n and degree d on C and define

$$\begin{aligned} B(n,d,k):= & {} \{E\in M(n,d)|h^0(E)\ge k\}\\ \widetilde{B}(n,d,k):= & {} \{[E]\in \widetilde{M}(n,d)|h^0({\text {gr}}(E))\ge k\}. \end{aligned}$$

(Here [E] denotes the S-equivalence class of a semistable bundle and ${\text {gr}}(E)$ denotes the graded object associated with E.) We write also $K_C$ for the canonical bundle of C and $B(2,K_C,k)$ ($\widetilde{B}(2,K_C,k)$) for the subvariety of $B(2, 2g-2,k)$ ($\widetilde{B}(2,2g-2,k)$) given by bundles of determinant $K_C$. Our first main result is

Theorem 3.2

Let C be a general curve of genus $g=\lambda (2\lambda -1)$ for $\lambda \in \mathbb {Z}$, $\lambda \ge 2$. Then $B(2,K_C,2\lambda )$ has pure dimension $4\lambda (\lambda -1)-3$ and is smooth outside the non-empty locus $B(2,K_C,2\lambda +1)$. Moreover $B(2,2g-2,2\lambda )$ has at least one irreducible component of dimension $4\lambda (\lambda -1)-3$ which is not contained in $B(2,K_C,2\lambda )$.

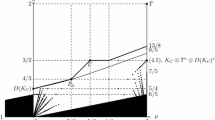
This is of particular significance in the case $\lambda =2$ or equivalently $g=6$, which is the first value of the genus for which the expected dimension of $B(2,2g-2,k)$ can be negative while that of $B(2,K_C,k)$ is non-negative. The appropriate value of k in this case is $k=5$ and we prove

Theorem 4.1

Let C be a general curve of genus 6. Then $\widetilde{B}(2,10,k)=\emptyset$ for $k\ge 6$. Moreover $B(2,10,5)=\widetilde{B}(2,10,5)=B(2,K_C,5)$ consists of a single point $E_{2,10,5}$ and $E_{2,10,5}$ is generated.

We show further that B(3, 10, 5) consists of a single point (Proposition 4.4). Also in Sect. 4, we relate our results for genus 6 to others in the literature and interpret them in terms of coherent systems.

In Sect. 5, we consider a somewhat analogous problem for $g=5$. Finally, in Sect. 6, we obtain some results on bundles computing rank-2 Clifford indices for low values of g which extend those of [9] and relate them to the recent result of Bakker and Farkas [2] confirming Mercat’s conjecture in rank 2 for general curves.

My thanks are due to the referee(s) for some useful suggestions.

2 Background and preliminaries

Throughout the paper, C will be a smooth curve of genus $g\ge 5$ defined over the complex numbers. For any vector bundle E on C, we write $n_E$ for the rank of E and $d_E$ for the degree of E. We define

$$\begin{aligned} {\text {Cliff}}(E):=\frac{1}{n_E}\left( d_E-2(h^0(E)-n_E)\right) \end{aligned}$$

and

$$\begin{aligned} {\text {Cliff}}_n(C):= & {} \min \big \{{\text {Cliff}}(E)|E \text{ semistable },\nonumber \\&n_E=n, h^0(E)\ge 2n_E, d_E\le n_E(g-1)\big \}. \end{aligned}$$

With this notation, ${\text {Cliff}}_1(C)$ is the classical Clifford index ${\text {Cliff}}(C)$. We recall that, for C a general curve of genus g, ${\text {Cliff}}(C)=\lfloor \frac{g-1}{2}\rfloor$ and the gonality of C (the minimal degree of a line bundle with $h^0\ge 2$) is ${\text {gon}}(C)=\lfloor \frac{g-1}{2}\rfloor +2$. It is clear that ${\text {Cliff}}_n(C)\le {\text {Cliff}}(C)$ for all n, and Mercat [12] conjectured that ${\text {Cliff}}_n(C)={\text {Cliff}}(C)$ (actually Mercat’s conjecture is a little stronger than this (see [8, Proposition 3.3]), but equivalent to it in rank 2). There are many counter-examples to this conjecture, but recently Bakker and Farkas [2] have proved that, for C a general curve of genus g,

$$\begin{aligned} {\text {Cliff}}_2(C)={\text {Cliff}}(C). \end{aligned}$$

(2.1)

The Brill–Noether locus B(n, d, k) has an “expected” dimension

$$\begin{aligned} \beta (n,d,k):=n^2(g-1)+1-k(k-d+n(g-1)). \end{aligned}$$

Provided $d<n(g-1)+k$, every irreducible component of B(n, d, k) has dimension $\ge \beta (n,d,k)$. The infinitesimal behaviour of B(n, d, k) is governed in part by the multiplication map (often referred to as the Petri map)

$$\begin{aligned} H^0(E)\otimes H^0(E^*\otimes K_C)\longrightarrow H^0(E\otimes E^*\otimes K_C). \end{aligned}$$

In fact, B(n, d, k) is smooth of dimension $\beta (n,d,k)$ at a point E if and only if the Petri map is injective. For $n=1$, one can define a Petri curve to be a curve for which

$$\begin{aligned} H^0(L)\otimes H^0(L^*\otimes K_C)\longrightarrow H^0(K_C) \end{aligned}$$

is injective for all line bundles L. The general curve of any genus is a Petri curve and, if C is Petri and $d<g-1+k$, B(1, d, k) is empty if $\beta (1,d,k)<0$, of dimension $\beta (1,d,k)$ if $\beta (1,d,k)\ge 0$ and irreducible if $\beta (1,d,k)>0$. Moreover, if $\beta (1,d,k)\ge 0$, the singular set of B(1, d, k) is $B(1,d,k+1)$. (For these and other results in classical Brill–Noether theory, see [1].) There is no analogue of these results for higher rank.

For $B(2,K_C,k)$, the expected dimension is not $\beta (2,2g-2,k)-g$, but instead it is

$$\begin{aligned} \beta (2,K_C,k)=3g-3-\frac{k(k+1)}{2}. \end{aligned}$$

There is also a different Petri map (obtained by symmetrizing the usual Petri map with respect to the natural isomorphism $E\simeq E^*\otimes K_C$)

$$\begin{aligned} {\text {Sym}}^2(H^0(E))\longrightarrow H^0({\text {Sym}}^2(E)). \end{aligned}$$

One can then prove that, on a general curve, this Petri map is always injective for stable E and hence $B(2,K_C,k)$ is smooth at any point E for which $h^0(E)=k$ (see [22]). There are also partial results on non-emptiness for $B(2,K_C,k)$ for all g [21] (see also [10, 24]) and complete results for small values of g [3]. Some detailed results for $k\le 3$ can be found in [7, section 7] and for $k=4$ in [6].

By a subpencil of a bundle E, we mean a rank-1 subsheaf L of E such that $h^0(L)=2$. The following lemmas will be useful.

Lemma 2.1

Let E be a bundle of rank 2 on C such that $h^0(E)=s+2$, $s\ge 1$. If E does not admit a subpencil, then $h^0(\det E)\ge 2s+1$.

Proof

This is the rank-2 case of [19, Lemma 3.9]. $\square$

Corollary 2.2

Let C be a general curve of genus $g\ge 6$ and E a semistable bundle with $d_E=2g-2$ which computes ${\text {Cliff}}_2(C)$. If $g=9$, suppose in addition that E is stable. If either g is even or $\det E\not \simeq K_C$, then E is expressible in the form

$$\begin{aligned} 0\longrightarrow L\longrightarrow E\longrightarrow L'^*\otimes K_C\longrightarrow 0, \end{aligned}$$

(2.2)

where $d_L=d_{L'}=\left\lfloor \frac{g-1}{2}\right\rfloor +2$ and $h^0(L)=h^0(L')=2$. Moreover, all sections of $L'^*\otimes K_C$ lift to E.

Proof

Suppose first that $g=2s$, so that ${\text {Cliff}}_2(C)={\text {Cliff}}(C)=s-1$ and $h^0(E)=s+2$. If E does not admit a subpencil, then, by Lemma 2.1, $h^0(\det E)\ge 2s+1=g+1$, a contradiction. Now suppose that $g=2s+1$, so that ${\text {Cliff}}_2(C)=s$ and again $h^0(E)=s+2$. Now, by Lemma 2.1, $h^0(\det E)\ge 2s+1=g$. Since $\det E\not \simeq K_C$, this is again a contradiction. So E admits a subpencil.

For $g=6$, the only possibility is given by (2.2). For $g\ge 7$, the existence of (2.2) follows from [9, Proposition 7.2 and Theorem 7.4]. Using Riemann-Roch, it is easy to check that

$$\begin{aligned}h^0(L)+h^0(L'^*\otimes K_C)=s+2=h^0(E),\end{aligned}$$

so all sections of $L'^*\otimes K_C$ must lift to E. $\square$

Lemma 2.3

Let C be a Petri curve of genus g and L a line bundle with $d_L={\text {gon}}(C)$ and $h^0(L)=2$. Then

• (1) L is generated, in other words, the evaluation map

  $$\begin{aligned} H^0(L)\otimes \mathcal {O}_C\rightarrow L \end{aligned}$$

  is surjective;

• (2) if g is even, $h^0(L\otimes L)=3$;

• (3) if g is odd, $h^0(L\otimes L)=4$.

Proof

(1) is obvious, since otherwise $h^0(L(-p))=2$ for some $p\in C$, contradicting the definition of ${\text {gon}}(C)$. For (2) and (3), see [9, Lemma 2.10]. $\square$

Lemma 2.4

There exist non-split exact sequences

$$\begin{aligned} 0\longrightarrow E_1\longrightarrow E\longrightarrow E_2\longrightarrow 0 \end{aligned}$$

of vector bundles for which all sections of $E_2$ lift to sections of E if and only if the multiplication map

$$\begin{aligned} m:H^0(E_2)\otimes H^0(E_1^*\otimes K_C)\longrightarrow H^0(E_2\otimes E_1^*\otimes K_C) \end{aligned}$$

fails to be surjective. Such extensions are classified up to isomorphism by ${\mathbb P}(({\text {Coker}}m)^*)$.

Proof

The extensions for which all sections lift are classified by the kernel of the natural map

$$\begin{aligned} H^1(E_2^*\otimes E_1)\longrightarrow {\text {Hom}}(H^0(E_2),H^1(E_1)). \end{aligned}$$

The map m is the dual of this map. $\square$

The following lemma is undoubtedly well known, but I have been unable to locate a reference.

Lemma 2.5

Let F be a vector bundle on C with $h^1(F)\ge r$ for some positive integer r. Then, for $\tau$ a general torsion sheaf of length $t\le r$ and

$$\begin{aligned} 0\longrightarrow F\longrightarrow E\longrightarrow \tau \longrightarrow 0 \end{aligned}$$

(2.3)

a general extension of $\tau$ by F, $H^0(E)=H^0(F)$.

Proof

By induction, it s clearly sufficient to prove this when $t=1$. In this case $\tau =\mathbb {C}_p$ for a general point $p\in C$. Dualising (2.3) and tensoring by $K_C$, we obtain an exact sequence

$$\begin{aligned} 0\longrightarrow E^*\otimes K_C\longrightarrow F^*\otimes K_C\longrightarrow \mathbb {C}_p\longrightarrow 0. \end{aligned}$$

Now note that $h^0(F^*\otimes K_C)\ne 0$ and for general p and the general homomorphism $F^*\otimes K_C\rightarrow \mathbb {C}_p$, the map $H^0(F^*\otimes K_C)\rightarrow \mathbb {C}_p$ is non-zero. It follows that $h^0(E^*\otimes K_C)=h^0(F^*\otimes K_C)-1$ and so $h^0(E)=h^0(F)$, giving the required result. $\square$

Finally, we recall that a coherent system on C of type (n, d, k) is a pair (E, V) consisting of a vector bundle E of rank n and degree d and a subspace V of $H^0(E)$ of dimension k. There is a concept of $\alpha$-stability for coherent systems for $\alpha \in \mathbb {R}$ and moduli spaces $G(\alpha ;n,d,k)$ and $\widetilde{G}(\alpha ;n,d,k)$ exist. (For basic information on this construction, see [4].) The definition of $\alpha$-stability depends on the $\alpha$-slope of (E, V) defined by $\mu _\alpha (E,V):=\frac{d+\alpha k}{n}$.

3 A reducible Brill–Noether locus

In this section, we prove our first main theorem. While the key case is for curves of genus 6, the theorem in fact holds for infinitely many values of the genus.

Lemma 3.1

Let C be a general curve of genus $g=\lambda (2\lambda -1)$ for $\lambda \in \mathbb {Z}$, $\lambda \ge 2$. Then $B(1,g-\lambda ,\lambda )$ is a finite set of cardinality

$$\begin{aligned} (\lambda (2\lambda -1))!\prod _{i=0}^{\lambda -1}\frac{i!}{(2\lambda +i-1)!}. \end{aligned}$$

Moreover, if $L\in B(1,g-\lambda ,\lambda )$, then L is generated and $h^0(L)=\lambda$.

Proof

This follows by classical Brill–Noether theory from the fact that $\beta (1,g-\lambda ,\lambda )=0$ (see [1, p.211 formula (1.2)] for the formula for the cardinality). $\square$

For C as in Lemma 3.1, a simple calculation gives

$$\begin{aligned} \beta (2,2g-2,2\lambda )=\beta (2,K_C,2\lambda )=4\lambda (\lambda -1)-3. \end{aligned}$$

(3.1)

Theorem 3.2

Let C be a general curve of genus $g=\lambda (2\lambda -1)$ for $\lambda \in \mathbb {Z}$, $\lambda \ge 2$. Then $B(2,K_C,2\lambda )$ has pure dimension $4\lambda (\lambda -1)-3$ and is smooth outside the non-empty locus $B(2,K_C,2\lambda +1)$. Moreover $B(2,2g-2,2\lambda )$ has at least one irreducible component of dimension $4\lambda (\lambda -1)-3$ which is not contained in $B(2,K_C,2\lambda )$.

Proof

The fact that $B(2,K_C,2\lambda )$ and $B(2,K_C,2\lambda +1)$ are both non-empty is proved in [3] for $\lambda =2$ and in [18, 21] for $\lambda \ge 3$. The rest of the first assertion is proved in [22].

To obtain bundles in $B(2,2g-2,2\lambda )$ which do not have determinant $K_C$, we consider exact sequences

$$\begin{aligned} 0\longrightarrow L_1\oplus L_2\longrightarrow E\longrightarrow \mathbb {C}_{p_1}\oplus \cdots \oplus \mathbb {C}_{p_{2\lambda -2}}\longrightarrow 0, \end{aligned}$$

(3.2)

where $L_1$, $L_2$ are distinct elements of $B(1,g-\lambda ,\lambda )$ (these exist by Lemma 3.1) and the $p_j$ are distinct points of C. The general such extension gives rise to a stable bundle E by [11, Théorème A-5]; moreover the homomorphism $E^*\rightarrow L_i^*$ obtained by dualising (3.2) is surjective. By Riemann-Roch, $h^1(L_i)=2\lambda -1$, so Lemma 2.5 implies that, for a general choice of $p_j$ and (3.2),

$$\begin{aligned}H^0(E)=H^0(L_1)\oplus H^0(L_2).\end{aligned}$$

Moreover, for general $p_j$, we have

$$\begin{aligned} h^0(L_i^*(-p_1-\cdots -p_{2\lambda -2})\otimes K_C)=1. \end{aligned}$$

We now show that, for a generic choice of the $p_j$, the Petri map of E is injective. This will prove that E belongs to a unique irreducible component $B_0$ of dimension $4\lambda (\lambda -1)-3$ [see (3.1)], which is evidently not contained in $B(2,K_C,4)$. In fact, the Petri map

$$\begin{aligned} H^0(E)\otimes H^0(E^*\otimes K_C)\longrightarrow H^0(E\otimes E^*\otimes K_C) \end{aligned}$$

splits into

$$\begin{aligned} \mu _1:H^0(L_1)\otimes H^0(E^*\otimes K_C)\longrightarrow H^0(L_1\otimes E^*\otimes K_C) \end{aligned}$$

and

$$\begin{aligned} \mu _2:H^0(L_2)\otimes H^0(E^*\otimes K_C)\longrightarrow H^0(L_2\otimes E^*\otimes K_C). \end{aligned}$$

It is sufficient to prove that both these maps are injective. Now we have a commutative diagram

$$\begin{aligned} \begin{array}{ccc} H^0(L_1)\otimes H^0(E^*\otimes K_C)&{}\mathop {\longrightarrow }\limits ^{\mu _1}&{}H^0(L_1\otimes E^*\otimes K_C)\\ \Big \downarrow &{}&{}\Big \downarrow \\ H^0(L_1)\otimes H^0(L_1^*\otimes K_C)&{}\longrightarrow &{} H^0(K_C), \end{array} \end{aligned}$$

where the vertical arrows are induced by the homomorphism $E^*\rightarrow L_1^*$. The lower horizontal map is injective since C is Petri, so

$$\begin{aligned} {\text {Ker}}\mu _1\subset H^0(L_1)\otimes {\text {Ker}}(H^0(E^*\otimes K_C)\longrightarrow H^0(L_1^*\otimes K)). \end{aligned}$$

Since $E^*\rightarrow L_1^*$ is surjective,

$$\begin{aligned}{\text {Ker}}(H^0(E^*\otimes K_C)\longrightarrow H^0(L_1^*\otimes K_C))=H^0(L_2^*(-p_1-\cdots -p_{2\lambda -2})\otimes K_C). \end{aligned}$$

Moreover, $h^0(L_2^*(-p_1-\cdots -p_{2\lambda -2})\otimes K_C)=1$, so

$$\begin{aligned} \mu _1|H^0(L_1)\otimes H^0(L_2^*(-p_1-\cdots p_{2\lambda -2})\otimes K_C) \end{aligned}$$

is injective. Hence ${\text {Ker}}\mu _1=0$ and $\mu _1$ is injective. The same argument applies to $\mu _2$, completing the proof that $B_0$ has dimension $4\lambda (\lambda -1)-3$. $\square$

Remark 3.3

The fact that $B(2,2g-2,2\lambda )$ has a component of dimension $4\lambda (\lambda -1)-3$ is proved in [20]. The argument in the proof above, using [11], is more precise and shows that there is a component not contained in $B(2,K_C,2\lambda )$. On the other hand, it is not proved in [11] that the component $B_0$ has dimension $4\lambda (\lambda -1)-3$, so we need to prove this directly.

Remark 3.4

See [7, Theorems 5.13, 5.17] for many examples of non-empty Brill–Noether loci. Our examples do not satisfy the hypotheses in these theorems.

4 Genus 6

In genus 6, one can prove a good deal more. In this case, we have

$$\begin{aligned} \beta (2,10,5)=-4,\ \beta (2,K_C,5)=0,\ \beta (2,10,4)=\beta (2,K_C,4)=5. \end{aligned}$$

Theorem 4.1

Let C be a general curve of genus 6. Then $\widetilde{B}(2,10,k)=\emptyset$ for $k\ge 6$. Moreover $B(2,10,5)=\widetilde{B}(2,10,5)=B(2,K_C,5)$ consists of a single point $E_{2,10,5}$ and $E_{2,10,5}$ is generated.

Proof

Let E be a semistable bundle of rank 2 and degree 10. Since ${\text {Cliff}}_2(C)={\text {Cliff}}(C)=2$ by (2.1), $h^0(E)\le 5$. This proves the first statement. By classical Brill–Noether theory, $B(1,5,3)=\emptyset$; hence $B(2,10,5)=\widetilde{B}(2,10,5)$. Note also that $B(2,K_C,5)$ is non-empty by [3] and is smooth of dimension 0 by [22]. Now suppose that $E\in B(2,10,5)$. By Corollary 2.2, there exists an exact sequence

$$\begin{aligned} 0\longrightarrow L\longrightarrow E\longrightarrow L''\longrightarrow 0, \end{aligned}$$

(4.1)

where $d_L=4$, $h^0(L)=2$, $d_{L''}=6$, $h^0(L'')=3$ and all sections of $L''$ lift to E. Tensoring (4.1) by L and taking global sections, we get

$$\begin{aligned} h^0(L''\otimes L)\ge h^0(E\otimes L)-h^0(L\otimes L). \end{aligned}$$

Since C is Petri, by Lemma 2.3, $h^0(L\otimes L)=3$, while the sequence

$$\begin{aligned} 0\longrightarrow E\otimes L^*\longrightarrow E\otimes H^0(L)\longrightarrow E\otimes L\longrightarrow 0 \end{aligned}$$

gives

$$\begin{aligned} h^0(E\otimes L)\ge 2h^0(E)-h^0(E\otimes L^*)\ge 9, \end{aligned}$$

since $E\otimes L^*$ is stable of rank 2 and degree 2, so that $h^0(E\otimes L^*)\le 1$ by [5, Theorem B]. So

$$\begin{aligned} h^0(L''\otimes L)\ge 9-3=6. \end{aligned}$$

Since $d_{L''\otimes L}=10$, this implies that $\det E=L''\otimes L\simeq K_C$. It follows that every $E\in B(2,10,5)$ can be expressed in the form (4.1) with $L''=L^*\otimes K_C$. By classical Brill–Noether theory, there are five choices for L [1, p.211 formula (1.2)]. However, since C can be embedded in a K3 surface S for which ${\text {Pic}}S$ is generated by the class of C, these five line bundles all determine the same bundle $E_{2,10,5}$ (see the paragraph following the statement of [23, Théorème 0.1]).

Note finally that, if $E_{2,10,5}$ is not generated, then an elementary transformation yields a bundle in B(2, 9, 5), contradicting the fact that ${\text {Cliff}}_2(C)=2$. $\square$

Remark 4.2

From the definition of ${\text {Cliff}}_2(C)$, it follows that any bundle computing ${\text {Cliff}}_2(C)$ has either degree 8 and $h^0=4$ or degree 10 and $h^0=5$. Hence, by Theorem 4.1 and [9, Proposition 5.10], the only such bundles are strictly semistable bundles of degree 8 with $h^0=4$ and the stable bundle $E_{2,10,5}$.

Corollary 4.3

For all $\alpha >0$,

$$\begin{aligned} G(\alpha ;2,10,5)=\{(E,H^0(E))|E=E_{2,10,5}\}. \end{aligned}$$

Proof

If $E=E_{2,10,5}$, then $h^0(E)=5$ by Theorem 4.1. Moreover, E is stable, so any line subbundle has degree $\le 4$ and hence $h^0\le 2$. Hence $(E,H^0(E))\in G(\alpha ;2,10,5)$ for all $\alpha >0$.

Conversely, suppose $(E,V)\in G(\alpha ;2,10,5)$. If E is not stable, then E admits a line subbundle L of degree $\ge 5$. Hence $d_{E/L}\le 5$ and $h^0(E/L)\le 2$. It follows that $\dim (V\cap H^0(L))\ge 3$, contradicting the $\alpha$-stability of (E, V). $\square$

The following proposition gives an example of a non-empty rank-3 Brill–Noether locus on C with negative Brill–Noether number.

Proposition 4.4

Let C be a general curve of genus 6. Then $B(3,10,k)=\emptyset$ for $k\ge 6$. Moreover B(3, 10, 5) consists of a single point $E_{3,10,5}$ and there exists a short exact sequence

$$\begin{aligned} 0\longrightarrow E_{3,10,5}^*\longrightarrow \mathcal {O}_C^{\oplus 5}\longrightarrow E_{2,10,5}\longrightarrow 0. \end{aligned}$$

(4.2)

Furthermore, for all $\alpha >0$,

$$\begin{aligned} G(\alpha ;3,10,5)=\{(F,H^0(F))|F=E_{3,10,5}\}. \end{aligned}$$

Proof

By [12, Theorem 2.1] or [8, Proposition 3.5], we have ${\text {Cliff}}_3(C)=2$. Hence any stable bundle F of rank 3 and degree 10 has $h^0(F)\le 5$.

Now recall that $E:=E_{2,10,5}$ is generated and $h^0(E)=5$. We define a bundle F of rank 3 and degree 10 (hence slope $\mu (F)=\frac{10}{3}$) by the exact sequence

$$\begin{aligned} 0\longrightarrow F^*\longrightarrow H^0(E)\otimes \mathcal {O}_C\longrightarrow E\longrightarrow 0. \end{aligned}$$

(4.3)

Dualising, we obtain

$$\begin{aligned} 0\longrightarrow E^*\longrightarrow H^0(E)^*\otimes \mathcal {O}_C\longrightarrow F\longrightarrow 0. \end{aligned}$$

Since $h^0(E^*)=0$, it follows that $h^0(F)=5$. It remains to prove that F is stable.

If L is a quotient line bundle of F, then L is generated and, since $L^*\subset F^*$ and $h^0(F^*)=0$ by (4.3), $h^0(L^*)=0$. Hence $h^0(L)\ge 2$ and $d_L\ge 4>\mu (F)$. Now suppose that G is a stable rank-2 quotient bundle of F. Then G is generated by the image V of $H^0(E)^*$ in $H^0(G)$ and $h^0(G^*)=0$, so $\dim V\ge 3$. Let K be the kernel of the canonical surjection $V\otimes \mathcal {O}_C\rightarrow G$. If $\dim V=3$, then K is a line bundle with $h^0(K^*)\ge 3$, so $d_G=-d_K\ge 6$. On the other hand, the homomorphism $E^*\rightarrow K$ is non-zero, since otherwise $E^*$ would map into a proper direct factor of $H^0(E)^*\otimes \mathcal {O}_C$, a contradiction. Hence $K^*\subset E$ and $-d_K\le 4$ by stability of E. This is a contradiction. It follows that $\dim V\ge 4$ and hence $d_G\ge 8$ since ${\text {Cliff}}(G)\ge {\text {Cliff}}_2(C)=2$. Thus F is stable. We define $E_{3,10,5}:=F$, so that (4.3) becomes (4.2).

Conversely, let $F\in B(3,10,5)$. We have already observed that $h^0(F)=5$. If F is not generated, then, applying an elementary transformation, there exists a semistable bundle of rank 3 and degree 9 with $h^0=5$; this contradicts the fact that ${\text {Cliff}}_3(C)=2$. We can therefore define a bundle G of rank 2 and degree 10 by the exact sequence

$$\begin{aligned} 0\longrightarrow G^*\longrightarrow H^0(F)\otimes \mathcal {O}_C\longrightarrow F\longrightarrow 0. \end{aligned}$$

Dualising, we have

$$\begin{aligned} 0\longrightarrow F^*\longrightarrow H^0(F)^*\otimes \mathcal {O}_C\longrightarrow G\longrightarrow 0. \end{aligned}$$

(4.4)

Now suppose L is a quotient line bundle of G and let V be the image of $H^0(F)^*$ in $H^0(L)$. Arguing as above, we have $\dim V\ge 2$. If $\dim V\ge 3$, then $d_L\ge 6>\mu (G)$. If $\dim V=2$, then $d_L\ge 4$. Moreover, by stability of F, the kernel of the surjection $V\otimes \mathcal {O}_C\rightarrow L$ is a line bundle of degree $\ge -3$, so $d_L=-d_K\le 3$. This gives a contradiction, so G is stable and hence $G\simeq E_{2,10,5}$. So (4.4) becomes (4.2) and $F\simeq E_{3,10,5}$.

Finally, if $F=E_{3,10,5}$, it is clear that $(F,H^0(F))\in G(\alpha ;3,10,5)$ for all $\alpha >0$. Conversely, if $(F,V)\in G(\alpha ;3,10,5)$ with F not stable, then F has either a line subbundle L of degree $\ge 4$ or a rank-2 subbundle G of degree $\ge 7$. In the first case, we must have $h^0(L)\le 1$, so $h^0(F/L)\ge 4$, which is impossible. In the second case, $h^0(G)\le 3$, so $h^0(F/G)\ge 2$, which again is impossible. So F is stable and hence $F\simeq E_{3,10,5}$. $\square$

Remark 4.5

The fact that $B(3,K_C,5)$ has dimension zero shows that Osserman’s lower bound for the dimension of the Brill–Noether locus for bundles of determinant $K_C$ when $r=3$, $k=5$ [17, Theorem 1.1(III)] can be sharp.

Remark 4.6

When $g=6$, there is a method of constructing bundles in B(2, 10, 4) with determinant different from $K_C$ which differs from that that in the proof of Theorem 3.2 (this is similar to the construction described in more generality in [7, Theorem 5.13], but our examples do not satisfy the hypotheses of this theorem). Consider non-trivial extensions

$$\begin{aligned} 0\longrightarrow L\longrightarrow E\longrightarrow L''\longrightarrow 0, \end{aligned}$$

(4.5)

where $L\in B(1,4,2)$ and $L''$ is a generated line bundle of degree 6 with $h^0(L'')=2$. If $h^0(E)=4$, then E is generated and stable. In fact, if E were not stable, it would admit a line subbundle M of degree 5 with $h^0(M)=2$. But then there would exist a non-zero homomorphism $M\rightarrow L''$; since $h^0(M)=h^0(L'')$, this implies that $L''$ is not generated. To obtain a bundle E with $h^0(E)=4$ in (4.5), we require all sections of $L''$ to lift to sections of E. For this, by Lemma 2.4, we need the multiplication map

$$\begin{aligned} H^0(L'')\otimes H^0(L^*\otimes K_C)\longrightarrow H^0(L''\otimes L^*\otimes K_C) \end{aligned}$$

(4.6)

to fail to be surjective. Calculating dimensions, the LHS of (4.6) has dimension 6, while the RHS has dimension 7, so surjectivity does indeed fail. Moreover, by the base-point free pencil trick, the kernel of (4.6) is $H^0(L^*\otimes L''^*\otimes K_C)$, which is zero since $L''\not \simeq L_1^*\otimes K_C$. It follows that the cokernel of (4.6) has dimension 1, so, for any given $L''$, the extension is unique up to isomorphism. By classical Brill–Noether theory, the bundles $L''$ form an irreducible variety of dimension $\beta (1,6,2)=4$. Hence all such extensions belong to a single irreducible component $B_1$ of B(2, 10, 4). Since there are five possible choices for L (see Lemma 3.1), we obtain a possible total of five irreducible components in this way. Similarly there are ten possibilities for the component $B_0$ in Theorem 3.2. If we could prove that $B_1=B_0$ (or equivalently that the bundles E in (3.2) belong to $B_1$), then these 15 components would all coincide.

Proposition 4.7

For all $\alpha >0$,

$$\begin{aligned} G(\alpha ;2,10,4)=\{(E,V)|E\in B(2,10,4), V\subset H^0(E), \dim V=4\} \end{aligned}$$

and

$$\begin{aligned} \widetilde{G}(\alpha ;2,10,4)=\{[(E,V)]|[E]\in \widetilde{B}(2,10,4), V\subset H^0(E), \dim V=4\}. \end{aligned}$$

Proof

The proof is similar to that of Corollary 4.3, the key point being that any line bundle L with $d_L\le 5$ has $h^0\le 2$. $\square$

5 Genus 5

Let C be a general curve of genus 5. Since ${\text {Cliff}}_2(C)=2$, it follows that $h^0(E)\le 4$ for any semistable bundle of rank 2 and degree $\le 2g-2=8$. Moreover the bundles which compute ${\text {Cliff}}_2(C)$ are precisely the semistable bundles of rank 2 and degree 8 with $h^0=4$. Note that

$$\begin{aligned} \beta (2,8,4)=1,\ \beta (2,K_C,4)=2. \end{aligned}$$

Proposition 5.1

Let C be a general curve of genus 5. Then $B(2,K_C,4)$ is smooth of dimension 2 and consists of the stable bundles with $h^0(E)=4$ which can be expressed in the form

$$\begin{aligned} 0\rightarrow M\rightarrow E\rightarrow M^*\otimes K_C\rightarrow 0, d_M=2, h^0(M)=1, \end{aligned}$$

(5.1)

with all sections of $K_C\otimes M^*$ lifting to E. Moreover, $B(2,K_C,4)$ is irreducible and

$$\begin{aligned} \widetilde{B}(2,8,4)=\overline{B(2,K_C,4)}\cup \{[L\oplus L']|L,L'\in B(1,4,2)\}. \end{aligned}$$

(5.2)

In particular, $B(2,8,4)=B(2,K_C,4)$.

Proof

The fact that $B(2,K_C,4)$ is smooth of dimension 2 follows from [3] and [22]. A bundle $E\in B(2,K_C,4)$ cannot contain a subpencil since $B(1,3,2)=\emptyset$; it follows from [9, Lemma 5.6] that E can be expressed in the form (5.1). Now consider the multiplication map

$$\begin{aligned} m: H^0(M^*\otimes K_C)\otimes H^0(M^*\otimes K_C)\longrightarrow H^0(M^{*2}\otimes K_C^2). \end{aligned}$$

This factors through $S^2H^0(M^*\otimes K_C)$, so $\dim ({\text {Ker}}m)\ge 3$. Now $M^*\otimes K_C$ is generated and the kernel F of its evaluation map has rank 2. If L is a quotient line bundle of $F^*$, then L is generated and $h^0(L^*)=0$, so $d_L \ge 4$; since $d_{F^*}=6$, this proves that F is stable. Moreover ${\text {Ker}}m\simeq H^0(F\otimes M^*\otimes K_C)\simeq H^0(F^*)$. Since ${\text {Cliff}}_2(C)=2$, it follows that $\dim ({\text {Ker}}m)\le 3$. Hence $\dim ({\text {Ker}}m)=3$ and $\dim ({\text {Coker}}m)=2$. It follows from Lemma 2.4 that the isomorphism classes of non-trivial extensions (5.1), for which all sections of $M^*\otimes K_C$ lift, form a $\mathbb {P}^1$-fibration W over B(1, 2, 1). Moreover, W is irreducible and the open subset for which E is stable maps surjectively to $B(2,K_C,4)$, which is therefore irreducible. For (5.2), see [9, Proposition 5.7]. $\square$

Remark 5.2

Mukai states that $\overline{B(2,K_C,4)}\simeq {\mathbb P}^2$ (see the table in [15, section 4]). Since $\beta (1,4,2)=1$, it follows from (5.2) that all components of $\widetilde{B}(2,8,4)$ have dimension 2. In particular, $\widetilde{B}(2,8,4)$ has no component of dimension $\beta (2,8,4)$. This does not contradict [20] since $\beta (1,4,2)=1$. Moreover $\overline{B(2,8,4)}\ne \widetilde{B}(2,8,4)$.

Proposition 5.3

For all $\alpha >0$,

$$\begin{aligned} G(\alpha ;2,8,4)=\{(E,H^0(E))|E\in B(2,8,4)\} \end{aligned}$$

and

$$\begin{aligned} \widetilde{G}(\alpha ;2,8,4)=\{[(E,H^0(E))]|[E]\in \widetilde{B}(2,8,4)\}. \end{aligned}$$

Proof

The proof is similar to that of Corollary 4.3. $\square$

6 Bundles computing the Clifford index

By [2, Proposition 11], the bundles computing ${\text {Cliff}}_2(C)$ on a general curve of genus g have either $h^0=4$ or degree $2g-2$ and $h^0=2+\left\lceil \frac{g-1}{2}\right\rceil$. This substantially improves [9, Theorem 7.4]. Bakker and Farkas prove further that the second possibility does not arise when g is even and $g\ge 10$ [2, Theorem 4] and conjecture that the same is true for g odd, $g\ge 15$. In fact, for g odd, $g\ge 15$, $\widetilde{B}(2,K_C,\frac{g+3}{2})=\emptyset$ and all $E\in B(2,2g-2,\frac{g+3}{2})$ can be expressed in the form (2.2) with $L\not \simeq L'$ [2, Remark 13], but it is not known whether any such exist.

For $g\le 5$, there are no semistable bundles of degree $\le 2g-2$ with $h^0>4$, while, for $g=6$, we have already answered the question in Remark 4.2. It remains to consider $g=7,8,9,11,13$.

Example 6.1

Let C be a general curve of genus 7. Then ${\text {Cliff}}_2(C)=3$ and we have

$$\begin{aligned} \beta (2,12,5)=0,\ \beta (2,K_C,5)=3. \end{aligned}$$

It is stated but not formally proved in [3] that $B(2,K_C,5)$ is non-empty. This is proved in [21] and [9, Proposition 7.7]. The more precise statement that $B(2,K_C,5)$ is a Fano 3-fold of Picard number 1 and genus 7 is [15, Theorem 8.1] (see also [13, Theorem 4.13]); this holds for all non-tetragonal curves of genus 7. By classical Brill–Noether theory, $B(1,6,3)=\emptyset$, so $\widetilde{B}(2,12,5)=B(2,12,5)$. Moreover, by Corollary 2.2, any bundle $E\in B(2,12,5)\setminus B(2,K_C,5)$ can be expressed in the form (2.2) with $L,L'\in B(1,5,2)$, $L\not \simeq L'$. It is easy to see that any bundle given by a non-trivial extension (2.2), for which all sections of $L'^*\otimes K_C$ lift, is stable, but it is not known whether such extensions exist.

Example 6.2

Let C be a general curve of genus 8. Then ${\text {Cliff}}_2(C)=3$ and we have

$$\begin{aligned}\beta (2,14,6)=-7,\ \beta (2,K_C,6)=0.\end{aligned}$$

Again, it is stated in [3] and proved in [9, Proposition 7.2] that $B(2,K_C,6)$ is non-empty. By classical Brill–Noether theory, $B(1,7,3)=\emptyset$, so $\widetilde{B}(2,14,6)=B(2,14,6)$. Furthermore, $B(2,K_C,6)$ is finite by [22] and consists of a single point by [23] (see also [13, Theorem 4.14], where the corresponding stable bundle is described). Finally, $B(2,14,6)=B(2,K_C,6)$ by [2, Proposition 11].

Example 6.3

Let C be a general curve of genus 9. Then ${\text {Cliff}}_2(C)=4$ and we have

$$\begin{aligned} \beta (2,16,6)=-3,\ \beta (2,K_C,6)=3. \end{aligned}$$

It is stated in [3] and proved in [21] and [9, Proposition 7.8] that $B(2,K_C,6)$ is non-empty. Moreover (see [9, Theorem 7.4(1)]), there exist strictly semistable bundles $Q\oplus Q'$ of degree 16 with $h^0=6$. In fact, since there are just 42 line bundles of degree 8 with $h^0=3$, there are 21 points of $\widetilde{B}(2,K_C,6)$ corresponding to strictly semistable bundles. In fact, Mukai [13] asserts that $\widetilde{B}(2,K_C,6)$ is a quartic 3-fold in $\mathbb {P}^4$ with 21 singular points. Finally, $\widetilde{B}(2,16,6)=\widetilde{B}(2,K_C,6)$ by [2, Proposition 11].

Example 6.4

Let C be a general curve of genus 11. Then ${\text {Cliff}}_2(C)=5$ and we have

$$\begin{aligned} \beta (2,20,7)=-8,\ \beta (2,K_C,7)=2. \end{aligned}$$

It is stated in [3] and proved in [9, Remark 7.5] that $\widetilde{B}(2,K_C,7)$ is non-empty. More precisely, Mukai [14, Theorem 1] shows that $\widetilde{B}(2,K_C,7)$ is a smooth K3 surface of genus 11. By classical Brill–Noether theory, $B(1,10,4)=\emptyset$, so $\widetilde{B}(2,20,7)=B(2, 20,7)$. Finally, $B(2,20,7)=B(2,K_C,7)$ by [2, Proposition 11].

Example 6.5

Let C be a general curve of genus 13. Then ${\text {Cliff}}_2(C)=6$ and we have

$$\begin{aligned} \beta (2,24,8)=-15,\ \beta (2,K_C,8)=0. \end{aligned}$$

By classical Brill–Noether theory, $B(1,12,4)=\emptyset$, so $\widetilde{B}(2,24,8)=B(2,24,8)$, but questions of existence are not clear. In fact, $g=13$, $k=8$ is the first case in which the existence part of the Bertram–Feinberg–Mukai conjecture

$$\begin{aligned} \beta (2,K_C,k)\ge 0\mathop {\Longrightarrow }\limits ^{?} B(2,K_C,k)\ne \emptyset \end{aligned}$$

is unresolved. The arguments of [3, 10, 15, 21, 23, 24] all fail, although [10, Theorem 3.5] (see also [15]) does reduce the problem to a purely combinatorial one. Moreover, by [23, Proposition 4.3] and Lemma 2.4, if $E\in B(2,K_C,8)$, then E does not admit a subpencil and is expressible in the form

$$\begin{aligned} 0\longrightarrow L\longrightarrow E\longrightarrow L^*\otimes K_C\longrightarrow 0 \end{aligned}$$

with $d_L\ge 6$ by [16]. We must have $h^0(L)\le 1$, so

$$\begin{aligned} h^0(E)\le h^0(L)+h^0(L^*\otimes K_C)\le 1+(13-d_L)\le 8. \end{aligned}$$

The only way to achieve equality is with $d_L=6$ and $h^0(L)=1$ and then all sections of $L^*\otimes K_C$ must lift to E. On the other hand, if $E\in B(2,24,8)\setminus B(2,K_C,8)$, then E is expressible in the form (2.2) with $L,L'\in B(1,8,2)$, $L\not \simeq L'$. It is not clear whether there are any L, $L'$ for which there exist non-trivial extensions of this form for which all sections of $L'^*\otimes K_C$ lift, but, if these do exist, E is necessarily stable. Note that, in this case, [2, Proposition 11] does not apply since the general curve of genus 13 cannot be embedded in a K3 surface.