Skip to main content
SpringerLink
Log in

Non-Standard Finite Difference Schemes for Solving Variable-Order Fractional Differential Equations

  • Original Research
  • Published:
Differential Equations and Dynamical Systems Aims and scope Submit manuscript

Abstract

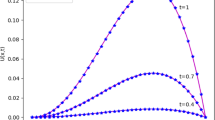
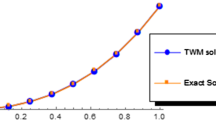
A non-standard finite difference (NSFD) methodology of Mickens is a popular method for the solution of differential equations. In this paper, we discusses how we can generalize NSFD schemes for solving variable-order fractional problems. The variable-order fractional derivatives are described in the Riemann–Liouville and Grünwald–Letinkov sense. Special attention is given to the Grünwald–Letinkov definition which is used to approximate the variable-order fractional derivatives. Some applications of the variable-order fractional in viscous-viscoelasticity oscillator model and chaotic financial system are included to demonstrate the validity and applicability of the proposed technique.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Agarwal, P., Choi, J.: Fractional calculus operators and their image formulas. J. Korean Math. Soc. 53(5), 1183–1210 (2016)

    Article  MathSciNet  Google Scholar 

  2. Agarwal, P., Karimov, E., Mamchuev, M., Ruzhansky, M.: On boundary-value problems for a partial differential equation with Caputo and Bessel operators. arXiv:1611.01624

  3. Agarwal, P., Nieto, J.J.: Some fractional integral formulas for the Mittag–Leffler type function with four parameters. Open Phys. 13(1), 537–546 (2015)

    MathSciNet  MATH  Google Scholar 

  4. Ayala, R., Tuesta, A.: Introduction to the concepts and applications of fractional and variable order differential calculus (2007). http://www3.nd.edu/msen/Teaching/UnderRes/

  5. Bagley, R.L., Torvik, P.J.: A theoretical basis for the application of fractional calculus. J. Rheol. 27(3), 201–210 (1983)

    Article  Google Scholar 

  6. Chen, W.: Nonlinear dynamics and chaos in a fractional-order financial system. Chaos Solitons Fract. 36, 1305–1314 (2008)

    Article  Google Scholar 

  7. Coimbra, C.F.M.: Mechanics with variable-order differential operators. Ann. Phys. (Leipzig) 12(11–12), 692–703 (2003)

    Article  MathSciNet  Google Scholar 

  8. Kempfle, S., Schäfer, I., Beyer, H.: Fractional Calculus via Functional calculus: theory and applications. Nonlinear Dyn. 29, 99–127 (2002)

    Article  MathSciNet  Google Scholar 

  9. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam (2006)

    MATH  Google Scholar 

  10. Kiymaz, I.O., Cetinkaya, A., Agarwal, P.: An extension of Caputo fractional derivative operator and its applications. J. Nonlinear Sci. Appl. 9(6), 3611–3621 (2016)

    Article  MathSciNet  Google Scholar 

  11. Lorenzo, C.F., Hartley, T.T.: Variable order and distributed order fractional operators. Nonlinear Dyn. 29(1–4), 57–98 (2002)

    Article  MathSciNet  Google Scholar 

  12. Loverro, A.: Fractional calculus: history, definitions and applications for the engineer (2004). http://www3.nd.edu/msen/Teaching/UnderRes/

  13. Ma, J., Chen, Y.: Study for the bifurcation topological structure and the global complicated character of a kind of nonlinear finance system (I). Appl. Math. Mech. (English Ed) 22, 1240–1251 (2001)

    Article  MathSciNet  Google Scholar 

  14. Meerschaert, M.M., Tadjeran, C.: Finite difference approximations for fractional advection-dispersion flow equations. J. Comput. Appl. Math. 172(1), 65–77 (2004)

    Article  MathSciNet  Google Scholar 

  15. Metzler, R., Klafter, J.: From stretched exponential to inverse power-law: fractional dynamics, ColeCole relaxation processes, and beyond. J. Non-Cryst. Solids 305, 81–87 (2002)

    Article  Google Scholar 

  16. Mickens, R.E.: Nonstandard finite difference models of differential equations. World Scientific, Singapore (1994)

    MATH  Google Scholar 

  17. Nagy, A.M., Sweilam, N.H.: An efficient method for solving fractional Hodgkin–Huxley model. Phys. Lett. A 378, 1980–1984 (2014)

    Article  MathSciNet  Google Scholar 

  18. Mickens, R.E.: Nonstandard finite difference schemes for reactions-diffusion equations. Numer. Methods Partial Differ. Equ. Fractals 15, 201–214 (1999)

    Article  MathSciNet  Google Scholar 

  19. Mickens, R.E.: A nonstandard finite difference scheme for a fisher PDF having nonlinear diffusion. Comput. Math. Appl. 45, 429–436 (2003)

    Article  MathSciNet  Google Scholar 

  20. Mickens, R.E.: A nonstandard finite-difference scheme for the Lotka-Volterra system. Appl. Numer. Math. 45(2–3), 309–314 (2003)

    Article  MathSciNet  Google Scholar 

  21. Miller, K.S., Ross, B.: An introduction to the fractional calculus and fractional differential equations. Wiley, New York (1993)

    MATH  Google Scholar 

  22. Ongun, M.Y., Arslan, D., Garrappa, R.: Nonstandard finite difference schemes for a fractional-order Brusselator system. Advances in difference equations, vol. 2013, Article 102 (2013). doi:10.1186/1687-1847-2013-102

  23. Podlubny, I.: Fractional Differential Equations. Academic, New York (1999)

    MATH  Google Scholar 

  24. Ramirez, L.E.S., Coimbra, C.F.M.: On the variable order dynamics of the nonlinear wake caused by a sedimenting particle. Phys. D 240(13), 1111–1118 (2011)

    Article  MathSciNet  Google Scholar 

  25. Samko, S.G.: Fractional integration and differentiation of variable order. Anal. Math. 21(3), 213–236 (1995)

    Article  MathSciNet  Google Scholar 

  26. Soon, C.M., Coimbra, C.F.M., Kobayashi, M.H.: The variable viscoelasticity oscillator. Ann. Phys. 14(6), 378–389 (2005)

    Article  Google Scholar 

  27. Sweilam, N.H., Nagy, A.M., Assiri, T.A., Ali, N.Y.: Numerical simulations for variable-order fractional non-linear delay differential equations. J. Fract. Calc. Appl. 6(1), 71–82 (2015)

    MathSciNet  Google Scholar 

  28. Sweilam, N.H., Nagy, A.M., El-Sayed, A.A.: On the numerical solution of space fractional order diffusion equation via shifted Chebyshev polynomials of the third kind. J. King Saud Univ. Sci. 28(1), 41–47 (2016)

    Article  Google Scholar 

  29. Sweilam, N.H., Nagy, A.M., El-Sayedl, A.A.: Second kind shifted Chebyshev polynomials for solving space fractional order diffusion equation. Chaos, Solitons Fract. 73, 141–147 (2015)

    Article  MathSciNet  Google Scholar 

  30. Sweilam, N.H., Nagy, A.M., El-Sayed, A.A.E.: Numerical approach for solving space fractional order diffusion equations using shifted Chebyshev polynomials of the fourth kind. Turk. J. Math. 40, 1283–1297 (2016)

    Article  MathSciNet  Google Scholar 

  31. Sweilam, N.H., Nagy, A.M., El-Sayed, A.A.: Solving time-fractional order telegraph equation via Sinc-Legendre collocation method. Mediterr. J. Math. 13(6), 5119–5133 (2016)

    Article  MathSciNet  Google Scholar 

  32. Wang, Z., Huang, X., Shen, H.: Control of an uncertain fractional order economic system via adaptive sliding mode. Neurocomputing 83, 83–88 (2012)

    Article  Google Scholar 

  33. Xin, B., Chen, T., Ma, J.: Neimark–Sacker bifurcation in a discrete-time financial system. Discrete Dyn. Nat. Soc. 2010, 405639 (2010)

    Article  MathSciNet  Google Scholar 

  34. Xin, B., Chen, T., Liu, Y.: Complexity evolvement of a chaotic fractional-order financial system. Acta Phys. Sin. 60(4), 048901 (2011)

    Google Scholar 

  35. Xin, B., Ma, J., Gao, Q.: The complexity of an investment competition dynamical model with imperfect information in a security market. Chaos Solitons Fract. 42, 2425–2438 (2009)

    Article  MathSciNet  Google Scholar 

  36. Yu, H., Cai, G., Li, Y.: Dynamic analysis and control of a new hyperchaotic finance system. Chaos Solitons Fract. 45, 1048–1057 (2012)

    Article  Google Scholar 

  37. Zhang, Xianmin, Agarwal, Praveen, Liu, Zuohua, Zhang, Xianzhen, Ding, Wenbin, Ciancio, Armando: On the fractional differential equations with not instantaneous impulses. Open Phys. 14(1), 676–684 (2016)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, Benha University, Benha, 13518, Egypt

    A. M. Nagy

Authors
  1. A. M. Nagy
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. M. Nagy.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Nagy, A.M. Non-Standard Finite Difference Schemes for Solving Variable-Order Fractional Differential Equations. Differ Equ Dyn Syst 29, 623–632 (2021). https://doi.org/10.1007/s12591-017-0378-2

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12591-017-0378-2

Keywords

Mathematics Subject Classification

Search

Navigation