Skip to main content
SpringerLink
Log in

Global Harvesting and Stocking Dynamics in a Modified Rosenzweig–MacArthur Model

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

Abstract

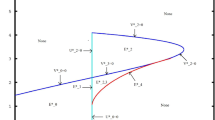
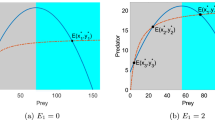
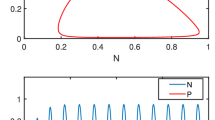
Effective management of predator–prey systems is crucial for sustaining ecological balance and preserving biodiversity, which requires full understanding the dynamics of such systems with harvesting and stocking. This paper aims to investigate the global dynamics of a Rosenzweig–MacArthur model considering the interplay of these intervention practices. We reveal that this model undergoes a sequence of bifurcations, including cusp of codimensions 2 and 3, saddle-node bifurcation, Bogdanov–Takens (BT) bifurcation of codimensions 2 and 3, and degenerate Hopf bifurcation of codimension 2. In particular, a codimension-2 cusp of limit cycles is found, which indicates the coexistence of three limit cycles. An interesting and novel scenario is discovered: two distinct homoclinic cycle curves connect their respective BT bifurcation points. This differs from most models where a single homoclinic cycle curve may connect both BT bifurcation points. Moreover, we find that two families of limit cycles converge toward a heteroclinic cycle, signaling the risk of overexploitation. From a biological perspective, the prey population may undergo extinction for all initial states under large constant harvesting rate. Further, the simultaneous stocking of both populations is not conducive to the coexistence of both species; the stocking of one population and the harvesting of the other will promote the coexistence of two populations; while the simultaneous harvesting of two populations may result in multiple limit cycles, which effectively underscore the positive effect of harvesting and stocking. Identifying the optimal timing to harvest or stock predators and prey is crucial to prevent system collapse. This work promotes to a deeper understanding of the dynamics of ecosystems when harvesting and stocking occurs simultaneously. Further, it reveals the important roles of harvesting and stocking, contributing to the effective management of predator–prey systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Data Availability

This paper has no associated data.

References

  1. Lotka, A.J.: Elements of Physical Biology. Williams & Wilkins, Baltimore (1925)

    Google Scholar 

  2. Volterra, V.: Fluctuations in the abundance of a species considered mathematically. Nature 118, 558–560 (1926). https://doi.org/10.1038/118558a0

    Article  Google Scholar 

  3. Leslie, P.H., Gower, J.C.: The properties of a stochastic model for the predator-prey type of interaction between two species. Biometrika 47, 219–234 (1960). https://doi.org/10.2307/2333294

    Article  MathSciNet  Google Scholar 

  4. Shigesada, N., Kawasaki, K.: Biological invasions: theory and practice. Japan. J. Ecol. 1:1 (1997). https://doi.org/10.18960/seitai.47.3_339

  5. Murray, J.D.: Mathematical Biology: I. An Introduction. Interdisciplinary Applied Mathematics. Springer, Berlin (2002)

    Book  Google Scholar 

  6. Li, B.T., Kuang, Y.: Heteroclinic bifurcation in the Michaelis-Menten-type ratio-dependent predator-prey system. SIAM J. Appl. Math. 67, 1453–1464 (2007). https://doi.org/10.1137/060662460

    Article  MathSciNet  Google Scholar 

  7. Li, Y.L., Xiao, D.M.: Bifurcations of a predator-prey system of Holling and Leslie types. Chaos Soliton. Fract. 34, 606–620 (2007). https://doi.org/10.1016/j.chaos.2006.03.068

    Article  MathSciNet  Google Scholar 

  8. Huang, W.Z.: Traveling wave solutions for a class of predator-prey systems. J. Dyn. Differ. Equ. 24, 633–644 (2012). https://doi.org/10.1007/s10884-012-9255-4

    Article  MathSciNet  Google Scholar 

  9. Zhu, C.R., Kong, L.: Bifurcations analysis of Leslie-Gower predator-prey models with nonlinear predator-harvesting. Discrete and Cont. Dyn. Sys. S 10, 1187–1206 (2017). https://doi.org/10.3934/dcdss.2017065

    Article  MathSciNet  Google Scholar 

  10. Seo, G., Kot, M.: A comparison of two predator-prey models with Holling’s type I functional response. Math. Biosci. 212, 161–179 (2008). https://doi.org/10.1016/j.mbs.2008.01.007

    Article  MathSciNet  Google Scholar 

  11. Sarif, N., Sarwardi, S.: Analysis of Bogdanov–Takens bifurcation of codimension 2 in a Gause-type model with constant harvesting of both species and delay effect. J. Biol. Syst. 29, 741–771 (2021). https://doi.org/10.1142/S0218339021500169

    Article  MathSciNet  Google Scholar 

  12. Wen, T., Xu, Y.C., He, M., Rong, L.B.: Modelling the dynamics in a predator-prey system with Allee effects and anti-predator behavior. Qual. Theor. Dyn. Syst. 22, 116 (2023). https://doi.org/10.1007/s12346-023-00821-z

    Article  MathSciNet  Google Scholar 

  13. May, R.M., Beddington, J.R., Clark, C.W., Holt, S.J., Laws, R.M.: Management of multispecies fisheries. Science 205, 267–277 (1979). https://doi.org/10.1126/science.205.4403.267

    Article  Google Scholar 

  14. Brauer, F., Soudack, A.C.: Coexistence properties of some predator-prey systems under constant rate harvesting and stocking. J. Math. Biol. 12, 101–114 (1982). https://doi.org/10.1007/BF00275206

    Article  MathSciNet  Google Scholar 

  15. Li, C., Rousseau, C.: A system with three limit cycles appearing in a Hopf bifurcation and dying in a homoclinic bifurcation: the cusp of order 4. J. Differ. Equ. 79, 132–167 (1989). https://doi.org/10.1016/0022-0396(89)90117-4

    Article  MathSciNet  Google Scholar 

  16. Dai, G.R., Tang, M.X.: Coexistence region and global dynamics of a harvested predator-prey system. SIAM J. Appl. Math. 58, 193–210 (1998). https://doi.org/10.1137/S0036139994275799

    Article  MathSciNet  Google Scholar 

  17. Xiao, D.M., Jennings, L.S.: Bifurcations of a ratio-dependent predator-prey system with constant rate harvesting. SIAM J. Appl. Math. 65, 737–753 (2005). https://doi.org/10.1137/S0036139903428719

    Article  MathSciNet  Google Scholar 

  18. Etoua, R.M., Rousseau, C.: Bifurcation analysis of a generalized Gause model with prey harvesting and a generalized Holling response function of type III. J. Differ. Equ. 249, 2316–2356 (2010). https://doi.org/10.1016/j.jde.2010.06.021

    Article  MathSciNet  Google Scholar 

  19. Laurin, S., Rousseau, C.: Organizing center for the bifurcation analysis of a generalized Gause model with prey harvesting and Holling response function of type III. J. Differ. Equ. 251, 2980–2986 (2011). https://doi.org/10.1016/j.jde.2011.04.017

    Article  MathSciNet  Google Scholar 

  20. Brauer, F., Soudack, A.C.: Stability regions in predator-prey systems with constant-rate prey harvesting. J. Math. Biol. 8, 55–71 (1979). https://doi.org/10.1007/BF00280586

    Article  MathSciNet  Google Scholar 

  21. Xiao, D.M., Ruan, S.G.: Bogdanov–Takens bifurcations in predator-prey systems with constant rate harvesting. Fields Inst. Commun. 21, 493–506 (1999)

    MathSciNet  Google Scholar 

  22. Brauer, F., Soudack, A.C.: Stability regions and transition phenomena for harvested predator-prey systems. J. Math. Biol. 7, 319–337 (1979). https://doi.org/10.1007/BF00275152

    Article  MathSciNet  Google Scholar 

  23. Brauer, F., Soudack, A.C.: Constant-rate stocking of predator-prey systems. J. Math. Biol. 11, 1–14 (1981). https://doi.org/10.1007/BF00275820

    Article  MathSciNet  Google Scholar 

  24. Myerscough, M.R., Gray, B.F., Hogarth, W.L., Norbury, J.: An analysis of an ordinary differential equation model for a two-species predator-prey system with harvesting and stocking. J. Math. Biol. 30, 389–411 (1992). https://doi.org/10.1007/BF00173294

    Article  MathSciNet  Google Scholar 

  25. Hogarth, W.L., Norbury, J., Cunning, I., Sommers, K.: Stability of a predator-prey model with harvesting. Ecol. Model. 62, 83–106 (1992). https://doi.org/10.1016/0304-3800(92)90083-Q

    Article  Google Scholar 

  26. Peng, G.J., Jiang, Y.L., Li, C.P.: Bifurcations of a Holling-type II predator-prey system with constant rate harvesting. Int. J. Bifurcat. and Chaos 19, 2499–2514 (2009). https://doi.org/10.1142/S021812740902427X

    Article  MathSciNet  Google Scholar 

  27. Ruan, S.G., Xiao, D.M.: Imperfect and Bogdanov–Takens bifurcations in biological models: from harvesting of species to isolation of infectives. J. Math. Biol. 87, 17 (2023). https://doi.org/10.1007/s00285-023-01951-3

    Article  MathSciNet  Google Scholar 

  28. Lin, X.Q., Xu, Y.C., Gao, D.Z., Fan, G.H.: Bifurcation and overexploitation in Rosenzweig–Macarthur model. Discrete Contin. Dyn. Syst. B 28, 690–706 (2023). https://doi.org/10.3934/dcdsb.2022094

    Article  MathSciNet  Google Scholar 

  29. Hsu, S.B.: On global stability of a predator-prey system. Math. Biosci. 39, 1–10 (1978). https://doi.org/10.1016/0025-5564(78)90025-1

    Article  MathSciNet  Google Scholar 

  30. Shan, C.H., Zhu, H.P.: Bifurcations and complex dynamics of an SIR model with the impact of the number of hospital beds. J. Differ. Equ. 257, 1662–1688 (2014). https://doi.org/10.1016/j.jde.2014.05.030

    Article  MathSciNet  Google Scholar 

  31. Lamontagne, Y., Coutu, C., Rousseau, C.: Bifurcation analysis of a predator-prey system with generalised Holling type III functional response. J. Dyn. Differ Equ. 20, 535–571 (2008). https://doi.org/10.1007/s10884-008-9102-9

    Article  MathSciNet  Google Scholar 

  32. Bogdanov, R.I.: Versal deformations of a singular point of a vector field on the plane in the case of zero eigenvalues. Funct. Anal. Appl. 9, 144–145 (1975). https://doi.org/10.1007/BF01075453

    Article  MathSciNet  Google Scholar 

  33. Bogdanov, R.I.: Bifurcation of the limit cycle of a family of plane vector fields/versal deformations of a singularity of a vector field on the plane in the case of zero eigenvalues. Sel. Math. Sov. 1, 373–387 (1984)

    Google Scholar 

  34. Takens, F.: Forced oscillations and bifurcations. In: Global Analysis of Dynamical Systems, pp. 11–71. CRC Press, Cambridge (2001)

  35. Perko, L.: Differential equations and dynamical systems. Differ. Equat. Dyn. Sys. 7, 181–314 (2001). https://doi.org/10.1007/978-1-4613-0003-8_3

    Article  MathSciNet  Google Scholar 

  36. Li, C.Z., Li, J.Q., Ma, Z.E.: Codimension 3 BT bifurcations in an epidemic model with a nonlinear incidence. Discrete Cont. Dyn. Sys. -B 20, 1107–1116 (2015). https://doi.org/10.3934/dcdsb.2015.20.1107

    Article  Google Scholar 

  37. Doedel, E.J., Champneys, A.R., Dercole, F., Fairgrieve, T.F., Kuznetsov, Y.A., Oldeman, B., Paffenroth, R.C., Sandstede, B., Wang, X.J., Zhang, C.H.: AUTO-07P: Continuation and Bifurcation Software for Ordinary Differential Equations. Concordia University, Montreal (2007)

    Google Scholar 

  38. Xu, Y.C., Yang, Y., Meng, F.W., Ruan, S.G.: Degenerate codimension-2 cusp of limit cycles in a Holling–Tanner model with harvesting and anti-predator behavior. Nonlinear Anal. Real World Appl. 76, 103995 (2024). https://doi.org/10.1016/j.nonrwa.2023.103995

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors are very grateful to professor Jiang Yu for his helpful and insightful suggestions. Y. C. Xu’s research is partially supported by the National NSF of China (No. 11671114) and L. B. Rong’s research is partially supported by the National NSF of USA (DMS-1950254).

Author information

Authors and Affiliations

  1. Department of Mathematics, Qufu Normal University, Qufu, 273165, Shandong, China

    Yue Yang & Fanwei Meng

  2. Department of Mathematics, China Jiliang University, Hangzhou, 310018, Zhejiang, China

    Yue Yang & Yancong Xu

  3. Department of Mathematics, University of Florida, Gainesville, FL, 32611, USA

    Libin Rong

Authors
  1. Yue Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yancong Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Fanwei Meng
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Libin Rong
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

Yue Yang and Yancong Xu wrote the main manuscript text, Fanwei Meng and Libin Rong prepared figures. All authors reviewed the manuscript.

Corresponding authors

Correspondence to Yancong Xu or Fanwei Meng.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Coefficients in the Proof of Theorem 1

$$\begin{aligned}{} & {} \hat{a}_{10}=\frac{\beta x^{*}(1-2x^{*})}{\delta (\alpha +x^{*})},\ \hat{a}_{01}=-\frac{x^{*}}{\alpha +x^{*}},\ \hat{a}_{20}=\frac{(2 x^{*}-1) (\beta x^{*}-\delta (\alpha +x^{*}))}{\delta (\alpha +x^{*})^2}-1,\\{} & {} \hat{a}_{11}=-\frac{\alpha }{(\alpha +x^{*})^{2}},\ \hat{a}_{30}=\frac{(2 x^{*}-1)(\delta (\alpha +x^{*})-\beta x^{*})}{\delta (\alpha +x^{*})^{3}},\ \hat{a}_{21}=\frac{\alpha }{(\alpha +x^{*})^{3}};\\{} & {} \hat{b}_{10}=\frac{\beta (2 x^{*}-1)(\beta x^{*}-\delta (\alpha +x^{*}))}{\delta (\alpha +x^{*})},\ \hat{b}_{01}=\frac{\beta x^{*}}{\alpha +x^{*}}-\delta ,\\{} & {} \hat{b}_{20}=-\frac{\beta (2 x^{*}-1) (\beta x^{*}-\delta (\alpha +x^{*}))}{\delta (\alpha +x^{*})^2},\ \hat{b}_{11}=\frac{\alpha \beta }{(\alpha +x^{*})^{2}},\\{} & {} \hat{b}_{30}=\frac{\beta (2x^{*}-1)(\beta x^{*}-\delta (\alpha +x^{*}))}{\delta (\alpha +x^{*})^{3}},\ \hat{b}_{21}=-\frac{\alpha \beta }{(\alpha +x^{*})^{3}};\\{} & {} \hat{c}_{11}=\frac{-2\hat{a}_{01}^{2}\hat{b}_{20}+\hat{a}_{01}\hat{b}_{01}(2\hat{a}_{20}-\hat{b}_{11}) +\hat{a}_{11}\hat{b}_{01}^{2}+\hat{a}_{10}(\hat{a}_{01}\hat{b}_{11}-\hat{a}_{11}\hat{b}_{01})}{\hat{a}_{01} (\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{c}_{02}=\frac{-\hat{a}_{01}^2 \hat{b}_{20}+\hat{a}_{01} \hat{b}_{01} (\hat{a}_{20}-\hat{b}_{11})+\hat{a}_{11} \hat{b}_{01}^2}{\hat{a}_{01} (\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{c}_{30}=\frac{\hat{a}_{10}(\hat{a}_{01}\hat{b}_{21}-\hat{a}_{21}\hat{b}_{01})+\hat{a}_{01} (\hat{a}_{30}\hat{b}_{01}-\hat{a}_{01}\hat{b}_{30})}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})},\\ \end{aligned}$$
$$\begin{aligned}{} & {} \hat{c}_{21}=\frac{-3\hat{a}_{01}^{2}\hat{b}_{30}+\hat{a}_{01}\hat{b}_{01}(3\hat{a}_{30}-\hat{b}_{21}) +\hat{a}_{21}\hat{b}_{01}^{2}+\hat{a}_{10}(2\hat{a}_{01}\hat{b}_{21}-2\hat{a}_{21}\hat{b}_{01})}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{c}_{12}=\frac{-3\hat{a}_{01}^{2}\hat{b}_{30}+\hat{a}_{01}\hat{b}_{01}(3\hat{a}_{30}-2\hat{b}_{21}) +2\hat{a}_{21}\hat{b}_{01}^{2}+\hat{a}_{10}(\hat{a}_{01}\hat{b}_{21}-\hat{a}_{21}\hat{b}_{01})}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{c}_{03}=\frac{-\hat{a}_{01}^2 \hat{b}_{30}+\hat{a}_{01} \hat{b}_{01} (\hat{a}_{30}-\hat{b}_{21})+\hat{a}_{21} \hat{b}_{01}^2}{\hat{a}_{01} (\hat{a}_{10}+\hat{b}_{01})};\\{} & {} \hat{d}_{20}=\frac{\hat{a}_{01}^2 \hat{b}_{20}+\hat{a}_{10} \hat{a}_{01} (\hat{a}_{20}-\hat{b}_{11})-\hat{a}_{10}^2 \hat{a}_{11}}{\hat{a}_{01} (\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{d}_{11}=\frac{\hat{a}_{10}(\hat{a}_{11}\hat{b}_{01}+\hat{a}_{01}(2\hat{a}_{20}-\hat{b}_{11})) +\hat{a}_{01}(2\hat{a}_{01}\hat{b}_{20}+\hat{b}_{01}\hat{b}_{11})-\hat{a}_{10}^{2}\hat{a}_{11}}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{d}_{02}=\frac{\hat{a}_{01}^{2}\hat{b}_{20}+\hat{a}_{01}(\hat{a}_{10}\hat{a}_{20}+\hat{b}_{01} \hat{b}_{11})+\hat{a}_{10}\hat{a}_{11}\hat{b}_{01}}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{d}_{30}=\frac{\hat{a}_{01}^2 \hat{b}_{30}+\hat{a}_{10} \hat{a}_{01} (\hat{a}_{30}-\hat{b}_{21})-\hat{a}_{10}^2 \hat{a}_{21}}{\hat{a}_{01} (\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{d}_{21}=\frac{\hat{a}_{10}(\hat{a}_{21}\hat{b}_{01}+\hat{a}_{01}(3 \hat{a}_{30}-2\hat{b}_{21})) +\hat{a}_{01}(3\hat{a}_{01}\hat{b}_{30}+\hat{b}_{01}\hat{b}_{21})-2\hat{a}_{21}\hat{a}_{10}^{2}}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{d}_{12}=\frac{\hat{a}_{10}(2\hat{a}_{21}\hat{b}_{01}+\hat{a}_{01}(3\hat{a}_{30}-\hat{b}_{21})) +\hat{a}_{01}(3\hat{a}_{01}\hat{b}_{30}+2\hat{b}_{01}\hat{b}_{21})-\hat{a}_{10}^{2}\hat{a}_{21}}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})},\\{} & {} \hat{d}_{03}=\frac{\hat{a}_{01}^{2}\hat{b}_{30}+\hat{a}_{01}(\hat{a}_{10}\hat{a}_{30}+\hat{b}_{01} \hat{b}_{21})+\hat{a}_{10}\hat{a}_{21}\hat{b}_{01}}{\hat{a}_{01}(\hat{a}_{10}+\hat{b}_{01})}.\\ \end{aligned}$$
$$\begin{aligned}{} & {} a_{10}^{*}=-\frac{\delta (2x^{*}-1)}{\delta -2x^{*}+1},\ a_{01}^{*}=-\frac{(\delta -1)\delta +2x^{*}(2x^{*}-1)}{(\delta -2x^{*}+1) (\delta +4x^{*}-2)},\\{} & {} a_{20}^{*}=\frac{\delta (\delta +2 x^{*}-1) (6 x^{*2}-(\delta +5) x^{*}+1)}{x^{*} (\delta -2 x^{*}+1)^2 (\delta +4 x^{*}-2)},\\{} & {} a_{11}^{*}=\frac{2(x^{*}(6 x^{*}-\delta -5)+1)((\delta -1)\delta +2 x^{*} (2 x^{*}-1))}{x^{*}(\delta -2 x^{*}+1)^{2}(\delta +4 x^{*}-2)^{2}},\\{} & {} a_{30}^{*}=-\frac{(1-2 x^{*})^{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))^{2}}{x^{*2}(\delta -2 x^{*}+1)^{3}(\delta +4 x^{*}-2)^{2}},\\{} & {} a_{21}^{*}=-\frac{2(x^{*}(6 x^{*}-\delta -5)+1)((\delta -1)\delta +2 x^{*} (2 x^{*}-1))^{2}}{x^{*2}(\delta -2 x^{*}+1)^{3}(\delta +4 x^{*}-2)^{3}},\\{} & {} a_{40}^{*}=\frac{(1-2 x^{*})^2 ((\delta -1) \delta +2 x^{*} (2x^{*}-1))^3}{x^{*3}(\delta -2x^{*}+1)^{4}(\delta +4x^{*}-2)^{3}},\\{} & {} a_{31}^{*}=\frac{2(x^{*}(6 x^{*}-\delta -5)+1)((\delta -1)\delta +2 x^{*} (2 x^{*}-1))^{3}}{x^{*3}(\delta -2 x^{*}+1)^{4}(\delta +4 x^{*}-2)^{4}};\\{} & {} b_{10}^{*}=\frac{(\delta +4 x^{*}-2)(\delta -2 \delta x^{*})^{2}}{(\delta -2 x^{*}+1) ((\delta -1)\delta +2 x^{*}(2 x^{*}-1))},\ b_{01}^{*}=\frac{\delta (2 x^{*}-1)}{\delta -2 x^{*}+1},\\{} & {} b_{20}^{*}=-\frac{(\delta -2 \delta x^{*})^{2}}{x^{*}(\delta -2 x^{*}+1)^{2}},\ b_{11}^{*}=\frac{2\delta ^{2}(x^{*}(\delta -6 x^{*}+5)-1)}{x^{*}(\delta -2 x^{*}+1)^{2} (\delta +4x^{*}-2)},\\ \end{aligned}$$
$$\begin{aligned}{} & {} b_{30}^{*}=\frac{(\delta -2 \delta x^{*})^{2}((\delta -1)\delta +2 x^{*}(2x^{*}-1))}{x^{*2}(\delta -2x^{*}+1)^{3}(\delta +4x^{*}-2)},\\{} & {} b_{21}=-\frac{2\delta ^{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))(x^{*} (\delta -6 x^{*}+5)-1)}{x^{*2}(\delta -2 x^{*}+1)^{3}(\delta +4 x^{*}-2)^{2}},\\{} & {} b_{40}^{*}=-\frac{\delta ^{2}(1-2 x^{*})^{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))^2}{x^{*3}(\delta -2 x^{*}+1)^{4}(\delta +4 x^{*}-2)^{2}},\\{} & {} b_{31}^{*}=\frac{2\delta ^{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))^{2}(x^{*} (\delta -6 x^{*}+5)-1)}{x^{*3}(\delta -2 x^{*}+1)^{4}(\delta +4 x^{*}-2)^{3}};\\{} & {} c_{20}^{*}=-a_{20}^{*}b_{01}^{*}+a_{11}^{*}b_{10}^{*}-a_{10}^{*}b_{11}^{*}+a_{01}^{*}b_{20}^{*},\ c_{02}^{*}=\frac{a_{11}^{*}}{a_{01}^{*}},\\{} & {} c_{30}^{*}=-a_{30}^{*}b_{01}^{*}+a_{21}^{*}b_{10}^{*}-a_{20}^{*}b_{11}^{*}+a_{11}^{*}b_{20}^{*} -a_{10}^{*}b_{21}^{*}+a_{01}^{*}b_{30}^{*},\\{} & {} c_{21}^{*}=\frac{a_{10}^{*}(a_{11}^{*2}-2a_{01}^{*}a_{21}^{*})-a_{01}^{*}a_{11}^{*}a_{20}^{*}}{a_{01}^{*2}}+3a_{30}^{*}+b_{21}^{*},\ c_{12}^{*}=\frac{2a_{01}^{*}a_{21}^{*}-a_{11}^{*2}}{a_{01}^{*2}},\\{} & {} c_{40}^{*}=-a_{40}^{*}b_{01}^{*}+a_{31}^{*}b_{10}^{*}-a_{30}^{*}b_{11}^{*}+a_{21}^{*}b_{20}^{*} -a_{20}^{*} b_{21}^{*}+a_{11}^{*}b_{30}^{*}-a_{10}^{*}b_{31}^{*}+a_{01}^{*}b_{40}^{*}, \\{} & {} c_{31}^{*}=\frac{1}{a_{01}^{*3}}\{a_{01}^{*}(a_{01}^{*}(a_{01}^{*}(4a_{40}^{*} +b_{31}^{*})-2 a_{20}^{*}a_{21}^{*})+a_{20}^{*}a_{11}^{*2}-a_{01}^{*}a_{30}^{*}a_{11}^{*})\\{} & {} \qquad -a_{10}^{*}(a_{11}^{*3}-3 a_{01}^{*}a_{21}^{*}a_{11}^{*}\\{} & {} \qquad +3a_{01}^{*2}a_{31}^{*})\},\\{} & {} c_{22}^{*}=\frac{a_{11}^{*3}-3a_{01}^{*}a_{21}^{*}a_{11}^{*}+3a_{01}^{*2}a_{31}^{*}}{a_{01}^{*3}}. \end{aligned}$$

Coefficients in the Proof of Theorem 2 and Theorem 3

$$\begin{aligned}{} & {} \bar{a}_{00}=-\lambda _{1},\ \bar{a}_{10}=\frac{\delta (1-2x^{*})}{\delta -2 x^{*}+1},\ \bar{a}_{01}=-\frac{x^{*}}{\alpha +x^{*}},\ \bar{a}_{20}=\frac{(1-2x^{*})^{2}}{(\alpha +x^{*})(\delta -2x^{*}+1)}-1,\\{} & {} \bar{a}_{11}=-\frac{\alpha }{(\alpha +x^{*})^{2}};\ \bar{b}_{00}=-\lambda _{2},\ \bar{b}_{10}=\frac{(\alpha +x^{*})(\delta -2\delta x^{*})^{2}}{x^{*}(\delta -2x^{*}+1)^2},\ \bar{b}_{01}=\frac{\delta (2 x^{*}-1)}{\delta -2x^{*}+1},\\{} & {} \bar{b}_{20}=-\frac{(\delta -2\delta x^{*})^{2}}{x^{*}(\delta -2x^{*}+1)^{2}},\ \bar{b}_{11}=\frac{\alpha \delta ^{2}}{x^{*}(\alpha +x^{*})(\delta -2x^{*}+1)};\\{} & {} \bar{c}_{00}=\bar{a}_{01}\bar{b}_{00}-\bar{a}_{00}\bar{b}_{01},\ \bar{c}_{10}=\bar{a}_{11}\bar{b}_{00}-\bar{a}_{10}\bar{b}_{01}+\bar{a}_{01}\bar{b}_{10}-\bar{a}_{00} \bar{b}_{11},\\{} & {} \bar{c}_{01}=\bar{a}_{10}-\frac{\bar{a}_{00}\bar{a}_{11}}{\bar{a}_{01}}+\bar{b}_{01},\, \bar{c}_{20}=-\bar{a}_{20}\bar{b}_{01}+\bar{a}_{11}\bar{b}_{10}-\bar{a}_{10}\bar{b}_{11}+\bar{a}_{01} \bar{b}_{20},\\{} & {} \bar{c}_{11}=\frac{\bar{a}_{11}(\bar{a}_{00}\bar{a}_{11}-\bar{a}_{01}\bar{a}_{10})}{\bar{a}_{01}^{2}}+2 \bar{a}_{20}+\bar{b}_{11}, \ \bar{c}_{02}=\frac{\bar{a}_{11}}{\bar{a}_{01}};\ \bar{d}_{00}=\bar{c}_{00},\ \bar{d}_{10}=\bar{c}_{10}-2\bar{c}_{00}\bar{c}_{02},\\ \end{aligned}$$
$$\begin{aligned}{} & {} \bar{d}_{01}=\bar{c}_{01},\ \bar{d}_{20}=\bar{c}_{00}\bar{c}_{02}^{2}-2\bar{c}_{10}\bar{c}_{02}+\bar{c}_{20},\\{} & {} \bar{d}_{11}=\bar{c}_{11}-\bar{c}_{01}\bar{c}_{02};\ \bar{e}_{00}=\bar{d}_{00}-\frac{\bar{d}_{10}^{2}}{4\bar{d}_{20}},\ \bar{e}_{01}=\bar{d}_{01}-\frac{\bar{d}_{10}\bar{d}_{11}}{2\bar{d}_{20}},\ \bar{e}_{20}=\bar{d}_{20}, \bar{e}_{11}=\bar{d}_{11}.\\{} & {} \bar{a}_{00}^{*}=-\epsilon _{1},\ \bar{a}_{10}^{*}=\frac{\delta (1-2x^{*})}{\delta -2x^{*}+1},\ \bar{a}_{01}^{*}=-\frac{(\delta -1)\delta +2x^{*}(2x^{*}-1)}{(\delta -2x^{*}+1) (\delta +4x^{*}-2)},\\{} & {} \bar{a}_{20}^{*}=\frac{\delta (\delta +2 x^{*}-1) (6 x^{*2}-(\delta +5) x^{*}+1)}{x^{*} (\delta -2 x^{*}+1)^2 (\delta +4 x^{*}-2)},\\{} & {} \bar{a}_{11}^{*}=\frac{2(x^{*}(6 x^{*}-\delta -5)+1)((\delta -1)\delta +2 x^{*} (2 x^{*}-1))}{x^{*}(\delta -2 x^{*}+1)^{2}(\delta +4 x^{*}-2)^{2}},\\{} & {} \bar{a}_{30}^{*}=-\frac{(1-2 x^{*})^{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))^2}{x^{*2}(\delta -2 x^{*}+1)^{3}(\delta +4 x^{*}-2)^{2}},\\{} & {} \bar{a}_{21}^{*}=-\frac{2(x^{*}(6 x^{*}-\delta -5)+1)((\delta -1)\delta +2 x^{*} (2 x^{*}-1))^{2}}{x^{*2}(\delta -2 x^{*}+1)^{3}(\delta +4 x^{*}-2)^{3}},\\{} & {} \bar{a}_{40}^{*}=\frac{(1-2 x^{*})^{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))^3}{x^{*3}(\delta -2 x^{*}+1)^{4}(\delta +4 x^{*}-2)^{3}},\\{} & {} \bar{a}_{31}^{*}=\frac{2( x^{*}(6 x^{*}-\delta -5)+1)((\delta -1)\delta +2 x^{*} (2 x^{*}-1))^{3}}{x^{*3}(\delta -2 x^{*}+1)^{4}(\delta +4 x^{*}-2)^{4}};\\{} & {} \bar{b}_{00}^{*}=-\frac{ x^{*} (1-2 x^{*})^2 (\delta +4 x^{*}-2)}{12 x^{*2}-2 (\delta +5) x^{*}+2} \epsilon _2-\epsilon _3,\\{} & {} \bar{b}_{10}^{*}=-\frac{(1-2 x^{*})^2 (\epsilon _2 ((\delta -1) \delta +4 x^{*2}-2 x^{*})+\delta ^2 (\delta +4 x^{*}-2))}{(-\delta +2 x^{*}-1) ((\delta -1) \delta +4 x^{*2}-2 x^{*})},\\{} & {} \bar{b}_{01}^{*}=\frac{\epsilon _{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))+\delta (2 x^{*}-1)(\delta +4 x^{*}-2)}{(\delta -2 x^{*}+1)(\delta +4 x^{*}-2)},\\{} & {} \bar{b}_{20}^{*}=-\frac{(1-2 x^{*})^2 (\epsilon _2 ((\delta -1) \delta +(2 x^{*}-1)2 x^{*})+\delta ^2 (\delta +4 x^{*}-2))}{x^{*} (\delta -2 x^{*}+1)^2 (\delta +4 x^{*}-2)},\\{} & {} \bar{b}_{11}^{*}=-\frac{2(x^{*}(6 x^{*}-\delta -5)+1) (\delta ^{2}(\delta +4 x^{*}-2)+\epsilon _{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1)))}{x^{*}(\delta -2 x^{*}+1)^{2} (\delta +4 x^{*}-2)^{2}},\\ \end{aligned}$$
$$\begin{aligned}{} & {} \bar{b}_{30}^{*}=\frac{(1{-}2 x^{*})^{2}((\delta {-}1)\delta +2 x^{*}(2 x^{*}{-}1)) (\delta ^{2}(\delta +4 x^{*}{-}2){+}\epsilon _{2}((\delta {-}1)\delta {+}2 x^{*}(2 x^{*}{-}1)))}{x^{*2}(\delta -2 x^{*}+1)^{3}(\delta +4 x^{*}-2)^{2}},\\{} & {} \bar{b}_{21}^{*}=\frac{1}{x^{*2}(\delta -2 x^{*}+1)^{3}(\delta +4 x^{*}-2)^{3}} (2(x^{*}(6 x^{*}-\delta -5)+1))((\delta -1)\delta \\{} & {} \quad \qquad +2 x^{*} (2 x^{*}-1)) (\delta ^{2} (\delta +4 x^{*}-2)+\epsilon _{2}((\delta -1)\delta +2 x^{*}(2 x^{*}-1))),\\{} & {} \bar{b}_{40}^{*}=\frac{(1{-}2 x^{*})^2 ((\delta {-}1) \delta {+}2 x^{*} (2 x^{*}-1))^2 (\epsilon _2 ((\delta {-}1) \delta {+}2 x^{*} (2 x^{*}{-}1)){+}\delta ^2 (\delta {+}4 x^{*}{-}2))}{x^{*3} (\delta -2 x^{*}+1)^4 (2-\delta -4 x^{*})^3},\\{} & {} \bar{b}_{31}^{*}=-\frac{1}{x^{*3} (\delta -2 x^{*}+1)^4 (\delta +4 x^{*}-2)^4} 2 ((\delta -1) \delta +2x^{*} (2x^{*}-1))^2 \\{} & {} \quad \qquad (6 x^{*2}-(\delta +5) x^{*}+1) (\epsilon _2 ((\delta -1) \delta +2x^{*} (2x^{*}-1))+\delta ^2 (\delta +4 x^{*}-2));\\{} & {} \bar{c}_{00}^{*}=\bar{a}^{*}_{01} \bar{b}^{*}_{00}-\bar{a}^{*}_{00} \bar{b}^{*}_{01},\ \bar{c}_{10}^{*}=\bar{a}^{*}_{11} \bar{b}^{*}_{00}-\bar{a}^{*}_{10} \bar{b}^{*}_{01} +\bar{a}^{*}_{01} \bar{b}^{*}_{10}-\bar{a}^{*}_{00} \bar{b}^{*}_{11},\\{} & {} \bar{c}_{01}^{*}=\frac{\bar{a}^{*}_{01} (\bar{a}^{*}_{10}+\bar{b}^{*}_{01})-\bar{a}^{*}_{00} \bar{a}^{*}_{11}}{\bar{a}^{*}_{01}},\\{} & {} \bar{c}_{20}^{*}=\bar{a}^{*}_{21} \bar{b}^{*}_{00}-\bar{a}^{*}_{20} \bar{b}^{*}_{01}+\bar{a}^{*}_{11} \bar{b}^{*}_{10}-\bar{a}^{*}_{10} \bar{b}^{*}_{11}+\bar{a}^{*}_{01} \bar{b}^{*}_{20}-\bar{a}^{*}_{00} \bar{b}^{*}_{21},\\{} & {} \bar{c}_{11}^{*}=2 \bar{a}^{*}_{20}+\frac{\bar{a}^{*}_{00} \bar{a}^{*2}_{11}-\bar{a}^{*}_{01} (\bar{a}^{*}_{10} \bar{a}^{*}_{11}+2 \bar{a}^{*}_{00} \bar{a}^{*}_{21})}{\bar{a}^{*2}_{01}}+\bar{b}^{*}_{11},\ \bar{c}_{02}^{*}=\frac{\bar{a}^{*}_{11}}{\bar{a}^{*}_{01}},\\{} & {} \bar{c}_{30}^{*}=\bar{a}^{*}_{31} \bar{b}^{*}_{00}-\bar{a}^{*}_{30} \bar{b}^{*}_{01}+\bar{a}^{*}_{21} \bar{b}^{*}_{10}-\bar{a}^{*}_{20} \bar{b}^{*}_{11}+\bar{a}^{*}_{11} \bar{b}^{*}_{20}-\bar{a}^{*}_{10} \bar{b}^{*}_{21}+\bar{a}^{*}_{01} \bar{b}^{*}_{30}-\bar{a}^{*}_{00} \bar{b}^{*}_{31},\\{} & {} \bar{c}_{21}^{*}=3 \bar{a}^{*}_{30}+\frac{-\bar{a}^{*}_{00} \bar{a}^{*3}_{11}+\bar{a}^{*}_{01} (\bar{a}^{*}_{10} \bar{a}^{*}_{11}+3 \bar{a}^{*}_{00} \bar{a}^{*}_{21}) \bar{a}^{*}_{11}-\bar{a}^{*2}_{01} (\bar{a}^{*}_{11} \bar{a}^{*}_{20}+2 \bar{a}^{*}_{10} \bar{a}^{*}_{21}+3 \bar{a}^{*}_{00}\bar{a}^{*}_{31})}{\bar{a}^{*3}_{01}}\\{} & {} \quad \qquad +\bar{b}^{*}_{21},\\ \end{aligned}$$
$$\begin{aligned}{} & {} \bar{c}_{12}^{*}=\frac{2 \bar{a}^{*}_{01} \bar{a}^{*}_{21}-\bar{a}^{*2}_{11}}{\bar{a}^{*2}_{01}},\\{} & {} \bar{c}_{40}^{*}=-\bar{a}^{*}_{40} \bar{b}^{*}_{01}+\bar{a}^{*}_{31} \bar{b}^{*}_{10}-\bar{a}^{*}_{30} \bar{b}^{*}_{11}+\bar{a}^{*}_{21} \bar{b}^{*}_{20}-\bar{a}^{*}_{20} \bar{b}^{*}_{21}+\bar{a}^{*}_{11} \bar{b}^{*}_{30}-\bar{a}^{*}_{10} \bar{b}^{*}_{31}+\bar{a}^{*}_{01} \bar{b}^{*}_{40},\\{} & {} \bar{c}_{31}^{*}=\frac{1}{\bar{a}^{*4}_{01}}(\bar{a}^{*}_{00} \bar{a}^{*4}_{11}-\bar{a}^{*}_{01} \bar{a}^{*2}_{11}(\bar{a}^{*}_{10} \bar{a}^{*}_{11}+4 \bar{a}^{*}_{00} \bar{a}^{*}_{21}) -\bar{a}^{*3}_{01} (2 \bar{a}^{*}_{20} \bar{a}^{*}_{21}+\bar{a}^{*}_{11} \bar{a}^{*}_{30}+3 \bar{a}^{*}_{10} \bar{a}^{*}_{31})\\{} & {} \quad \qquad +\bar{a}^{*2}_{01} (\bar{a}^{*}_{20} \bar{a}^{*2}_{11}+ (3 \bar{a}^{*}_{10} \bar{a}^{*}_{21}+4 \bar{a}^{*}_{00} \bar{a}^{*}_{31}) \bar{a}^{*}_{11}+2 \bar{a}^{*}_{00} \bar{a}^{*2}_{21}))+4 \bar{a}^{*}_{40}+\bar{b}^{*}_{31},\\{} & {} \bar{c}_{22}^{*}=\frac{\bar{a}^{*3}_{11}-3 \bar{a}^{*}_{01} \bar{a}^{*}_{21} \bar{a}^{*}_{11}+3 \bar{a}^{*2}_{01} \bar{a}^{*}_{31}}{\bar{a}^{*3}_{01}};\ \bar{d}_{10}^{*}=\bar{c}_{10}^{*}-\bar{c}_{00}^{*} \bar{c}_{02}^{*},\ \bar{d}_{01}^{*}=\bar{c}_{01}^{*},\\{} & {} \bar{d}_{20}^{*}=\bar{c}_{20}^{*}+\bar{c}_{00}^{*} \bar{c}_{02}^{*2}-\frac{\bar{c}_{10}^{*} \bar{c}_{02}^{*}}{2},\ \bar{d}_{11}^{*}=\bar{c}_{11}^{*},\ \bar{d}_{30}^{*}=\bar{c}_{30}^{*}+\frac{1}{2} (\bar{c}_{10}^{*}-2 \bar{c}_{00}^{*} \bar{c}_{02}^{*}) \bar{c}_{02}^{*2},\\{} & {} \bar{d}_{21}^{*}=\bar{c}_{21}^{*}+\frac{\bar{c}_{02}^{*} \bar{c}_{11}^{*}}{2},\ \bar{d}_{12}^{*}=\bar{c}_{12}^{*}+2 \bar{c}_{02}^{*2},\ \bar{d}_{40}^{*}=\bar{c}_{40}^{*}+\bar{c}_{00}^{*} \bar{c}_{02}^{*4}+\frac{1}{4} (\bar{c}_{02}^{*} (\bar{c}_{20}^{*}\\{} & {} \quad \qquad -2 \bar{c}_{02}^{*} \bar{c}_{10}^{*})+2 \bar{c}_{30}^{*}) \bar{c}_{02}^{*},\\{} & {} \bar{d}_{31}^{*}=\bar{c}_{31}^{*}+\bar{c}_{02}^{*} \bar{c}_{21}^{*},\ \bar{d}_{22}^{*}=\bar{c}_{22}^{*}-\bar{c}_{02}^{*3}+\frac{3 \bar{c}_{12}^{*} \bar{c}_{02}^{*}}{2};\ \bar{e}_{00}^{*}=\bar{d}_{00}^{*},\ \bar{e}_{10}^{*}=\bar{d}_{10}^{*},\ \bar{e}_{01}^{*}=\bar{d}_{01}^{*},\\{} & {} \bar{e}_{20}^{*}=\bar{d}_{20}^{*}-\frac{\bar{d}_{00}^{*} \bar{d}_{12}^{*}}{2},\ \bar{e}_{11}^{*}=\bar{d}_{11}^{*},\ \bar{e}_{30}^{*}=\bar{d}_{30}^{*}-\frac{\bar{d}_{10}^{*} \bar{d}_{12}^{*}}{3},\ \bar{e}_{21}^{*}=\bar{d}_{21}^{*},\\{} & {} \bar{e}_{40}^{*}=\bar{d}_{40}^{*}+\frac{\bar{d}_{00}^{*} \bar{d}_{12}^{*2}}{4} -\frac{\bar{d}_{12}^{*} \bar{d}_{20}^{*}}{6},\ \bar{e}_{31}^{*}=\bar{d}_{31}^{*}+\frac{\bar{d}_{11}^{*} \bar{d}_{12}^{*}}{6},\ \bar{e}_{22}^{*}=\bar{d}_{22}^{*}; \bar{f}_{00}^{*}=\bar{e}_{00}^{*},\ \bar{f}_{10}^{*}=\bar{e}_{10}^{*},\\{} & {} \bar{f}_{01}^{*}=\bar{e}_{01}^{*},\ \bar{f}_{20}^{*}=\bar{e}_{20}^{*},\ \bar{f}_{11}^{*}=\bar{e}_{11}^{*}, \ \bar{f}_{30}^{*}=\bar{e}_{30}^{*}-\frac{\bar{e}_{00}^{*} \bar{e}_{22}^{*}}{3},\ \bar{f}_{21}^{*}=\bar{e}_{21}^{*},\ \bar{f}_{40}^{*}=\bar{e}_{40}^{*}-\frac{\bar{e}_{10}^{*} \bar{e}_{22}^{*}}{4},\\{} & {} \bar{f}_{31}^{*}=\bar{e}_{31}^{*};\ \bar{g}_{00}^{*}=\bar{f}_{00}^{*},\ \bar{g}_{10}^{*}=\bar{f}_{10}^{*}-\frac{\bar{f}_{00}^{*} \bar{f}_{30}^{*}}{2\bar{f}_{20}^{*}},\ \bar{g}_{01}^{*}=\bar{f}_{01}^{*},\\ \end{aligned}$$
$$\begin{aligned}{} & {} \bar{g}_{20}^{*}=\bar{f}_{20}^{*}+\frac{9 \bar{f}_{00}^{*} \bar{f}_{30}^{*2}}{16 \bar{f}_{20}^{*2}}-\frac{3 (5 \bar{f}_{10}^{*} \bar{f}_{30}^{*}+4 \bar{f}_{00}^{*} \bar{f}_{40}^{*})}{20 \bar{f}_{20}^{*}},\ \bar{g}_{11}^{*}=\bar{f}_{11}^{*}-\frac{\bar{f}_{01}^{*}\bar{f}_{30}^{*}}{2\bar{f}_{20}^{*}},\\{} & {} \bar{g}_{30}^{*}=\frac{\bar{f}_{10}^{*}(35\bar{f}_{30}^{*2} -32\bar{f}_{20}^{*}\bar{f}_{40}^{*})}{40\bar{f}_{20}^{*2}},\ \bar{g}_{21}^{*}=\bar{f}_{21}^{*}-\frac{3 (20 \bar{f}_{11}^{*} \bar{f}_{20}^{*} \bar{f}_{30}^{*}+\bar{f}_{01}^{*} (16 \bar{f}_{20}^{*} \bar{f}_{40}^{*}-15 \bar{f}_{30}^{*2}))}{80\bar{f}_{20}^{*2}},\\{} & {} \bar{g}_{40}^{*}=\frac{\bar{f}_{10}^{*} \bar{f}_{30}^{*} (16 \bar{f}_{20}^{*} \bar{f}_{40}^{*}-15 \bar{f}_{30}^{*2})}{64 \bar{f}_{20}^{*3}},\ \bar{g}_{31}^{*}=\bar{f}_{31}^{*}+\frac{7\bar{f}_{11}^{*}\bar{f}_{30}^{*2}}{8\bar{f}_{20}^{*2}} -\frac{5\bar{f}_{21}^{*}\bar{f}_{30}^{*}+4\bar{f}_{11}^{*}\bar{f}_{40}^{*}}{5\bar{f}_{20}^{*}};\\{} & {} \bar{h}_{00}^{*}=\bar{g}_{00}^{*},\ \bar{h}_{10}^{*}=\bar{g}_{10}^{*},\ \bar{h}_{01}^{*}=\bar{g}_{01}^{*}-\frac{\bar{g}_{00}^{*}\bar{g}_{21}^{*}}{\bar{g}_{20}^{*}},\ \bar{h}_{20}^{*}=\bar{g}_{20}^{*},\ \bar{h}_{11}^{*}=\bar{g}_{11}^{*}-\frac{\bar{g}_{10}^{*}\bar{g}_{21}^{*}}{\bar{g}_{20}^{*}},\\{} & {} \bar{h}_{31}^{*}=\bar{g}_{31}^{*}-\frac{\bar{g}_{21}^{*}\bar{g}_{30}^{*}}{\bar{g}_{20}^{*}};\bar{j}_{00}^{*}=\bar{h}_{00}^{*} \bar{h}_{31}^{*\frac{4}{5}} \bar{h}_{20}^{*-\frac{7}{5}},\ \bar{j}_{10}^{*}=\bar{h}_{10}^{*} \bar{h}_{31}^{*\frac{2}{5}} \bar{h}_{20}^{*-\frac{6}{5}}, \ \bar{j}_{01}^{*}=\bar{h}_{01}^{*} \bar{h}_{31}^{*\frac{1}{5}} \bar{h}_{20}^{*-\frac{3}{5}},\\{} & {} \bar{j}_{11}^{*}=\bar{h}_{11}^{*} \bar{h}_{31}^{*-\frac{1}{5}} \bar{h}_{20}^{*-\frac{2}{5}}. \end{aligned}$$

Coefficients in the Proof of Theorem 5

$$\begin{aligned} \sigma _{22}= & {} 20 (\alpha +\tilde{x})^4 (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1))^7-20 \tilde{x} (\alpha +\tilde{x})^3 (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1))^6 \\{} & {} \times (2 \alpha h-19 \tilde{x}^3 +(7-22 \alpha ) \tilde{x}^2+\alpha \tilde{x} (-5 \alpha -2 \beta +5))\\{} & {} +(\alpha +\tilde{x})^4 (-5 \alpha h-2 \tilde{x}^3) (\alpha \beta \tilde{x}^2 (h+(\tilde{x}-1) \tilde{x})+ (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1))^2)^3\\{} & {} -180 \tilde{x}^3 (\alpha +\tilde{x})^3 (\alpha +3 \tilde{x}-1) (-\alpha h+2 \tilde{x}^3+(\alpha -1) \tilde{x}^2)^5\\{} & {} \times (-\alpha h+5 \tilde{x}^3+(5 \alpha -2) \tilde{x}^2+\alpha \tilde{x} (\alpha +\beta -1))\\{} & {} +20 \tilde{x}^3 (\alpha +\tilde{x}) \left( -\alpha h+2 \tilde{x}^3+(\alpha -1) \tilde{x}^2\right) ^4 (2 \alpha h+17 \tilde{x}^3+(26 \alpha -5) \tilde{x}^2\\{} & {} +\alpha \tilde{x} (7 \alpha -2 \beta -7)) (-\alpha h+5 \tilde{x}^3+(5 \alpha -2) \tilde{x}^2+\alpha \tilde{x} (\alpha +\beta -1))^2\\{} & {} -20 \tilde{x}^4 (-\alpha h+2 \tilde{x}^3+(\alpha -1) \tilde{x}^2)^3 (\alpha h+4 \tilde{x}^3+(7 \alpha -1) \tilde{x}^2\\{} & {} +\alpha \tilde{x} (2 \alpha -\beta -2)) (-\alpha h+5 \tilde{x}^3+(5 \alpha -2) \tilde{x}^2+\alpha \tilde{x} (\alpha +\beta -1))^3\\{} & {} +(\alpha +\tilde{x})^2 (\alpha \beta \tilde{x}^2 (h+(\tilde{x}-1) \tilde{x})+(\alpha h- \tilde{x}^2 (\alpha +2 \tilde{x}-1))^2)^2\\{} & {} \times (30 (\alpha +\tilde{x})^2 (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1))^3+10 \tilde{x} (\alpha +\tilde{x})\\{} & {} \times (-\alpha h+2 \tilde{x}^3+(\alpha -1) \tilde{x}^2)^2 (-\alpha h+23 \tilde{x}^3+(30 \alpha -9) \tilde{x}^2\\{} & {} +\alpha \tilde{x} (8 \alpha +\beta -8))+\tilde{x}^3 (\alpha +\tilde{x}) (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x} -1)) (-10 (\alpha -1) \alpha h\\{} & {} +2 \alpha \tilde{x} (5 (\alpha -1) (7 \alpha +\beta -7)-24 h)+510 \tilde{x}^4+(821 \alpha -407) \tilde{x}^3\\{} & {} + \tilde{x}^2 (419 \alpha ^2+\alpha (48 \beta -499)+80))+(1-\alpha ) \tilde{x}^4\\{} & {} \times (\alpha h+13 \tilde{x}^3+(19 \alpha -4) \tilde{x}^2+\alpha \tilde{x} (5 \alpha -\beta -5))\\{} & {} \times (\alpha h-14 \tilde{x}^3+(5-17 \alpha ) \tilde{x}^2-\alpha \tilde{x} (4 \alpha +\beta -4)))\\{} & {} -(\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1)) (\alpha ^2 h+3\tilde{x}^4+(2 \alpha -1) \tilde{x}^3\\{} & {} +\alpha \beta \tilde{x}^2) (\alpha \beta \tilde{x}^2 (h+(\tilde{x}-1) \tilde{x})+(\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1))^2) (\tilde{x}^2 (\alpha +\tilde{x})\\{} & {} \quad \times (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1)) (3 \tilde{x}^2 (\alpha +\tilde{x}) (7 \alpha h-7 \tilde{x} (\alpha \beta +\tilde{x} (\alpha +2 \tilde{x}-1))\\{} & {} -46 \tilde{x} (\alpha +\tilde{x}) (\alpha +3 \tilde{x}-1))+19 \tilde{x} (-\alpha -3 \tilde{x}+1) (\alpha +\tilde{x})\\{} & {} \times (\alpha h-\tilde{x} (\alpha \beta +\tilde{x} (\alpha +2 \tilde{x}-1)))-5 (\alpha h-\tilde{x} (\alpha \beta +\tilde{x} (\alpha +2 \tilde{x}-1)))^2\\{} & {} +120 \tilde{x}^2 (\alpha +\tilde{x})^2 (\alpha +3 \tilde{x}-1)^2)+45 (\alpha +\tilde{x})^3 (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1))^3\\{} & {} +\tilde{x}^3 (\alpha h+4 \tilde{x}^3+(7 \alpha -1) \tilde{x}^2+\alpha \tilde{x} (2 \alpha -\beta -2)) \\{} & {} \times (5 (\alpha h-\tilde{x} (\alpha \beta +\tilde{x} (\alpha +2 \tilde{x}-1)))^2+19 \tilde{x} (-\alpha -3 \tilde{x}+1) \\{} & {} \times (\alpha +\tilde{x}) (\alpha h-\tilde{x} (\alpha \beta +\tilde{x} (\alpha +2 \tilde{x}-1)))+20 \tilde{x}^2 (\alpha +\tilde{x})^2 (\alpha +3 \tilde{x}-1)^2)\\{} & {} - \tilde{x} (\alpha +\tilde{x})^2 (\alpha h-\tilde{x}^2 (\alpha +2 \tilde{x}-1))^2 (5 \alpha h-301 \tilde{x}^3+(135-426 \alpha ) \tilde{x}^2\\{} & {} -5 \alpha \tilde{x} (26 \alpha +\beta -26))). \end{aligned}$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, Y., Xu, Y., Meng, F. et al. Global Harvesting and Stocking Dynamics in a Modified Rosenzweig–MacArthur Model. Qual. Theory Dyn. Syst. 23, 196 (2024). https://doi.org/10.1007/s12346-024-01056-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12346-024-01056-2

Keywords

Mathematics Subject Classification

Search

Navigation