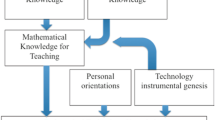
Abstract
When students engage with a digital tool to tackle a mathematical task, the interplay between their digital competencies and mathematical abilities gives rise to what is termed mathematical digital competency (MDC). Recognising the significance of MDC as an integral component for contemporary students, the relevance of cultivating mathematical digital competency for teaching (MDCT) becomes apparent. We posit that for teachers to effectively guide students in the development of their MDC, possessing MDCT is imperative. In this paper, drawing upon the theoretical frameworks of instrumental orchestration, the documentational approach to didactics, and the Danish competencies framework’s six didactico-pedagogical competencies of mathematics teachers, we propose a theoretical conceptualization of teachers’ mathematical and digital competencies in the context of teaching mathematics with technology. Expanding upon the preliminary definition of students’ MDC, we incorporate pedagogic, theoretical elements tailored to teachers, encapsulating it as MDCT. To expound on the various facets of MDCT, we provide an illustrative example featuring a mathematics teacher proficient in the utilisation of digital technologies and programming.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
Some years ago, Geraniou and Jankvist (2018) put forward their ideas about the demands of the digital era on students, arguing about the need for students to acquire both mathematical and digital competencies. In their efforts to better appreciate what students should be equipped with, they suggested a tentative conceptual notion—mathematical digital competency (MDC)—combining mathematical and digital competencies, in particular drawing on the KOM mathematics competencies framework (Niss & Højgaard, 2011), and the European Digital Competencies framework (Ferrari, 2013). Eventually, the latter was abandoned due to its focus on simple skills not directly linked with using digital tools in mathematics education. In the mathematics education literature, we find (at least) two other relevant constructs closely related to the idea of MDC. The first is that of techno-mathematical literacies, which concerns the functional mathematical knowledge one should have as mediated by, often digital, technologies within a given workplace practice (Kent et al., 2005; Van der Wal et al., 2017). The second is that of techno-mathematical fluency, which is “the ability to combine two types of background knowledge and skills—mathematical and technological—constantly being intertwined to develop techno-mathematical thinking”, emphasising also “the need to be fluent in a ‘language’ that entails both mathematical and technological knowledge” (Jacinto & Carreira, 2017, p. 1122).
The original motivation for introducing the MDC was the observation that students in the digital era grow up with digital technologies (DT), leading to a new “special” way of thinking about the world that surrounds them. When learning mathematics, they are often exposed to situations involving techno-mathematical discourse, e.g., when the teacher models how to draw a graph using GeoGebra.Footnote 1 Such situations inevitably lead to understanding mathematical concepts in the path that the digital tool in question (e.g., GeoGebra) prescribes and they thus develop instrumented techniques, which can be applied in similar and hopefully different situations too. Therefore, it was argued that one cannot view mathematical competencies and digital competencies separately. Rather these become a “merged entity” (Geraniou & Jankvist, 2019).
In their further work on MDC, Geraniou and Jankvist (2019) incorporated the theory of instrumental genesis (TIG) (e.g., Trouche, 2005) and the theory of conceptual fields (TCF) (Vergnaud, 2009). TIG because it is a dominant approach in the mathematics education literature concerning mathematics learning with the use of DT, and TCF as this enables the analysis of the process of instrumental genesis involving the transformation of DT into mathematical instruments that then become part of students’ cognitive schemes. With the help of an empirical example, Geraniou and Jankvist (2019) exemplified that both mathematical competencies and digital competencies can, and in fact should, be activated together in a teaching and learning situation involving DT. Both sets of competencies as well as mathematical knowledge and knowledge of the DT in question, influence the ways in which one interacts with the tool, what can be learned and what can be achieved. The two theoretical frameworks of TIG and TCF acted as a bridge for successfully linking mathematical and digital competencies. Similarly, and with departure in KOM, Geraniou and Misfeldt (2022) argued that both the mathematical competencies of students and those of mathematics teachers are influenced by DT; and although MDC is valuable when characterising the digital influence on mathematical competencies, the core of the digital influence for the teacher didactico-pedagogical competencies has until now been harder to pinpoint.
The influence of DT on the competencies needed to engage in teaching are wide (Geraniou & Misfeldt, 2022). It appears obvious that for students to develop MDC, we need to consider teachers’ MDC. But not only that, we also must consider the pedagogy necessary for teachers to employ to develop their students’ MDC. This notion is what we characterise as MDC for teaching (MDCT). Recently, Tabach (2021) raised a similar argument to support these views on MDC. She argued: “…the MDC defined by Geraniou and Jankvist (2019) also applies to teachers. Beyond this is a complementary set of competencies, specifically didactical digital mathematical competencies, that are relevant to the work of mathematics teachers” (p. 44). Tabach’s “didactical digital mathematical competencies” thus correspond to our notion of MDCT. While the first mentioning of a notion of MDCT stems from 2020 (Geraniou & Jankvist, 2020), a later study sought to illustrate its existence empirically through a case study of an expert teacher (Geraniou et al., 2022). In the present paper, we revisit the notion of MDCT, only this time from a theoretical stance. A theoretical conceptualisation of MDCT may eventually contribute with a language for observing, understanding, describing, and even explaining or predicting certain phenomena (Bikner-Ahsbahs & Prediger, 2010), in our case those of a successful and effective use of multiple DT for teaching mathematics. Yet, to do so, we must first consider how to go from MDC, and the cohort of theoretical constructs on which it builds, to MDCT—and in that process identify suitable theoretical constructs related to teaching to provide a more theoretical notion of MDCT. This of course involves linking (or combining) the theoretical perspectives involved, although not necessarily performing “networking of theories” in its strictest sense.
2 From MDC to MDCT: phrasing the research question
As mentioned above, drawing on KOM (Niss & Højgaard, 2019), TIG (Trouche, 2005) and TCF (Vergnaud, 2009), Geraniou & Jankvist, (2019) proposed that students possessing MDC display the following characteristics:
-
[MDC1]: Being able to engage in a techno-mathematical discourse. […] this involves aspects of the artefact-instrument duality in the sense that instrumentation has taken place and thereby initiated the process of becoming techno-mathematically fluent.
-
[MDC2]: Being aware of which digital tools to apply within different mathematical situations and context and being aware of the different tools’ capabilities and limitations. […] this involves aspects of the instrumentation–instrumentalization duality.
-
[MDC3]: Being able to use digital technology reflectively in problem solving and when learning mathematics. This involves being aware and taking advantage of digital tools serving both pragmatic and epistemic purposes, and in particular, aspects of the scheme-technique duality, both in relation to one’s predicative and operative form of knowledge. (p. 43)
Drijvers et al. (2013) delineated the three dualities referred to above by linking Vergnaud’s (2009) notion of schemes with techniques of instrumental genesis.
Still building on KOM—but now looking at the teacher—we apply KOM’s so-called didactico-pedagogical competencies (Niss & Højgaard, 2011, 2019), as a primary lens to understand teaching. KOM’s six didactico-pedagogical competencies enable us to describe mathematics teachers’ practices. Teacher competencies are not to be mistaken for solely a set of traits or skills; rather, they are defined in the way in which specific actions are implemented and the intentionality and importance that both precede and follow those actions (Winch, 2017). Teacher competencies are defined as the personal qualities—specifically, knowledge, beliefs, and motivation, as distinguished from behaviours and interactions—required for teachers to meet the demands in their profession (Fauth et al., 2019). Krumsvik and Jones’s (2013) characterisation of teacher’s digital competencies involves two dimensions; that of the competency to use DT for personal use and that of the competency to use DT in pedagogical settings. This has also been conceptualised as the double instrumental genesis (Haspekian, 2011), a process involving a pedagogical instrumental genesis on top of a teacher’s personal instrumental genesis. In relation to KOM, as was the case with the development of MDC, this framework proves insufficient for addressing a technological situation on its own. Two additional theoretical developments, both related to the instrumental approach, are thus adopted to support KOM’s didactico-pedagogical competencies.
The first one is that of the Theory of Instrumental Orchestration (TIO), which was initially developed to describe the pedagogical challenges in organising and managing how students apply different DT and resources in the classroom. TIO concerns the complexity of managing a classroom heavily dependent on use of DT and provides a language of “orchestrations” to describe the new possibilities and difficulties that emerge in such situations. Hence, TIO is an ideal framework for considering and making sense of teachers’ orchestrations when a variety of digital resources are used in mathematics lessons. The framework should be considered in the light of the three different dualities of the TIG as formulated by Drijvers et al. (2013) (see above), the reason being that any teacher deciding upon their orchestrations needs to consider how best to support their students “achieve” these three dualities.
The second is the Documentational Approach to Didactics (DAD), which focuses on the way the teachers adopt technologies to support their own work. The key idea of DAD is that teachers’ pedagogical work builds on instrumented techniques in ways that have similarities with the students’ mathematical work with digital artefacts (Trouche et al., 2018). In DAD, the tools that support teaching are described as “documents” to distinguish them from the mathematical instruments used by students. Such documents include lesson plans, online learning platforms, slides, and handouts. Hence, the approach studies the documentational genesis in which these artefacts influence and are transformed by teaching processes. DAD, therefore, allows us to identify the strategies of teachers for adopting DT to support their own work. In a situation of increasing focus on MDC for students, teachers are likely to increase their use of DT for preparation, planning, presentation, and documentation of teaching situations. Enacting MDCT for transforming teaching to build MDC with the students, is bound to influence teachers’ documentation work. DAD allows us to untangle influences from the technological infrastructure (or documentational system) from influences that originate, for instance, from new learning objectives.
Considering the above arguments, we ask the following research question: What components of TIO, DAD, and KOM might a notion of MDCT draw upon and how?
The reason for augmenting KOM’s didactico-pedagogical competencies with TIO and DAD is due to the way these specific frameworks both are centred round mathematics teachers’ work situations, while taking the specificity of mathematics into account. Building on the original notion of MDC, we thus apply the three lenses of TIO, DAD, and KOM to put forward a theoretical conceptualisation for the notion of MDC for teaching, MDCT, illustrating through evidence from a mathematics teacher’s practices. As mentioned, this may contribute to the development of a “language” for mediating educational practices and mathematics education research, which is in line with Silver and Herbst’s (2007) perspective on theoretical constructs being developed for certain purposes. Hence, combining and capitalising on existing theoretical constructs and frameworks related to how mathematics teachers rely on their knowledge and experience of how best to orchestrate resources, plan and teach their lessons (TIO), and the various “documents” that are needed for teaching a lesson (DAD), will serve the long-term aim of gaining a theoretical basis of MDCT. This will enable us to better communicate and understand the different didactico-pedagogical competencies (KOM) that come into play when mathematics teachers use, interact and teach with DT while supporting students’ development of MDC.
3 Theoretical constructs
We now explain in more detail the theoretical constructs of TIO, DAD, and KOM’s six didactico-pedagogical competencies for teaching.
3.1 The theory of instrumental orchestration
The Theory of Instrumental Orchestration (TIO) was developed by Trouche (2004) and further developed by Drijvers et al. (2014). It refers to “the teacher’s intentional and systematic organisation and use of the various artefacts available in a learning environment—in this case a computerised environment—in a given mathematical task situation, in order to guide students’ instrumental genesis” (p. 191). TIO comprises three key components: (a) a didactic configuration, which refers to the arrangement of artefacts in the teaching environment; (b) an exploitation mode, which denotes the approach chosen by a teacher to utilise a didactic configuration in line with their instructional intentions; and (c) a didactic performance, involving the instantaneous decisions made by a teacher during instruction to accommodate the chosen didactic configuration and exploitation mode. Current research studies have identified seven orchestration strategies for whole class teaching and one for individual or paired student work with DT (Drijvers et al., 2014). All seven orchestrations focusing on whole-class teaching have been developed to describe the teacher’s role in supporting and guiding students as they interact with DT, facilitating their learning of mathematics concepts and resource utilisation skills.
The first orchestration strategy is that of technical-demo, where the teacher demonstrates tool techniques. Such demonstrations can support students with their interactions with different tools and consider what the tools can and cannot do. The second is link-screen-board orchestration, which emphasises the connection between what happens in a technological environment and how this may be represented on paper, in books, and on the board. Third is the discuss-the-screen orchestration, which involves a whole-class discussion about the activities displayed on the computer screen. Such discussions welcome students’ reflections and ideas as to what was shown on the screen. The fourth is the explain-the-screen orchestration, which entails whole-class explanations by the teacher guided by what appears on the computer screen. Teachers can be in a “constant” dialogue with their students, to always explain what is happening on the “screen”. The fifth orchestration is spot-and-show, which highlights students’ reasoning by identifying their work during lesson preparation and using it in classroom discussions. Such guided interactions with a digital tool can support students’ understanding and reasoning. The sixth orchestration is Sherpa-at-work, where a designated student (referred to as a “Sherpa” by Trouche, 2004, 2005) uses the technology to present their work or perform actions requested by the teacher. The seventh is work-and-walk-by orchestration, where the didactic configuration involves students sitting at their technological devices while the teacher moves around the classroom. In their walk-around, the teacher can offer support and prompt students’ reflections on the immediate feedback offered by the tools considering their actions.
Finally, a feature from TIG, which is also important for TIO, is the distinction between two types of mediations—epistemic mediations and pragmatic mediations (see, Trouche, 2004)—here related to a teacher’s usage of resources. Pragmatic mediations (defined as the conversion of knowledge into action) involve enabling a teacher to act when meeting specific pragmatic goals in the classroom, for example activating students by providing them with a set of tasks in a digital learning environment. Epistemic mediations (the conversion of action into knowledge) allow the teacher to learn something about the teaching situation, for example by overviewing the students’ progress in a digital platform.
3.2 The documentational approach to didactics
MDCT should also include the ability to choose, and appropriate, tools and resources to support the various aspects of teacher work. The Documentational Approach to Didactics (DAD) is an extension to the instrumental approach that accounts for the design, pedagogic use, and adaptation of curriculum resources by teachers (Trouche et al., 2018). DAD focuses on how teachers appropriate resources and turn them into documents for teaching activities. Resources are broadly defined as “anything likely to intervene in teachers’ work” (Adler, 2000, p. 210) and are not limited to teaching materials, but may include social, cultural, and material elements as well. When teachers appropriate and utilise resources, it leads to the creation of a “document” that is considered the outcome of resource usage (Gueudet & Trouche, 2009). A document, in this context, is not a physical entity but a psychological construct that emerges from a specific use of a particular resource. Trouche et al. (2018) refers to this interplay with the metaphorical “equation” resources + schemes of usages = document. The genesis of such documents is the focus of the DAD framework. The process of document creation is considered dialectical as the resource can shape how it is used, and conversely, the usage can shape the resource. These two processes are referred to as instrumentation (resource shaping usage) and instrumentalization (usage shaping resources). The three dualities of the instrumental approach (Drijvers et al., 2013) are reinterpreted accordingly for the documental approach, i.e., as dualities between: (1) resource and document; (2) instrumentation and instrumentalization (the documentational genesis); and (3) teaching-technique and teaching-scheme.
As to the first duality, DAD distinguishes between a “resource” and a “document”. Gueudet and Trouche (2009) argue for the term resource to broaden the scope of curriculum materials, spanning from written textbooks, over personal notes, to multiple digital devices and platforms. The two terms (resource and document) also account for how resources influence, or re-sources teachers work (Adler, 2000), leading to a document that describes the interplay between the resource and schemes of usage (Trouche et al., 2018). A “document” is thus the joint set of resources, the practices involving using the resources, and the knowledge and schemes guiding the use (Trouche et al., 2020).
The dual process whereby a document is developed was coined by Gueudet and Trouche (2009) as documentational genesis. As with the instrumental genesis, this process is bidirectional. For one, the teacher’s knowledge and dispositions transform the resource into a document through its instrumentalization. In turn, the resource’s affordances and limitations influence the teacher’s practice, views on teaching as a process of instrumentation of the teacher. This dialectical genesis takes place during the design, re-design and design-in-use of resources into documents (Pepin et al., 2017).
The third duality in what we use to describe DAD, takes its departure in the distinction between technique and scheme developed in the context of instrumental genesis (Drijvers et al., 2013), and in what Bozkurt and Uygan (2020) define as professional instrumental genesis, with the difference that they focus on the influence of the mathematical tool (dynamic geometry in their case), not taking other resources (e.g., curriculum standards) into account. Documentational genesis is at the service of the teacher to act deliberately with the document in a teaching situation. As such, a resource-aided teaching-technique does not suffice to account for documentational genesis as well as a teaching scheme does, since the latter takes the teacher’s experience and dispositions into account. In that sense, we view DAD in direct continuity to TCF, where Vergnaud (1998) defines “scheme” as an invariant organisation of activity (or behaviour) for a certain class of situations. In the context of DAD, this corresponds to classes of teaching situations where schemes of usage apply. A scheme of usage is characterised by: (1) the aim of the activity; (2) the rules of action; and (3) the operational invariants (relevant knowledge), including the possibilities of inferences and adaptation to the variety of situations. As schemes are mental organisations of behaviour, one cannot observe these, but they can of course be inferred from behaviour or explored through conversations. In the context of DAD we will, building on Bozkurt and Uygan (2020), refer to these as teaching-schemes.
3.3 KOM’s didactico-pedagogical competencies
Niss and Højgaard (2019) distinguish between mathematical “competence” and “competency”: “Mathematical competence is someone’s insightful readiness to act appropriately in response to all kinds of mathematical challenges pertaining to given situations” (p. 12), whereas “mathematical competency is someone’s insightful readiness to act appropriately in response to a specific sort of mathematical challenge in given situations” (p. 14). The KOM framework covers eight such mathematical competencies: mathematical thinking, problem handling, modelling, reasoning, representation, symbols and formalism, communication, aids and tools. Both competence in general as well as mathematical competency are relevant in relation to KOM’s six so-called didactico-pedagogical competencies for teaching and thus also for MDCT.
Firstly, the curriculum competency involves the ability to study, analyse, and relate to both current and future mathematics curricula at a specific educational stage, but also to create a range of curricula and course plans that have different goals and purposes. Additionally, it entails evaluating the associated plans and their impact on teaching tasks. The teaching competency comprises the ability to devise, plan, and execute concrete mathematics teaching sequences, either independently or in collaboration with students, with different purposes and objectives. This encompasses creating a wide range of teaching and learning situations for diverse student groups, encouraging, and motivating them to take part in mathematical activities, including the ability to find, assess, select, and generate various teaching materials. The competency of revealing learning involves the ability to uncover and interpret students’ actual mathematical learning, their mastery of the eight mathematical competencies, as well as their conceptions, beliefs, and attitudes towards mathematics. This includes tracking the development of these aspects over time, delving deeper into how an individual’s mathematical learning and understanding are expressed in specific situations and contexts. The assessment competency encompasses the ability to select or create a wide range of tools for revealing and evaluating students’ learning outcomes and competencies, aiding them in rectifying, enhancing, and advancing their mathematical competencies. This can be in the context of specific courses, assessing the extent of students’ progress during the course, or in a more global sense, whether absolute or relative. Moreover, this competency necessitates the capacity to critically assess the accuracy and range of conclusions derived from the utilisation of assessment tools.
Next, the cooperation competency includes the ability to collaborate with colleagues, both within the field of mathematics and across other subjects, on matters relevant to teaching. This entails leveraging the pedagogical and didactic competencies. Furthermore, this competency extends to working with non-colleagues, such as students’ parents, administrative agencies, and educational authorities, to address issues related to teaching and its contextual boundaries. Finally, the professional development competency revolves around the ability to enhance one’s competence as a mathematics teacher, essentially a meta-competency. This encompasses engaging in activities that promote the development of one’s mathematical, didactic, and pedagogical competencies, while considering evolving conditions, circumstances, and opportunities. It involves reflecting on one’s teaching and engaging in discussions with mathematics colleagues, identifying areas for growth, and selecting or organising and evaluating activities, such as in-service courses, conferences, or projects, aimed at facilitating desired development.
4 The proposed MDCT conceptualisation
Recalling our research question, we explore KOM’s mathematical and didactico-pedagogical competencies and understand MDCT as the mathematical and digital competencies teachers need (or have) to select and implement technology in their practice in pedagogically productive ways. In a similar fashion as mathematics teachers must themselves possess the eight mathematical competencies to teach mathematics; we assume that mathematics teachers to some extent also possess the three characteristics of MDC in order to develop these in their students—a standpoint in line with both Haspekian (2011) and Krumsvik and Jones (2013). Inspired and informed by the previous literature and research on MDC (Geraniou & Jankvist, 2019; Tabach, 2021; Thurm et al., 2023) and KOM’s description of both students and teachers’ competencies, we apply the definition for students’ MDC to conceptualise teachers’ competencies in using technology, re-defined to suit teachers by including pedagogic elements from TIO and DAD. TIO because it is a natural extension of TIG, used in the conceptualisation of MDC, when it comes to orchestrating the use of DT in the classroom, and DAD because it brings depth to our analyses of MDCT in a similar way as TCF did for MDC. In the original definition of MDC, TCF allowed for a discussion of how both the tools at hand as well as the context and reasons for conducting mathematics are influenced by digital transformation. The concept of scheme and the conceptual field explain how an individual acts according to perceived invariants. In a similar fashion, DAD conceptualises how resources, such as DT and curricular documents, together with the teachers’ experience and dispositions develop the change in practice.
4.1 From MDC1 to MDCT1
As mentioned, MDC1 is about “Being able to engage in a techno-mathematical discourse. […] this involves aspects of the artefact-instrument duality in the sense that instrumentation has taken place and thereby initiated the process of becoming techno-mathematically fluent” (Geraniou & Jankvist, 2019, p. 43).
From the perspective of TIO, a teacher would be expected to have techno-mathematical fluency in using DT not only for doing mathematics, but also for teaching mathematics or in other words from a pedagogical perspective. Yes, teachers may showcase how to use DT, e.g., a graphic calculator, but they also need to utilise it in a meaningful way when planning teaching. When teaching how to draw a linear graph, would a teacher use a graphic calculator, GeoGebra, Desmos or other digital tools? Would a teacher ask students to copy their actions in their own graphic calculators or computers/tablets? Teachers need to be fluent in the technical features of a variety of tools and decide upon which to use and in what didactic configuration. To be successful in this important element of instrumental orchestration, a teacher needs to be techno-mathematically fluent with a variety of mathematical DT to enrich students’ learning experiences and have the competency of didactically configuring them for their teaching.
From the perspective of DAD, as teachers continue to develop their practice and as technology advances, new DT may become available, which a teacher may wish to incorporate into their practice. For example, a teacher may wish to use the relatively recent ChatGPT tool as a resource that can assist students to self-assess their mathematical work. Such new resources will be shaped and tailored into documents by teachers to specific teaching needs, but the resources will also push back on the teaching practice in a process of documentational genesis. In that sense, an adoption of ChatGPT as a self-assessment partner for students’ mathematical arguments will require a specific guideline on how to use the resource to self-assess (e.g., use a prompt in the style of “act as a mathematics teacher and give me a suggestion for improving this solution to the exercise”). This “document” will of course influence teaching practices. For example, it might add a new layer of interpreting and discussing “AI-replies”. This documentational genesis can lead to changes in how we talk about and conceptualise solving and evaluating mathematical word problems, exemplifying the scheme-technique duality. Furthermore, teachers develop their techno-mathematical fluency as they become competent in finding, assessing, selecting, and generating various teaching materials involving DT, i.e., artefacts that become instruments, for a wide range of teaching and learning situations for diverse groups of students.
From the perspective of KOM, teachers also need to develop their capacity to engage students in discussions regarding the content, forms, and perspectives of mathematics teaching, motivating, and inspiring them to participate in mathematical activities. All of which are elements of the teaching competency. To evaluate students’ progress, learning outcomes, competencies, and of course techno-mathematical fluency and MDC1, teachers would need to exercise and/or develop their assessment competency. This competency requires the ability to critically evaluate the validity and scope of conclusions drawn from the use of specific assessment instruments, including DT. Finally, it entails characterising an individual student’s learning outcomes and mathematical competencies, as well as effectively communicating with the student about these aspects and assisting them in improving and further developing their mathematical competencies.
Considering all the above characteristics of MDC, TIO, DAD and KOM, we propose MDCT’s first characteristic to be described as:
-
[MDCT1] Being able to engage in a techno-mathematical discourse at a pedagogic and a meta-pedagogic level. This means being able to orchestrate students’ engagements in a techno-mathematical discourse as well as incorporating new resources and techno-mathematical development into well-known teaching situations building on the resource-document and scheme-technique dualities. In particular, the didactico-pedagogical competencies of teaching and assessment are relevant in this regard.
4.2 From MDC2 to MDCT2
The second characteristic of MDC, MDC2, is about “Being aware of which digital tools to apply within different mathematical situations and context, and being aware of the different tools’ capabilities and limitations. […] this involves aspects of the instrumentation–instrumentalization duality” (Geraniou & Jankvist, 2019, p. 43).
From the perspective of TIO, teachers need to reflect on what each tool can offer and how it can be utilised for mathematics teaching, to organise their use to meet the objectives of their teaching and support students’ learning outcomes (exploitation mode). They need to be aware of the tools’ affordances and how these may restrict their use for doing, learning, or teaching mathematics, and decide which tool to use, when, how, and for what.
From the perspective of DAD, teachers need to be aware of two simultaneous processes when they bring resources into the classroom: (1) an instrumentation process, during which the digital resource would “shape” its usage by themselves for their own teaching demonstrations, but also how they would support students’ usage of the resource; and (2) the instrumentalization process, during which the way teachers use the digital resource “shapes” the resource itself. What is key in both these perspectives (TIO and DAD) is the pedagogical element they bring to the MDC notion. It is not about the process of using a digital resource, considering its capabilities and limitations to find a solution to a mathematical task. It is about the process of pedagogically supporting students in their interactions with DT, enacting both their mathematical knowledge and competencies and their digital competencies and knowledge of the tool.
From the perspective of KOM, a teacher is expected to be familiar with the school mathematics curriculum, and be competent in studying, analysing, and relating current with past and future curricula for every educational stage. This would enable them to choose wisely any DT for their teaching to design and implement diverse curricula and course plans with varying objectives and aims. This all while considering overarching frameworks and terms of reference that may exist under both current and future conditions, especially in relation to DT in mathematics education.
Considering the above, we propose MDCT’s second characteristic to be described as:
-
[MDCT2] Being aware of which digital tools to apply within different mathematical situations and context and being aware of the different tools’ capabilities and limitations, so as to think, and act, pedagogically with these tools, while considering the benefits and limitations of these. This means being able to engage in a process of documentational genesis, i.e., the instrumentation-instrumentalization duality, which brings resources into the classroom as well as orchestrating students’ explorations of affordances and limitations. In particular the didactico-pedagogical competency of curriculum plays a central role here.
4.3 From MDC3 to MDCT3
The third and last characteristic of MDC, MDC3, is about “Being able to use digital technology reflectively in problem solving and when learning mathematics. This involves being aware and taking advantage of digital tools serving both pragmatic and epistemic purposes, and in particular, aspects of the scheme-technique duality, both in relation to one’s predicative and operative form of knowledge” (Geraniou & Jankvist, 2019, p. 43).
From the perspective of TIO, a teacher is the “subject expert” that can most certainly guide students to the right path for solving a mathematical problem using DT as necessary. They can of course plan for modelling a solution path, but they can also take “instantaneous” decisions during their teaching, which is characterised as didactic performance, and support their didactic configurations (as explained in MDCT1) along with their exploitation mode (as explained in MDCT2).
From the perspective of DAD, a teacher should have the ability to engage in conversations with their students or observe them to identify how they interact with DT to solve a mathematical problem. Students’ schemes, as observed in each situation by the teacher, can reveal their thinking processes and progress in mathematical learning. Of course, teachers need to support their students in taking advantage of a digital tool using their predicative form of knowledge (knowing why), but also their operative knowledge (knowing how to), as such competencies should enable students to connect their instrumented techniques to mathematical schemes.
From the perspective of KOM, a teacher needs to be mathematically competent in identifying students’ difficulties and interpreting their solving approaches and mathematical way of thinking and learning. This of course entails being able to identify all eight mathematical competencies from the KOM framework, but also consider how best to support students in further developing these mathematical competencies over time with the support of DT, while at the same time using DT themselves (students and teachers). All these are of course aspects of the didactico-pedagogical competencies, as a teacher would need to exercise these to find, assess, select, and generate various teaching materials and digital resources.
So, the third and final characteristic of the MDCT conceptualisation can be described as follows:
-
[MDCT3] Being able to use digital technology reflectively in problem solving and when doing (learning or teaching) mathematics. This means being able to orchestrate students’ approaches to problem-solving, involving a scheme-technique duality, where the digital resources allow students to connect instrumented techniques to mathematical schemes. Besides the teaching competency, in particular the didactico-pedagogical competency of uncovering learning plays a central role for this type of MDCT.
So, possessing MDCT implies demonstrating the above described three characteristics.
5 Illustrative example: the case of Grace
Based on our experiences thus far and our interactions with teachers in a Danish project focussing on MDC alongside Programming and Computational Thinking (PCT) (Tamborg et al., 2023), we have noticed the following with regards to MDCT. In relation to MDCT1, integrating programming resources into the mathematics classroom requires teachers to consider how mathematical objects are represented, and in what ways students can interact with them. If teachers succeed in orchestrating students’ work with such tools and contrast their representations to other mathematical tools, the students can develop deep understandings of the relation between mathematical ideas and their representation in different environments. If not, new PCT tools represent a source of confusion. Regarding MDCT2, teachers generally have different levels of insights into what tools are available for teaching PCT. Therefore, mathematics teachers’ choice of tools is based on what they know rather than on an analysis of a tool’s affordances and constraints. Teachers are however capable of identifying when and how the given tools can be used, and to inform decisions on lesson design based on this. Finally, with respect to MDCT3, our studies have found that pedagogical frameworks from mathematics education and PCT by themselves fall short when designing lessons and teaching PCT as part of mathematics. Teachers need to develop new interdisciplinary pedagogical approaches to integrate PCT and mathematics. Even teachers with experience in teaching mathematics and PCT as separate subjects are challenged when these are combined.
One of the teachers we worked with, Grace, demonstrated strong evidence of all three characteristics of MDCT in her teaching. She designed a learning sequence that began by introducing the ScratchFootnote 2 environment to a class of 6th-graders, allowing them to explore its features, and challenged them to fix faulty code. The next activity involved teaching how to “create” regular polygons in Scratch. Besides considering properties of regular polygons (e.g., n equal sides and n equal angles), another learning objective was to teach the sum of exterior angles and the sum of interior angles. For the sum of exterior angles, Grace thought the best tool to use was Excel, since this would allow for relevant data to be tabulated (e.g., number of sides, angle size, exterior angle, of each n-sided regular polygon, starting from a triangle, see Fig. 1).
Excel table of angles and sum of angles by means of Scratch and GeoGebra (‘Kant’ is ‘side’; ‘Vinkel’ is ‘angle’; ‘Vinkelsum’ is ‘sum of angles’) (as cited in Elicer & Tamborg, 2023, p. 62)
Grace also introduced another digital resource, GeoGebra, and guided students to use the “Regular Polygons” feature. For each regular polygon created, students had to record the interior angles (beginning with a triangle) and the sum of interior angles in the same Excel spreadsheet as for the exterior angles. The use of these three different resources enabled Grace to discuss the numerical patterns between angles and sum of angles in both Scratch and GeoGebra, displayed in the Excel spreadsheet. The last activity involved students choosing a building of their own interest, drawing the building’s skyline on paper while making notes on how to code it in Scratch. Finally, the students applied their gained knowledge on polygons to create their skyline in Scratch. Even though there may be an interplay between the three characteristics of MDCT for a teacher, here Grace, we analyse for each of these separately in the following sections.
5.1 A teacher’s display of MDCT1
MDCT1 is about fostering situations that assist students in developing MDC1. It concerns the ability and willingness to discuss and develop what MDC means and how it can be fostered. At a meta-pedagogic level, this involves reflecting upon and examining the principles, strategies, and practices of teaching and learning. Grace was aware of how she fostered a techno-mathematical discourse in the classroom and how she engaged her students in this. Grace explained her decisions regarding the activities with an aim to accommodate the transitions from pen and paper to the three DT used. Firstly, she wanted her students to be the ones posing the problem and exploring their own solution strategies as sub-problems would appear. The context of the last task was agreed to be the drawing of skylines of buildings by learning first to draw polygons on paper, in Scratch and in GeoGebra.
When coding regular polygons in Scratch, the students had the freedom to choose colours, size, and order. The original version offered by the researchers had an initial code and a specific order to reduce difficulties (see, Elicer & Tamborg, 2023). However, Grace challenged this approach by insisting that the students needed to ask themselves how to solve the problem. Secondly, the solution to the problem should involve both computational and mathematical knowledge and skills. This criterion validates the task’s original purpose. Thirdly, Grace suggested involving more DT in the students’ work. She included Excel to systematise the data collection and aid pattern recognition. When a student asked: “why can we not simply use GeoGebra, which draws regular polygons automatically?”, Grace argued that she used Excel as an additional tool to support their recollection of the different angles in polygons and enable them to compare, reflect and derive mathematical statements regarding interior and exterior angles of polygons. She evidenced her pedagogy involving the use of different DT (Scratch, GeoGebra and Excel) for mathematical learning, each of which played a different role in the activity sequence. Students thus explored their affordances and limitations, an important mathematical learning process with DT and an important element of acquisition of MDC.
During our observations, Grace used a combination of orchestrations, such as: work-and-walk-by to support students when and if needed while they interacted with Scratch; technical-demo, discuss-the-screen and explain-the-screen in an effort to draw students’ attention to key features (e.g., create and remix blocks), and allow them to learn and appreciate what these features do. This prepared the students to interact with Scratch and initiated their engagement in a techno-mathematical discourse (MDC1). When Grace presented her students with a pre-made Scratch code and asked them to correct it, this led them to consider Scratch as an instrument to support their mathematical explorations and develop further their techno-mathematical discourse.
In her teaching, Grace quite often reminded students of past activities, allowing them to reflect on their own development of a techno-mathematical discourse regarding the DT used (MDC1). She used a variety of orchestrations to draw attention to students’ past work. The students’ and teachers’ MDC were enacted once again with the last task of modelling a skyline of their choice using Scratch. Grace actively fostered her students’ MDC1 through open-ended tasks and classroom discussions. Yet, being able to engage in a techno-mathematical discourse at a meta-pedagogic level does also influence how Grace used resources and planned her teaching. Her idea to use the spreadsheet helped to scaffold the discussion, so students could engage from a multitude of perspectives. Grace clearly engaged in a process of documentational genesis with the resources, pushing more discussion and investigation and less scaffolding than the resources initially suggest. She expressed that the explorative process and the students’ engagement with the discourse should be at the centre. Grace’s teaching shows an interplay between teaching scheme and teaching technique. Her goal of providing open-ended learning experiences was complemented by classroom discussion and clear tasks that acted as a safety guard for the teaching processes and ensured a balance between student discourse and Grace’s inputs and formative evaluation. Grace was able to engage her students in a techno-mathematical discourse and revealed her MDC1 along with her ability to support the students’ MDC1 at a pedagogic and a meta-pedagogic level. This is MDCT1.
5.2 A teacher’s display of MDCT2
In our interviews with Grace, we recognised her teaching objective as comparing different approaches to creating regular polygons and investigating their interior and exterior angles and the sum of those angles. She demonstrated awareness of which digital tools to apply within different mathematical situations and context (MDC2). She drew students’ attention to how the sprite in Scratch “forced” them to visualise the direction the sprite was going to move; hence, recognising that the focus was indeed on identifying how many degrees the sprite had to “turn” to draw the next side of the polygon, and that “turn” was in fact the exterior angle of the polygon. She also drew students’ attention to the angle indicated in their GeoGebra constructed polygons, which indeed was the interior angle of those polygons. She used the explain-the-screen orchestration to discuss the two different computations taking place in Scratch and GeoGebra, but also to showcase the data on angles of polygons presented in a different digital resource, Excel (see Fig. 1).
This latter action encouraged students to reflect on and compare exterior and interior angles of polygons of different numbers of sides, and spot any numerical patterns, e.g., the sum of exterior angles of any polygon always being 360 degrees. As argued earlier, Grace linked what was happening in Scratch and in GeoGebra, by using Excel, which in our view is an “evolution” of the link-screen-board orchestration and which we term as link-different-digital-resources orchestration (Geraniou et al., 2022). The use of spreadsheet is a clear example of documentational genesis, where a structured way of providing the information, influences the possibilities of moving from open exploration towards more convergence, allowing the class to focus on the difference between the different tools and seeing the value of adding an extra tool supporting student development of MDC2. The (re)organisation of this task to include Excel and GeoGebra is an example of Grace displaying didactico-pedagogical competencies of both teaching and curriculum in relation to digital tools’ different affordances, potentials, and limitations. In fact, all her decisions reveal her MDC2 and her awareness and application of didactical pedagogical strategies for teaching mathematics with the chosen three DT, which can be characterised as an awareness of different tools’ capabilities and limitations and when to use them, to think, and act, pedagogically with these tools, while considering the benefits and limitations of these. This is MDCT2.
5.3 A teacher’s display of MDCT3
Grace took advantage of the three DT serving both epistemic and pragmatic purposes for her own teaching and her students’ problem-solving and mathematical learning (MDC3). In one of her classroom discussions, they addressed the numerical patterns between the angles and sum of angles in both Scratch and GeoGebra, displayed in the Excel spreadsheet.
Grace: But what is the difference? Over here, the angle sum was 360 all the time. It was like that with the circle. Will you be able to calculate what the angle will be on a 30-sided [polygon]? I can figure out on a 20-sided [polygon] that it is 18 degrees because…?
Andy: Yes, the angle sum is 360.
Grace: Yes, the angle sum is 360, and I know it is a 20-sided [polygon], and what does it give there? 18. What is the relationship between 20, 18 and 360, Kate?
Kate: 20 times 18 is 360.
Elvis: There is the same angle and angle sum in Scratch as in GeoGebra.
Grace: No. How many polygons actually have the angle sum 360 in GeoGebra?
Hector: I can see two right now. Or not! One at 360 and then one at 3600.
Grace: Anyone see a pattern? Should I try to zoom in? Just a moment, Danny has the pattern, yes?
Danny: 180, yes! That means it [the sum of angles] is increasing all the time by 180.
Grace’s didactico-pedagogical competency of uncovering learning was displayed here, when recognising Danny’s discovery of a pattern in the increase of the sum of angles when increasing the number of sides because of relying on a variety of different tools. Also, Grace often recapped and reflected on what took place earlier and considered how the use of Scratch, GeoGebra and Excel supported the students’ learning (MDC3). Grace combined a complex and creative task (draw a skyline), which aligned well with the mathematical scheme for the learning sequence she put together, with teaching techniques that created safety and clarity. She used orchestrations such as discuss-the-screen and explain-the-screen, to draw students’ attention to their past work on Scratch, GeoGebra and Excel. We also noticed a “new” orchestration in Grace’s actions. As already mentioned earlier, she ran a classroom discussion on the two different computations taking place in Scratch and GeoGebra, focusing on the data on angles of polygons presented in a different digital resource, Excel. As mentioned, Grace took the link-screen-board orchestration a step further and instead of using the physical board to link what was happening in Scratch and in GeoGebra, she decided to use a third digital resource, Excel, that supported a tabular representation. In a way, she used a link-different-digital-resources orchestration, which allowed her to move between the three different interfaces showing students’ mathematical work. The students’ and teacher’s MDC were enacted once again with the final activity to model a skyline using Scratch and thus allowed for consolidating their gained mathematical knowledge on polygons to problem-solve in Scratch (MDC3). This last activity engaged students the most, as they used a real-life context of their own choice and applied their MDC to produce their own codes in Scratch, leading to the creation of buildings’ skyline models (see Fig. 2). Grace thus showcased her competency in developing students’ MDC3 in a didactico-pedagogical reflective manner. In other words, Grace was able to orchestrate students’ approaches to problem-solving while using different DT and when doing (learning or teaching) mathematics. This is MDCT3.
A student’s model of the Brandenburg Tower on paper (left) and in Scratch (right) (as cited in Elicer & Tamborg, 2023, p. 65)
6 Concluding remarks
The illustration of Grace’s teaching shows that she surely demonstrates MDC herself. This was evident when: (a) “making” the DT accessible to students by allowing students to explore Scratch and “debug” a code, supporting them in developing a techno-mathematical discourse (MDC1); (b) identifying the best tools to the various mathematical purposes (MDC2); (c) encouraging students to use Scratch to model the skylines, and support them in reflecting on the mathematical aspects of their solution (MDC3). These examples show Grace’s MDC enacted in a teaching situation focusing on the interplay between programming and mathematics.
Grace also showed an ability to engage in a techno-mathematical discourse at a pedagogic and a meta-pedagogic level (MDCT1), by orchestrating students’ techno-mathematical engagement (TIO), incorporating various resources into well-known teaching situations (DAD), and showing didactico-pedagogical competencies of teaching and assessment (KOM). Grace reflected about her use of DT. She was able to think, and act, pedagogically with these tools, considering benefits and limitations (MDCT2). She orchestrated the students’ explorations of affordances and limitations of different DT (TIO) and exercised the didactico-pedagogical competency of curriculum (KOM). Finally, Grace used DT reflectively in mathematical problem solving, both her own and the students (MDCT3). By orchestrating her students’ problem-solving, she showcased her possession of the didactico-pedagogical competency of uncovering learning (KOM).
Recalling our research question of which components of TIO, DAD and KOM might contribute to a notion of MDCT and how, we may now briefly summarise our findings. Firstly, TIO enabled us to consider teachers’ orchestrations with a variety of digital (and non-digital) resources considering the artefact-instrument duality. Secondly, DAD allowed us to identify the strategies of teachers for adopting DT to support their own work. Thirdly, KOM’s didactico-pedagogical competencies allowed us to describe teachers’ practice using the different competencies for teaching mathematics. Building on the original notion of MDC (Geraniou & Jankvist, 2019), these three different theoretical lenses enabled us to put forward a theoretical conceptualisation for the notion of MDC for teaching (MDCT), as presented in Sect. 6, and illustrated in Sect. 7 with evidence from a mathematics teacher, Grace.
The articulation of MDCT and the relations to the three theoretical constructs TIO, DAD and KOM have allowed us to characterise in detail how Grace worked with supporting the MDC of her students. This is an initial step in characterising the changes to teacher competencies that the digital era brings. Our approach differs from other attempts to characterise how the skills and knowledge of mathematics teachers are affected by digital transformation, because: (1) by departing in the construct of MDC, we take seriously that the learning objectives of mathematics teaching are broadly influenced by digitalisation, and that this influence is in no way superfluous or orthogonal to the “core” of mathematics; and (2) by building actively on TIO, DAD and KOM we place the articulation of MDCT in the mainstream discussions of teacher competencies and the influence of digitalisation on teaching.
Ahead of us lies both a fuller empirical and theoretical clarification of MDCT, but the case presented in this paper suggests the value of the construct. We hope to have convinced our readers that mathematics teachers teaching in the digital era are faced with situations where students may showcase MDC. Subsequently, it seems only natural to talk about teacher competencies for developing and furthering MDC. Hence, it becomes a matter of mathematical digital competency for teaching—that is MDCT.
References
Adler, J. (2000). Conceptualising resources as a theme for teacher education. Journal of Mathematics Teacher Education, 3(3), 205–224. https://doi.org/10.1023/A:1009903206236.
Bikner-Ahsbahs, A., & Prediger, S. (2010). Networking of theories—An approach for exploiting the diversity of theoretical approaches. In B. Sriraman, & L. English (Eds.), Theories of Mathematics Education: Seeking New Frontiers (pp. 483–506). Springer.
Bozkurt, G., & Uygan, C. (2020). Lesson hiccups during the development of teaching schemes: A novice technology-using mathematics teacher’s professional instrumental genesis of dynamic geometry. ZDM—Mathematics Education, 52(7), 349–1363. https://doi.org/10.1007/s11858-020-01184-4.
Drijvers, P., Godino, J. D., Font, V., & Trouche, L. (2013). One episode, two lenses: A reflective analysis of student learning with computer algebra from instrumental and onto-semiotic perspectives. Educational Studies in Mathematics, 82(1), 23–49. https://doi.org/10.1007/s10649-012-9416-8.
Drijvers, P., Tacoma, S., Besamusca, A., van den Heuvel, C., Doorman, M., & Boon, P. (2014). Digital technology and mid-adopting teachers’ professional development: A case study. In A. Clark-Wilson, O. Robutti, & N. Sinclair (Eds.), The mathematics teacher in the digital era (pp. 189–212). Springer.
Elicer, R., & Tamborg, A. L. (2023). A critical case study of the implementation of computation thinking in mathematics education. Implementation and Replication Studies in Mathematics Education, 3(1), 44–74. https://doi.org/10.1163/26670127-bja10011.
Fauth, B., Decristan, J., Decker, A. T., Büttner, G., Hardy, I., Klieme, E., & Kunter, M. (2019). The effects of teacher competence on student outcomes in elementary science education: The mediating role of teaching quality. Teaching and Teacher Education, 86, 102882. https://doi.org/10.1016/j.tate.2019.102882.
Ferrari, A. (2013). DIGCOMP: A Framework for developing and understanding Digital competence in Europe. A Scientific and Policy Report by the Joint Research Centre of the European Commission. European Union.
Geraniou, E., & Jankvist, U. T. (2018). A tentative framework for students’ mathematical digital competencies. In J. Golding, N. Bretscher, C. Crisan, E. Geraniou, J. Hodgen, & C. Morgan (Eds.), Research Proceedings of the 9th British Congress on Mathematics Education - BCME9 (pp. 56–63), University of Warwick, UK.
Geraniou, E., & Jankvist, U. T. (2019). Towards a definition of mathematical digital competency. Educational Studies in Mathematics, 102(1), 29–45. https://doi.org/10.1007/s10649-019-09893-8.
Geraniou, E., & Jankvist, U. T. (2020). Mathematical digital competencies for teaching from a networking of theories perspective. In B. Barzel, R. Bebernik, L. Göbel, M. Pohl, H. Ruchniewicz, F. Schacht, & D. Thurm (Eds.), Proceedings of the 14th International Conference on Technology in Mathematics Teaching (pp. 368–375). University of Duisburg-Essen.
Geraniou, E., & Misfeldt, M. (2022). The Mathematical competencies Framework and Digital Technologies. In U. T. Jankvist, & E. Geraniou (Eds.), Mathematical competencies in the Digital era (pp. 39–60). Springer.
Geraniou, E., Jankvist, U. T., Elicer, R., Tamborg, A. L., & Misfeldt, M. (2022). On mathematical digital competency for teaching: The case of an expert teacher. In H.-G. Weigand, A. Donevska-Todorova. E. Faggiano, P. Iannone, J. Medová; M. Tabach, & M. Turgut (Eds.), MEDA3 Mathematics Education in Digital Age 3, Proceedings of the 13th ERME Topic Conference (ETC13) (pp. 161–168). Constantine the Philosopher University in Nitra.
Gueudet, G., & Trouche, L. (2009). Towards new documentation systems for mathematics teachers? Educational Studies in Mathematics, 71(3), 199–218. https://doi.org/10.1007/s10649-008-9159-8.
Haspekian, M. (2011). The co-construction of a mathematical and a didactical instrument. In M. Pytak, T. Rowland, & E. Swobada (Eds.), Proceedings of the Seventh Congress of the European Society for Research in Mathematics Education (pp. 2298–2307). University of Rzeszów & ERME.
Jacinto, H., & Carreira, S. (2017). Mathematical Problem solving with technology: The Techno-Mathematical fluency of a student-with-GeoGebra. International Journal of Science and Mathematics Education, 15(6), 115–1136. https://doi.org/10.1007/s10763-016-9728-8.
Kent, P., Bakker, A., Hoyles, C., & Noss, R. (2005). Techno-mathematical literacies in the workplace. MSOR Connections, 5(1), 1–3.
Krumsvik, R., & Jones, L. (2013). Teachers’ digital competence in upper secondary school: (work in progress). Proceedings of the 2013 edition of ICICTE (pp. 171–183).
Niss, M., & Højgaard, T. (Eds.). (2011). Competencies and mathematical learning ideas and inspiration for the development of mathematics teaching and learning in Denmark. IMFUFA tekst, 485. Roskilde University. (Published in Danish in 2002). http://milne.ruc.dk/imfufatekster/pdf/485web_b.pdf.
Niss, M., & Højgaard, T. (2019). Mathematical competencies revisited. Educational Studies in Mathematics, 102(1), 9–28. https://doi.org/10.1007/s10649-019-09903-9.
Pepin, B., Gueudet, G., & Trouche, L. (2017). Refining teacher design capacity: Mathematics teachers’ interactions with digital curriculum resources. ZDM—Mathematics Education, 49(5), 799–812. https://doi.org/10.1007/s11858-017-0870-8.
Silver, E. A., & Herbst, P. G. (2007). Theory in mathematics education scholarship. In F. K. Lester (Ed.), Second handbook of Research on mathematics Teaching and Learning (pp. 39–68). NCTM.
Tabach, M. (2021). Competencies for teaching mathematics in the digital era: Are we ready to characterize them? In M. Inprasitha, N. Changsri, & N. Boonsena (Eds.), Proceedings of the 44th Conference of the International Group for the Psychology of Mathematics Education (Vol. 1, pp. 32–47). PME.
Tamborg, A. L., Elicer, R., Bråting, K., Geraniou, E., Jankvist, U. T., & Misfeldt, M. (2023). The politics of computational thinking and programming in Mathematics education– comparing curricula and resources in England, Sweden and Denmark. In B. Pepin, G. Gueudet, & J. Choppin (Eds.), Handbook of Digital Resources in Mathematics Education. Springer.
Thurm, D., Geraniou, E., & Jankvist, U. T. (2023). Unpacking Teacher Beliefs about Mathematical Digital Competency. In D. Diamantidis, M. Karavakou, M. Grizioti, & C. Kynigos (Eds.), 16th International Conference on Technology in Mathematics Teaching. NKUA.
Trouche, L. (2004). Managing the complexity of human/machine interactions in computerized learning environments: Guiding students’ command process through instrumental orchestrations. International Journal of Computers for Mathematical Learning, 9(3), 81–307. https://doi.org/10.1007/s10758-004-3468-5.
Trouche, L. (2005). Instrumental genesis, individual and social aspects. In D. Guin, K. Ruthven, & L. Trouche (Eds.), The didactical challenge of symbolic calculators: Turning a computational device into a mathematical instrument (pp. 197–230).
Trouche, L., Gueudet, G., & Pepin, B. (2018). Documentational approach to didactics. In S. Lerman (Ed.), Encyclopedia of Mathematics Education. Springer.
Trouche, L., Gueudet, G., & Pepin, B. (2020). The documentational approach to didactics. ArXiv. https://arxiv.org/pdf/2003.01392.pdf.
Van der Wal, N. J., Bakker, A., & Drijvers, P. (2017). Which Techno-Mathematical literacies are essential for future engineers? International Journal of Science and Mathematics Education, 15(1), 87–104. https://doi.org/10.1007/s10763-017-9810-x.
Vergnaud, G. (1998). Towards a cognitive theory of practice. In A. Sierpinska, & J. Kilpatrick (Eds.), Mathematics education as a research domain: A search for identity (pp. 227–240). Springer.
Vergnaud, G. (2009). The theory of conceptual fields. Human Development, 52(2), 83–94.
Winch, C. (2017). Teachers’ know-how: A philosophical investigation. Wiley.
Acknowledgements
The paper was partly written in the frame of project 8018-00062B under Independent Research Fund Denmark and project NNF19OC0058651 under Novo Nordisk Foundation.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Geraniou, E., Jankvist, U.T., Elicer, R. et al. Towards a definition of “mathematical digital competency for teaching”. ZDM Mathematics Education (2024). https://doi.org/10.1007/s11858-024-01585-9
Accepted:
Published:
DOI: https://doi.org/10.1007/s11858-024-01585-9