Abstract
This paper presents the results of a literature review focusing on the use and potential of Fermi problems (FPs) in the STEM disciplines. We identify different usages and roles given to FPs in each of the STEM disciplines and highlight their potential to promote modeling of real phenomena and situations as well as learning about the estimation of quantities. Modeling is found to be at the heart of integrating the different STEM disciplines and estimation is a key facilitator that enables students to tackle complex situations and content in all disciplines. The results of the review are discussed in terms of the potential and possibilities of FPs to support the development of twenty-first century competencies in the STEM disciplines.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.Avoid common mistakes on your manuscript.
1 Introduction and background
STEM Education, which brings together the disciplines of Science, Technology, Engineering and Mathematics into a single integrated field of study (Kelley and Knowles 2016), aims at providing students with competencies to meet employers’ growing demands in these areas. Both integrative and holistic approaches have been used to conceptualize the interdisciplinary nature of STEM (Fitzallen 2015). However, the integration of the different disciplines has proven challenging. During the last two decades STEM education primarily has focused on improving Science and Mathematics with little emphasis on integration and only limited attention given to Technology or Engineering (Bybee 2010). For example, the learning opportunities for enhancing the understanding and developing of mathematical skills within a STEM context was illustrated by Magiera (2013), but the way in which mathematics can contribute to the development of an understanding of the concepts of the other STEM disciplines has not been given much attention (Fitzallen 2015). Perhaps this is not so surprising given the protectionist view of the individual disciplines as illustrated in the case of mathematics by Shaughnessy (2013). He argued that mathematics “will become silent if not given significant attention” (p. 324) when disciplines are integrated in STEM education programs. It is still an open question for STEM educators how the disciplines can be effectively integrated while at the same time ensuring the integrity of each individual discipline (English 2017).
Although little is known about how this integration can be achieved (Honey et al. 2014), interdisciplinary and/or integrated approaches to STEM education are nevertheless widely stressed and argued for (The Common Core State Standards for Mathematics (http://www.corestandards.org/Math/); the Next Generation Science Standards (http://www.nextgenscience.org/); STEM Task Force Report 2014). For example, Kennedy and Odell (2014) have argued that “STEM education requires programs to include rigorous curriculum, instruction, and assessment, integrate technology and engineering into the science and mathematics curriculum, and also promotes scientific inquiry and the engineering design process” (p. 246). However, to be able to implement a “rigorous curriculum”, it is essential to know more precisely what should be implemented. Although there are numerous attempts in the literature trying to establish a set of skills and specify the knowledge needed to be developed in order to be a competent professional in the STEM field, no consensus has yet been reached (Binkley et al. 2012). One such attempt is by Binkley et al. (2012) who analyzed twelve twenty-first century skills frameworks from a number of different countries and identified ten skills grouped into four broad categories:
Ways of thinking
-
1.
Creativity and innovation.
-
2.
Critical thinking, problem solving, decision making.
-
3.
Learning to learn.
Ways of working
-
4.
Communication.
-
5.
Collaboration (teamwork).
Tools for working
-
6.
Information literacy (includes research on sources, evidence, biases, etc.).
-
7.
ICT (Information and Communication Technology) literacy.
Living in the World
-
8.
Citizenship—local and global.
-
9.
Life and career.
-
10.
Personal and social responsibility—including cultural awareness and competence.
(p. 36, italics in original).
Jang (2016), on the other hand, used data from STEM workplaces and identified the competencies called for as explicated in STEM job listings. Jang’s analysis resulted in a list of 18 skills, seven categories of knowledge and 27 work activities important in the STEM professions. Given the breadth and diversity of the competencies identified, Jang (2016) concluded that students should be motivated to solve interdisciplinary sets of complex problems collaboratively using critical thinking and knowledge from all STEM disciplines. Drawing on multiple studies in different STEM disciplines highlighting the possibilities of learning from real problems, Jang (2016) claimed that “problem solving that addresses ill-defined problems and demands the evaluation of multiple solution paths should be more encouraged in STEM education programs” (p. 296). This is consistent with Fitzallen (2015) who cited multiple authors advocating the integration of the STEM disciplines through engaging students in real-world problem solving, and Clark and Sengupta (2013) have argued that modeling “can productively engage students in inquiry-based activities that support learning of complex scientific concepts as well as the core argumentation and modeling practices at the heart of scientific inquiry” (p. 85).
Given this recommendation from STEM education research to focus on real world problems, and motivated by recent research carried out on modeling in the field of mathematics education showing that Fermi problems (FPs) can be useful in introducing mathematical modeling at educational levels ranging from primary to tertiary (Albarracín and Gorgorió 2014; Ärlebäck 2009; Czocher 2016; Peter-Koop 2009), we focus our review on what FPs have to offer in relation to STEM education and twenty-first century competences.
1.1 Fermi problems, estimation and mathematical modeling
FPs were originally used by the physicist Enrico Fermi to show the power of deductive thinking and to prepare his students for experimental laboratory work. Efthimiou and Llewellyn (2006) characterized FPs as open questions offering little or no specific information for the problem solvers to direct them in the solution process. Examples of FPs include: estimating the number of cars on a 3 km tailback on a motorway (Peter-Koop 2009); estimating to within one order of magnitude the number of hemoglobin molecules that your body makes every second (White 2004); and estimating how many shopping malls are in the United States (Anderson and Sherman 2010). The most prominent characteristic that defines FPs is the way in which their solutions are achieved. By focusing on a question asking for an estimate of a specific quantity, the idea is to tackle the problem by making assumptions based on the knowledge already available to the problem solver and following simple chains of reasoning. The procedure proposed by Fermi was to decompose the original problem into simpler sub-problems and to reach a solution to the original question by making reasonable estimates or educated guesses after first considering the individual sub-problems (Carlson 1997). In the literature this way of working is known as the Fermi (estimates) method. Thus, in our review, we consider that FPs are those questions that ask students to estimate quantities in the real world by following the Fermi method.
Given the emphasis on and the role of estimates and educated guesses in solving a FP, the research on FP can be seen as connected to the long tradition on estimation in the teaching and learning of mathematics. Generally, estimation is a process that gives a rough solution to a problem in counting or measurement (Siegel et al. 1982). Wagner and Davies (2010) connected estimation skills with number sense, which must be practiced with students until they reach a critical level allowing them not only to manipulate quantities meaningfully, but also to derive meaning from them so that they can be useful in making decisions. In mathematics, the focus on using estimates often occurs as students develop an understanding of, and methods for, counting and measuring (Reys 1984). But when FPs are used in other disciplines, the understanding of quantities and their relationships are on equal footing with concepts and skills of counting and measuring. This equal footing is directly related to seeing FPs as modeling problems.
Mathematical modeling, as understood in this paper, is a general problem-solving process encompassing the mathematising of a real-world phenomenon and elaborating a mathematical model to describe, understand or predict the behavior or properties of the phenomenon studied. We follow Lesh and Harel (2003), who define mathematical models as conceptual systems that describe other systems and take models to be constructed of a set of concepts to describe or explain the mathematical objects relevant to the phenomenon studied. Such models include the procedures used to create useful constructions, manipulations, or predictions for achieving clearly recognized goals. From this perspective, we concur with Robinson (2008), who considered FPs to be miniature-modeling problems in the sense that they are smaller, well-defined and delimited contextualized problems, not real world problems given in all their complexity. Both full scale modeling problems and FPs confront the problem solver with the need to conduct a detailed analysis of the situation presented in the statement of the problem, to decompose the original problem into simpler sub-problems, and to engage in validating the results in the context of the original problem. In this paper, we consider modeling being connected to real world problems whereas generic problem solving refers to general reasoning skills and metacognition.
1.2 Research questions
To investigate the role FPs can have as a facilitator and integrator in terms of teaching and learning in the STEM disciplines and developing twenty-first century competencies, we review the literature to answer the following research questions:
-
How, and based on what arguments, have FPs been used in the different STEM disciplines?
-
What are the research findings on the use of FPs in the STEM disciplines?
2 Method and analysis
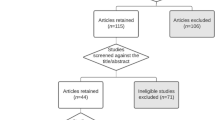
We have conducted a systematic literature review inspired by the six steps outlined by Templier and Paré (2015): formulating research questions and objectives; searching the literature; screening for inclusion; assessing the quality of primary studies; extracting data; and analyzing data. Given our research questions and objectives, we set out to do an exhaustive search of the literature to ensure the inclusion of all relevant journal articles, conference papers, book chapters, theses and research reports. To this end we used various databases, particularly Google Scholar, Scopus, Web of Science, Jstor and Crossref, and search phrases involving Fermi problem/s, Fermi question/s and Back-of-envelope and their counterparts in German, Portuguese, French, Spanish and Swedish. The searching and screening for inclusion was complicated due to the wide and diverse scientific production of Enrico Fermi; a large number of physics phenomena, institutions and instruments are associated with his name such as for example Fermi surfaces, the Fermi-Ulam model, the Fermi paradox, Fermi large area telescope, and Fermilab.
Our search resulted in a set of documents that was further expanded using forward- and backward searches (cf. Templier and Paré 2015), by looking through the references of the documents found and using the Google Scholar tool that lists the publications citing a specific source. This allowed us to identify a set of 117 relevant publications. Given our goal to be as exhaustive as possible and that the traditions and norms of writing in different disciplines follow different standards, we did not discriminate between research- or practitioner-oriented publications when screening the documents for inclusion and assessing their quality. For example, FPs appeared in American university physics classes (and in science and engineering classes more generally) in the middle of the twentieth century, predating the modern development of didactical fields of research. Hence, there exists a long tradition and shared knowledge about the learning promoted by FPs among students in physics and other science and engineering disciplines. However, the first academic work we found investigating the didactic potential of FPs was by Moore (1987). Thus, the knowledge about the potential of FPs as didactical tools in the STEM disciplines is expressed in two ways: first, in articles focusing on the reporting of knowledge obtained through experience and reflection on accumulated teaching practices, and, second and more recently, in traditional research studies.
During the screening of articles, we found multiple instances of one or more authors having a number of publications addressing similar questions and presenting basically the same data sets and analysis in different publications. A typical example of such an instance is when a conference paper later was developed into a full journal article. In these cases, only the more extended publication presenting the research was included in the review. We also excluded publications from the original 117 when the document consisted of a mere list of problems without any discussion or argumentation on the use of the problems. Most of the documents found are written in English, but we also found documents in Spanish, Portuguese, Swedish, French, Dutch, German, Japanese and Turkish. We managed to translate all papers written in languages we could not read using online tools, except for two Japanese articles which appeared to be practitioner-oriented papers. The screening process reduced the 117 initial publications to a core of 91 documents explicitly addressing the use of FPs in different educational settings.
All 91 documents were read and analyzed using a coding schema containing seven broader themes with accompanying guiding questions. The themes were: (A) Type of paper; (B) Focus and aim of the paper; (C) Definition of FP; (D) Setting of the research presented; (E) Methods applied in the research presented; (F) Analysis; and (G) Results and implications (see Table 1). During the first reading of the documents some of the data were straightforwardly recorded (such as year of publication or classifying the publication as being research- or practitioner-oriented) or resulted in various emerging lists (such as the theoretical frameworks or the actual FPs used in the publication). For some of the categories the relevant section(s) in the documents were marked in order to be later collectively subjected to open coding.
This process of analysis allowed us to connect the results of different empirical and practitioner-oriented studies to the theoretical foundations on which other studies were based.
The 91 documents analyzed were diverse in nature. Articles published in research journals in different fields used different frameworks and methodologies, especially when research articles in education were published in pure scientific journals. We found that 43 of the 91 publications reported on empirical studies focusing on teaching or learning using FPs. Eighteen of the 91 documents argued for the use of FPs in connection with curriculum changes, competence development or other education goals (such as changing beliefs or fostering critical thinking). Notably, 30 of the 91 publications describing the possibilities and potential of using FPs were written based on the author’s own teaching experiences.
The development of publications over time is shown in Table 2 by period of publication and STEM discipline. In science we found 22 documents with 19 of those documents in physics. This is not surprising given the origin of FPs in the work of Fermi. Mathematics had the largest number of documents (48) which is not surprising given the developments in the field of mathematical modeling in mathematics education over the last 10 years. The numbers of documents found in technology and engineering were scarce and hence are reported together. The documents were found in 61 different sources and Table 3 lists the sources with two or more contributions.
3 Results
In this section, we present the results from the literature review with respect to our research questions. We first give an overview of the arguments and results identified in the 91 publications analyzed. Then, we answer the second research question organized in four sections: estimation; number sense and beliefs; problem solving; and modeling. In the result section we then discuss FPs as facilitators and integrators among the different STEM disciplines, and how working on FPs can support students developing twenty-first century STEM competencies.
The actual arguments identified are elaborated in the following sections, but Table 4 summarizes the total number arguments for, and results on, FPs in each discipline. Mathematics education was found to be the discipline that provided the most arguments and results on FPs, and is, in addition the discipline that had the largest proportion of research studies.
In order to organize the identified arguments and results, we classified them by the type of content to which they are related as a result of open coding: the development of skills for estimation; number sense within the different disciplines and changes in beliefs based on numerical analysis of phenomena; aspects related to generic problem solving such as general reasoning or metacognition; and aspects related to the modeling of real phenomena. The number of arguments and results for each of these content areas is shown in Table 5. Most identified arguments are related to problem solving, whereas most results are related to modeling. The latter finding is possibly due to the increase of empirical studies of mathematical modeling in recent years (Schukajlow et al. 2018).
3.1 Estimation
The role and function of making and using estimates in different contexts is a common theme found in the literature. For example, Moore (1987) argued that engineers should be able to make adequate estimates based on their knowledge given limited data, and he advocated for introducing FPs in programming courses as a way to engage students in working with ill-defined problems. Similarly, Bentley (1999) advocated that computer science students needed to master some common techniques in programming similar to the Fermi estimates method in order to decide if their programs are adequate and effective for solving the problems they are trying to address. One such example of an FP is about deciding whether technical aspects (such as the speed of a network) are adequate for the intended use, or whether it is worth investing a certain amount of time in improving an algorithm given the impact on performance this can achieve.
Working with first year engineering students, Shakerin (2006) used a sequence of FPs focusing on the topic of energy. With the aim of developing students’ estimation skills, the objective of the early tasks in the sequence was to create a set of reference points to be used in later tasks as a basis for comparison. Students initially worked with basic estimates of quantities of length, area, volume, weight, density, energy and temperature. An important part of the approach was to verify the estimated quantities experimentally. In one task students estimated the weight of a person’s limb, such as an arm or leg, by using the water displaced in a bathtub. In other tasks, such as the estimation of the electrical energy consumed in a household, information measured and collected by others (e.g., meter readings) could be used to verify the estimate. According to Shakerin, students can experience the potential in the Fermi method and make connections to the theories on which the meaning and relationships among quantities are based when they tackle more complex problem situations. Shakerin argued that this can be done by attending to the units of the involved quantities, and that this sequencing of tasks supports the development of the knowledge and skills needed to use estimation properly in engineering workplace activities. Using FPs in connection with laboratory experiments and in situations where it is not possible or feasible to make direct measurements to make decisions, resonates with the practice of engineering by encouraging students to use and integrate previous knowledge about physical laws, about units and dimension, and their own estimates and references.
The work of Moore (1987), Bentley (1999) and Shakerin (2006) illustrate educational usages of FPs in the decision-making and planning phases in engineering processes where estimation skills are crucial. Other authors working in different disciplines share this idea. Robinson (2008) described order of magnitude calculations as an essential skill in physics that can confirm the feasibility of a model or determine whether an onerous and time-consuming calculation is required. In economics, Anderson and Sherman (2010) observe that it is often necessary to make quick estimates when neither time nor resources are available for making more time consuming or costly assessments, as in the first stages of product development when entrepreneurs evaluate the commercial viability of new commodities.
However, as illustrated in Table 5, the reviewed research has not focused on explicitly establishing evidence supporting the argument that FPs promote students’ developing of their estimation skills.
3.2 Number sense and beliefs
Wagner and Davies (2010) examined how FPs promote connecting experiences of quantities and computation skills in terms of number-, quantity- and critical sense. They argued that it is essential that students as citizens be able to interpret and respond critically to the barrage of numbers that they meet every day. Albarracín and Gorgorió (2015) showed how secondary students developed their own methods to solve real situation FPs and became more confident in their calculations and results than in the data reported in newspapers. Along the same lines, Carlson (1997) argued that FPs and the Fermi method offer opportunities for presenting contemporary problems to students and proposed the study of environmental problems related to fuel consumption, such as the consequences of changing large cars to smaller cars with lower fuel consumption. Carlson pointed out that working on FPs can quickly make students conscious of their abilities, skillful in giving quick answers based on simple calculations in various settings, and that engaging in problems connected with economic and social contexts enables the students to develop their opinions and beliefs in an informed manner.
Although not experimentally verified, one of the most common claims for using FPs in the literature is that they promote understandings and meanings of quantities within the disciplines. The quantitative study of Furjanic and Müller (2001) concluded that secondary students were better at estimating everyday quantities than estimating the more discipline specific quantities relevant for the physics courses they were taking. White (2004) argued that the quantitative approach promoted by FPs provides the basic component for understanding quantitative problems in biology. Phillips and Milo (2009) stressed that for problems in biology that cannot be addressed qualitatively, a quantitative approach can provide relevant information to better understand and explain processes that are difficult to study. Thus, they proposed that the Fermi method be used as a research tool in the life sciences: “the time is ripe for the emergence of a similar tradition [using Fermi estimates] in the biological setting because as we argue… such estimates can reveal gaps in our understanding” (Phillips and Milo 2009, p. 21467). In order to have reliable quantitative data in the field of biology readily available, Milo’s team created a free easy-access Wikipedia-like community effort to collect numerical data that have been published in peer-reviewed literature (see www.bionumbers.org). The Fermi estimation method has been used successfully on a large number of problems for which it is difficult to find a solution using traditional and common methods in biology as illustrated by the work of Machtans and Thogmartin (2014) on estimating bird populations from very small data sets. These authors emphasized that Fermi estimates often are more accurate than expected because the multiplication of several estimated factors will normally include both overestimated and underestimated quantities, potentially cancelling out some of the approximation error.
Focusing on generating meaningful understanding of quantities, Cordry (2010) used the Fermi method to address the earth’s limited resources and its capacity to sustain the world’s population from the point of view of thermodynamics. Cordry used FPs to introduce a unit of reference in terms of the energy consumption a person needs for survival. The suggested task raised multiple questions such as: Will the world’s population continue to grow without restraint? Are there sufficient sources of food and energy to provide a decent living-standard for all people? The students are generally surprised by the results of their calculations, especially when they learn that the present world population’s energy needs exceeds the sources that they have just calculated are available. The activity was used at the college level in a unit on the conservation of energy and allowed the scientific content to be presented in a context relevant for the students and to open up a discussion that goes beyond the physics class.
The findings by Cordry (2010) can be complemented by those of Morgan (2017), who studied the changes in college students’ perceptions regarding the prevalence of life in the galaxy after using estimates while working on the Drake equation. Using a pre-/post-test design, Morgan noted a shift towards a more optimistic stance regarding the existence of extra-terrestrial life in the galaxy. Morgan argued that the Fermi method provided a tool for making unknown aspects closer and more accessible to students and that FPs have a real potential to make an impact on students’ beliefs about the world.
3.3 Problem solving
Many of the analyzed documents highlighted aspects of problem solving that can be worked on using FPs. Taggart et al. (2007) stressed that FPs promote flexible thinking and creating one’s own method to deal with problems when no more direct solution method is readily applicable or available. Seiwald (2016) argued that FPs move away from a strong emphasis on routine tasks and focus on developing and deepening the understanding of important concepts. Seiwald claimed that FPs can support educational contexts in which different solutions to the same problem are promoted and can support a culture of discussion and debate in the classroom. Other researchers have tried to connect FPs to various mathematical competencies, or in other ways attempted to theorize the potential and possibilities of FPs in classrooms. For example, Sriraman and Knott (2009) stressed that working with educated guesses in socially relevant contexts can be a form of fostering mathematical and critical thinking. Other studies have shown how teachers can use FPs to promote tolerance for the ambiguities arising in problem solving (Palmér et al. 2018). By studying students’ work on FPs in an engineering problem contest, Holubova (2017) showed that students can use many different activities in the analytical phase of solving a problem (selecting key reference points, graphical sketching, and analyzing the physics of the situation), and their results suggested that this the phase of problem solving in which students struggled the most. Holubova’s analysis showed that students tended not to validate their obtained results adequately and argued that this crucial part of the problem-solving process needs to be taught explicitly.
Some authors investigated the use of FPs complemented with different technologies in order to have a larger impact on the mathematical thinking of the students. Tangney and Bray (2013), for example, proposed the use of mobile tools such as still or video cameras and stopwatches to capture data on problems related to counting groups or flows of people in spaces such as schools or supermarkets. Keune and Henning (2003) pointed to the possibility of using spreadsheets to provide a dynamic way for the students to test their solutions by slightly changing their estimated values for each sub-problem in order to see what might be a large effect. At the college level, Vidal et al. (2017) discussed using Matlab to calculate estimated volumes of different shaped mountains.
A main research result with respect to problem solving is that the Fermi method can successfully be taught (Raviv et al. 2016; Barahmeh et al. 2017). Barahmeh et al. (2017) demonstrated this aspect in the context of teaching 9th grade students physics, and illustrated how the students improved their skills in taking measurements, making measurement predictions, and making productive use of measurements. Consistent with the claims of Robinson (2008), Barahmeh et al. stressed the value of the Fermi method for students to think freely and without predetermined formulas for solving the tasks, and for promoting discussions on different solutions. A way of teaching FPs, argued for by Anderson and Sherman (2010), is based on creating a graphical representation that structures and divides the main problem into different sub-problems, which provides the students with a tool to organize their work. Taken together, the studies mentioned above exemplify the emphasis identified in the literature that FPs promote the learning of generic problem solving skills in terms of general reasoning skills and metacognition.
3.4 Modeling
According to Robinson (2008), in order to solve a FP, one has to: (1) synthesize a physical model; (2) examine the relevant physical principles applicable; (3) determine constraints and boundary conditions; (4) decide how simple the model can be while still maintaining realism; and (5) apply some rough estimation. Using this approach, Robinson taught first year college physics by proposing a problem and then allowing the students to generate their own methods and solutions, before engaging the students in discussing these among themselves in relation to a solution provided by the teacher. By studying the impact of the Fermi method on students’ examination marks Robinson showed that students can learn the Fermi method to solve FPs effectively, but Robinson found that this approach to develop more general problem-solving competencies was challenging for some students.
In mathematics education, multiple studies have investigated the potential of FPs to introduce mathematical modeling with students of different age groups. In upper primary school (ages 10–12), Peter-Koop (2009) used FPs to analyze students’ problem solving strategies, and concluded that (a) students solve problems in several ways; (b) students develop new mathematical knowledge while solving the problem; and (c) students display problem-solving processes that are multi-cyclic in nature and relatable to the modeling cycle (cf. Blum and Leiss 2007). Other studies demonstrated the following: how primary school students engaged in modelling when solving FPs focusing on collecting and processing real data (Henze and Fritzlar 2010); the great variety of mathematical models that students generated when solving FPs (Albarracín and Gorgorió 2019); and the positive development of the modeling sub-competences such as simplify, mathematize, interpret and explain (Haberzettl et al. 2018).
Ärlebäck (2009) analyzed the modeling activities of groups of secondary school mathematics students engaged in solving a FP and highlighted the importance of social relationships between the students, and the role of extra-mathematical knowledge in the problem solving situation. The discussion within the groups was a key factor in generating new mathematical knowledge connected to content from other disciplines (Ärlebäck and Frejd 2013). Also working with secondary students, Albarracín and Gorgorió (2014) investigated students’ proposed solving strategies on several FPs and observed that those FPs that allowed an effective solution to the problems were connected to the construction of mathematical models. Similarly, by characterizing the outputs produced by secondary school students from a sequence of FPs, Ferrando et al. (2017) identified the procedures and concepts that shaped the students’ models. Ferrando et al. concluded that experienced students used a greater number of concepts to develop their models and that this resulted in more accurate and realistic models when compared to the models developed by students without prior modeling experience. Albarracín and Gorgorió (2018) showed that sequences of FPs can encourage secondary students to construct mathematical models that successively better describe reality, allowing them to connect different mathematical concepts in order to create a useful model in various situations. Their study also showed that students changed the conceptual strategies that supported their models based on procedural obstacles rather than on conceptual limitations.
Lastly, Czocher (2016) used FPs with engineering students and confirmed that the modeling processes needed to solve a FP are complex even for these students, requiring them to regulate their modeling processes by monitoring how their immediate goals or subgoals are related to the problem statement. In a follow-up study, Czocher (2018) showed that college students do not construct their models independently of validation, but that validating is an integral part of the model construction process.
The articles collected in this section show that FPs can be used as modeling tasks with different goals depending on the age of the students. For example, in primary education FPs can be used to introduce modelling, in secondary education they can support students to connect or refine knowledge from different disciplines, and in higher education FPs can be used to introduce modeling as well as fostering self-monitoring and highlighting validation processes involved in the modelling process.
4 Discussion and conclusions
The review of the literature on FPs in the STEM disciplines summarized above shows a wide range of usages for FPs and the Fermi method. All the reviewed articles promote the use of FPs for various reasons that in one way or another are connected to at least one of the two main characteristics of the Fermi method: modeling a situation by simplifying and decomposing the initial problem into sub-problems; or deriving and using appropriate estimates to make progress on (sub-)problems. Based on the results from the previous sections, we now discuss FPs as facilitators and integrators among the different STEM disciplines, and how working on FPs can support students in developing twenty-first century STEM competencies.
4.1 FPs as integrators between STEM disciplines
Creating models to describe, explain and make predictions about different phenomena is fundamental in the sciences and engineering (Hestenes 2010). This role of modeling is consistent with the view in mathematics education that models are conceptual systems constructed to describe, explain or predict some other systems for achieving clearly recognized goals (Lesh and Harel 2003). Our review of the literature reveals numerous articles highlighting the potential of FPs to introduce and engage students in creating models that describe phenomena or situations specific to science (Cordry 2010; Efthimiou and Llewellyn 2006; Robinson 2008), engineering (Shakerin 2006), or economics (Anderson and Sherman 2010). We have also shown that the literature on FPs promotes problem-solving competencies in contextualized situations in the teaching and learning of mathematics (Albarracín and Gorgorió 2014, 2018; Ärlebäck 2009; Czocher 2016, 2018; Ferrando et al. 2017; Gallart et al. 2017; Keune and Henning 2003; Peter-Koop 2009). All these studies have in common that they confront students with accessible realistic situations and require an analysis using the Fermi method as a first attempt to create a model, allowing the students to develop their methods and solutions by discussing these among themselves. Mathematization of realistic phenomena is a key process for creating conceptual models and developing meaning and relevant concepts within and across disciplines. Since real phenomena are not neatly delimited and concerned only with a single knowledge domain, the integration of content and disciplines through FPs comes naturally as a consequence of well chosen and designed FPs and the use of the Fermi method to approach the tasks. When studying a particular phenomenon, different questions can and do arise, belonging to different disciplines, which elicits the need for students to identify, explore and understand relevant quantities from the various disciplines. In other words, the type of modeling required when working on a FP can act as an integrating activity for the STEM disciplines.
4.2 FPs as a facilitator for learning in the STEM disciplines
One of the features that define FPs is the need to deal with sub-problems based on estimates or educated guesses (Sriraman and Knott 2009). Indeed, in solving FPs it is possible for students to engage in making two different kind of estimations: computational- and measurement estimation (cf. Hogan and Brezinski 2003). What is essential however, is that the process of assigning values to the quantities involved in a problem is intimately connected to understanding the concepts and quantities being estimated (Resnick et al. 2017).
In schools, measurement estimation is often part of the mathematics curricula as a way of introducing and consolidating the learning of measurement (Sarama and Clements 2009). By tradition, measurement is learned in the discipline of mathematics where basic concepts and quantities are defined and students use different methods to measure these quantities (e.g., length, area, volume, time). A key aspect for students to estimate quantities in an appropriate way is the development of a well-defined set of mental units of reference on which to base their estimates (Bright 1976). However, when dealing with any problem in a real situation the relevant quantities often belong to disciplines other than mathematics, such as life sciences (heart rate) or physics (energy). Vasterink (2011) argued that competence in estimation is universal to all the sciences and is widely applicable to and used in many other disciplines. In essence, estimation allows results to be obtained for quantities that otherwise can be almost impossible to measure. The Fermi method provides a tool for reducing the complexity of the task at hand. This reduction of complexity allows students to approach the solution in an alternative way by considering discipline specific quantities and relationships in order to simplify the situation to more manageable sub-problems and by using estimates. In the Fermi method, working with and understanding individual quantities and their relationships within different disciplines comes to the fore. It is in this sense FPs work as facilitators for learning in the STEM disciplines. Indeed, Vasterink (2011) argued that FPs can be a central part of the learning of estimation in the different STEM disciplines. This is exemplified in science by Chesnutt et al. (2018) who investigated the role of estimation on students’ developing understanding of size scale, and the study of Resnick et al. (2017) on using scale as the basis for comparing size using relational reasoning. These last two studies illustrate that FPs on interdisciplinary themes involving different scales (from the nanoscale to the macro scale) can be a productive way to teach about concepts such as geological time and about estimating size and scale.
In engineering, estimation plays a particularly important role in the preliminary stages of the engineering design process when decisions have to be made based on incomplete information or partly unavailable data, or when selections must be made from a multitude of options (Shakerin 2006). Estimation is often used to find answers to ill-defined problems, when detailed solutions not are required, or to check the validity of answers obtained experimentally. Successful estimation in professional engineering practice is often based on the knowledge of dimensions and units, basic laws of physics, and the ability to define relationships and make comparisons (Joshi et al. 2013). This use of estimation in workplace environments has been proposed as one of the twenty-first century STEM competencies in product design (Jang 2016).
4.3 Connection to twenty-first century skills
In relation to the development of twenty-first century competencies, this review shows that FPs promote the learning of several skills and competencies identified by Binkley et al. (2012) within and across multiple disciplines. When working on ill-defined problems, FPs engage students in problem solving, helping them learn how to deal with complex situations by developing their own strategies for tackling unfamiliar problems (Moore 1987).
Researchers and practitioners have argued that FPs promote communication and collaboration skills by engaging students in group work and class discussions, both within single and across different disciplines (Efthimiou and Llewellyn 2006; Ärlebäck 2009). Although FPs generally are accessible to students, in a multidisciplinary context they naturally elicit non-trivial discussion and critical reflection on the selection and use of relevant concepts, constructs, and needed data (estimates). This way of working supports the students in developing critical thinking skills when they evaluate the potential of each of the different strategies and solutions generated to solve the problem (Seiwald 2016). Students’ critical thinking skills are also developed when they see how strategies and solutions can be used in other problems using the same basic strategies adjusted to other contexts (Albarracín and Gorgorió 2018). The Fermi method provides students with a tool to explore ways of thinking and working without being tied to a concrete formula or routine that must be learned and used, but rather students can work freely, making their own decisions and developing as flexible thinkers (Barahmeh et al. 2017; White 2004).
Some authors highlight that aspects of citizenship, information literacy and personal and social responsibility can be actualized for students through working with FPs situated in cultural, social and environmental settings. Examples of this aspect include FPs on the consumption of fossil fuels (Carlson 1997) and critical analysis of newspaper information (Albarracín and Gorgorió 2015).
4.4 Final remarks and future research
Since FPs originated in the context of physics to coordinate theoretical knowledge with experimental work, and transitioned into physics classrooms as a didactic tool, their usages have expanded to other disciplines. However, much of the research on FPs is discipline specific and unconnected, with little cross-discipline referencing. In this review, we have connected isolated results on FPs, which, when taken together, suggest a promising potential for integrating learning in the STEM disciplines with the development of estimation skills and modeling as ways of making complex phenomena and concepts accessible to students. However, more systematic research on the teaching and learning, using FPs that are cross-disciplinary in nature, is needed. Given the results of the review, sequences of FPs might be a promising line of inquiry.
The research shows that the Fermi method can be taught effectively and hence provide an accessible tool for teachers and learners. The research on FPs also shows that students can engage in solving real complex and realistic problems and that students can reflect on the impact of their results, sometimes changing their beliefs about the problems that affect the world. In this way, FPs have the potential to promote the development of many of the twenty-first century skills, not only to develop skills that enable high and better performance in the workplace, but also for people to grow individually and as citizens, by fostering the critical thinking necessary to meet the challenges of tomorrow.
A largely unexplored research area identified in the review is that of research on FPs used with technology. However, the few studies found suggest that technology might be a productive way for students to deal with complex cross-disciplinary FPs, which in turn could contribute to students developing ICT literacy.
Similarly to most of the papers we have reviewed, in this paper we have focused on the potential of using FPs and not on limitations and challenges associated with working with FPs and the Fermi method. Although sparely mentioned in the literature, we consider limitations and challenges important to investigate in the future and in developing this area of research.
Given both the strong connection between the learning of measurement and the development of number sense, and that individuals in numerous diverse professions report applied measurement estimation skills to be a critical component for success in their careers and as a tool to foster critical thinking, we concur with Sriraman and Knott (2009), who argued that the importance given to learning measurement estimation and identifying key aspects of a problem (i.e., the Fermi method) should not be limited to just those students who will pursue careers in STEM fields, but rather to all students.
References
Albarracín, L., & Gorgorió, N. (2014). Devising a plan to solve Fermi problems involving large numbers. Educational Studies in Mathematics, 86(1), 79–96.
Albarracín, L., & Gorgorió, N. (2015). On the role of inconceivable magnitude estimation problems to improve critical thinking. In U. Gellert, J. Giménez, C. Hahn, & S. Kafoussi (Eds.), Educational paths to mathematics (pp. 263–277). Dordrecht: Springer.
Albarracín, L., & Gorgorió, N. (2018). Students estimating large quantities: From simple strategies to the population density model. EURASIA Journal of Mathematics, Science and Technology Education, 14(10), 1–15.
Albarracín, L., & Gorgorió, N. (2019). Using large number estimation problems in primary education classrooms to introduce mathematical modelling. International Journal of Innovation in Science and Mathematics Education, 27(2), 33–45.
Anderson, P. M., & Sherman, C. A. (2010). Applying the Fermi estimation technique to business problems. Journal of Applied Business and Economics, 10(5), 33–42.
Ärlebäck, J. B. (2009). On the use of realistic Fermi problems for introducing mathematical modelling in school. The Mathematics Enthusiast, 6(3), 331–364.
Ärlebäck, J. B., & Frejd, P. (2013). Modelling from the perspective of commognition–An emerging framework. In G. Stillman, G. Kaiser, W. Blum, & J. P. Brown (Eds.), Teaching mathematical modelling: Connecting to research and practice (pp. 47–56). Dordrecht: Springer.
Barahmeh, H. M., Hamad, A. M. B., & Barahmeh, N. M. (2017). The effect of Fermi questions in the development of science processes skills in physics among Jordanian ninth graders. Journal of Education and Practice, 8(3), 186–194.
Bentley, J. (1999). Excerpt from programming Pearls: The back of the envelope. IEEE Software, 16(5), 121–125.
Binkley, M., Erstad, O., Herman, J., Raizen, S., Ripley, M., Miller-Ricci, M., et al. (2012). Defining twenty–first century skills. In P. Griffin, B. McGaw, & E. Care (Eds.), Assessment and teaching of 21st century skills (pp. 17–66). Dordrecht: Springer.
Blum, W., & Leiss, D. (2007). “Filling Up”—the problem of independence–preserving teacher interventions in lessons with demanding modelling tasks. In M. Bosch (Ed.), Proceedings of the Fourth Congress of the European Society for Research in Mathematics Education (pp. 1623–1633). Sant Feliu de Guixols, Spain: ERME.
Bright, G. W. (1976). Estimation as part of learning to measure. In D. Nelson, & R. Reys (Eds.), Measurement in school mathematics: 1976 yearbook. National Council of Teachers of Mathematics Yearbook: Reston.
Bybee, R. (2010). Advancing STEM education: a 2020 vision. Technology and Engineering Teacher, 70(1), 30–35.
Carlson, J. E. (1997). Fermi problems on gasoline consumption. The Physics Teacher, 35(5), 308–309.
Chesnutt, K., Jones, M. G., Hite, R., Cayton, E., Ennes, M., Corin, E. N., et al. (2018). Next generation crosscutting themes: Factors that contribute to students’ understandings of size and scale. Journal of Research in Science Teaching, 55(6), 876–900.
Clark, D. B., & Sengupta, P. (2013). Argumentation and modeling: Integrating the products and practices of science to improve science education. In M. Khine, & I. Saleh (Eds.), Approaches and strategies in next generation science learning (pp. 85–105). Hershey: IGI Global.
Cordry, S. M. (2010). Thermodynamics and human population, The Physics Teacher, 48(6), 403–407
Czocher, J. A. (2016). Introducing modeling transition diagrams as a tool to connect mathematical modeling to mathematical thinking. Mathematical Thinking and Learning, 18(2), 77–106.
Czocher, J. A. (2018). How does validating activity contribute to the modeling process? Educational Studies in Mathematics, 99(2), 137–159.
Efthimiou, C. J., & Llewellyn, R. A. (2006). Avatars of Hollywood in physical science. The Physics Teacher, 44, 28–33.
English, L. D. (2017). Advancing elementary and middle school STEM education. International Journal of Science and Mathematics Education, 15(1), 5–24.
Ferrando, I., Albarracín, L., Gallart, C., García-Raffi, L. M., & Gorgorió, N. (2017). Analysis of mathematical models produced when solving Fermi problems. Bolema Boletim de Educação Matemática, 31(57), 220–242.
Fitzallen, N. (2015). STEM education: What does mathematics have to offer? In M. Marshman, V. Geiger, & A. Bennison (Eds.), Proceedings of the 38th Annual Conference of the Mathematics Education Research Group of Australia (pp. 237–244). Sydney: Australia: MERGA.
Furjanic, D., & Müller, R. (2001). Fermiprobleme im unterrichtspraktischen Einsatz. Praxis der Natur–wissenschaften—Physik in der, 50(8), 35–26.
Gallart, C., Ferrando, I., García-Raffi, L. M., Albarracín, L., & Gorgorió, N. (2017). Design and implementation of a tool for analysing student products when they solve Fermi problems. In G. A. Eilerts, W. Blum, & G. Kaiser (Eds.), Mathematical modelling and applications. Crossing and researching boundaries in mathematics education (pp. 265–275). Cham: Springer.
Haberzettl, N., Klett, S., & Schukajlow, S. (2018). Mathematik rund um die Schule—Modellieren mit Fermi-Aufgaben. In K. Eilerts, & K. Skutella (Eds.), Neue Materialien für einen realitätsbezogenen Mathematikunterricht 5. Ein ISTRON-Band für die Grundschule (pp. 31–41). Wiesbaden: Springer Spectrum.
Henze, J., & Fritzlar, T. (2010). Primary school children’s model building processes by the example of Fermi questions. In A. Ambrus & E. Vásárhelyi (Eds.), Problem Solving in Mathematics Education. Proceedings of the 11th ProMath conference September 3-6, 2009 in Budapest (pp. 60–75). Budapest: Eötvös Loránd University.
Hestenes, D. (2010). Modeling theory for math and science education. In R. A. Lesh, P. Galbraith, C. Haines, & A. Hurford (Eds.), Modeling students’ mathematical modeling competencies (ICTMA 13) (pp. 13–41). New York: Springer.
Hogan, T. P., & Brezinski, K. L. (2003). Quantitative estimation: One, two, or three abilities? Mathematical Thinking and Learning, 5(4), 259–280.
Holubova, R. (2017). STEM education and Fermi problems. In L. Valovicova, & J. Ondruska (Eds.), Proceedings of the 20th International Conference DIDFYZ (Vol. 1804, No. 030001, pp 1–6). Rackova Valley, Slovakia: AIP Publishing.
Honey, M., Pearson, G., & Schweingruber, H. (Eds.). (2014). STEM integration in K-12 education: Status, prospects, and an agenda for research. Washington, DC: National Academies Press.
Jang, H. (2016). Identifying 21st century STEM competencies using workplace data. Journal of Science Education and Technology, 25(2), 284–301.
Joshi, M. S., Denson, N., & Downes, A. (2013). Quant job interview questions and answers. Parkville: Pilot Whale Press.
Kelley, T. R., & Knowles, J. G. (2016). A conceptual framework for integrated STEM education. International Journal of STEM Education, 3(1), 11.
Kennedy, T. J., & Odell, M. R. L. (2014). Engaging students in STEM education. Science Education International, 25(3), 246–258.
Keune, M., & Henning, H. (2003). Modelling and spreadsheet calculation. In Q. X. Ye, W. Blu, S.-K. Houston, & Q. Y. Yiab (Eds.), Mathematical modelling in education and culture (pp. 101–110). Chichester: Horwood.
Lesh, R., & Harel, G. (2003). Problem solving, modeling, and local conceptual development. Mathematical Thinking and Learning, 5(2), 157–189.
Machtans, C. S., & Thogmartin, W. E. (2014). Understanding the value of imperfect science from national estimates of bird mortality from window collisions. The Condor, 116(1), 3–7.
Magiera, M. T. (2013). Model eliciting activities: A home run. Mathematics Teaching in the Middle School, 18(6), 348–355.
Moore, J. L. (1987). Back–of–the–envelope problems (GK–3). California: Berkeley University.
Morgan, D. L. (2017). Measuring the effect of an astrobiology course on student optimism regarding extraterrestrial life. International Journal of Astrobiology, 16(3), 293–295.
Palmér, H., Johansson, M., & Karlsson, L. (2018). Teaching for entrepreneurial and mathematical competences: teachers stepping out of their comfort zone. In H. Palmér, & J. Skott (Eds), Students’ and teachers’ values, attitudes, feelings and beliefs in mathematics classrooms (pp. 13–23). Dordrecht: Springer.
Peter-Koop, A. (2009). Teaching and understanding mathematical modelling through Fermi–problems. In B. Clarke, B. Grevholm, & R. Millman (Eds.), Tasks in primary mathematics teacher education (pp. 131–146). Dordrecht: Springer.
Phillips, R., & Milo, R. (2009). A feeling for the numbers in biology. Proceedings of the National Academy of Sciences, 106(51), 21465–21471.
Raviv, D., Harris, A., & Dezotti, T. (2016). Estimation as an essential skill in entrepreneurial thinking. In Proceedings 123rd ASEE annual conference and exposition. New Orleans, LA: American Society for Engineering Education.
Resnick, I., Davatzes, A., Newcombe, N. S., & Shipley, T. F. (2017). Using relational reasoning to learn about scientific phenomena at unfamiliar scales. Educational Psychology Review, 29(1), 11–25.
Reys, R. E. (1984). Mental computation and estimation: Past, present, and future. The Elementary School Journal, 84(5), 547–557.
Robinson, A. W. (2008). Don’t just stand there—teach Fermi problems! Physics Education, 43(1), 83–87.
Sarama, J., & Clements, D. H. (2009). Early childhood mathematics education research: Learning trajectories for young children. London: Routledge.
Schukajlow, S., Kaiser, G., & Stillman, G. (2018). Empirical research on teaching and learning of mathematical modelling: A survey on the current state-of-the-art. ZDM Mathematics Education, 50(1–2), 5–18.
Seiwald, L. (2016). Fermi-Aufgaben—Nähern und Abschätzen. Delta Phi B, 2016, 1–7.
Shakerin, S. (2006). The art of estimation. International Journal of Engineering Education, 22(2), 273–278.
Shaughnessy, M. (2013). Mathematics in a STEM context. Mathematics Teaching in the Middle School, 18(6), 324.
Siegel, A. W., Goldsmith, L. T., & Madson, C. R. (1982). Skill in estimation problems of extent and numerosity. Journal for Research in Mathematics Education, 13(3), 211–232.
Sriraman, B., & Knott, L. (2009). The mathematics of estimation: Possibilities for interdisciplinary pedagogy and social consciousness. Interchange, 40(2), 205–223.
STEM Task Force Report. (2014). Innovate: a blueprint for science, technology, engineering, and mathematics in California public education. Dublin, California: Californians Dedicated to Education Foundation.
Taggart, G. L., Adams, P. E., Eltze, E., Heinrichs, J., Hohman, J., & Hickman, K. (2007). Fermi questions. Mathematics Teaching in the Middle School, 13(3), 164–167.
Tangney, B., & Bray, A. (2013). Mobile technology, maths education & 21C learning. Proceedings of the 12th world conference on mobile and contextual learning, (pp. 20–27). Doha, Qatar: College of the North Atlantic—Qatar.
Templier, M., & Paré, G. (2015). A framework for guiding and evaluating literature reviews. Communications of the Association for Information Systems, 37(6), 112–137.
Vasterink, R. P. (2011). Een schoorsteen van 1800 meter hoog (Master thesis). Universiteit Utrecht, Netherlands.
Vidal, A., Estruch, V. D., & Boigues, F. J. (2017). Flipped teaching aplicado al estudio de los métodos elementales de integración aproximada. Una experiencia educativa. In R. Roig-Vila, J. M. Antolí, J. Blasco, A. Lledó, & N. Pellín (Eds.), Redes colaborativas en torno a la docencia Universitaria (pp. I392–401). Alacant: Universitat d’Alacant.
Wagner, D., & Davis, B. (2010). Feeling number: Grounding number sense in a sense of quantity. Educational studies in Mathematics, 74(1), 39–51.
White, H. B. (2004). Math literacy. Biochemistry and Molecular Biology Education, 32(6), 410–411.
Acknowledgements
Open access funding provided by Linköping University. The authors thank Helen M. Doerr for her comments and suggestions on an earlier draft of this work. Lluís Albarracín is a Serra Húnter Fellow in UAB and supported by the projects EDU2017-82427-R (Ministerio de Economía, Industria y Competitividad, Spain), 2017 SGR 497 (AGAUR, Generalitat de Catalunya) and José Castillejo program (CAS17/00289 reference).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Ärlebäck, J.B., Albarracín, L. The use and potential of Fermi problems in the STEM disciplines to support the development of twenty-first century competencies. ZDM Mathematics Education 51, 979–990 (2019). https://doi.org/10.1007/s11858-019-01075-3
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11858-019-01075-3