Analysis of the Solidus Temperature of Multicomponent Steel by a Finite Thickness Model with Heat- and Solute-Transfer Equations in the Solid–Liquid Zone

Abstract

To examine the assumption of a constant solidus temperature—which has been empirically adopted in general heat analyses without firm validation—in all solidification stages for multicomponent steels, heat- and solute-transfer equations were simultaneously solved using the finite thickness model, which focuses on early-to-late-stage solidification except final-stage solidification. In early-to-middle-stage solidification, the model provides a constant solidus temperature, as predicted by the previously reported semi-infinite thickness model wherein the solidification front was far from the strand center. In late-stage solidification, however, the present model exhibited a slightly decreased solidus temperature—almost within the temperature measurement accuracy range. This suggests that the assumption of a constant solidus temperature does not exactly hold in late-stage solidification but is not unreasonable from a practical viewpoint. The obtained solutions agree well with numerical analyses and are in reasonable agreement with thermoanalytical measurements and industrial findings. Thus, the present model supports the assumption of a constant solidus temperature and estimates the solidus temperature in early-to-late-stage solidification, which can play a role in search of an adequate solidus temperature as an approximate analytical solution for multicomponent steels.

Appendices

Appendix A

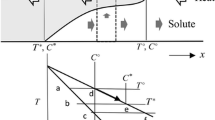
The equivalent surface temperature^[9] for extracting the same amount of heat from continuously cast steel is approximated by assuming the surface temperature (T^s) decreases stepwise, where T^s is kept constant within a step as follows:

The solid temperature is given by Eq. [11] as follows:

$$ T = \frac{{(T^{*} - T^{\text{ s}} )}}{{{\text{erf}}(\eta_{1}^{*} )}}{\text{erf}}(\eta_{1} ) + T^{\text{ s}} , $$

(A1)

where η₁ is given by:

$$ \eta_{1} = \frac{x}{{2\sqrt {\beta_{1} t} }} . $$

(A2)

The heat extracted from the surface (x = 0, η₁= 0) per unit of time is given by Eqs. [A1] and [A2] as follows:

$$ q = \left. { - K_{1} \frac{\partial T}{{\partial x}}} \right|_{x = 0} = - K_{1} \frac{{(T^{*} - T^{\text{ s}} )}}{{{\text{erf}}(\eta_{1}^{*} )}} \, \frac{1}{{\sqrt {\pi \beta_{1} t} }} . $$

(A3)

Integrating Eq. [A3] from t = 0 to t° gives the total heat extraction:

$$ Q^{\text{real}} = \int_{0}^{{t^{ \circ } }} {q{\text{ d}}t} = - H \, \int_{0}^{{t^{ \circ } }} { \, \frac{{(T^{*} - T^{\text{ s}} (t))}}{\sqrt t }{\text{d}}t} , $$

(A4)

where H is given as follows:

$$ H = \frac{{K_{1} }}{{\sqrt {\pi \beta_{1} } {\text{{{erf}}(}}\eta_{1}^{*} )}} . $$

(A5)

When T^s is constant, the total heat extraction is obtained from Eq. [A4] as follows:

$$ Q^{{{\text{const}}}} = - 2H(T^{*} - T^{\text{ s}} )\sqrt {t^{ \circ } } . $$

(A6)

The equivalent surface temperature (T^s) is obtained using $Q^{{{\text{const}}}} = Q^{{{\text{real}}}}$ as follows:

$$ T_{{{\text{eq}}}}^{\text{ s}} = \frac{1}{{2\sqrt {t^{ \circ } } }}\int_{0}^{{t^{ \circ } }} {\frac{{T^{\text{ s}} (t)}}{\sqrt t }} {\text{ d}}t . $$

(A7)

Cicutti and Boeri^[62] proposed a similar method of evaluating $T_{\text{eq}}^{\text{ s}} \, ({\text{K}})$ for pure metals by adopting Neumann’s solution. In the present model, Eq. [11] was adopted for steel instead of Neumann’s solution.

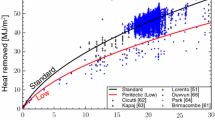
Meng and Thomas^[31] carefully calibrated the surface temperature transition with process data for mold and successive water spray-cooling zones for slab casting (V = 0.55 to 1.25 m/min). Referring to their process data, the equivalent surface temperature for continuously cast steel for slabs and blooms was obtained (Table AI). The cooling condition (i.e., transition surface temperature) for bloom casting—widely used in the 1970s—was estimated to match the process-data-calibrated numerical analysis.^[63] The adopted cooling condition wherein the water spray amount was defined as the specific spray water was 0.6 to 0.7 L/kg-steel, which corresponds to the cooling condition adopted during the shot bullet measurements^[56] with steels M1 and M2, (0.24-m-thick blooms, V = 1.0 m/min). In the 1970s, cast steels were widely intensively cooled at the upper spray zone to prevent internal cracks. The cumulative total heat extracted every 0.1 m from the meniscus (or every 0.05 m in the meniscus vicinity) to the target position (or until the targeted time) was obtained using the transient surface temperatures listed in Table AI and converted into the equivalent surface temperature ($T_{\text{eq}}^{\text{ s}}$) using Eq. [A7]. Notably, the finite thickness and semi-infinite thickness models both adopted the equivalent surface temperature ($T_{\text{eq}}^{\text{ s}}$) at the corresponding targeted time. As shown in Section IV–B, the finite thickness model agrees well with the numerical analysis, which adopted the solidus temperature predicted using the finite thickness model (Table V). To validate the equivalent surface temperature, the finite thickness model was compared with separately performed numerical analyses, which adopted the transient surface temperature (Table AI) denoted as the “as-cast numerical analysis.” Fig. A1 shows that the finite thickness model and the corresponding numerical analysis are both in fair agreement with the as-cast numerical analysis. Thus, the equivalent surface temperature obtained using the process data can be used to simplify the model.

Fig. A1

Comparison of results obtained using finite thickness model and numerical analysis—which both adopted equivalent surface temperature—and as-cast numerical analysis, which adopted transient surface temperature estimated using Steel K process data. Slab thickness: 22 × 10⁻² m, V = 0.015 m/s (0.9 m/min), ΔT = 15 K, $T^{ \, * }$ = 1746 K at 520 s, and $T^{\circ }$ = 1791 K. Mesh size: x increment dx = 6 × 10⁻⁵ m and time increment dt = 3.125 × 10⁻⁵ s

Appendix B

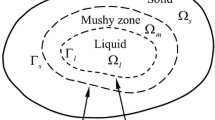
The equation set to obtain the approximate analytical solutions for the heat- and solute-transfer equations are summarized as follows:

[I] solid zone:

$$ T = \frac{{(T^{*} - T^{\text{ s}} )}}{{{\text{erf}}(\eta_{1}^{*} )}}{\text{erf}}(\eta_{1} ) + T^{\text{ s}} . $$

(11)

[II] mushy zone:

$$ T = \frac{{(T^{*} - T^{ \circ } )\left\{ {{\text{erf}}(\eta_{2} ) - {\text{erf}}(\eta_{2}^{ \circ } )} \right\}}}{{\left\{ {{\text{erf}}(\eta_{2}^{*} ) - {\text{erf}}(\eta_{2}^{ \circ } )} \right\}}} + T^{ \circ } . $$

(13)

[II] mushy zone solute concentration:

$$ C_{i} = \frac{{(C_{i}^{*} - C_{i}^{ \circ } )\left\{ {{\text{erf}}(\eta_{2} ) - {\text{erf}}(\eta_{2}^{ \circ } )} \right\}}}{{\left\{ {{\text{erf}}(\eta_{2}^{*} ) - {\text{erf}}(\eta_{2}^{ \circ } )} \right\}}} + C_{i}^{ \circ } . $$

(22)

[III] liquid zone:

$$ T(x,t) = \frac{{(T^{\text{i}} - T^{ \circ } )\left\{ {\varphi (x^{{}} ,t) - \varphi (x^{ \circ } ,t)} \right\}}}{{2 - \varphi (x^{ \circ } ,t)}} + T^{ \circ } , $$

(70)

where

$$ \varphi (x,t) = {\text{erf}}\left( {\frac{x}{{2\sqrt {\beta_{3} t} }}} \right) + {\text{erf}}\left( {\frac{2R - x}{{2\sqrt {\beta_{3} t} }}} \right). $$

(64)

It is noted that the solution set for Eqs. [11], [13], [22], and [70] is valid in the early-to-late-stage solidification and can be solved without determining the solidification stage in advance. The convergence is stably obtained within the model limit in late-stage solidification, although the limit depends on the steel composition.

Table BI lists the unknown variables, including the solidus temperature, and many physical properties used in the model.

Parameters λ_i and s_i in Eq. [3] are used to link the liquid fraction and concentration of solute i. λ_i is an important parameter that determines the solidus point solute concentration and yields the solute constraint conditions, as indicated by Eq. [33]. $M_{i} {\text{ and }}N_{i}$ are parameters for the liquid and solid interdiffusion coefficients, respectively.

Four unknown variables can be determined using Eqs. [7], [14], [33], and [76]. Herein, $\eta_{2}^{ * } , \, \eta_{2}^{ \circ } {\text{ and }}\beta_{2}$ (Table II) obtained using $T^{ \, * }$ yield solidification constants $\nu^{ * } {\text{ and }}\nu^{ \circ } \,$ by Eq. [9], and $\lambda_{i}$ yields $T^{ \, * }$ by Eq. [7]. The process for obtaining the set of unknown variables ($T^{ \, * } , \, \eta_{2}^{ * } , \, \eta_{2}^{ \circ } {\text{, and }}\lambda_{i}$) for the multicomponent system used in the semi-infinite thickness models^[8,9] was adopted in the present model. The left and right sides of Eqs. [14] and [76] are denoted as A, B, and C (B is commonly used). The unknown parameters were sought to minimize the function Z, which is defined as follows:

Z = (1 − A/B)² + (1 − C/B)²,

$$ A = \, \frac{{K_{1} (T^{*} - T^{\text{ s}} )\exp \left\{ {\left( {1 - \frac{{\beta_{2} }}{{\beta_{1} }}} \right)(\eta_{2}^{*} )^{2} } \right\}}}{{(T^{*} - T^{ \circ } ){\text{erf}}\left( {\sqrt {\frac{{\beta_{2} }}{{\beta_{1} }}} \, \eta_{2}^{*} } \right)\sqrt {\beta_{1} } }}, $$

$$ B = \frac{{K_{2} }}{{\left\{ {{\text{erf}}(\eta_{2}^{*} ) - {\text{erf}}(\eta_{2}^{ \circ } )} \right\}\sqrt {\beta_{2} } }}, $$

$$ C = \frac{{K_{3} (T^{\text{i}} - T^{ \circ } )\left( {e^{{ - (\eta_{3}^{ \circ } )^{2} }} - e^{{ - (\eta_{3}^{2R} - \eta_{3}^{ \circ } )^{2} }} } \right)e^{{(\eta_{2}^{ \circ } )^{2} }} }}{{(T^{ * } - T^{ \circ } )\left\{ {2 - {\text{erf}}(\eta_{3}^{ \circ } ) - {\text{erf}}(\eta_{3}^{2R} - \eta_{3}^{ \circ } )} \right\}\sqrt {\beta_{3} } }}, $$

$$ D \, : \, \frac{{\left\{ {1 - {\text{erf}}(\eta_{2}^{ \circ } )} \right\}}}{{\left\{ {{\text{erf}}(\eta_{2}^{*} ) - {\text{erf}}(\eta_{2}^{ \circ } )} \right\}}} = \frac{{2(1 - k_{i} )}}{{(2 - k_{i} - \alpha_{i} k_{i} )}}\lambda_{i} C_{i}^{ \circ } + \frac{{(1 + \alpha_{i} )k_{i} }}{{(2 - k_{i} - \alpha_{i} k_{i} )}} - \frac{{N_{i} }}{{M_{i} }}. $$

Note that $N_{i} {/}M_{i}$ is neglected because $N_{i} < < M_{i}$ in steel.

• 1. First, the initial values for $T^{*}$, $\eta_{2}^{*}$, and $\eta_{2}^{ \circ }$ are given. $T^{*}$ calculate staged using the reported formula is used as the initial value for $T^{*}$. The initial $\eta_{2}^{ \circ }$ is 1.0, and $\eta_{2}^{*}$ is given as $\eta_{2}^{*}$ = γ $\eta_{2}^{ \circ }$, where the initial γ = 0.3.

• 2. Second, $\eta_{2}^{ \circ }$ is changed to minimize Z for the initial T ^*. Convergence is quickly obtained.

• 3. Then, the value of η^* that minimizes Z is obtained by changing γ ($\eta_{2}^{*}$ = γ $\eta_{2}^{ \circ }$).

• 4. Substituting $\eta_{2}^{*}$ and $\eta_{2}^{ \circ }$ obtained using the process on the left side of Eq. [33] provides $\lambda_{i}$ for solute i (right side of D, shown above). $\lambda_{j}$ is obtained using the same procedure for the other solute (j). Substituting these $\lambda_{i}$ into Eq. [7] yields $T^{*}$. Using this $T^{*}$ as the initial $T^{*}$ and repeating the same process from steps (2) to (4) yields the set ($T^{*}$,$\eta_{2}^{*}$, $\eta_{2}^{ \circ }$, and $\lambda_{i}$) matching Eqs. [7], [14], [33], and [76].

• 5. This process is repeated until Z < 0.1 when the four decimal digits do not change in the analysis.