An Analytical Approach of One-Compartmental Pharmacokinetic Models with Sigmoidal Hill Elimination

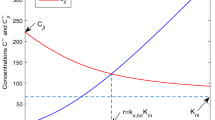
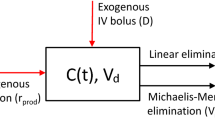
Abstract

In this study, we aim to develop the analytical solutions of one-compartment pharmacokinetic models with sigmoidal Hill elimination and quantitatively revisit some widely used pharmacokinetic indexes. For this purpose, we have first established the closed-form solutions of the model with intravenous bolus administration through introducing a transcendent H function, which is proved a generalized form of the Lambert W function. Then, in the case of a single dose, we have obtained the explicit formulas for several pharmacokinetic surrogates, such as the clearance, elimination half-life and partial/total drug exposure. All these surrogates are found concentration-dependent and sensitive to the Hill coefficient $\alpha$. Meanwhile, in establishing the closed-form formulas for multiple repeated dosing regimens, we have discovered phase transitions for steady states with different ranges of $\alpha$ in function of the lengths of dosing intervals. Further, our results are illustrated with two drug examples. To conclude, the present findings elucidate the intrinsic quantitative structural properties of pharmacokinetic models with Hill elimination and provide new knowledge for nonlinear pharmacokinetics and guidance for rational drug designs.

Appendices

Appendix A: Proof of Remark 3

From Eq. (24), if $0<\alpha <2$, $\mathrm{AUC}_{0-\infty }$ can be rewritten as

$$\begin{aligned} \mathrm{AUC}_{0-\infty } = \frac{1}{V_{\mathrm{max}}}\left[ \frac{K^{\alpha }_D}{2-\alpha }e^{(2-\alpha )\ln \frac{D}{V_\mathrm{d}}}+\frac{1}{2}(\frac{D}{V_\mathrm{d}})^2\right] . \end{aligned}$$

Using the fact $\ln (1+x)\approx x$ for x close to zero, we have $\ln \frac{D}{V_\mathrm{d}}=\ln (1+(\frac{D}{V_\mathrm{d}}-1))\approx (\frac{D}{V_\mathrm{d}}-1)$ for a low enough D. Doing the Taylor series expansion on D, we have

$$\begin{aligned} \mathrm{AUC}_{0-\infty }\approx & {} \frac{1}{V_{\mathrm{max}}}\left[ \frac{K^{\alpha }_D}{2-\alpha }e^{(2-\alpha )(\frac{D}{V_\mathrm{d}}-1)}+\frac{1}{2}(\frac{D}{V_\mathrm{d}})^2\right] \\= & {} \frac{1}{V_{\mathrm{max}}}\left[ \frac{K^{\alpha }_D}{2-\alpha }(1+(2-\alpha )(\frac{D}{V_\mathrm{d}}-1)+o((2-\alpha )(\frac{D}{V_\mathrm{d}}-1))) + \frac{1}{2}(\frac{D}{V_\mathrm{d}})^2 \right] \\= & {} \frac{K_\mathrm{D}^{\alpha }}{V_{\mathrm{max}}(2-\alpha )}+\frac{1}{V_{\mathrm{max}}}(\frac{D}{V-d}-1)+\frac{1}{2V_{\mathrm{max}}}(\frac{D}{V_\mathrm{d}})^2+o(\frac{D}{V_{\mathrm{d}}}-1)\\\approx & {} \frac{K_\mathrm{D}^{\alpha }}{V_{\mathrm{max}}(2-\alpha )}. \end{aligned}$$

However, for a large enough D, we can deduce from Eq. (24) that $\mathrm{AUC}_{0-\infty }$ is predominantly determined by the quadratic term w.r.t. D, namely $\frac{1}{2V_{\mathrm{max}}}(\frac{D}{V_\mathrm{d}})^2$. When $\alpha =1$, we have calculated $\mathrm{AUC}_{0-\infty }=\frac{K_\mathrm{D}}{V_{\mathrm{max}}}\frac{D}{V_\mathrm{d}}+\frac{1}{2V_{\mathrm{max}}}(\frac{D}{V_\mathrm{d}})^2$, which is also predominantly determined by the latter term $\frac{1}{2V_{\mathrm{max}}}(\frac{D}{V_\mathrm{d}})^2$ for a large enough dose D.

Appendix B: Sensitivity Indices Calculation

• (i) By Definition (27) and Eq. (5), the sensitivity index of C(t) to $\alpha$ is $\lambda ^{C(t)}_{\alpha }=\frac{\partial C(t)}{\partial \alpha }\frac{\alpha }{C(t)}$, and

  $$\begin{aligned} \frac{\partial C(t)}{\partial \alpha }= & {} \frac{K_\mathrm{D}^{\alpha }}{1-\alpha }\left[ \left( C_0^{1-\alpha }-C^{1-\alpha }(t)\right) \left( \frac{1}{1-\alpha }+\ln K_\mathrm{D}\right) \right. \nonumber \\&\quad \left. -\, \left( C_0^{1-\alpha }\ln C_0-C^{1-\alpha }(t)\ln C(t)\right) \right] . \end{aligned}$$

  (38)

• (ii) From Eq. (17), the sensitivity index of $T_{1/2}$ to $\alpha$ is $\lambda ^{ T_{1/2}}_{\alpha }=\frac{\partial T_{1/2}}{\partial \alpha }\frac{\alpha }{ T_{1/2}}$, and

  $$\begin{aligned} \frac{\partial T_{1/2}}{\partial \alpha }&= \frac{K_\mathrm{D}}{(\alpha -1)V_{\max }}\left( \frac{K_\mathrm{D}}{C(t)}\right) ^{\alpha -1} \left[ 2^{\alpha -1}\ln 2-\frac{2^{\alpha -1}-1}{\alpha -1}+(2^{\alpha -1}-1)\ln \frac{K_\mathrm{D}}{C(t)}\right] \nonumber \\&\quad +\, \left[ \frac{1}{2V_{\max }}-\frac{2^{\alpha -1}-1}{V_{\max }}\left( \frac{K_\mathrm{D}}{C(t)}\right) ^{\alpha }\right] \frac{\partial C(t)}{\partial \alpha }. \end{aligned}$$

  (39)

• (iii) From Eq. (25), the sensitivity indices of $\mathrm{AUC}_{0-\infty }$ to model parameters are

  $$\begin{aligned} \lambda _{\alpha }^{\mathrm{AUC}_{0-\infty }}&=\left( \frac{\alpha }{2-\alpha }+\alpha \ln \frac{K_\mathrm{D}}{C_0}\right) \Big /\left( 1+\frac{2-\alpha }{2}\left( \frac{C_0}{K_\mathrm{D}}\right) ^{\alpha }\right) , \end{aligned}$$

  (40)
  $$\begin{aligned} \lambda _{V_{\max }}^{\mathrm{AUC}_{0-\infty }}&=-1, \end{aligned}$$

  (41)
  $$\begin{aligned} \lambda _{K_\mathrm{D}}^{\mathrm{AUC}_{0-\infty }}&=\alpha \Big /\left( 1+\frac{2-\alpha }{2}\left( \frac{C_0}{K_\mathrm{D}}\right) ^{\alpha }\right) , \end{aligned}$$

  (42)
  $$\begin{aligned} \lambda _{V_\mathrm{d}}^{\mathrm{AUC}_{0-\infty }}&= (\alpha -2)\times \left( 1+\left( \frac{C_0}{K_\mathrm{D}}\right) ^{\alpha }\right) \Big /\left( 1+\frac{2-\alpha }{2}\left( \frac{C_0}{K_\mathrm{D}}\right) ^{\alpha }\right) . \end{aligned}$$

  (43)

Appendix C: Proof of Lemma 1

Proof

First, we assume Eq. (29) has a fixed point. As a sequence $\{C_{\max ,n}\}_{n=1}^{\infty }$ is non decreasing, we denote $C^{ss}_{\max }=\lim \limits _{n\rightarrow \infty }C_{\max ,n}>D/V_\mathrm{d}$. Following the definition of H(s) (Eq. (8)), we have

$$\begin{aligned} \frac{1}{1-\alpha }\left( \frac{C^{ss}_{\max }-D/V_\mathrm{d}}{K_\mathrm{D}}\right) ^{1-\alpha }+\frac{C^{ss}_{\max }-D/V_\mathrm{d}}{K_\mathrm{D}}=\frac{1}{1-\alpha }\left( \frac{C^{ss}_{\max }}{K_\mathrm{D}}\right) ^{1-\alpha }+\frac{C^{ss}_{\max }-V_{\max }T}{K_\mathrm{D}}, \end{aligned}$$

which can be simplified as

$$\begin{aligned} \left( C^{ss}_{\max }\right) ^{1-\alpha }-\left( C^{ss}_{\max }-\frac{D}{V_\mathrm{d}}\right) ^{1-\alpha }=\frac{V_{\max }(1-\alpha )}{K_\mathrm{D}^{\alpha }}\left( T-\frac{D}{V_\mathrm{d}V_{\max }}\right) . \end{aligned}$$

(44)

Consider the function $g:[D/V_\mathrm{d},\infty ) \longrightarrow \mathbb {R}$; $g(x)=x^{1-\alpha }-(x-D/V_\mathrm{d})^{1-\alpha }$, whose derivative is

$$\begin{aligned} g'(x)=(1-\alpha )\left( \frac{1}{x^{\alpha }}-\frac{1}{(x-D/V_\mathrm{d})^{\alpha }}\right) . \end{aligned}$$

(45)

We can notice that, for all positive $\alpha \not =1$, $\displaystyle \frac{1}{x^{\alpha }}<\frac{1}{(x-D/V_\mathrm{d})^{\alpha }}$ if $x>D/V_\mathrm{d}$. So, on $[D/V_\mathrm{d},\infty )$, g(x) is monotonically decreasing for $0<\alpha <1$ as $g'(x)<0$, and monotonically increasing for $\alpha >1$ as $g'(x)>0$.

Now, we discuss the asymptotic property of $C_{\max ,n}$.

• (i) $0<\alpha <1$. In this case, $g(D/V_\mathrm{d})=(D/V_\mathrm{d})^{1-\alpha }>0$ and $\lim \nolimits _{x\rightarrow +\infty }g(x)=0$. It indicates that g(x) monotonically decreases from $(D/V_\mathrm{d})^{1-\alpha }$ to 0, which conversely implies that Eq. (29) has a unique fixed point $C^{ss}_{\max }$ larger than $D/V_\mathrm{d}$ if and only if

  $$\begin{aligned} 0<\frac{V_{\max }(1-\alpha )}{K_\mathrm{D}^{\alpha }}\left( T-\frac{D}{V_\mathrm{d}V_{\max }}\right) <\left( \frac{D}{V_\mathrm{d}}\right) ^{1-\alpha }, \end{aligned}$$

  (46)

  or

  $$\begin{aligned} T_0^*=\frac{D}{V_\mathrm{d}V_{\max }}<T<\frac{D}{V_\mathrm{d}V_{\max }}\left( 1+\frac{1}{1-\alpha }\left( \frac{K_\mathrm{D}}{D/V_\mathrm{d}}\right) ^{\alpha }\right) =T_1^*. \end{aligned}$$

  (47)

  Otherwise, $C_{\max ,n}$ will continue to increase if $T<T_0^*$, or $C_{\min ,n}=0$ and $C_{\max ,n}=D/V_\mathrm{d}$ for all n if $T>T_1^*$. Particularly,

  $$\begin{aligned} \lim \limits _{\alpha \rightarrow 1^-}\frac{D}{V_\mathrm{d}V_{\max }}\left( 1+\frac{1}{1-\alpha }\left( \frac{K_\mathrm{D}}{D/V_\mathrm{d}}\right) ^{\alpha }\right) =+\infty \end{aligned}$$

  which is consistent with the condition of the existence of $C^{ss}_{\max }$ for one-compartment pharmacokinetic model with a single Michaelis–Menten elimination pathway (Tang and Xiao 2007).

• (ii) $\alpha >1$. In this case, we have $\lim \nolimits _{t\rightarrow (D/V_\mathrm{d})^+}g(x)=-\infty$ and $\lim \nolimits _{t\rightarrow +\infty }g(x)=0$. This indicates that g(x) monotonically increases from negative infinity to zero as x goes up from $D/V_\mathrm{d}$ to $+\infty$. Subsequently, Eq. (29) has a unique fixed point $C^{ss}_{\max }$ which is greater than $D/V_\mathrm{d}$ if and only if

  $$\begin{aligned} \frac{1-\alpha }{K_\mathrm{D}^{\alpha }}\left( V_{\max }T-\frac{D}{V_\mathrm{d}}\right) <0\Leftrightarrow T>\frac{D}{V_\mathrm{d}V_{\max }}=T_0^*. \end{aligned}$$