1 Introduction

Exploratory technologies for underground environments are usually highly cumbersome, energy-demanding, and intrusive. The need for high dexterity for moving into soil led to a growing interest in bioinspired approaches in geotechnics [26] and robotics [15, 42]. Among several, plant roots represent an important natural model displaying various efficient strategies of soil digging and exploration [23]. They colonize the underground by following environmental stimuli [5, 17] and exploiting low friction channels and cracks to move into the soil easily [23]. Unlike animals that use muscles to move, plant roots advance by growing at the apical region [4, 8, 14, 21, 41]. The root apex comprises different zones (Fig. 1) [23]: meristem or cell division, elongation, and maturation zones.

Fig. 1
figure 1

Scheme of the root structure and zonation. (a) Main root zones and features; (b) a successive evolution of the root in (a) after a certain period of time. The root has grown from the tip by cell division and elongation, lengthening its structure of a Δl. (c) Phenomenon of radial expansion that can occur above the tip

Full size image

In particular, the meristem zone includes an organizational center surrounded by stem cells that divide and proliferate in the meristem. In the transition zone, cells leave the meristematic status and elongate. Once their maturity is reached, they enter the mature zone and differentiate. The cells issued from the stem cells are moved away from the meristem zone by younger cells: New cell material is created continuously while the structure remains stationary in the environment. Both cell division and elongation thus produce the advancement of the root apex. This combination provides the required pressure (up to 1 MPa [33]) to penetrate soil successfully. This peculiar strategy of movement and other adaptive strategies of plant roots inspired several burrowing and soil exploratory systems [29, 36]. Mazzolai et al. (2011) [28] proposed for the first time a mechatronic system for soil penetration that mimicked the apical part of plant roots and could steer following gravity and moisture stimuli. Later, Sadeghi et al. [36, 37] proposed a robot-embedded additive manufacturing process to imitate the apical addition of material observed in plants and realized a robotic system capable of building its own body while showing its potential to dig in granular media. The authors also adopted a robot physical approach to verify the contribution of apical growth in improving penetration performances [37]. They demonstrated a reduction of 70% in energy consumption when the growth from the tip was employed with respect to pushing from the top of an analogous probe. In addition to axial growth, roots can implement a radial enlargement during development. When roots grow in soil with high mechanical impedance, they develop a thicker body than roots growing in less impeded soils [27, 32, 43]. Ticker roots penetrate compacted soils more successfully than thinner roots [27] and show higher resistance to bending and buckling [23]. The root radial growth has been hypothesized to create cracks in the soil ahead of the root tip [1, 6] facilitating penetration. Root thickening is challenging to analyze due to several physiological, chemical, and physical factors that govern the root growth process [1, 3, 7, 9, 19, 24, 27, 35, 43]. It is necessary to pose simplifications to be able to discriminate among parameters affecting plant growth. For example, the soil is often assumed to be a continuum medium, and parameters such as temperature, soil pH, soil aeration, the incidence of light, or nutrient status are kept at adequate and constant levels [6]. Besides plant roots, radial expansion is employed by other burrowing organisms (e.g., polychaetes, clams, and earthworms), and penetration performances of their different modalities of actuation have been investigated [10, 12, 20, 25, 30]. In [10], the authors analyzed numerically the penetration in granular soil of a bioinspired probe, which penetrates through a three-phase process: The probe is first pushed into the soil until reaching a target depth, then a region located behind the tip (named anchor) expands radially, and finally, the tip is displaced downward whereas the anchor is moved upward. The authors investigated the ability of the probe to generate enough anchorage forces to penetrate successfully. They found that (a) longer anchors and anchors with higher coefficients of friction can generate greater anchorage, and (b) anchors expanding radially temporarily reduce the penetration resistance. This study did not analyze how the penetration resistance is affected by soil properties (e.g., density and cohesion) and quantified only partially the penetration resistance decrease since there were no indications of the penetration depth affected by such reduction. In [30], the authors experimentally investigated the performances of a Polychaeta-inspired soft robot during locomotion in an artificial granular medium consisting of plastic polypropylene pellets. Specifically, the authors considered a robot whose central region elongates and the tip is actuated through periodic radial expansion or bidirectional bending. They examined the drag force exerted on the robot during locomotion by running experiments focused on the robot tip. The results showed that the actuation through radial expansion outperforms bidirectional bending since it implies a lower drag force. In [12], an earthworm-inspired probe penetrating in homogeneous clear mud analog was experimentally investigated. Specifically, this probe includes a region located behind the tip implementing radial expansion with a balloon mechanism. The authors observed a penetration resistance reduction due to the balloon inflation/deflation cycle and quantified the penetration depth affected by this resistance decrease. Additionally, this probe was numerically analyzed in a two-dimensional (2D) environment by considering penetration in dry Ottawa sand particles with no adhesive force [25]. The implemented balloon inflation/deflation cycle results in a penetration resistance reduction. However, no indications of the penetration depth affected by this reduction are provided. In both these studies, the effects of soil properties (as density or particle cementation) on the penetration resistance reduction and the extension of the soil volume affected by this reduction are not investigated. An additional contribution is represented by the analysis proposed in [20], where a clam-inspired burrowing system is numerically investigated assuming its locomotion to be described by a four-phase process, which includes the entire cylinder expansion, cone penetration, cylinder contraction, and cylinder retraction. The authors evaluated penetration resistance and energy consumption during dry sand penetration, and the results show that, with respect to pure cone penetration, the cylinder expansion induces a temporary reduction in penetration resistance, which increases again with further increase in penetration depth, and a slight decrease in energy consumption.

This paper aims to better understand the role of plant-inspired radial growth in penetration performances by investigating the effects over different soil conditions (varying density and inter-particle cementation) and proposes a three-dimensional (3D) numerical model for a plant rootlike simulant penetrating soil. A 3D discrete element model (DEM) [13, 31] was developed to simulate the apical growth into cohesionless granular and cemented media. In the former case, both dense and loose media are investigated. A comparative analysis is presented considering an intruder performing penetration with and without radial growth. To evaluate the penetration performances of the system, we estimated pressure and energy requirements and evaluated whether and in which soil conditions radial growth benefits soil penetration. This investigation also provides a methodology to extract design specifications for implementing efficient excavating robotic systems. Indeed, the model can be further elaborated to analyze the effects of intruder size, geometry, and other penetration strategies and define actuation system requirements for an artificial probe.

The proposed methodology is detailed in Sect. 2, followed by results in Sect. 3, and discussions with conclusive remarks in Sect. 4.

2 Methodology

2.1 Model setting and parameters

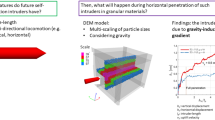
The numerical model was implemented in a 3D environment DEM simulator using the Yade software [39]. Our approach considers a representative elementary volume of soil having a cubic shape. Soil volume boundaries comprise 6 rigid, frictionless walls with a side length Dchamber. The simulated granular assembly comprises 116,696 heterogeneously sized spherical particles, characterized by a median particle size D50. The simulated rootlike intruder includes a cylindrical body and a conic tip. The cylindrical body and the base section of the cone have a diameter Droot, and the tip has an aperture angle \(2\alpha =60^\circ\). The axial soil resistance perceived by the intruder is analyzed at the steady-state conditions (i.e., when the average penetration force reaches a mostly stable value): Based on results from previous studies [16], the root tip is initially located at the center (in x, y, and z) of the soil volume. In order to reduce the boundary effects and to maintain computational efficiency in the DEM simulations, the relative dimensions of the chamber and penetrating root are fundamental [10, 22]. This work assessed input parameters by performing a sensitivity analysis (SA) (detailed in Appendix A). The SA results led to the selection of the root size-to-the median particle size ratio \(Droot/D50=6.8\) and the chamber size-to-root size ratio \(Dchamber/Droot=20\). Figure 2 shows a schematic of (a) the rootlike intruder and the soil chamber and (b) the contact models adopted to model the inter-particle and the root–particle interactions.

Fig. 2
figure 2

(a) Scheme of the considered soil chamber and rootlike intruder. (b) Scheme of the used elastic perfectly plastic contact model with dry friction plus rolling resistance

Full size image

Soil grains are approximated with spheres [11, 34, 40] with rolling resistance to simulate the mechanical behavior of assemblies of subrounded or subangular particles [2]. As the particle shape is different in the model with respect to real soil, the microstructure of the numerical packing, or even its porosity, is not identical to the one of the soil. Nevertheless, the effect of particle shape on the macroscopic soil response is represented accurately via the rolling friction introduced in the model at the scale of contacts [38]. The inter-particle and root–particle interactions were modeled using an elastic, perfectly plastic contact model with dry friction and rolling resistance. With respect to the contact plane between two spherical particles having radii \(R1\) and \(R2\), the normal Fn and shear (or tangential) Fs contact forces between the two spheres are expressed by Eqs. 1 and 2 in normal and tangential directions, respectively:

$${\varvec{F}}_{n} = K_{n} \delta_{n} {\varvec{n}}$$
(1)
$$\Delta {\varvec{F}}_{s} = - K_{s} \Delta {\varvec{U}}_{s} \;\;\;with\;\;\;{\varvec{F}}_{s} \le {\varvec{F}}_{n} tan\varphi_{c}$$
(2)

where \({K}_{n}\) and \({K}_{s}\) are, respectively, the normal and tangential stiffnesses, \({\varphi }_{c}\) represents the contact friction angle with a coefficient of contact friction \(\mu =tan{\varphi }_{c}\), \({\varvec{n}}\) is the normal unit vector to the contact plane, \({\delta }_{n}\) is the overlap distance between the spheres, and \({{\varvec{U}}}_{{\varvec{s}}}\) is the relative tangential displacement at the contact point. This law considers only normal compressive forces (in the lack of inter-particle adhesion), and the contact disappears when the overlap \({\delta }_{n}\) vanishes. Considering that the rolling resistance at the contact is expressed through the rolling stiffness \({K}_{r}\) and the coefficient of rolling friction\({\eta }_{r}\), the rolling moment Mr acting against the relative rolling rotation of particles \({\theta }_{r}\) is expressed as reported in Eq. (3):

$$\Delta {\varvec{M}}_{r} = - K_{r} \Delta \theta_{r} \;\;with\;\;{\varvec{M}}_{r} \le {\varvec{F}}_{n} \eta_{r} \min \left( {R1, \;R2} \right)$$
(3)

where \(\Delta {\theta }_{r}\) represents the tangential component of the incremental relative rotation of the contacting spheres. Contact stiffnesses are defined as functions of stiffness modulus Ec, shear-to-normal stiffness ratio αs, and rolling-to-shear stiffness ratio αr as expressed in Eq. (4):

$$K_{n} = 2E_{c} \frac{{R_{1} R_{2} }}{{R_{1} + R_{2} }};\;\; K_{s} = \alpha_{s} K_{n} ; \;\; K_{r} = \alpha_{r} R_{1} R_{2} K_{s}$$
(4)

Moreover, the contact law can include adhesive normal and tangential forces, which are defined from an adhesive stress σA as expressed in Eq. (5):

$${\varvec{F}}_{n}^{A} = {\varvec{F}}_{s}^{A} = \sigma_{A} \left[ {\min \left( {R1, \;R2} \right)} \right]^{2}$$
(5)

where σA has the dimension of stress, but it generates global adhesion forces independently of the size of the particles. In this case, the contact is characterized by resistance to a normal tensile force as expressed in Eq. (6), and the maximum tangential force against sliding is defined as in Eq. (7):

$${\varvec{F}}_{n } {\varvec{n}} > - {\varvec{F}}_{n}^{A}$$
(6)
$${\varvec{F}}_{s} \le ({\varvec{F}}_{n } {\varvec{n}})tan\varphi_{c} + {\varvec{F}}_{s}^{A}$$
(7)

This study simulates the intruder penetration in granular cohesionless and cemented soils characterized through drained triaxial compressions. Table 1 reports the DEM input parameters for the different case studies. In order to analyze how the penetration performances depend on the soil porosity, dense and loose cohesionless granular media were modeled. The dense and loose granular media result, respectively, dilatant and contractant when sheared during triaxial compression at a confining pressure of 100 kPa (Fig. 3a). The peak of the deviatoric stress corresponds to internal friction angles of 38.1° for the dense medium and 36.1° for the loose one (Fig. 3b). Furthermore, cemented soil is also represented, with drained triaxial compressions at 50 kPa and 100 kPa confining pressures. Figure 3c and d shows the macroscopic properties of the cemented soil model with cohesion of 69.2 kPa and internal friction angle of 31.5° (determined from the peaks of the deviatoric stress).

Table 1 DEM simulation parameters
Full size table
Fig. 3
figure 3

Simulations of drained triaxial compressions (porosity change on the left and deviatoric stress on the right) for dense and loose cohesionless granular soils at a confining pressure of 100 kPa (a, b) and a cemented soil at confining pressures of 50 kPa and 100 kPa (c, d)

Full size image

The soil penetration process consists of two main steps: First, the packing is initialized by creating a cloud of heterogeneously sized spheres not in contact with each other; then, it is compacted isotropically (resulting in the earth pressure coefficient at rest equal to 1) to reach a confining target pressure of 100 kPa. The porosity reached after the compaction is controlled by tuning the contact friction angle during compaction: Lower inter-particle friction leads to lower porosity. Once the packing compaction is completed, the contact friction angle is fixed to the nominal value given in Table 1, and the intruder starts penetrating by growing from the tip.

2.2 Intruder penetration implementation

The numerical model of the soil intruder is a rigid body composed of a stationary upper cylindrical region (in green in Fig. 2a) and an apical part with a conical shape that is pushed downward in the soil through growth (in blue in Fig. 2a). The intruder grows axially from the tip, as in plant roots, by adding a new body section (in red in Fig. 2a) between the green and the blue regions (Fig. 2a). As no mechanisms of viscous dissipation are introduced in the numerical model, the simulated response should be independent of the growth rate as long as the latter is sufficiently low for considering inertial terms as negligible. Results of the SA (Appendix A) on the axial growth rate show that the intruder penetration pressure is almost independent of the growth rate for rates equal to or lower than 1 m/s, suggesting that, in these conditions, the penetration pressure depends only on the mechanical properties of the contacts and the geometric arrangement of the particles. For higher penetration velocities, the penetration pressure may also be affected by the inertia of the soil particles and depend on the penetration velocity itself. The latter corresponds to a dynamic regime not considered in this study. Consequently, in this study, the growth is simulated at a rate of 1 m/s, and the simulation is assumed to represent the quasi-static response of the soil–intruder system. The root radial expansion process is performed by inflating a section of the intruder with a spherical shape until reaching a final diameter Dthickened. Three different values of Dthickened are analyzed: 1.5Droot, 2Droot, and 2.5Droot. As for the axial growth, the radial expansion occurs at a rate of 1 m/s since it represents the threshold value below which the speed effect is negligible.

The radial growth process is accompanied by a downward translational motion of the tip (at a rate of 1 m/s) to avoid overlapping between the growing section and the tip. Two different analyses were performed, referred to as Analysis I and Analysis II, for all the considered soil conditions. In Analysis I, the root is considered to penetrate soil by growing axially (Fig. 4a). In Analysis II, the root penetrates soil by following a three-phase process (Fig. 4b): (a) The root starts penetrating by growing axially; (b) after a tip displacement of 0.03 m, the axial growth process is temporarily interrupted, and the radial expansion process is activated; and (c) once the root expands its diameter until a certain value (Dthickened), the radial expansion process is interrupted, and the axial growth process resumes and continues for the remaining penetrating depth.

Fig. 4
figure 4

Schematic of the implementations used for modeling the rootlike intruder axial growth (Analysis I) and radial expansion (Analysis II)

Full size image

3 Results

3.1 Dense granular medium

In a dense granular medium, Analysis I shows a characteristic fluctuating behavior of the axial soil resistance pressure experienced by the tip around a value of 23 MPa (Fig. 5a). See Appendix A for an analysis of the noise on the estimated soil resistance pressure. Analysis II shows that the resistance pressure exerted by the soil on the root tip during penetration drops when the root grows radially. Specifically, the pressure decrease is more relevant when larger Dthickened are considered (Fig. 5b).

Fig. 5
figure 5

Penetration pressure when the intruder penetrates cohesionless dense granular soil by (a) growing axially only and (b) in both axial and radial directions. In the latter case, the system was considered to expand radially until reaching three different final diameters. In both (a) and (b), the noise on the estimated soil resistance pressure was analyzed (see Appendix A)

Full size image

Once the radial root expansion is completed and the axial growth process resumes, our results show that, after a certain tip displacement (Δz), the tip pressure recovers the mean value reached at the steady state (around 24 MPa) when only axial growth is performed (i.e., Analysis I). Moreover, we observe that larger Dthickened allow the tip to move in a reduced pressure regime for longer displacements (i.e., \({\Delta z}_{\mathrm{Dthickened }= 2.5\mathrm{Droot }}>{\Delta z}_{\mathrm{Dthickened }= 2\mathrm{Droot }}\gg {\Delta z}_{\mathrm{Dthickened }= 1.5{\text{Droot}}}\)) (Fig. 5b). When doubling the root diameter (i.e., blue curve in Fig. 5b), the pressure drops down importantly (i.e., close to 0 Pa) compared to the case where Dthickened = 1.5 Droot (i.e., red curve), where the pressure decreases down to about 13 MPa. A further increase of Dthickened does not significantly reduce the experienced soil resistance pressure (i.e., the minimum pressure values tend to 0 Pa in both green and blue curves). A lower computational time is needed considering Dthickened = 2 Droot rather than Dthickened = 2.5 Droot. Hence, in the following analyses, a radial root growth until reaching Dthickened = 2 Droot is assumed to investigate further the penetration performance dependence on the root radial expansion process in one soil packing.

3.2 Loose granular medium

As for the dense medium, in the loose granular medium, Analysis I shows that the root tip is subjected to an axial soil resistance pressure fluctuating around a steady-state value, in this case of about \(4\) MPa (Fig. 6a).

Fig. 6
figure 6

Penetration pressure when the intruder penetrates cohesionless loose granular soil by growing (a) axially and (b) in axial and radial directions

Full size image

In Analysis II with loose granular soil, the tip resistance pressure drops when the root grows radially (in correspondence to the gray region in Fig. 6b), as also obtained in dense granular soil. However, differently from the dense granular soil case, in the loose medium, after this immediate benefit, the tip pressure increases rapidly once the radial growth is stopped to reach higher values than those obtained at the steady state in Analysis I (~4 MPa). Then, the tip pressure seems to gradually recover the mean pressure observed in Analysis I. The depth at which this recovery occurs should be confirmed by pursuing further the axial penetration. However, the simulation has to be stopped due to the limited size of the simulation domain.

We computed the local soil porosity within a cubic soil volume located just below the root tip to understand why the radial root expansion affects the system penetration performances differently based on the initial soil porosity (Fig. 7a). This cubic volume of investigation has a side equal to the root size before expanding radially (i.e., Droot = 0.02 m) and moves downward following the same translational motion as the tip. Figure 7b and Fig. 7c shows, respectively, the computed local porosity with respect to the tip displacement and the root diameter expansion for both dense and loose media. Figure 7c highlights that the dense and loose granular media result to be dilatant and contractant, respectively, when the intruder grows radially (in correspondence to the gray region): During the intruder radial expansion, the soil behavior is similar to the one shown during triaxial compression (Fig. 3). The initially dense soil dilates below the tip, weakening its shear strength (and penetration impedance) at this location. On the contrary, the densification of the initially loose soil improves its shear strength (and penetration impedance). The different behavior shown by the local soil porosity during the radial root expansion phase explains why the root thickening affects the root penetration efficiency differently in granular media with different porosities.

Fig. 7
figure 7

(a) The soil porosity was computed locally below the tip during penetration in both cohesionless dense and loose granular media. For both dense and loose granular media, the soil porosity is plotted with respect to (b) the tip displacement during the penetration process and (c) the root diameter during the radial expansion

Full size image

3.3 Cemented soil

Similarly to the analysis executed for the granular media, the intruder radial growth effect was investigated considering penetration in cemented soil. When Analysis I is executed, the intruder tip perceives an axial soil resistance pressure that fluctuates around a stabilization value of about 20 MPa (Fig. 8a). Concerning Analysis II, our results highlight that during the system radial growth (in correspondence to the gray region in the graph), the soil resistance pressure perceived from the tip drops. Then, once the radial growth process stops and the intruder starts growing axially again, the tip perceives pressures that rise sharply to stabilize around the average pressure value observed when only axial growth is considered (Analysis I) (Fig. 8b). This occurs after a lower tip translation Δz with respect to what was observed for the dense cohesionless granular medium (Fig. 5b).

Fig. 8
figure 8

Penetration pressure when the system penetrates cemented soil by growing (a) axially only and (b) in axial and radial directions

Full size image

In cemented soil, our findings show that the radial expansion facilitates penetration over a lower depth than in dense granular soil. In this context, we evaluated how the system radial expansion effect depends on the soil inter-particles contact adhesion σA (cf. Equation 5). The impact of the soil cohesion on the efficiency of the intruder penetration due to its radial expansion has been investigated by computing the tip pressure (Ptip) reduction observed during the radial growth and the additional displacement (Δz) necessary to recover the tip resistance observed in Analysis I for different inter-particle adhesions. Results are presented in Fig. 9, where the global trends are highlighted with linear regressions. Both Ptip reduction and Δz decrease to increase inter-particle contact adhesion values, showing that the intruder radial expansion becomes less advantageous with increasing inter-particle contact adhesion values. To test the hypothesis that the Ptip reduction and Δz depend on the inter-particle contact adhesion, a Wald Test is used [22]. Specifically, the Wald Test assumes as a null hypothesis that the dependence of the Ptip reduction and Δz depend on the adhesion with a constant and calculates the probability (represented by the P value) of this assumption being true: If P value < 0.05, the null hypothesis is rejected [22]. Our results show that the alternative hypothesis (i.e., Ptip reduction and Δz do not depend on the adhesion with a constant) is true with a significance level of 0.05.

Fig. 9
figure 9

For penetration in cemented soil, the dependence of the radial expansion effect on the inter-particle adhesion in terms of (a) tip pressure reduction during the actuation of radial expansion and (b) tip displacement needed to reach the stabilization pressure value observed during axial growth once the radial growth is completed

Full size image

3.4 Comparative analysis of the radial growth effect

Finally, we analyzed the dependence between the gain introduced by the radial growth and the Dthickened value with changing soil conditions.

Specifically, we analyzed the dependence of Δz on Dthickened by applying a Wald Test similar to the procedure presented in Sect. 3.3 [22]. In this case, the Wald test assumes as a null hypothesis that a constant defines the dependence of the Ptip reduction and Δz on Dthickened and computes the probability (P-value) of this assumption being true: If P value < 0.05, the null hypothesis is rejected [22]. As reported in Fig. 10a, our results show that the alternative hypothesis (i.e., the dependence of Δz on Dthickened is not a constant) is confirmed with a significance level of 0.05 for the cases where the intruder penetrates dense granular and cemented soils. By contrast, the null hypothesis for cohesionless loose granular soil cannot be rejected, implying that the tip displacement does not significantly depend on Dthickened. Furthermore, a linear regression was performed to quantify the analyzed dependence. We found that when a dense granular soil is penetrated, the radial expansion effect on Δz is stronger than in the cemented soil case, consistent with the results shown in Fig. 9b. As shown in Fig. 10a, the linear model suggests that (1) as Dthickened tends to zero, Δz is close to zero, and (2) as Dthickened grows, Δz grows.

Fig. 10
figure 10

For penetration in granular (both dense and loose) and cemented media, the dependence of the radial expansion effect in terms of (a) tip displacement needed to reach the stabilization pressure value observed during axial growth once the intruder stops growing radially, and (b) tip pressure reduction during the radial expansion process

Full size image

Furthermore, we analyzed the dependence of Ptip reduction on Dthicknened (Fig. 10b) with the model proposed in Eq. (8). By plotting simulation results, we can observe that with increasing Dthickened-to-Droot ratio in all three soil types, the Ptip reduction tends to reach Ptip before thickening. We thus defined \({P}_{red}^{max}\) as the maximum normalized \({P}_{tip}reduction\) (i.e., the maximum value of \({P}_{tip}reduction/{P}_{tip}before thickening\)) and assumed the reduction cannot exceed pressure before thickening, so that \({P}_{red}^{max}=1\).

$$\frac{{P_{tip} \;reduction}}{{P_{tip} \;before\; thickening}} = P_{red}^{max} - e^{{k\left( {1 - \frac{Dthickened}{{Droot}}} \right)}}$$
(8)

\(k\) is the rate of \({P}_{tip}reduction\) in terms of normalized Dthickened. By fitting the numerical simulation data with Eq. (8) for the three different soil conditions, we get \(k\) = 1.7 for cohesionless dense granular soil, \(k\) = 5 for cohesionless loose granular soil, and \(k\) = 1.2 for cemented soil (Fig. 10b).

This result highlights how the benefit of radial expansion raises faster in loose cohesionless granular soil, but that it is also important in cohesionless dense and cemented soils, being capable of cutting to half the pressure with 1.5Droot radial enlargement. From Fig. 10b and the proposed model, we can also deduce the existence of a limiting threshold of Dthickened, different for the three soil cases, above which the benefit stabilizes.

Additionally, for each soil case, the total energy required to penetrate by growing axially only was compared to the one to penetrate by growing both axially and radially (Fig. 11). Specifically, the total energy was computed approximating the integrals reported in Eq. (9) using the trapz function in Numpy [18].

$$E = \int\limits_{{z_{{start}} }}^{{z_{{end}} }} {F_{{axial}} dz} + \int\limits_{{D_{{root}} }}^{{D_{{thickened}} }} {F_{{radial}} dD}$$
(9)

where \({F}_{axial}\) is the axial force magnitude perceived by the tip during penetration integrated along the axial tip displacement \(dz\), \({z}_{start}-{z}_{end}\) is the range of depth affected by radial expansion (i.e., radial growth phase + Δz), whereas \({F}_{radial}\) represents the radial force magnitude and is computed as \({F}_{radial}=\sum_{i=1}^{N}\Vert {{\varvec{F}}}_{C(i)}\Vert\) where \({{\varvec{F}}}_{C(i)}\) is the radial contact force of an interaction i between the radially expanding region of the intruder and soil particles, and N is the number of interactions. In Fig. 11, the estimated energy \(E\) is normalized with respect to \(\upzeta ={(z}_{start}-{z}_{end})\).

Fig. 11
figure 11

Energy required to penetrate by a system growing axially only and a system growing both axially and radially depending on the type of soil (both dense and loose granular media and cemented soil)

Full size image

Considering the energy required by a system penetrating using axial growth only, the energy requirement of a system growing both axially and radially results to (a) drop-down by about 24% in cohesionless dense granular medium, (b) increase by about 38% in cohesionless loose granular soil, and (c) increase by about 12% for a cemented medium (Fig. 11).

4 Discussion and conclusions

This paper presents a 3D DEM numerical model developed to simulate the penetration process of a plant root-inspired soil intruder and evaluate its penetration performances with different penetration strategies and soil types. Specifically, we considered strategies adopted by plant roots to move and explore the soil to analyze root behavior and select the most convenient for implementation in artificial autonomous soil exploratory systems. Roots enter the soil by cell division and elongation at their apical extremities, named apical growth. In addition, they display root morphing, adjusting their diameter according to soil characteristics. Root thickening is investigated in this paper and hypothesized to facilitate penetration by generating voids under the tip. To verify this hypothesis, the model implements an intruder that penetrates soil by growing at the tip level and can radially expand. Penetration performances are analyzed in terms of pressures experienced by the system over different soil conditions, i.e., cohesionless granular and cemented media. Both dense and loose cohesionless granular soils are included in the analysis.

Our results highlight that, compared to a system that grows axially only, an intruder penetrating soil by growing in both axial and radial directions performs differently based on the explored soil.

When penetration occurs into cohesionless dense granular soil, we found that implementing a system growing both axially and radially is advantageous in terms of penetration performances and energy requirements. Indeed, when the intruder radial expansion process is activated, the soil resistance pressure perceived by the tip decreases significantly. Once this process is completed and the intruder axial growth process resumes, the tip keeps perceiving, over a finite penetration distance, resistance pressures that are lower than those perceived when the radial growth is not implemented. Furthermore, a system growing axially and radially requires about 24% lower energy than one that grows axially only. Therefore, it is extremely beneficial to implement an intruder that grows both axially and radially for penetrating in cohesionless dense granular soil. The resulting advantages of implementing radial expansion are in accordance with previous investigations [10]–[25] where, despite divergencies with the current study in the region that radially expands and its motion relative to the probe, the radial expansion results in a soil resistance decrease, facilitating penetration in granular cohesionless dense soil. Differently from these previous investigations, this paper further explores the effects of radial expansion on penetration performances by considering cohesionless loose granular medium and cemented soil, thus informing on the conditions where this penetration strategy can be more convenient to implement.

In particular, considering an intruder embedding radial expansion results counterproductive for penetrating in cohesionless loose granular soil since it implies higher soil resistance perceived by the intruder tip during penetration and higher energy requirements compared to a system growing axially only. Specifically, the resistance pressure perceived by the tip drops advantageously during the radial thickening if unlimited growth can occur. However, once the radial expansion stops and the intruder starts growing axially again, the benefit of the radial expansion rapidly erases, and the tip is subjected to higher resistance pressures than those observed in the absence of radial expansion. We analyzed the soil porosity locally below the tip during the radial growth process to investigate the reason for the divergent results achieved in dense and loose cohesionless granular soils. The different soil responses to the radial expansion resulted from soil decompaction (porosity increase)/compaction (porosity decrease) in dense/loose medium, respectively. Additionally, the energy requirements for penetrating cohesionless loose granular soil by apical axial and radial growths are about 38% higher than for a system with only axial growth.

Finally, we evaluated the effect of radial expansion in the case of cemented soil exploration. Although in this soil, an intruder implementing radial expansion requires more energy (about 12%) than a system growing axially only, radial growth is advantageous since it implies a reduction of the soil resistance perceived by the intruder tip during penetration. Specifically, the radial growth is beneficial over a lower penetration depth with respect to the dense cohesionless granular soil case, but it is not disadvantageous as in the loose granular soil case, where higher soil pressures on the tip are found after radial growth. For penetration in cemented soil, we also evaluated how the radial expansion effect changes with different inter-particle adhesion values, finding that radial expansion advantages drop with increasing inter-particle contact adhesion values.

Biological systems can suggest efficient strategies of movements over various domains. However, the role and effects of different features in improving operational performances are difficult to quantify in natural systems. The proposed DEM model represents a tool to analyze soil intruder behaviors and correlated performances over various soil conditions and intruder features. It can support selecting advantageous features to be implemented in robotic artifacts for autonomous soil exploration, thus providing important guidelines for designing performant penetrating robotic systems. As future perspectives, the proposed model can be used to analyze penetration in soils with different mechanical properties (e.g., other terrestrial and extraterrestrial soils) and can be extended to analyze the performances when other bioinspired penetration strategies are implemented, e.g., circumnutation movements of plant roots [16]. Other tip geometries can also be analyzed to establish the most convenient to facilitate movements in particularly harsh conditions (e.g., Martian or Lunar-like soils). All these analyses may provide additional hints for the optimal design and manufacturing requirements of performant penetrating robotic systems. Furthermore, soil microstructural quantities (e.g., contact force network and particle displacement) may be analyzed to advance our understanding of soil behavior during intruder penetration. As for a pressuremeter test, we expect the soil to shear around a region of the intruder that expands radially and just below the tip. In soil, shear induces a volumetric deformation and, in particular, for dense soil, dilation. This shear-induced dilation may reduce tip pressure when the intruder radially expands while penetrating dense granular soil. Maps of strain and stress fields may better detail these mechanisms.