Coexistence of competitive species with a stage-structured life cycle

Abstract

Ecological theory provides explanations for exclusion or coexistence of competing species. Most theoretical works on competition dynamics that have shaped current perspectives on coexistence assume a simple life cycle. This simplification, however, may omit important realities. We present a simple two-stage structured competition model to investigate the effects of life-history characteristics on coexistence. The achievement and the stability of coexistence depend not only on competition coefficients but also on a set of life-history parameters that reflect the viability of an individual, namely, adult death rate, maturation rate, and birth rate. High individual viability is necessary for a species to persist, but it does not necessarily facilitate coexistence. Intense competition at the juvenile or adult stage may require higher or lower viability, respectively, for stable coexistence to be possible. The stability mechanism can be explained by the refuge effect of the less competitive stage, and the birth performance, which preserves the less competitive stage as a refuge. Coexistence might readily collapse if the life-history characteristics, which together constitute individual viability, change, even though two species have an inherent competitive relation conducive to stable coexistence.

Appendices

Appendix 1

The nontrivial equilibrium values of Eqs. 6a–d are

$$ X^* = \frac{{\left( {\gamma /c^2 } \right)\left\{ {b_0 \gamma - c\left( {\gamma + \phi _0 } \right)} \right\}\left\{ {b_0 \gamma ^2 \left( { - 1 + \alpha } \right) - c^2 \left( {1 - {\alpha '}} \right)} \right\}}} {{\nu ^2 \left( { - 1 + \alpha \beta } \right) + \nu \left( { - 2 + \alpha {\beta '} + {\alpha '}\beta } \right) + c^2 \left( { - 1 + {\alpha '}{\beta '}} \right)}} $$

(20)

$$ x^* = \frac{{(1/c)\left\{ {b_0 \gamma - c\left( {\gamma + \phi _0 } \right)} \right\}\left\{ {b_0 \gamma ^2 \left( { - 1 + \alpha } \right) - c^2 \left( {1 - {\alpha '}} \right)} \right\}}} {{v^2 \left( { - 1 + \alpha \beta } \right) + v\left( { - 2 + \alpha {\beta '} + {\alpha '}\beta } \right) + c^2 \left( { - 1 + {\alpha '}{\beta '}} \right)}} $$

(21)

$$ Y^* = \frac{{(\gamma /c^2 )\left\{ {b_0 \gamma - c\left( {\gamma + \phi _0 } \right)} \right\}\left\{ {b_0 \gamma ^2 \left( { - 1 + \beta } \right) - c^2 \left( {1 - {\beta '}} \right)} \right\}}} {{v^2 \left( { - 1 + \alpha \beta } \right) + v\left( { - 2 + \alpha {\beta '} + {\alpha '}\beta } \right) + c^2 \left( { - 1 + {\alpha '}{\beta '}} \right)}} $$

(23)

and

$$ y^* = \frac{{(1/c)\left\{ {b_0 \gamma - c\left( {\gamma + \phi _0 } \right)} \right\}\left\{ {b_0 \gamma ^2 \left( { - 1 + \beta } \right) - c^2 \left( {1 - {\beta '}} \right)} \right\}}} {{v^2 \left( { - 1 + \alpha \beta } \right) + v\left( { - 2 + \alpha {\beta '} + {\alpha '}\beta } \right) + c^2 \left( { - 1 + {\alpha '}{\beta '}} \right)}}, $$

(24)

where v=b₀γ²/c².

Appendix 2

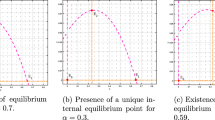
Biological feasibility of the equilibrium population densities is satisfied when the fractions C₁/B and C₂/B in Eqs. 7a and b are positive, given that the persistence condition in Ineq. 9 holds. The necessary condition for the equilibrium to be positive is either of the following:

Case 1:

$$ v\left( { - 1 + \alpha } \right) > 1 - {\alpha '} $$

(25a)

$$ v\left( { - 1 + \beta } \right) > 1 - {\beta '} $$

(25b)

$$ \left( { - 1 + \alpha \beta } \right)v^2 + \left( { - 2 + \alpha {\beta '} + {\alpha '}\beta } \right)v + \left( { - 1 + {\alpha '}{\beta '}} \right) > 0, $$

(25c)

Case 2:

$$ v\left( { - 1 + \alpha } \right) < 1 - {\alpha '} $$

(26a)

$$ v\left( { - 1 + \beta } \right) < 1 - {\beta '} $$

(26b)

$$ \left( { - 1 + \alpha \beta } \right)v^2 + \left( { - 2 + \alpha {\beta '} + {\alpha '}\beta } \right)v + \left( { - 1 + {\alpha '}{\beta '}} \right) < 0. $$

(26c)

We verified that if Ineqs. 25a and 25b are satisfied, Ineq. 25c is necessarily satisfied and if Ineqs. 26a and 26b are satisfied, Ineq. 26c is necessarily satisfied. Therefore, the necessary condition for the equilibrium to be positive is reduced to: Ineqs. 25a and 25b in case 1, and Ineqs. 26a and 26b in case 2.

Appendix 3

We chose a set of values of parameters, α, β, α′, β′, v, and φ₀, and judged whether the set of parameters is classified into either of the conditions of positive interior equilibrium, i.e., case 1 or 2 in Appendix 2. If the parameters set satisfied case 1 or 2, we designed b₀, γ, and c for the given value of v. Then, we get a set of values of parameters, α, β, α′, β′, φ₀, b₀, γ, and c. For the set of parameters and the equilibrium values (Appendix 1), the dominant eigenvalue associated with the 4×4 Jacobian matrix

$$ \begin{aligned} J = & \left( {\begin{array}{*{20}l} {{ - c} \hfill} & {\gamma \hfill} \\ {{ - b_{0} X^{*} + b_{0} {\left( {1 - X^{*} - \alpha Y^{*} } \right)}} \hfill} & {{ - x^{*} - \gamma - \phi _{0} - x^{*} - {\alpha }\ifmmode{'}\else$'$\fi y^{*} } \hfill} \\ {0 \hfill} & {0 \hfill} \\ {{ - b_{0} \beta Y^{*} } \hfill} & {{ - {\beta }\ifmmode{'}\else$'$\fi y^{*} } \hfill} \\ \end{array} } \right. \\ & \quad \left. {\begin{array}{*{20}l} {0 \hfill} & {0 \hfill} \\ {{ - b_{0} \alpha X^{*} } \hfill} & {{ - {\alpha }\ifmmode{'}\else$'$\fi x^{*} } \hfill} \\ {{ - c} \hfill} & {\gamma \hfill} \\ {{ - b_{0} Y^{*} + b_{0} {\left( {1 - Y^{*} - \beta X^{*} } \right)}} \hfill} & {{ - y^{*} - \gamma - \phi _{0} - y^{*} - {\beta }\ifmmode{'}\else$'$\fi x^{*} } \hfill} \\ \end{array} } \right), \\ \\ \end{aligned} $$

which is acquired by the linearized system of Eqs. 6a–d, is evaluated at the equilibrium point. We repeated this procedure systematically for various combinations of the parameters, and inductively confirmed that the equilibrium is stable for the sets of parameters belonging to case 1.

Appendix 4

The partial analysis of the local stability of the equilibrium is constrained, x=(c/γ)X and y=(c/γ)Y, by the direction of perturbations of each species being restricted on each line. With this constraint, we can describe the dynamic equations of both species only by the adult populations, denoted as F _X (X,Y) and F _Y (X,Y), respectively. The Jacobean matrix of the linearized system of F _X and F _Y in the state of a nontrivial equilibrium was obtained, and then we found the eigenvalues, −A and C₁C₂A²/B (these variables are as defined in Eqs. 7a and b). Because we consider that A is positive (thus, −A<0), stability is determined only by the sign of the latter eigenvalue. Therefore, the stability condition is reduced to

$$ \frac{{C_1 C_2 }} {B} < 0. $$

(27a)

Now, since we consider that equilibrium is positive (that is, Eqs. 7a, b are both positive), C₁ and C₂ have same sign. Therefore, B must be negative for stability, and, consequently, C₁ and C₂ are both negative. Thus, the stability conditions are again reduced to

$$ C_{1} < 0,\,C_{2} < 0. $$

(27b)

Appendix 5

When the interspecific competition is intense at the adult stage and weak at the juvenile stage, the condition of stable coexistence is

$$ \frac{{\gamma \left( {\gamma + \phi _0 } \right)}} {c} < \frac{{b_0 \gamma ^2 }} {{c^2 }} < v_{\sup } . $$

(28a)

Rearrangement of the inequality in terms of b₀ leads to the following:

$$ \frac{{c\left( {\gamma + \phi _0 } \right)}} {\gamma } < b_0 < \left( {\frac{c} {\gamma }} \right)^2 v_{\sup } . $$

(28b)

Then, the allowable maximum maturation rate while keeping a positive interval of b₀ in Ineq. 28b is obtained by solving the equality,

$$ \frac{{c\left( {\gamma + \phi _0 } \right)}} {\gamma } = \frac{{c^2 }} {{\gamma ^2 }}v_{\sup } . $$

(28c)

Considering γ>0, the allowable maximum maturation rate is

$$ \gamma _{\sup } = \frac{1} {2}\left( { - \phi _0 + \sqrt {4cv_{\sup } + \phi _0 ^2 } } \right). $$

(28d)