Abstract
The singularities of an irrotational magnetic field are lines of electric current. This property derives from the relationship between vector fields and the topology of the underlying three-space and allows for a definition of cosmic field flux tubes and flux ropes as cores (in the sense of the physics of defects) of helical singularities. When applied to force-free flux ropes, and assuming current conservation, an interesting feature is the quantization of the radii, pitches, and helicities. One expects similar quantization effects in the general case. In the special case when the total electric current vanishes, a force-free rope embedded in a medium devoid of magnetic field is nonetheless topologically stable, because it is the core of a singularity of the vector potential. Magnetic merging is also briefly discussed in the same framework.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Notes
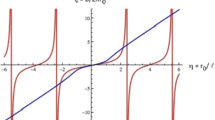
One gets, starting from Equation (26), that \({\partial {\mathcal{E}}} / {\partial{r_{0}}} = (B_{0}^{2}/4) r_{0} (1-\zeta^{2}+ \zeta(\zeta+\zeta^{-1})/D )= 0\), where D=1−(r 0/ℓ)(ζ+ζ −1). Using Equation (27), one gets that, at a critical point r 0 of \({\mathcal{E}}\),
$$\frac{\partial^2{\mathcal{E}}}{\partial{r_0}^2} =- \frac{B_0^2}{4} \frac {2}{D \ell} \zeta^2 \bigl(\zeta+\zeta^{-1}\bigr). $$Using Equation (27), it is easy to show that b D<0, regardless of the sign of b. Therefore \({\partial^{2}{\mathcal{E}}}/{\partial {r_{0}}^{2} }>0\) at all critical points.
The vector potential being continuous amounts to saying that there are no δ-function singularities in the B-field. Consider, e.g., the discontinuous vector potential
$${\mathbf{A}}(r,\theta,z) = 0, \quad r < r_0, \qquad {\mathbf{A}}(r,\theta,z)= C \boldsymbol {\nabla }\theta, \quad r > r_0; $$then \({\mathbf{B}}= {\nabla \times }{\mathbf{A}}=C/(2\pi r_{0}) \delta(r- r_{0}) \hat{\mathbf{z}} \). That is, one gets a δ-function B-field on the cylinder r=r 0.
Remember that a magnetic vortex line in a type II superconductor is also the singularity of an irrotational vector potential; the magnetic field is expelled from the specimen, except in the core region, which carries a quantized magnetic flux (de Gennes 1989).
References
Abramowicz, M., Stegun, I.A. (eds.): 1970, Handbook of Mathematical Functions, Dover, New York.
Alfvén, H., Fälthammar, C.-G.: 1963, Cosmical Electrodynamics, Oxford University Press, Oxford.
Aschwanden, M.J.: 2006, Physics of the Solar Corona, Springer, Berlin.
Aschwanden, M.J., Nightingale, R.W., Alexander, D.: 2000, Evidence for non-uniform heating of coronal loops inferred from multithread modeling of TRACE data. Astrophys. J. 541, 1059 – 1077.
Bellan, P.M.: 2003, Why helicity injection causes coronal flux tubes to develop an axially invariant cross-section. Adv. Space Res. 32, 1923 – 1929.
Berger, M.A., Field, G.B.: 1984, The topological properties of magnetic helicity. J. Fluid Mech. 147, 133 – 148.
Birkeland, R.: 1908, The Norwegian Aurora Polaris Expedition – 1902 – 1903, Part I, H. Aschehoug and Co., Oslo.
Cantarella, J., DeTurck, D., Gluck, H.: 2002, Vector calculus and the topology of domains in 3-space. Am. Math. Mon. 109, 409 – 442.
Cattaneo, F., Emonet, T., Weiss, N.: 2003, On the interaction between convection and magnetic fields. Astrophys. J. 588, 1183 – 1198.
Dahlgren, H., Carlqvist, P., Gahm, G.: 2007, Filamentary structures in planetary nebulae. Astrophys. Space Sci. 310, 65 – 72.
de Gennes, P.-G.: 1989, Superconductivity of Metals and Alloys, Addison-Wesley, Reading.
Fabian, A.R., Johnstone, J., Sanders, J., Conselice, C.J., Crawford, C.S., Gallagher, J.S. III, Zweibel, E.: 2008, Magnetic support of the optical emission line filaments in NGC 1275. Nature 454, 968 – 970.
Friedel, J.: 1964, Dislocations, Pergamon, Oxford.
Griffiths, D.J.: 1999, Introduction to Electrodynamics, 3rd edn., Prentice Hall, New York.
Kleman, M.: 1980, Dislocations, disclinations, and magnetism. In: Nabarro, F.R.N. (ed.) Dislocations in Solids 5, North Holland, Amsterdam, 349 – 402.
Kleman, M., Friedel, J.: 2008, Disclinations, dislocations and continuous defects: a reappraisal. Rev. Mod. Phys. 80, 61 – 115.
Kleman, M., Lavrentovich, O.D., Nastishin, Y.A.: 2004, Dislocations and disclinations in mesomorphic phases. In: Nabarro, F.R.N., Hirth, J.P. (eds.) Dislocations in Solids 12, Elsevier, Amsterdam, 147 – 271.
Linton, M.G.: 2006, Reconnection of nonidentical flux tubes. J. Geophys. Res., Space Phys. 111. doi: 10.1029/2006JA011891 .
Longcope, D.W.: 2005, Topological methods for the analysis of solar magnetic fields. Living Rev. Solar Phys. 2. doi: 10.12942/lrsp-2005-7 .
Mahajan, S.M., Yoshida, Z.: 1998, Double curl Beltrami flow: diamagnetic structures. Phys. Rev. Lett. 81, 4863 – 4866.
Parker, E.N.: 1979, Cosmical Magnetic Fields, Clarendon Press, Oxford.
Parker, E.N.: 1994, Spontaneous Current Sheets in Magnetic Fields, Oxford University Press, Oxford.
Parker, E.N.: 2007, Conversation on Electric and Magnetic Fields in the Cosmos, Princeton University Press, Oxford.
Parker, E.N.: 2009, Solar magnetism: the state of our knowledge and ignorance. Space Sci. Rev. 144, 15 – 24.
Sakurai, T.: 1979, A new approach to the force-free field and its application to the magnetic field of solar active regions. Publ. Astron. Soc. Japan 31, 209 – 230.
Schrijver, C.J., Siscoe, G.L. (eds.): 2011, Heliophysics: Plasma Physics and the Local Cosmos, Cambridge University Press, Cambridge.
Suzuki, T., Ito, A., Yoshida, Z.: 2003, Statistical model of current filaments in a turbulent plasma. Fluid Dyn. Res. 32, 247 – 260.
Taylor, J.B.: 1986, Relaxation and magnetic reconnection in plasmas. Rev. Mod. Phys. 58, 741 – 763.
Török, T., Chandra, R., Pariat, E., Démoulin, P., Schmieder, B., Aulanier, G., Linton, M.G., Mandrini, C.H.: 2011, Filament interaction modeled by flux rope reconnection. Astrophys. J. 728, 65 – 71.
Vishniac, E.T.: 1995, The dynamics of flux tubes in a high-β plasma. I. A general description. Astrophys. J. 446, 724 – 740.
Yoshida, Z., Mahajan, S.M.: 1999, Simultaneous Beltrami conditions in coupled vortex dynamics. J. Math. Phys. 40, 5080 – 5091.
Acknowledgements
We are grateful to Jean-Louis Le Mouël for a careful reading of the manuscript and useful remarks. JMR thanks the Institut de Physique du Globe for hospitality when this work was started. This is IPGP contribution # 3382.
Author information
Authors and Affiliations
Corresponding author
Appendix: Some Fundamentals of Defect Theory
Appendix: Some Fundamentals of Defect Theory
The key ingredient of the theory of line defects in an ordered medium [Ω] (a crystal) is the Volterra process (Friedel 1964); it describes the creation of a line singularity characterized by an isometry of the medium, namely a translational symmetry [b] and/or a rotational symmetry [ω=ω ν], ω being the angle of rotation, ν its axis.
Consider in a perfectly ordered Ω a line [L], and cut the crystal along an element of surface [Σ] bound by L (L=∂Σ is a loop or an infinite line); Σ1 and Σ2 are the two lips of Σ, the cut surface. Displace the two lips Σ1 and Σ2 by a relative rigid displacement [b] and a rotation [ω], viz. by a total displacement (we assume ω small):
b is the Burgers’ vector of the line defect, ω its rotation vector (Kleman and Friedel 2008). Introduce now a perfect wedge of matter in the void left by the displacement, in order to fill it with exact contacts with the lips (which is possible since d(r) is an isometry) – or remove matter in the regions of double covering. Then reestablish the bonds at the lips [Σ1 and Σ2] and let the medium relax elastically. The line defect thus obtained is called a dislocation if ω=0, a disclination if b=0. Figure 6 shows dislocations in a smectic phase, in which there is only one finite repeat distance [d] perpendicular to the layers (here |b|=2d). A screw dislocation is when b is along the line L (here along the axis of the helically transformed layers); an edge dislocation when b is orthogonal to L (here b is in the plane of the drawing, L perpendicular to it, |b|=d).
Elementary dislocations (displacement d(r) reduced to a pure translation) in a smectic phase: (a) screw dislocation, b is in the direction of L; (b) edge dislocation, b is perpendicular to L. In both cases L is an infinite straight line.
Such a process has of course no meaning along L itself, and one assumes henceforth that a toric region has been removed along this line. This “core” is a region where the above process is not valid. The Volterra process undoubtedly introduces internal stresses in an elastic medium, but these stresses are non-singular on Σ1 and Σ2 insofar as d(r) obeys Hookean elasticity. Henceforth, the final result does not depend on the exact choice of Σ.
Vortex Lines:
This term refers to a line defect [L] whose characteristic invariant is not an element of symmetry of the medium as above but the circulation γ=∮ C u⋅ds of a vector u. In an irrotational fluid this would be the flow velocity; in a superconductor the vector potential. Notice that the vector u itself has the geometry of a disclination, since it rotates by 2π about the line [L]. One will have recognized that the singularities investigated in the present work are of the vortex line type, but display a geometry locally reminiscent of a screw dislocation in a smectic.
Rights and permissions
About this article
Cite this article
Kleman, M., Robbins, J.M. Tubes of Magnetic Flux and Electric Current in Space Physics. Sol Phys 289, 1173–1192 (2014). https://doi.org/10.1007/s11207-013-0399-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11207-013-0399-0