Invariance of bipartite separability and PPT-probabilities over Casimir invariants of reduced states

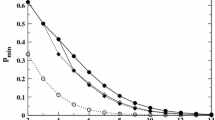
Abstract

Milz and Strunz (J Phys A 48:035306, 2015) recently studied the probabilities that two-qubit and qubit–qutrit states, randomly generated with respect to Hilbert–Schmidt (Euclidean/flat) measure, are separable. They concluded that in both cases, the separability probabilities (apparently exactly $\frac{8}{33}$ in the two-qubit scenario) hold constant over the Bloch radii (r) of the single-qubit subsystems, jumping to 1 at the pure state boundaries ($r=1$). Here, firstly, we present evidence that in the qubit–qutrit case, the separability probability is uniformly distributed, as well, over the generalized Bloch radius (R) of the qutrit subsystem. While the qubit (standard) Bloch vector is positioned in three-dimensional space, the qutrit generalized Bloch vector lives in eight-dimensional space. The radii variables r and R themselves are the lengths/norms (being square roots of quadratic Casimir invariants) of these (“coherence”) vectors. Additionally, we find that not only are the qubit–qutrit separability probabilities invariant over the quadratic Casimir invariant of the qutrit subsystem, but apparently also over the cubic one—and similarly the case, more generally, with the use of random induced measure. We also investigate two-qutrit ($3 \times 3$) and qubit–qudit ($2 \times 4$) systems—with seemingly analogous positive partial transpose-probability invariances holding over what has been termed by Altafini the partial Casimir invariants of these systems.