The singlet–triplet transition of two interacting electrons in a Frost–Musulin quantum dot

Abstract

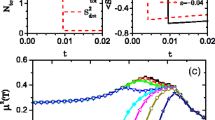
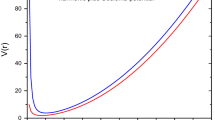
In the paper, the electronic properties of a quantum dot (QD) using the Frost–Musulin potential model are investigated taking into account both an external magnetic field and electron–electron interaction. For this purpose, the Schrödinger equation (SE) is analytically solved without considering electron–electron interaction by employing the Nikiforov–Uvarov (NU) procedure, and the energy levels and wave functions are determined. Then, the singlet–triplet (ST) transition is studied for different values of magnetic fields. According to our results, Both the dot size and magnetic field have key roles in the ground state transition. The ST transition of the ground state moves to lower magnetic fields as the QD size is increased. However the transition occurs at higher magnetic fields when the potential depth is increased. The transition for small QD size occurs only from ¹S to ³P. But by increasing the QD size, another transition is also observed from ¹D to ³F. These transitions occur at smaller magnetic fields when the QD size is increased.

Appendix 1

In this part, we present a detail of the solution of the Schrödinger equation (SE) for one particle with FM potential exposed to magnetic field. In 2D, the SE can be written as

$$\left\{\frac{1}{2{m}_{e}}{\left({\varvec{P}}-e{\varvec{A}}\right)}^{2}+\left[{D}_{0}-{D}_{0}\left(1+\alpha {r}_{0}\right){e}^{-\alpha x}+{D}_{0}\frac{\alpha {r}_{0}^{2}}{r}{e}^{-\alpha x}\right]\right\}{\varphi }_{nl}\left(r,\theta \right)={E}_{nl}{\varphi }_{nl}\left(r,\theta \right)$$

(8)

where ${E}_{nl}$, and ${m}_{e}$ are the system’s energy and the effective mass. The vector potential in the cylindrical coordinates is chosen as

$${\varvec{A}}=\left(0,\frac{B{e}^{-\alpha r}}{1-{e}^{-\alpha r}},0\right)$$

(9)

The wave function in the cylindrical coordinates is written as

$${\varphi }_{nl}\left(r,\theta \right)=\frac{1}{2\pi r}{e}^{il\theta }{f}_{nl}\left(r\right)$$

(10)

Inserting Eqs. (8) and (10) in Eq. (8), we obtain

$$\frac{{d}^{2}{f}_{nl}(r)}{d{r}^{2}}+\left\{{E}_{nl}+{D}_{0}\alpha {r}_{0}{e}^{-\alpha x}+\frac{{D}_{0}{\alpha }^{2}{r}_{0}^{2}{e}^{-\alpha x}}{1-{e}^{-\alpha x}}-\frac{\hslash {\omega }_{c}l\alpha {e}^{-\alpha x}}{{\left(1-{e}^{-\alpha x}\right)}^{2}}-\frac{{m}_{e}{\omega }_{c}^{2}{e}^{-2\alpha x}}{2{\left(1-{e}^{-\alpha x}\right)}^{2}}-\frac{{\hslash }^{2}}{2{m}_{e}}\left(\frac{{l}^{2}-\frac{1}{4}}{{r}^{2}}\right)\right\}{f}_{nl}\left(r\right)=0$$

(11)

where ${\omega }_{c}=eB/{m}_{e}$. To solve Eq. (11), we use the Greene-Aldrich approximation (Greene and Aldrich 1976). The approximation is written as

$$\frac{1}{{r}^{2}}\approx \frac{{\alpha }^{2}}{{\left(1-{e}^{-\alpha x}\right)}^{2}}$$

(12)

Now, we introduce a new parameter as $t={e}^{-\alpha x}$. Then, Eq. (11) can be written as

$$\frac{{d}^{2}{f}_{nl}}{d{t}^{2}}+\frac{(1-t)}{t(1-t)}\frac{d{f}_{nl}}{dt}+\frac{1}{{t}^{2}{\left(1-t\right)}^{2}}\left[{-A}_{1}{t}^{2}+{A}_{2}t-{A}_{3}\right]{f}_{nl}=0$$

(13)

where

$${A}_{1}=-{\epsilon }_{nl}+2{c}_{0}-{c}_{1}+{c}_{3}, {A}_{2}=2{\epsilon }_{nl}+{c}_{0}-{c}_{1}-{c}_{2},$$

$${A}_{3}={\epsilon }_{nl}+{\rho }_{nl}$$

(14)

In Eq. (14), the used parameters are as

$${\epsilon }_{nl}=-\frac{2{m}_{e}{E}_{nl}}{{\hslash }^{2}{\alpha }^{2}}, {c}_{0}=\frac{2{m}_{e}{D}_{0}{r}_{0}}{{\hslash }^{2}\alpha }, {c}_{1}=\frac{2{m}_{e}{r}_{0}^{2}}{{\hslash }^{2}},$$

$${c}_{2}=\frac{2{m}_{e}{\omega }_{c}}{\hslash \alpha }, {c}_{3}=\frac{{m}_{e}^{2}{\omega }_{c}^{2}}{{\hslash }^{2}{\alpha }^{2}}, {\rho }_{nl}={l}^{2}-\frac{1}{4}$$

(15)

Now, we use the Nikiforov–Uvarov (NU) procedure to obtain the energy levels and wave functions. A brief illustration of the NU method is explained here that has been proposed by Nikiforov and Uvarov (Nikiforov and Uvarov 1988). Using the following coordinate transformation, $t=t(x)$, this equation is given by

$$\frac{{d}^{2}\psi (t)}{d{t}^{2}}+\frac{\overline{\omega }(t)}{\vartheta (t)}\frac{d\psi (t)}{dt}+\frac{\widetilde{\vartheta }\left(t\right)}{{\vartheta }^{2}\left(t\right)}\psi \left(t\right)=0$$

(16)

Here $\overline{\omega }(t)$, $\vartheta (t)$, and $\widetilde{\vartheta }\left(t\right)$ are polynomials. Then, the parametric NU equation is written as (Okon et al. 2017)

$$\frac{{d}^{2}\psi (t)}{d{t}^{2}}+\frac{{(a}_{1}-{a}_{2}t}{t(1-{a}_{3}t)}\frac{)d\psi (t)}{dt}+\frac{1}{{t}^{2}{(1-{a}_{3}t)}^{2}}\left[-{\xi }_{1}{t}^{2}+{\xi }_{2}t-{\xi }_{3}\right]\psi \left(t\right)=0$$

(17)

The used constants in Eq. (17) are as below

$${a}_{1}={a}_{2}={a}_{3}=1, {a}_{4}=\frac{1}{2}\left(1-{a}_{1}\right), {a}_{5}=\frac{1}{2}\left({a}_{2}-2{a}_{3}\right), {a}_{6}={a}_{5}^{2}+{\xi }_{1}$$

(18)

$${a}_{7}=2{a}_{4}{a}_{5}-{\xi }_{2}, {a}_{8}={a}_{4}^{2}+{\xi }_{3}, {a}_{9}={a}_{3}{a}_{7}+{a}_{3}^{2}{a}_{8}+{a}_{6}$$

(19)

The energy equation is given by

$${a}_{2}n-\left(2n+1\right){a}_{5}+\left(2n+1\right)\left(\sqrt{{a}_{9}}+{a}_{3}\sqrt{{a}_{8}}\right)+n\left(n-1\right){a}_{3}+$$

$${a}_{7}+2{a}_{3}{a}_{8}+2\sqrt{{a}_{8}{a}_{9}}=0$$

(20)

Applying the NU method, we can obtain the energy levels as

$${E}_{nl}=\frac{{\alpha }^{2}{\hslash }^{2}{\rho }_{nl}}{2{m}_{e}}-\frac{{\alpha }^{2}{\hslash }^{2}}{8{m}_{e}}{\left[\frac{{\Lambda }_{1}+{\Lambda }_{2}}{\sqrt{{\Lambda }_{1}}}\right]}^{2}$$

(21)

where

$${\Lambda }_{1}={\left[n+\frac{1}{2}+{\left(\frac{{m}_{e}^{2}{\omega }_{c}^{2}}{{\alpha }^{2}{\hslash }^{2}}+\frac{2{m}_{e}{\omega }_{c}}{\alpha \hslash }+\frac{2{m}_{e}{D}_{0}{r}_{0}}{\alpha {\hslash }^{2}}+{l}^{2}\right)}^{1/2}\right]}^{2}$$

(22)

$${\Lambda }_{2}=-\frac{{m}_{e}^{2}{\omega }_{c}^{2}}{{\alpha }^{2}{\hslash }^{2}}-\frac{4{m}_{e}{D}_{0}{r}_{0}}{\alpha {\hslash }^{2}}+\frac{2{m}_{e}{r}_{0}^{2}}{{\hslash }^{2}}+{\rho }_{nl}$$

(23)

The wave functions can be written as

$${f}_{nl}\left(t\right)={N}_{nl}{t}^{\sqrt{{\epsilon }_{nl}+{c}_{2}}}{\left(1-t\right)}^{\frac{1}{2}+\sqrt{\frac{1}{4}+{c}_{2}}}{P}_{n}^{\left(\delta ,\kappa \right)}\left(1-2t\right)$$

(24)

where ${P}_{n}^{(\alpha ,\beta )}\left(x\right)$ are the Jacobi polynomials and

$$\delta =2\sqrt{{\epsilon }_{nl}+{c}_{2}}, \kappa =\sqrt{\frac{1}{4}+{c}_{2}}$$

(25)