Skip to main content
SpringerLink
Log in

Multi-directional vibration isolation performances of a scissor-like structure with nonlinear hybrid spring stiffness

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

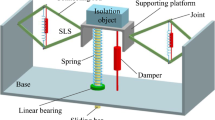
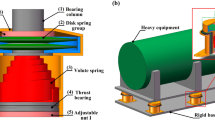
Abstract

Most existing quasi-zero stiffness (QZS) vibration isolators with excellent vibration isolation performance focus on suppressing single-directional vibration. There are limitations to applications. In this paper, a multidirectional vibration isolation system with the QZS effect is proposed based on the designed geometrical relationship between the torsion spring and the linear spring. The effect of the mechanism’s structural parameters on its static as well as dynamic characteristics is analyzed. The results reveal that (a) consisting only of elastic elements and connecting rods, the compact mechanism has ideal vibration isolation performance in all three directions; (b) the mechanism characteristics can be flexibly adjusted by tuning the structural parameters; (c) when the excitation amplitudes are large, the vibration isolator remains preferable vibration attenuation performance; (d) compared with the traditional QZS structure, the typical X-shaped QZS structure, and the proposed structure I, the designed structure has a larger QZS area and a wider vibration attenuation area in the main vibration isolation direction.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19

Similar content being viewed by others

Data availability

The datasets generated during the current study are available from the corresponding author on reasonable request.

References

  1. Chai, Y.Y., Jing, X.J., Chao, X.: X-shaped mechanism based enhanced tunable QZS property for passive vibration isolation. Int. J. Mech. Sci. 218, 107077 (2022)

    Article  Google Scholar 

  2. Li, H., Li, Y.C., Li, J.C., et al.: Negative stiffness devices for vibration isolation applications: a review. Adv. Struct. Eng. 23(8), 1739–1755 (2020)

    Article  Google Scholar 

  3. Xing, Z.Y., Yang, X.D.: A combined vibration isolation system capable of isolating large amplitude excitation. Nonlinear Dyn. 112, 2523–2544 (2024)

    Article  Google Scholar 

  4. Hao, Z., Cao, Q., Wiercigroch, M.: Nonlinear dynamics of the quasi-zero-stiffness SD oscillator based upon the local and global bifurcation analyses. Nonlinear Dyn. 87(2), 987–1014 (2017)

    Article  Google Scholar 

  5. Zhao, F., Ji, J., Ye, K., et al.: Increase of quasi-zero stiffness region using two pairs of oblique springs. Mech. Syst. Signal Process. 144, 106975 (2020)

    Article  Google Scholar 

  6. Zhao, F., Ji, J.C., Ye, K., et al.: An innovative quasi-zero stiffness isolator with three pairs of oblique springs. Int. J. Mech. Sci. 192, 106093 (2021)

    Article  Google Scholar 

  7. Yan, G., Wu, Z.Y., Wei, X.S., et al.: Nonlinear compensation method for quasi-zero stiffness vibration isolation. J. Sound Vib. 523, 116743 (2022)

    Article  Google Scholar 

  8. Zhao, F., Cao, S.Q., Luo, Q.T., et al.: Practical design of the QZS isolator with one pair of oblique bars by considering pre-compression and low-dynamic stiffness. Nonlinear Dyn. 108, 3313–3330 (2022)

    Article  Google Scholar 

  9. Papaioannou, G., Voutsinas, A., Koulocheris, D.: Optimal design of passenger vehicle seat with the use of negative stiffness elements. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 234(2–3), 610–629 (2020)

    Article  Google Scholar 

  10. Yan, G., Zou, H.X., Wang, S., et al.: Large stroke quasi-zero stiffness vibration isolator using three-link mechanism. J. Sound Vib. 478, 115344 (2020)

    Article  Google Scholar 

  11. Gatti, G., Shaw, A.D., Gonçalves, P.J.P., et al.: On the detailed design of a quasi-zero stiffness device to assist in the realisation of a translational Lanchester damper. Mech. Syst. Signal Process. 164, 108258 (2022)

    Article  Google Scholar 

  12. Zou, S., Wang, D.Y., Zhang, Y.S.: Design and testing of a parabolic cam-roller quasi-zero-stiffness vibration isolator. Int. J. Mech. Sci. 220, 107146 (2022)

    Article  Google Scholar 

  13. Ye, K., Ji, J., Brown, T.: A novel integrated quasi-zero stiffness vibration isolator for coupled translational and rotational vibrations. Mech. Syst. Signal Process. 149, 107340 (2021)

    Article  Google Scholar 

  14. Zhang, Y., Wei, G., Wen, H., et al.: Design and analysis of a vibration isolation system with cam–roller–spring–rod mechanism. J. Vib. Control 28(13/14), 1781–1791 (2022)

    Article  MathSciNet  Google Scholar 

  15. Yao, Y., Li, H., Li, Y., et al.: Analytical and experimental investigation of a high-static-low-dynamic stiffness isolator with cam-roller-spring mechanism. Int. J. Mech. Sci. 186, 105888 (2020)

    Article  Google Scholar 

  16. Xu, J.W., Yang, X.F., Li, W., et al.: Design of quasi-zero stiffness joint actuator and research on vibration isolation performance. J. Sound Vib. 479, 115367 (2020)

    Article  Google Scholar 

  17. Zheng, Y., Zhang, X., Luo, Y., et al.: Analytical study of a quasi-zero stiffness coupling using a torsion magnetic spring with negative stiffness. Mech. Syst. Signal Process. 100, 135–151 (2018)

    Article  Google Scholar 

  18. Wang, Q., Zhou, J.X., Wang, K., et al.: Design and experimental study of a compact quasi-zero-stiffness isolator using wave springs. Sci. China Technol. Sci. 64, 2255–2271 (2021)

    Article  Google Scholar 

  19. Wu, M.K., Wu, J.L., Che, J.X., et al.: Analysis and experiment of a novel compact magnetic spring with high linear negative stiffness. Mech. Syst. Signal Process. 198, 110387 (2023)

    Article  Google Scholar 

  20. Yan, B., Yu, N., Wang, Z.H., et al.: Lever-type quasi-zero stiffness vibration isolator with magnetic spring. J. Sound Vib. 527, 116865 (2022)

    Article  Google Scholar 

  21. Chen, R.Z., Li, X.P., Tian, J., et al.: On the displacement transferability of variable stiffness multi-directional low frequency vibration isolation joint. Appl. Math. Model. 112, 690–707 (2022)

    Article  MathSciNet  Google Scholar 

  22. Lian, X.Y., Deng, H.X., Han, G.H., et al.: A low-frequency micro-vibration absorber based on a designable quasi-zero stiffness beam. Aerosp. Sci. Technol. 132, 108044 (2023)

    Article  Google Scholar 

  23. Zhang, C., He, J.S., Zhou, G.Q., et al.: Compliant quasi-zero-stiffness isolator for low-frequency torsional vibration isolation. Mech. Mach. Theory 181, 105213 (2023)

    Article  Google Scholar 

  24. Dalela, S., Balaji, P.S., Jena, D.P.: Design of a metastructure for vibration isolation with quasi-zero-stiffness characteristics using bistable curved beam. Nonlinear Dyn. 108, 1931–1971 (2022)

    Article  Google Scholar 

  25. Lu, D.C., Zhou, Y., Ma, K.Q., et al.: Investigation on the free vibration characteristics of vertical quasi-zero stiffness isolation system considering the disc spring’s loading position. Soil Dyn. Earthq. Eng. 178, 108484 (2024)

    Article  Google Scholar 

  26. Chen, P., Wang, B., Zhou, D.S., et al.: Performance evaluation of a nonlinear energy sink with quasi-zero stiffness property for vertical vibration control. Eng. Struct. 282, 115801 (2023)

    Article  Google Scholar 

  27. Ye, K., Ji, J.C.: An origami inspired quasi-zero stiffness vibration isolator using a novel truss-spring based stack Miura-ori structure. Mech. Syst. Signal Process. 165, 108383 (2022)

    Article  Google Scholar 

  28. Han, H.S., Sorokin, V., Tang, L.H., et al.: Lightweight origami isolators with deployable mechanism and quasi-zero-stiffness property. Aerosp. Sci. Technol. 121, 107319 (2022)

    Article  Google Scholar 

  29. Inamoto, K., Ishida, S.: Improved feasible load range and its effect on the frequency response of origami-inspired vibration isolators with quasi-zero-stiffness characteristics. J. Vib. Acoust. 141(2), 021004 (2019)

    Article  Google Scholar 

  30. Sadeghi, S., Li, S.: Fluidic origami cellular structure with asymmetric quasi-zero stiffness for low-frequency vibration isolation. Smart Mater. Struct. 28(6), 065006 (2019)

    Article  Google Scholar 

  31. Liu, S.W., Peng, G.L., Jin, K.: Towards accurate modeling of the Tachi-Miura origami in vibration isolation platform with geometric nonlinear stiffness and damping. Appl. Math. Model. 103, 674–695 (2022)

    Article  MathSciNet  Google Scholar 

  32. Liu, S.W., Peng, G.L., Li, Z.X., et al.: Design and experimental study of an origami-inspired constant-force mechanism. Mech. Mach. Theory 179, 105117 (2023)

    Article  Google Scholar 

  33. Fulton, F., Sorokin, V.S.: Analysis of the effects of nonlinear damping on a multiple-degree-of-freedom quasi-zero-stiffness vibration isolator. Mech. Res. Commun. 130, 104121 (2023)

    Article  Google Scholar 

  34. Han, H.S., Tang, L.H., Wu, J.N., et al.: Origami-inspired isolators with quasi-zero stiffness for coupled axial-torsional vibration. Aerosp. Sci. Technol. 140, 108438 (2023)

    Article  Google Scholar 

  35. Chai, Y.Y., Jing, X.J., Guo, Y.Q.: A compact X-shaped mechanism based 3-DOF anti-vibration unit with enhanced tunable QZS property. Mech. Syst. Signal Process. 168, 108651 (2022)

    Article  Google Scholar 

  36. Chai, Y.Y., Jing, X.J.: Low-frequency multi-direction vibration isolation via a new arrangement of the X-shaped linkage mechanism. Nonlinear Dyn. 109, 2383–2421 (2022)

    Article  Google Scholar 

  37. Sun, X.T., Jing, X.J., Xu, J., et al.: Vibration isolation via a scissor-like structured platform. J. Sound Vib. 333(9), 2404–2420 (2014)

    Article  Google Scholar 

  38. Sun, X.T., Jing, X.J.: Analysis and design of a nonlinear stiffness and damping system with a scissor-like structure. Mech. Syst. Signal Process. 66–67, 723–742 (2016)

    Article  Google Scholar 

  39. Wei, C.F., Zhang, K.J., Hu, C., et al.: A tunable nonlinear vibrational energy harvesting system with scissor-like structure. Mech. Syst. Signal Process. 125, 202–214 (2019)

    Article  Google Scholar 

  40. Sun, X.T., Jing, X.J.: A nonlinear vibration isolator achieving high-static-low-dynamic stiffness and tunable anti-resonance frequency band. Mech. Syst. Signal Process. 80, 66–188 (2016)

    Article  Google Scholar 

  41. Sun, X.T., Jing, X.J.: Multi-direction vibration isolation with quasi-zero stiffness by employing geometrical nonlinearity. Mech. Syst. Signal Process. 62–63, 149–163 (2015)

    Article  Google Scholar 

  42. Zeng, R., Wen, G.L., Zhou, J.X., et al.: Limb-inspired bionic quasi-zero stiffness vibration isolator. Acta Mech. Sin. 37, 1152–1167 (2021)

    Article  MathSciNet  Google Scholar 

  43. Chen, T.F., Zheng, Y.X., Song, L.H., et al.: Design of a new quasi-zero-stiffness isolator system with nonlinear positive stiffness configuration and its novel features. Nonlinear Dyn. 111, 5141–5163 (2023)

    Article  Google Scholar 

  44. Yan, G., Qi, W.H., Lu, J.J., et al.: Bio-inspired multi-joint-collaborative vibration isolation. J. Sound Vib. 568, 118089 (2024)

    Article  Google Scholar 

  45. Tutsoy, O., Polat, A.: Linear and non-linear dynamics of the epidemics: System identification based parametric prediction models for the pandemic outbreaks. ISA Trans. 124, 90–102 (2022)

    Article  Google Scholar 

  46. Tutsoy, O., Tanrikulu, M.Y.: Priority and age specific vaccination algorithm for the pandemic diseases: a comprehensive parametric prediction model. BMC Med. Inform. Decis. Mak. 22(1), 1–12 (2022)

    Article  Google Scholar 

  47. Ermentrout, B.: Simulating, analyzing, and animating dynamical systems: a guide to XPPAUT for researchers and students. SIAM, Philadelphia (2002)

    Book  Google Scholar 

  48. Dai, H.H., Jing, X.J., Wang, Y., et al.: Post-capture vibration suppression of spacecraft via a bio-inspired isolation system. Mech. Syst. Signal Process. 105, 214–240 (2018)

    Article  Google Scholar 

Download references

Acknowledgements

This research is supported by the Natural Science Foundation of Liaoning (2020-MS-092).

Funding

The authors have not disclosed any funding.

Author information

Authors and Affiliations

  1. School of Mechanical Engineering and Automation, Northeastern University, Shenyang, China

    Jingxuan Wang & Guo Yao

Authors
  1. Jingxuan Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Guo Yao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Guo Yao.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix A

Appendix A

$$ f_{xl1} = \frac{{ - l_{1} \left( {2l_{2}^{2} \sin \varphi_{2} - \chi_{1} } \right)\sin \alpha_{1} + 2l_{2}^{3} \cos^{2} \varphi_{2} - 2l_{2}^{3} + \chi_{1} l_{2} \sin \varphi_{2} }}{{l_{1} l_{2} \left( {\chi_{1} \cos \varphi_{2} - \zeta_{1} \sin \varphi_{2} } \right)\sin \alpha_{1} }} $$
(A.1)
$$ f_{xr1} = \frac{{ - l_{1} \left( {2l_{2}^{2} \sin \varphi_{3} - \chi_{2} } \right)\sin \alpha_{2} + 2l_{2}^{3} \cos^{2} \varphi_{3} - 2l_{2}^{3} + \chi_{2} l_{2} \sin \varphi_{3} }}{{l_{1} l_{2} \left( {\chi_{2} \cos \varphi_{3} - \zeta_{2} \sin \varphi_{3} } \right)\sin \alpha_{2} }} $$
(A.2)
$$ f_{xl2} = \frac{{ - 2l_{2}^{2} \sin^{2} \varphi_{2} + \chi_{1} \sin \varphi_{2} }}{{l_{1} \left( {\chi_{1}^{{}} \cos \varphi_{2} - \zeta_{1}^{{}} \sin \varphi_{2} } \right)\sin \alpha_{1} }} $$
(A.3)
$$ f_{xr2} = \frac{{ - 2l_{2}^{2} \sin^{2} \varphi_{3} + \chi_{2} \sin \varphi_{3} }}{{l_{1} \left( {\chi_{2}^{{}} \cos \varphi_{3} - \zeta_{2}^{{}} \sin \varphi_{3} } \right)\sin \alpha_{2} }} $$
(A.4)
$$ f_{xl3} = \frac{{\left( {2\zeta_{1} l_{2}^{2} \sqrt {\chi_{1}^{2} + \zeta_{1}^{2} } \cos \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right) - 2\chi_{1} l_{2}^{2} \sqrt {\chi_{1}^{2} + \zeta_{1}^{2} } \sin \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right) + \chi_{1}^{3} + \chi_{1} \zeta_{1}^{2} } \right)}}{{l_{2} \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)^{\frac{3}{2}} \cos \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right)}} $$
(A.5)
$$ f_{xr3} = \frac{{\left( {2\zeta_{2} l_{2}^{2} \sqrt {\chi_{2}^{2} + \zeta_{2}^{2} } \cos \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right) - 2\chi_{2} l_{2}^{2} \sqrt {\chi_{2}^{2} + \zeta_{2}^{2} } \sin \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right) + \chi_{2}^{3} + \chi_{2} \zeta_{2}^{2} } \right)}}{{l_{2} \left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)^{\frac{3}{2}} \cos \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right)}} $$
(A.6)
$$ f_{yl1} = \frac{{\left( {l_{1} l_{2}^{2} 2\sin \alpha_{1} + 2\sin \varphi_{2} l_{2}^{3} + l_{2} \chi_{1} } \right)\cos \varphi_{2} - \zeta_{1} \left( {l_{1} \sin \alpha_{1} + 2l_{2} \sin \varphi_{2} } \right)}}{{l_{1} l_{2} \left( {\chi_{1} \cos \varphi_{2} - \zeta_{1} \sin \varphi_{2} } \right)\sin \alpha_{1} }} $$
(A.7)
$$ f_{yr1} = \frac{{\left( { - 2l_{1} l_{2}^{2} \sin \alpha_{2} - 2\sin \varphi_{3} l_{2}^{3} - l_{2} \chi_{2} } \right)\cos \varphi_{3} - \zeta_{2} \left( {l_{1} \sin \alpha_{2} + 2l_{2} \sin \varphi_{3} } \right)}}{{l_{1} l_{2} \left( {\chi_{2} \cos \varphi_{3} - \zeta_{2} \sin \varphi_{3} } \right)\sin \alpha_{2} }} $$
(A.8)
$$ f_{yl2} = \frac{{\left( {2l_{2}^{2} \sin \varphi_{2} + \chi_{1} } \right)\cos \varphi_{2} - 2\zeta_{1} \sin \varphi_{2} }}{{l_{1} \left( {\chi_{1} \cos \varphi_{2} - \zeta_{1} \sin \varphi_{2} } \right)\sin \alpha_{1} }} $$
(A.9)
$$ f_{yr2} = \frac{{\left( { - 2l_{2}^{2} \sin \varphi_{3} - \chi_{2} } \right)\cos \varphi_{3} + 2\zeta_{2} \sin \varphi_{3} }}{{l_{1} \left( {\chi_{2} \cos \varphi_{3} - \zeta_{2} \sin \varphi_{3} } \right)\sin \alpha_{2} }} $$
(A.10)
$$ f_{yl3} = \frac{{\left( {2\zeta_{1} l_{2}^{2} \sqrt {\chi_{1}^{2} + \zeta_{1}^{2} } \sin \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right) + 2\chi_{1} l_{2}^{2} \sqrt {\chi_{1}^{2} + \zeta_{1}^{2} } \cos \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right) - \zeta_{1}^{3} - \zeta_{1} \chi_{1}^{2} } \right)}}{{l_{2} \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)^{\frac{3}{2}} \cos \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right)}} $$
(A.11)
$$ f_{yr3} = \frac{{\left( { - 2\zeta_{2} l_{2}^{2} \sqrt {\chi_{2}^{2} + \zeta_{2}^{2} } \sin \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right) - 2\chi_{2} l_{2}^{2} \sqrt {\chi_{2}^{2} + \zeta_{2}^{2} } \cos \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right) + \zeta_{2}^{3} + \zeta_{2} \chi_{2}^{2} } \right)}}{{l_{2} \left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)^{\frac{3}{2}} \cos \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right)}} $$
(A.12)
$$ \begin{aligned} f_{\varphi l1} = & L\frac{{\left( { - 2l_{2}^{2} \sin \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right) + \sqrt {\chi_{1}^{2} + \zeta_{1}^{2} } } \right)\left( {\chi_{1} \cos \varphi + \zeta_{1} \sin \varphi } \right)\left( {l_{1} \sin \alpha_{1} + l_{2} \sin \varphi_{2} } \right)}}{{l_{1} l_{2} \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)\sin \alpha_{1} \cos \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right)}} \\ & \quad + \frac{{L\left( { - 2l_{1} l_{2} \left( {\chi_{1} \sin \varphi - \zeta_{1} \cos \varphi } \right)\sin \alpha_{1} - \left( {2\sin \varphi_{2} l_{2}^{2} \chi_{1} + \chi_{1}^{2} + \zeta_{1}^{2} } \right)\sin \varphi + 2\zeta_{1} l_{2}^{2} \cos \varphi \sin \varphi_{2} } \right)}}{{l_{1} \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)\sin \alpha_{1} }} \\ \end{aligned} $$
(A.13)
$$ \begin{aligned} f_{\varphi r1} = & L\frac{{\left( { - 2l_{2}^{2} \sin \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right) + \sqrt {\chi_{2}^{2} + \zeta_{2}^{2} } } \right)\left( { - \chi_{2} \cos \varphi + \zeta_{2} \sin \varphi } \right)\left( {l_{1} \sin \alpha_{2} + l_{2} \sin \varphi_{3} } \right)}}{{l_{1} l_{2} \left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)\sin \alpha_{1} \cos \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right)}} \\ & \quad + \frac{{L\left( {2l_{1} l_{2} \left( {\chi_{2} \sin \varphi + \zeta_{2} \cos \varphi } \right)\sin \alpha_{2} + \left( {2\chi_{3} l_{2}^{2} \sin \varphi_{3} + \chi_{2}^{2} + \zeta_{2}^{2} } \right)\sin \varphi + 2\zeta_{2} l_{2}^{2} \cos \varphi \sin \varphi_{3} } \right)}}{{l_{1} (\chi_{1}^{2} + \zeta_{1}^{2} )\sin \alpha_{1} }} \\ \end{aligned} $$
(A.14)
$$ \begin{aligned} f_{\varphi l2} = & \frac{{\left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)l_{1} \sin \alpha_{1} - L\left( {2l_{2}^{2} \left( {\zeta_{1} \cos \varphi - \chi_{1} \sin \varphi } \right)\sin \varphi_{2} - \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)\sin \varphi } \right)}}{{l_{1} \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)\sin \alpha_{1} }} \\ & \quad - L\sin \varphi_{2} \frac{{ - 2l_{2}^{2} \sin \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right) + \sqrt {\chi_{1}^{2} + \zeta_{1}^{2} } }}{{l_{1} \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)\sin \alpha_{1} \cos \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right)}}\left( {\chi_{1} \cos \varphi + \zeta_{1} \sin \varphi } \right) \\ \end{aligned} $$
(A.15)
$$ \begin{aligned} f_{\varphi r2} = & \frac{{\left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)l_{1} \sin \alpha_{2} - L\left( {2l_{2}^{2} \left( {\zeta_{2} \cos \varphi + \chi_{2} \sin \varphi } \right)\sin \varphi_{3} + \left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)\sin \varphi } \right)}}{{l_{1} \left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)\sin \alpha_{2} }} \\ & \quad - L\sin \varphi_{3} \frac{{ - 2\sin \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right)l_{2}^{2} + \sqrt {\chi_{2}^{2} + \zeta_{2}^{2} } }}{{l_{1} \left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)\sin \alpha_{2} \cos \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right)}}\left( {\chi_{2} \cos \varphi - \zeta_{2} \sin \varphi } \right) \\ \end{aligned} $$
(A.16)
$$ f_{\varphi l3} = - \frac{{L\left( {2l_{2}^{2} \sin \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right) - \sqrt {\chi_{1}^{2} + \zeta_{1}^{2} } } \right)\left( {\zeta_{1} \sin \varphi + \chi_{1} \cos \varphi } \right)}}{{l_{2} \left( {\chi_{1}^{2} + \zeta_{1}^{2} } \right)\cos \left( {\varphi_{2} + \arctan \left( {\frac{{\zeta_{1} }}{{\chi_{1} }}} \right)} \right)}} - \frac{{2Ll_{2} \left( {\chi_{1} \sin \varphi - \zeta_{1} \cos \varphi } \right)}}{{\chi_{1}^{2} + \zeta_{1}^{2} }} $$
(A.17)
$$ f_{\varphi r3} = \frac{{L\left( {2l_{2}^{2} \sin \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right) - \sqrt {\chi_{2}^{2} + \zeta_{2}^{2} } } \right)\left( {\chi_{2} \cos \varphi - \zeta_{2} \sin \varphi } \right)}}{{l_{2} \left( {\chi_{2}^{2} + \zeta_{2}^{2} } \right)\cos \left( {\varphi_{3} + \arctan \left( {\frac{{\zeta_{2} }}{{\chi_{2} }}} \right)} \right)}} - \frac{{2Ll_{2} \left( {\chi_{2} \sin \varphi - \zeta_{2} \cos \varphi } \right)}}{{\chi_{2}^{2} + \zeta_{2}^{2} }} $$
(A.18)
$$ f_{x4} = \Delta s_{l} \frac{{\left( {H + h_{1} \sin \varphi_{4} - h_{2} \cos \varphi_{4} - X - LL\cos \varphi } \right)}}{{4\left( {\Delta s_{l} + l_{0} } \right) \, }} + \Delta s_{r} \frac{{\left( {H - h_{1} \sin \varphi_{4} - h_{2} \cos \varphi_{4} - X - LL\cos \varphi } \right)}}{{4\left( {\Delta s_{r} + l_{0} } \right)}} $$
(A.19)
$$ f_{y4} = \Delta s_{l} \frac{{(h_{1} \cos \varphi_{4} + h_{2} \sin \varphi_{4} - Y + LL\sin \varphi )}}{{4(\Delta s_{l} + l_{0} )}} + \Delta s_{r} \frac{{( - h_{1} \cos \varphi_{4} + h_{2} \sin \varphi_{4} - Y + LL\sin \varphi )}}{{4(\Delta s_{r} + l_{0} )}} $$
(A.20)
$$ \begin{aligned} f_{\varphi 4} = & \Delta s_{l} LL\frac{{\left( {h_{2} \cos \varphi_{4} - h_{1} \sin \varphi_{4} - H + X} \right)\sin \varphi - \cos \varphi \left( {h_{1} \cos \varphi_{4} + h_{2} \sin \varphi_{4} - Y} \right)}}{{4\left( {\Delta s_{l} + l_{0} } \right)}} \, \\ & \quad + \Delta s_{r} LL\frac{{\left( {h_{2} \cos \varphi_{4} + h_{1} \sin \varphi_{4} - H + X} \right)\sin \varphi + \cos \varphi \left( {h_{1} \cos \varphi_{4} - h_{2} \sin \varphi_{4} + Y} \right)}}{{4\left( {\Delta s_{r} + l_{0} } \right)}} \\ \end{aligned} $$
(A.21)

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, J., Yao, G. Multi-directional vibration isolation performances of a scissor-like structure with nonlinear hybrid spring stiffness. Nonlinear Dyn (2024). https://doi.org/10.1007/s11071-024-09561-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11071-024-09561-4

Keywords

Search

Navigation