A hybrid linear dynamic absorber and nonlinear energy sink for broadband absorption of a circular ring

Abstract

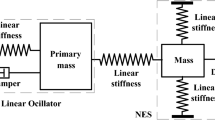
Nonlinear energy sinks (NES) have been shown to have broadband absorption for multiple modes. Furthermore, linear dynamic absorbers (LDA) often only operate effectively within located frequency ranges and are unable to achieve broadband absorption. Therefore, this manuscript first proposed using a hybrid LDA and NES to design the circular ring absorption system. Compared with LDA, broadband absorption could be achieved through configuring and tuning linear stiffness. Both the linear and nonlinear stiffnesses are introduced by adding vertical and horizontal springs. The linear and nonlinear stiffness effects on the circular ring absorption system are investigated simultaneously. The dynamic model of the circular ring absorption system with hybrid LDA and NES is constructed, and the approximate analytical solution is obtained using the harmonic balance analysis. The expressions of frequency response and force transmissibility are given, and the analytical results are verified numerically. By comparing the amplitude-frequency response curves of the circular ring with LDA only, NES only, and a hybrid LDA and NES, it is confirmed that a hybrid LDA and NES can better control the forced vibration of the circular ring absorption system. Hybrid LDA and NES were shown to suppress main resonance peaks of the circular ring absorption system, making the device resistant to misalignments. In a linear system, this can only be achieved by resonance matching, so a hybrid LDA and NES have the same effect as adding multiple linear absorbers. Some properties of damping, nonlinear stiffness, and mass ratios in a hybrid LDA and NES are investigated. These parameters determine the specific shape of the force transmissibility curve of the ring absorption system. It is found that a proper increase in the mass ratio of the hybrid LDA and NES can obtain a better vibration reduction effect; adjusting the damping ratio and nonlinear stiffness ratio of the hybrid LDA and NES can improve the vibration reduction effect to a certain extent. Based on the analysis of the parameters effects of hybrid LDA and NES on vibration control, the parameters of hybrid LDA and NES are compared. The analysis of the results demonstrates that by selecting reasonable damping, nonlinear stiffness, and mass ratios, the frequency band of the circular ring absorption system can be broadened. Finally, an experiment is performed to validate the correctness of the proposed method.

Appendices

Appendix A: Stability analysis

To analyze the stability of the harmonic solutions, the fundamental harmonic solution is rewritten as:

$$\begin{aligned}{} & {} \hat{x}_\mathrm{{a}}^{*}(\tau )=\cos (\Omega \tau ){{A}_{11}}(\tau )+\sin (\Omega \tau ){{A}_{21}}(\tau ) \end{aligned}$$

(A1a)

$$\begin{aligned}{} & {} q_\mathrm{{u}2}^{*}(\tau )=\cos (\Omega \tau ){{B}_{11}}(\tau )+\sin (\Omega \tau ){{B}_{21}}(\tau ) \end{aligned}$$

(A1b)

$$\begin{aligned}{} & {} q_\mathrm{{v}2}^{*}(\tau )=\cos (\Omega \tau ){{D}_{11}}(\tau )+\sin (\Omega \tau ){{D}_{21}}(\tau ) \end{aligned}$$

(A1c)

The first and second derivatives of (A1a–A1c) versus $\tau$ yield

$$\begin{aligned}{} & {} \hat{x}_{\text {a}}^{'*}(\tau )=\cos (\Omega \tau )({{\text {A}}^{'}}_{11}+\Omega {{A}_{21}})\nonumber \\{} & {} \quad +\sin (\Omega \tau )({{\text {A}}^{'}}_{21}-\Omega {{A}_{11}}) \end{aligned}$$

(A2a)

$$\begin{aligned}{} & {} q_{u2}^{'*}(\tau )=\cos (\Omega \tau )({{B}^{'}}_{11}+\Omega {{B}_{21}})\nonumber \\{} & {} \quad +\sin (\Omega \tau )({{B}^{'}}_{21}-\Omega {{B}_{11}}) \end{aligned}$$

(A2b)

$$\begin{aligned}{} & {} q_{\text {v}2}^{'*}(\tau )=\cos (\Omega \tau )({{D}^{'}}_{11}+\Omega {{D}_{21}})\nonumber \\{} & {} \quad +\sin (\Omega \tau )({{D}^{'}}_{21}-\Omega {{D}_{11}}) \end{aligned}$$

(A2c)

$$\begin{aligned}{} & {} \hat{x}_{\text {a}}^{''*}(\tau )=\cos (\Omega \tau )({{\text {A}}^{''}}_{11}+2\Omega {{A}^{'}}_{21}-{{\Omega }^{2}}{{A}_{11}})\nonumber \\{} & {} \quad +\sin (\Omega \tau )({{\text {A}}^{''}}_{21}-2\Omega {{A}^{'}}_{11}-{{\Omega }^{2}}{{A}_{21}}) \end{aligned}$$

(A2d)

$$\begin{aligned}{} & {} q_{u2}^{''*}(\tau )=\cos (\Omega \tau )({{B}^{''}}_{11}+2\Omega {{B}^{'}}_{21}-{{\Omega }^{2}}{{B}_{11}})\nonumber \\{} & {} \quad +\sin (\Omega \tau )({{B}^{''}}_{21}-2\Omega {{B}^{'}}_{11}-{{\Omega }^{2}}{{B}_{21}}) \end{aligned}$$

(A2e)

$$\begin{aligned}{} & {} q_{\text {v}2}^{''*}(\tau )=\cos (\Omega \tau )({{D}^{''}}_{11}+2\Omega {{D}^{'}}_{21}-{{\Omega }^{2}}{{D}_{11}})\nonumber \\{} & {} \quad +\sin (\Omega \tau )({{D}^{''}}_{21}-2\Omega {{D}^{'}}_{11}-{{\Omega }^{2}}{{D}_{21}}) \end{aligned}$$

(A2f)

Substituting equations (A2a-A2f) into the equations (17a-17c) and equating the coefficients of and to zero, six differential-algebraic equations are obtained, in which the points of HBM are fixed ones.

$$\begin{aligned}{} & {} \begin{aligned}&\mu A_{11}^{''}+2\mu \Omega A_{21}^{'}-\mu {{\Omega }^{2}}A_{11}^{{}}\\&+F({{A}_{11}},{{A}_{21}},{{D}_{11}},{{D}_{21}},A_{11}^{'},A_{21}^{'},D_{11}^{'}, \\&D_{21}^{'},{{\zeta }_{\text {n}}},\gamma ,\varepsilon ,\mu ,\Omega )=0 \\ \end{aligned} \end{aligned}$$

(A3a)

$$\begin{aligned}{} & {} \begin{aligned}&\mu A_{21}^{''}-2\mu \Omega A_{11}^{'}-\mu {{\Omega }^{2}}{{A}_{21}}\\&+F({{A}_{11}},{{A}_{21}},{{D}_{11}},{{D}_{21}},A_{11}^{'},A_{21}^{'},D_{11}^{'}, \\&D_{21}^{'},{{\zeta }_{\text {n}}},\gamma ,\varepsilon ,\mu ,\Omega )=0 \\ \end{aligned} \end{aligned}$$

(A3b)

$$\begin{aligned}{} & {} \begin{aligned}&{{\mu }_{\text {r}}}B_{11}^{''}+2{{\mu }_{\text {r}}}\Omega B_{21}^{'}-{{\mu }_{\text {r}}}{{\Omega }^{2}}{{B}_{11}}\\&+F({{B}_{11}},{{B}_{21}},{{D}_{11}},{{D}_{21}},B_{11}^{'},B_{21}^{'},D_{11}^{'}, \\&D_{21}^{'},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r11}}},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r12}}},{{\zeta }_{1}},{{\mu }_{\text {r}}},\Omega )=0 \\ \end{aligned} \end{aligned}$$

(A3c)

$$\begin{aligned}{} & {} \begin{aligned}&{{\mu }_{\text {r}}}B_{21}^{''}-2{{\mu }_{\text {r}}}\Omega B_{11}^{'}-{{\mu }_{\text {r}}}{{\Omega }^{2}}{{B}_{21}}\\&+F({{B}_{11}},{{B}_{21}},{{D}_{11}},{{D}_{21}},B_{11}^{'},B_{21}^{'},D_{11}^{'}, \\&D_{21}^{'},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r}11}},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r}12}},{{\zeta }_{1}},{{\mu }_{\text {r}}},\Omega )=0 \\ \end{aligned} \end{aligned}$$

(A3d)

$$\begin{aligned}{} & {} \begin{aligned}&D_{11}^{''}+2\Omega D_{21}^{'}-{{\Omega }^{2}}{{D}_{11}}\\&+F({{A}_{11}},{{A}_{21}},{{B}_{11}},{{B}_{21}},{{D}_{11}}, {{D}_{21}},A_{11}^{'},A_{21}^{'},B_{11}^{'}, \\&B_{21}^{'},D_{11}^{'},D_{21}^{'},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r11}}},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r12}}},{{\zeta }_{\text {n}}},{{\zeta }_{2}},\mu ,\gamma ,\varepsilon ,\Omega )=0 \\ \end{aligned} \nonumber \\ \end{aligned}$$

(A3e)

$$\begin{aligned}{} & {} \begin{aligned}&D_{21}^{''}-2\Omega D_{11}^{'}-{{\Omega }^{2}}{{D}_{21}}\\&+F({{A}_{11}},{{A}_{21}},{{B}_{11}},{{B}_{21}},{{D}_{11}},{{D}_{21}},A_{11}^{'},A_{21}^{'},B_{11}^{'}, \\&B_{21}^{'},D_{11}^{'},D_{21}^{'},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r11}}},{{\overset{\wedge }{\mathop {k}}\,}_{\text {r}12}},{{\zeta }_{\text {n}}},{{\zeta }_{2}},\mu ,\gamma ,\varepsilon ,\Omega )=0 \\ \end{aligned} \nonumber \\ \end{aligned}$$

(A3f)

$q_{u2}^{*}$, $q_{v2}^{*}$ and $x_{a}^{*}$ are HBM solutions, $\Delta q_{u2}$, $\Delta q_{v2}$ and $\Delta x_{a}$ are the deviation values of HBM solution, so:

$$\begin{aligned} {{x}_{\text {a}}}= & {} x_{\text {a}}^{*}+\Delta {{x}_{\text {a}}} \end{aligned}$$

(A4a)

$$\begin{aligned} {{q}_{u2}}= & {} q_{u2}^{*}+\Delta {{q}_{u2}} \end{aligned}$$

(A4b)

$$\begin{aligned} {{q}_{v2}}= & {} q_{v2}^{*}+\Delta {{q}_{v2}} \end{aligned}$$

(A4c)

where

$$\begin{aligned} \Delta {{x}_{a}}= & {} \Delta {{A}_{11}}\cos (\Omega \tau )+\Delta {{A}_{21}}\sin (\Omega \tau ) \end{aligned}$$

(A5a)

$$\begin{aligned} \Delta {{q}_{u2}}= & {} \Delta {{B}_{11}}\cos (\Omega \tau )+\Delta {{B}_{21}}\sin (\Omega \tau ) \end{aligned}$$

(A5b)

$$\begin{aligned} \Delta {{q}_{\text {v}2}}= & {} \Delta {{D}_{11}}\cos (\Omega \tau )+\Delta {{D}_{21}}\sin (\Omega \tau ) \end{aligned}$$

(A5c)

The perturbation equation is obtained by linearizing six algebraic differential equations at fixed points

$$\begin{aligned} \textbf{M}_{1}{{\mathbf {\varphi }}^{\prime \prime }}+\textbf{C}_{1}{{\mathbf {\varphi }}^{\prime }}+\textbf{K }_{1}\varphi =0 \end{aligned}$$

(A6)

where $\varphi =\left[ \begin{matrix} \Delta {{A}_{11}} \\ \Delta {{A}_{21}} \\ \Delta {{B}_{11}} \\ \Delta {{B}_{21}} \\ \Delta {{D}_{11}} \\ \Delta {{D}_{21}} \\ \end{matrix} \right]$,${{\varphi }^{'}}=\left[ \begin{matrix} \Delta {{A}^{'}}_{11} \\ \Delta {{A}^{'}}_{21} \\ \Delta {{B}^{'}}_{11} \\ \Delta {{B}^{'}}_{21} \\ \Delta {{D}^{'}}_{11} \\ \Delta {{D}^{'}}_{21} \\ \end{matrix} \right]$,${{\varphi }^{''}}=\left[ \begin{matrix} \Delta {{A}^{''}}_{11} \\ \Delta {{A}^{''}}_{21} \\ \Delta {{B}^{''}}_{11} \\ \Delta {{B}^{''}}_{21} \\ \Delta {{D}^{''}}_{11} \\ \Delta {{D}^{''}}_{21} \\ \end{matrix} \right]$, $\textbf{M}_{1}=\left[ \begin{array}{cccccc} \mu &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} \mu &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} \mu _r &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} \mu _r &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 1 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 1 \end{array}\right]$,

$$\begin{aligned} \textbf{C}_{1}= & {} \left[ \begin{matrix} 2\mu {{\zeta }_{n}} &{}\quad 2\mu \Omega &{}\quad 0 &{}\quad 0 &{}\quad 2\mu {{\zeta }_{n}} &{}\quad 0 \\ -2\mu \Omega &{}\quad 2\mu {{\zeta }_{n}} &{}\quad 0 &{}\quad 0 &{}\quad 0 &{}\quad -2\mu {{\zeta }_{n}} \\ 0 &{}\quad 0 &{}\quad 2{{\mu }_{r}}{{\zeta }_{1}} &{}\quad 2{{\mu }_{r}}\Omega &{}\quad 0 &{}\quad 0 \\ 0 &{}\quad 0 &{}\quad -2{{\mu }_{r}}\Omega &{}\quad 2{{\mu }_{r}}{{\zeta }_{1}} &{}\quad 0 &{}\quad 0 \\ -2\mu {{\zeta }_{n}} &{}\quad 0 &{}\quad 0 &{}\quad 0 &{}\quad 2\mu {{\zeta }_{n}}+2{{\zeta }_{2}} &{}\quad 2\Omega \\ 0 &{}\quad -2\mu {{\zeta }_{n}} &{}\quad 0 &{}\quad 0 &{}\quad -2\Omega &{}\quad 2\mu {{\zeta }_{n}}+2{{\zeta }_{2}} \\ \end{matrix} \right] ,\\ \end{aligned}$$

$$\begin{aligned} \textbf{K}_{1}= & {} \left[ \begin{matrix} {{K}_{11}} &{}\quad {{K}_{12}} &{}\quad {{K}_{13}} &{}\quad {{K}_{14}} &{}\quad {{K}_{15}} &{}\quad {{K}_{16}} \\ {{K}_{21}} &{}\quad {{K}_{22}} &{}\quad {{K}_{23}} &{}\quad {{K}_{24}} &{}\quad {{K}_{25}} &{}\quad {{K}_{26}} \\ {{K}_{31}} &{}\quad {{K}_{32}} &{}\quad {{K}_{33}} &{}\quad {{K}_{34}} &{}\quad {{K}_{35}} &{}\quad {{K}_{36}} \\ {{K}_{41}} &{}\quad {{K}_{42}} &{}\quad {{K}_{43}} &{}\quad {{K}_{44}} &{}\quad {{K}_{45}} &{}\quad {{K}_{46}} \\ {{K}_{51}} &{}\quad {{K}_{52}} &{}\quad {{K}_{53}} &{} \quad {{K}_{54}} &{}\quad {{K}_{55}} &{}\quad {{K}_{56}} \\ {{K}_{61}} &{}\quad {{K}_{62}} &{}\quad {{K}_{63}} &{}\quad {{K}_{64}} &{}\quad {{K}_{65}} &{}\quad {{K}_{66}} \\ \end{matrix} \right] \\ {{K}_{11}}= & {} \varepsilon -\mu {{\Omega }^{2}}+\frac{3}{4}\gamma (3D_{11}^{2}-2{{A}_{21}}{{D}_{21}}\\{} & {} +A_{21}^{2}+D_{21}^{2}+A_{11}^{2}-3{{A}_{11}}{{D}_{11}}),\\ {{K}_{12}}= & {} 2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma ({{D}_{11}}{{D}_{21}}-{{A}_{11}}{{D}_{21}}\\{} & {} +2{{A}_{11}}{{A}_{21}}-2{{A}_{21}}{{D}_{11}}),\\ {{K}_{13}}= & {} 0,\\ {{K}_{14}}= & {} 0,\\ {{K}_{15}}= & {} -\varepsilon +\frac{3}{2}\gamma \left( \frac{3}{2}{{A}_{11}}{{D}_{11}}+{{A}_{21}}{{D}_{21}}-\frac{1}{2}D_{11}^{2}\right. \\{} & {} \left. -\frac{1}{2}D_{21}^{2}-\frac{1}{2}A_{11}^{2}{{D}_{11}}-\frac{1}{2}A_{21}^{2}{{D}_{11}}\right) ,\\ {{K}_{16}}= & {} -2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma \left( {{A}_{11}}D_{21}^{2}\right. \\{} & {} \left. +2{{A}_{21}}{{D}_{11}}-{{D}_{11}}D_{21}^{2}-{{A}_{11}}{{A}_{21}}\right) ,\\ {{K}_{16}}= & {} -2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma \left( {{A}_{11}}D_{21}^{2}\right. \\{} & {} \left. +2{{A}_{21}}{{D}_{11}}-{{D}_{11}}D_{21}^{2}-{{A}_{11}}{{A}_{21}}\right) ,\\ {{K}_{22}}= & {} \varepsilon -\mu {{\Omega }^{2}}+\frac{3}{4}\gamma \left( A_{21}^{2}-3{{A}_{21}}{{D}_{21}}\right. \\{} & {} \left. +3D_{21}^{2}-2{{A}_{11}}{{D}_{11}}+A_{11}^{2}+D_{11}^{2}\right) ,\\ {{K}_{23}}= & {} 0,{{K}_{24}}=0,\\ {{K}_{25}}= & {} 2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma (2{{A}_{11}}{{D}_{21}}\\{} & {} -2{{A}_{11}}{{A}_{21}}+{{A}_{21}}{{D}_{11}}-{{D}_{11}}{{D}_{21}}),\\ {{K}_{26}}= & {} -\varepsilon +\frac{3}{4}\gamma (3{{A}_{21}}{{D}_{21}}\\{} & {} +2{{A}_{11}}{{D}_{11}}-D_{21}^{2}-3A_{21}^{2}-A_{11}^{2}-D_{11}^{2}),\\ {{K}_{31}}= & {} 0,{{K}_{32}}=0,\\ {{K}_{33}}= & {} -\mu {{\Omega }^{2}}+{{\overset{\wedge }{\mathop {k}}\,}_{r11}},\\ {{K}_{34}}= & {} 2{{\zeta }_{1}}{{\mu }_{r}}\Omega ,{{K}_{35}}={{\overset{\wedge }{\mathop {k}}\,}_{r22}},\\ {{K}_{36}}= & {} 0,{{K}_{41}}=0,{{K}_{42}}=0,\\ {{K}_{43}}= & {} -2{{\zeta }_{1}}{{\mu }_{r}}\Omega ,\\ {{K}_{44}}= & {} -{{\mu }_{r}}{{\Omega }^{2}}+{{\overset{\wedge }{\mathop {k}}\,}_{r11}},\\ {{K}_{45}}= & {} 0,\\ {{K}_{46}}= & {} {{\overset{\wedge }{\mathop {k}}\,}_{r12}},\\ {{K}_{51}}= & {} -\varepsilon +\frac{3}{4}\gamma (3{{A}_{11}}{{D}_{11}}+2{{A}_{21}}{{D}_{21}}\\{} & {} -D_{21}^{2}-A_{21}^{2}-3D_{11}^{2}-A_{11}^{2}),\\ {{K}_{52}}= & {} -2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma ({{A}_{21}}{{D}_{11}}+2{{A}_{11}}{{D}_{21}}\\{} & {} -{{A}_{11}}A_{21}^{2}-2{{D}_{11}}{{D}_{21}}),{{K}_{53}}=0,{{K}_{54}}=0,\\ {{K}_{55}}= & {} 1+\varepsilon -{{\Omega }^{2}}+\frac{3}{4}\gamma (A_{21}^{2}+D_{21}^{2}+3A_{11}^{2}\\{} & {} -3{{A}_{11}}{{D}_{11}}-2{{A}_{21}}{{D}_{21}}+D_{11}^{2}),\\ {{K}_{56}}= & {} 2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma ({{D}_{11}}{{D}_{21}}\\{} & {} -{{A}_{11}}{{D}_{21}}+2{{A}_{11}}{{A}_{21}}-2{{A}_{21}}{{D}_{11}}),\\ {{K}_{61}}= & {} 2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma (2{{A}_{11}}{{D}_{21}}+2{{A}_{21}}{{D}_{11}}\\{} & {} -{{A}_{11}}{{A}_{21}}-{{D}_{11}}{{D}_{21}}),\\ {{K}_{62}}= & {} -\varepsilon +\frac{3}{4}\gamma (3{{A}_{21}}{{D}_{21}}+2{{A}_{11}}{{D}_{11}}-A_{11}^{2}\\ {}{} & {} -D_{11}^{2}-3D_{21}^{2}-A_{21}^{2}),{{K}_{63}}=0,{{K}_{64}}={{\overset{\wedge }{\mathop {k}}\,}_{r22}},\\ {{K}_{65}}= & {} -2{{\zeta }_{2}}\Omega -2{{\zeta }_{n}}\mu \Omega +\frac{3}{4}\gamma ({{D}_{11}}{{D}_{21}} \\{} & {} -{{A}_{21}}{{D}_{11}}-2{{A}_{11}}{{D}_{21}}+2{{A}_{11}}{{A}_{21}}),\\ {{K}_{66}}= & {} 1+\varepsilon -{{\Omega }^{2}}+\frac{3}{4}\gamma (A_{11}^{2}+D_{11}^{2}\\{} & {} +3A_{21}^{2}-3{{A}_{21}}{{D}_{21}}-2{{A}_{11}}{{D}_{11}}+D_{21}^{2}). \end{aligned}$$

The following formula can determine the eigenvalues:

$$\begin{aligned} \textbf{M}{{\mathbf {\varphi }}^{\prime \prime }}+\textbf{C}{{\mathbf {\varphi }}^{\prime }}+\mathbf {K\varphi }=0 \end{aligned}$$

(A7)

where $\lambda$ is the eigenvalue. The stability is determined by calculating the eigenvalues. If the real part of the eigenvalue is negative, the solution of HBM is stable. Otherwise, the solution of HBM is considered unstable.

Appendix B: Table of parameters

The parameters in Eq. (14) are as follows:

$$\begin{aligned} \begin{array}{ll} a_{j k}=\int _{0}^{2 \pi } \phi _{u j}(s) \phi _{u k}(s) ds &{} b_{j k}=\int _{0}^{2 \pi } \phi _{u j}(s) \phi _{u k, s s}(s) d s \\ c_{j k}=\int _{0}^{2 \pi } \phi _{u j}(s) \phi _{v k, s}(s) d s &{} d_{j k}=\int _{0}^{2 \pi } \phi _{u j}(s) \phi _{v k, s s s}(s) d s\\ e_{j k}=\int _{0}^{2 \pi } \phi _{v j}(s) \phi _{v k}(s) d s &{} f_{j k}=\int _{0}^{2 \pi } \phi _{v j}(s) \phi _{v k, s s s s}(s) d s \\ g_{j k}=\int _{0}^{2 \pi } \phi _{v j}(s) \phi _{u k, s s s}(s) d s &{} h_{j k}=\int _{0}^{2 \pi } \phi _{v j}(s) \phi _{u k, s}(s) d s \\ l_{j k}=\int _{0}^{2 \pi } \phi _{v j}(s) \phi _{v k}(s) d s &{}m_{j k}=\int _{0}^{2 \pi } \phi _{u^{*} j}(s) \phi _{u^{*} k}(s) d s \\ n_{j k}=\int _{0}^{2 \pi } \phi _{u^{*} j}(s) \phi _{u^{*} k, s s}(s) d s &{} o_{j k}=\int _{0}^{2 \pi } \phi _{u^{*} j}(s) \phi _{v^{*} k, s}(s) d s\\ p_{j k}=\int _{0}^{2 \pi } \phi _{u^{*} j}(s) \phi _{v^{*} k, s s s}(s) d s &{} q_{j k}=\int _{0}^{2 \pi } \phi _{v^{*} j}(s) \phi _{v^{*} k}(s) d s \\ r_{j k}=\int _{0}^{2 \pi } \phi _{v^{*} j}(s) \phi _{v^{*} k, s s s s}(s) d s &{} s_{j k}=\int _{0}^{2 \pi } \phi _{v^{*} j}(s) \phi _{u^{\circ } k, s s s}(s) d s\\ w_{j k}=\int _{0}^{2 \pi } \phi _{v^{*} j}(s) \phi _{u^{*} k, s}(s) d s &{} y_{j k}=\int _{0}^{2 \pi } \phi _{v^{*} j}(s) \phi _{v^{*} k}(s) d s \\ \end{array} \end{aligned}$$

The parameters in equation (21) are as follows:

${{X}_{1}}={{A}_{11}}$,${{X}_{2}}={{A}_{21}}$,${{X}_{3}}={{A}_{13}}$,${{X}_{4}}={{A}_{23}}$, ${{X}_{5}}={{B}_{11}}$,${{X}_{6}}={{B}_{21}}$,${{X}_{7}}={{B}_{13}}$,${{X}_{8}}={{B}_{23}}$, ${{X}_{9}}={{D}_{11}}$,${{X}_{10}}={{D}_{21}}$,${{X}_{11}}={{D}_{13}}$,${{X}_{12}}={{D}_{23}}$, ${{X}_{13}}={{E}_{11}}$,${{X}_{14}}={{E}_{21}}$,${{X}_{15}}={{E}_{13}}$,${{X}_{16}}={{E}_{23}}$,${{Y}_{1}}={{\zeta }_{1}}$,${{Y}_{2}}={{\zeta }_{2}}$,${{Y}_{3}}={{\mu }_{\text {r}}}$,${{Y}_{4}}=\mu$,${{Y}_{5}}={{\omega }_{\text {n}}}$,${{Y}_{6}}={{\hat{k}}_{r11}}$,${{Y}_{7}}={{\hat{k}}_{\text {r}22}}$,${{Y}_{8}}=\varepsilon$,${{Y}_{9}}=\tau$,${{Y}_{9}}=\tau$,${{Y}_{10}}={{\hat{F}}_{\text {e}}}$.