Consequences and benefits of utilizing continuous vibro-impact representations in constrained pipeline conveying fluid systems

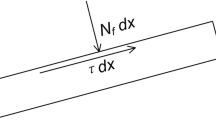
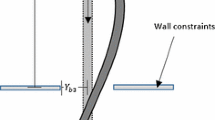
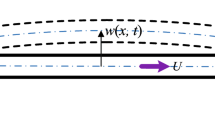
Abstract

The effectiveness of continuous vibro-impact forcing representations for the cantilevered pipe that conveys fluid is explored and analyzed. The previously accepted forcing model utilizing a smoothened trilinear spring is estimated using three continuous forcing representations, namely, polynomial, rational polynomial, and hyperbolic tangent. The accuracy of the estimated forcing functions is investigated and analyzed by calculating the root mean square error, and bifurcation diagrams are generated and compared to the nominal system. Additionally, the dynamic response of the system is further characterized using Poincare maps, power spectra, and basins of attraction. Once all continuous forcing representations are analyzed and compared to the nominal system, the computational cost of each method is examined, and further limitations of the hyperbolic tangent method are discovered. It is proved that the hyperbolic tangent forcing representation most accurately captures the dynamic response of the pipeline, and the least accurate representation is the rational polynomial representation. Additionally, considerable computational cost is saved when employing the hyperbolic tangent representation compared to the discontinuous representation.

Appendix

Dimensional equation of motion:

$$ \begin{aligned} & EI\left( {Y^{iv} + \eta \dot{Y}^{iv} } \right) + \left( {m + M} \right)gY^{\prime} + 2MU\dot{Y}^{\prime} + MU^{2} Y^{\prime\prime} - \left( {m + M} \right)g\left( {L - S} \right)Y^{\prime\prime} + \left( {m + M} \right)\ddot{Y} + 2MU\dot{Y}^{\prime}Y^{{\prime}{2}} \\ & \quad + Y^{\prime\prime}Y^{{\prime}{2}} \left[ {MU^{2} - \frac{3}{2}\left( {m + M} \right)g\left( {L - S} \right)} \right] + \frac{1}{2}g\left( {m + M} \right)Y^{{\prime}{3}} + EI\left( {Y^{iv} Y^{{\prime}{2}} + 4Y^{\prime\prime\prime}Y^{\prime\prime}Y^{\prime} + Y^{{\prime\prime}{3}} } \right) \\ & \quad - Y^{\prime\prime}\left[ {\int_{s}^{L} {2MUY^{\prime}\dot{Y}^{\prime} + MU^{2} Y^{\prime}Y^{\prime\prime} + \int_{0}^{s} {\left( {m + M} \right)\left( {\dot{Y}^{{\prime}{2}} + Y^{\prime}\ddot{Y^{\prime}}} \right){\text{d}}S} \,{\text{d}}S} } \right] + Y^{\prime}\int_{0}^{s} {\left( {m + M} \right)\left( {\dot{Y}^{{\prime}{2}} + Y^{\prime}\ddot{Y}^{\prime}} \right){\text{d}}S = 0} \\ \end{aligned} $$

(10)

Terms from Eq. (4)

$$ C_{ij} = \xi \int_{0}^{1} {\lambda^{4} \phi_{j} \phi_{i} {\text{d}}s = \delta_{ij} \xi_{i} \lambda_{i}^{2} } $$

(11)

$$ \left( {Cu} \right)_{ij} = 2\sqrt {m_{r} } \int_{0}^{1} {\phi^{\prime}_{j} \phi_{i} } \, $$

(12)

$$ K_{ij} = \int_{0}^{1} {\left[ {\lambda^{4} \phi_{j} - \gamma \left( {1 - s} \right)\phi^{\prime\prime}_{j} + \phi^{\prime}_{j} } \right]\phi_{i} {\text{d}}s} $$

(13)

$$ \left( {Ku} \right)_{ij} = \int\limits_{0}^{1} {\phi^{\prime\prime}_{j} \phi_{i} {\text{d}}s} $$

(14)

$$ C = C_{ij} + u\left( {C_{u} } \right)_{ij} $$

(15)

$$ K = K_{ij} + u^{2} \left( {K_{u} } \right)_{ij} $$

(16)

$$ M_{ijkl} = \int_{0}^{1} {\left( {\phi^{\prime}_{l} \int_{0}^{s} {\phi^{\prime}_{k} \phi^{\prime}_{j} {\text{d}}s} - \phi^{\prime\prime}_{l} \int_{s}^{1} {\int_{0}^{s} {\phi^{\prime}_{k} \phi^{\prime}_{j} {\text{d}}s} {\text{d}}s} } \right)\phi_{i} {\text{d}}s} $$

(17)

$$ N_{ijkl} = 2\sqrt {m_{r} } \int_{0}^{1} {\left( {\phi^{\prime}_{l} \phi^{\prime}_{k} \phi^{\prime}_{j} + \phi^{\prime}_{l} \int_{0}^{s} {\phi^{\prime\prime}_{k} \phi^{\prime}_{j} {\text{d}}s} - \phi^{\prime\prime}_{l} \int_{s}^{1} {\left( {\phi^{\prime\prime}_{k} \phi^{\prime}_{j} {\text{d}}s - \int_{0}^{s} {\phi^{\prime\prime}_{k} \phi^{\prime}_{j} {\text{d}}s} } \right){\text{d}}s} } \right)\phi_{i} {\text{d}}s} $$

(18)

$$ \begin{gathered} P_{ijkl} = \int_{0}^{1} {} \left[ { - \frac{3}{2}\gamma \left( {1 - s} \right)\phi^{\prime\prime}_{l} \phi^{\prime}_{k} \phi^{\prime}_{j} - \frac{1}{2}g\phi^{\prime}_{l} \phi^{\prime}_{k} \phi^{\prime}_{j} + 3\phi^{\prime\prime\prime}_{l} \phi^{\prime\prime}_{k} \phi^{\prime}_{j} + \phi^{\prime\prime}\phi^{\prime\prime}\phi^{\prime\prime}} \right. \hfill \\ + \phi^{\prime}_{l} \int_{0}^{s} {\left( {\gamma \left( {1 - s} \right)\phi^{\prime\prime\prime}_{k} \phi^{\prime}_{j} + \phi_{k}^{\prime \prime \prime } \phi^{\prime\prime}_{j} } \right){\text{d}}s} - \phi^{\prime\prime}_{l} \int_{s}^{1} {\left( {\left( { - \gamma \phi^{\prime}_{k} \phi^{\prime}_{j} + \phi_{k}^{iv} \phi^{\prime\prime}_{j} } \right){\text{d}}s} \right.} \hfill \\ \left. {\left. { + \int_{0}^{s} {\left( {\gamma \left( {1 - s} \right)\phi^{\prime\prime\prime}_{k} \phi^{\prime}_{j} + \phi_{k}^{iv} \phi^{\prime\prime}_{j} } \right)_{j} {\text{d}}s} } \right){\text{d}}s} \right]\phi_{i} {\text{d}}s \hfill \\ \end{gathered} $$

(19)

$$ \left( {Pu} \right)_{ijkl} = \int_{0}^{1} {\left( {\phi^{\prime\prime}_{l} \phi^{\prime}_{k} \phi^{\prime}_{j} - \phi^{\prime}_{l} \int_{0}^{s} {\phi^{\prime\prime\prime}_{k} \phi^{\prime}_{j} {\text{d}}s} - \phi^{\prime\prime}_{l} \int_{s}^{1} {\left( {\phi^{\prime\prime}_{k} \phi^{\prime}_{j} {\text{d}}s - \int_{0}^{s} {\phi^{\prime\prime\prime}_{k} \phi^{\prime}_{j} {\text{d}}s} } \right){\text{d}}s} } \right)\phi_{i} {\text{d}}s} $$

(20)