Dynamical characterization of fully nonlinear, nonsmooth, stall fluttering airfoil systems

Abstract

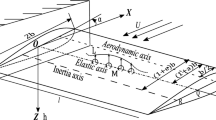
Stall flutter is turning into a more likely condition to be encountered as the demand for increasingly more flexible wings grows for HALE-like aircraft. Due to the various nonlinearities involved that can lead to complex motion, the characterization of the dynamical behavior in the post-flutter condition becomes important. The dynamics of a pitch–plunge idealized HALE typical section with aerodynamic, structural and kinematic nonlinearities in the stall flutter regime was investigated using an aeroelastic state-space formulation which includes a modified Beddoes-Leishman dynamic stall model. The results reveal that period-doubling was possible without stall, but chaos arose at discontinuity-induced bifurcations due to dynamic stall. A parametric study has been conducted to assess the influence of key parameters in the development of bifurcations and chaos.

Appendices

Semi-empirical model parameters

The Beddoes-Leishman model coefficients arising from the circulatory indicial functions for the NACA 0012 airfoil are given in Table 5:

Table 5 Circulatory indicial response coefficients for the NACA 0012 airfoil

Table 6 presents the constants used in the Beddoes-Leishman model as functions of the Mach number for that same airfoil. The values are spline interpolated for intermediary Mach numbers, and for $M < 0.035$, the values at $M = 0.035$ are taken.

Table 6 NACA 0012 airfoil Mach-dependent empirical constants

Semi-empirical model functions

In Eqs. 16 –  18, the offsets of the breakpoint angles, $\varDelta \alpha _{1_n}$, $\varDelta \alpha _{1_m}$ and $\varDelta \alpha _{1_c}$, are dependent of the current state space and on the motion condition (i.e., upstroke or downstroke), which are determined by the variables S and P. For instance, S determines if stall has occurred (if $S = 1$, stall has occurred), and P determines how light that stall was (if $P \approx 1$, stall was very light, if $P \approx 0$, stall was deep), given as

$$\begin{aligned} S&= {\left\{ \begin{array}{ll} 1 &{}\text{ if } \vert \theta _{max}\vert \ge 1 \text{ and } q{\bar{\alpha }}^{\prime } < 0 \text{(downstroke) } \\ 0 &{}\text{ otherwise } \end{array}\right. } \end{aligned}$$

(33)

$$\begin{aligned} P&= {\left\{ \begin{array}{ll} \exp {\left[ -\gamma _{LS}\left( {\theta _{max}}^4-1\right) \right] } &{}\text{ if } \vert \theta _{max}\vert \ge 1 \text{ and } q{\bar{\alpha }}^{\prime } < 0 \\ \\ 0 &{} \text{ otherwise } \end{array}\right. } \end{aligned}$$

(34)

where $\gamma _{LS}$ is a Mach-dependent constant, and $\theta _{max}$ is the maximum previously attained value of $\theta$. An important caveat to be noticed is that $\theta _{max}$ may be attained considerably after the end of the upstroke, that is, during the downstroke, since it is a function of the lagged effective angle of attack, ${\bar{\alpha }}^{\prime }$. Since the P parameter affects the response on the downstroke, the value of $\theta _{max}$ must be updated at every time-step as soon as it reaches a value above 1 and until it begins to decrease. Also, $\theta _{max}$ must be reset to zero whenever $\theta$ crosses zero. Then, the offsets of the breakpoint angles can be written as

$$\begin{aligned} \varDelta \alpha _{1_n}&= {\left\{ \begin{array}{ll} R^{\prime }_\theta (\alpha _{ds_0}-\alpha _{ss}) &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0\\ -S\left[ \delta \alpha _0 + \delta \alpha _1 \frac{r}{r_0}\left( 1+2P\sqrt{R^{\prime }}\right) \right] &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(35)

$$\begin{aligned} \varDelta \alpha _{1_m}&= {\left\{ \begin{array}{ll} \varDelta \alpha _{1_n} + d_m &{}\text{ if } q\alpha ^{\prime } > 0 \\ \varDelta \alpha _{1_n}\left( 1-z_mR^2\right) + d_m(1-R^{\prime }_\theta )\\ +S\delta \alpha _0(1-R^{\prime }_\theta ) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(36)

$$\begin{aligned} \varDelta \alpha _{1_c}&= {\left\{ \begin{array}{ll} \varDelta \alpha _{1_n}z_c + d_c\left( 1-R^{\prime }_\theta \right) &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \\ \varDelta \alpha _{1_n}\left[ 1+\left( 1-R^{\prime }_\theta \right) \left( 1-P\right) \right] \\ + d_c(1-R^{\prime }_\theta )+S\delta \alpha _0(1-R^{\prime }_\theta ) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(37)

where $\delta \alpha _0$, $\delta \alpha _1$, $d_m$, $d_c$, $z_m$ and $z_c$ are Mach-dependent constants.

Still referring to Eqs. 16 to 18, the $S_1^{\prime }$ and $S_2^{\prime }$ coefficients are

$$\begin{aligned}&S_{1_n}^{\prime } = {\left\{ \begin{array}{ll} S_1 &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \text{(upstroke) } \\ S_1\left( 1+R^2\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \text{(downstroke) } \end{array}\right. } \end{aligned}$$

(38)

$$\begin{aligned}&S_{2_n}^{\prime } = {\left\{ \begin{array}{ll} S_2\left[ 1+(1-R^{\prime ^{1/2}})/3+5\sigma _2\left( R^{\prime }-R^{\prime ^2}\right) \right] &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \\ S_2\left[ 1+(1-R^{\prime ^{1/2}})/3+5\sigma _2\left( R^{\prime }-R^{\prime ^2}\right) +5\sigma _1\right] &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(39)

$$\begin{aligned}&S_{1_m}^{\prime } = S_1\left( 1-R^{\prime ^2}/2\right) \end{aligned}$$

(40)

$$\begin{aligned}&S_{2_m}^{\prime } = {\left\{ \begin{array}{ll} S_2\left( 1+R^{\prime }\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \\ S_2\left( 1+2R^{\prime }\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(41)

$$\begin{aligned}&S_{1_c}^{\prime } = {\left\{ \begin{array}{ll} S_1\left( 1-3R^{\prime }/4\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \\ S_1\left( 1+R^{\prime }\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(42)

$$\begin{aligned}&S_{2_c}^{\prime } = {\left\{ \begin{array}{ll} S_2\left( 1+2R^{2}\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \\ S_2\left( 1+2R^{2}+2\sigma _1R^{\prime ^{1/4}}\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(43)

where $\sigma _1$ and $\sigma _2$ are given as

$$\begin{aligned} \sigma _1&= {\left\{ \begin{array}{ll} P^3(1-T) &{}\text{ if } \vert \theta _{max}\vert \ge 1 \\ 0 &{}\text{ otherwise } \end{array}\right. } \end{aligned}$$

(44)

$$\begin{aligned} \sigma _2&= {\left\{ \begin{array}{ll} \min \left( \left[ {\theta _{min}}^{5/2}; \;\; 1/2 \right] \right) &{}\text{ if } {{\,\mathrm{sgn}\,}}{\left( {\bar{\alpha }}^{\prime }\theta _{min}\right) } = 1 \\ 0 &{}\text{ otherwise } \end{array}\right. } \end{aligned}$$

(45)

in which $\theta _{min}$ is the minimum previously attained value of $\theta$, analogous to $\theta _{max}$. Furthermore, the totally separated flow points are:

$$\begin{aligned} f_{0_n} =&\; f_0 + \frac{1}{4}\sigma _2\left\{ \frac{1}{2}\left[ 1-\cos {\left( 2\pi {R^{\prime }_{tv}}\right) }\right] +\frac{1-{R^{\prime }_{tv}}^{2}}{5}\right\} \end{aligned}$$

(46)

$$\begin{aligned} f_{0_m} =&\; f_0 + \frac{1}{4}\sigma _2\left\{ \frac{1}{2}\left[ 1-\cos {\left( 2\pi {R^{\prime }_{tv}}\right) }\right] +1-{R^{\prime }_{tv}}^{2}\right\} \end{aligned}$$

(47)

$$\begin{aligned} f_{0_c} =&\; f_0 + \frac{1}{4}\sigma _2\left\{ \left[ 1-\cos {\left( 2\pi {R^{\prime }_{tv}}\right) }\right] +1-{R^{\prime }_{tv}}^{2}\right\} \end{aligned}$$

(48)

At last, the time delay variables are:

$$\begin{aligned} T_{f_n}&= {\left\{ \begin{array}{ll} T_{f_0} &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \\ T_{f_0}\left[ 1+S\left( R^{\prime ^{-1/4}}-R^{1/4}\right) \right] &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(49)

$$\begin{aligned} T_{f_m}&= {\left\{ \begin{array}{ll} T_{f_0} &{}\text{ if } q{\bar{\alpha }}^{\prime } > 0 \\ T_{f_n}/(4-2P) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \end{array}\right. } \end{aligned}$$

(50)

$$\begin{aligned} T_{f_c}&= {\left\{ \begin{array}{ll} T_{f_0}/2 &{}\text{ if } q{\bar{\alpha }}^{\prime }> 0 \text{ and } \vert \theta \vert< 1 \\ T_{f_0}\theta ^2/2 &{}\text{ if } q{\bar{\alpha }}^{\prime }> 0 \text{ and } \vert \theta \vert> 1 \\ T_{f_0}\left( 1/2+R\right) &{}\text{ if } q{\bar{\alpha }}^{\prime }< 0 \text{ and } \vert \theta \vert< 1 \\ T_{f_0}\left( \theta ^2/2+R\right) &{}\text{ if } q{\bar{\alpha }}^{\prime } < 0 \text{ and } \vert \theta \vert > 1 \end{array}\right. } \end{aligned}$$

(51)

where $T_{f_0}$ is a Mach-dependent constant time delay. The factor $\zeta _c$ in Eq. 24 is:

$$\begin{aligned} \zeta _c = \min \left( \left[ {R^{\prime }}^{-1}; \;\; 20 \right] \right) \end{aligned}$$

(52)