Nonlinear forced vibration analysis of the composite shaft-disk system combined the reduced-order model with the IHB method

Abstract

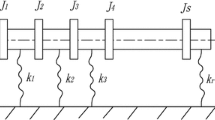
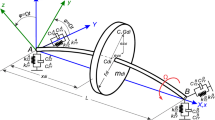
In this paper, the internal resonance phenomena of a composite shaft-disk system with multi-degrees-of-freedom are analyzed. The force caused by the unbalanced mass of the disk is considered as an external excitation force. The shaft is simply supported. Shear deformation and gyroscopic effects are considered. The strain–displacement relationship of the shaft element is expressed using the Timoshenko beam theory. Each node has 5 degrees of freedom. SHBT (simplified homogenized beam theory) is applied to calculate the stiffness of the composite shaft. WQEM (weak form quadrature element method) is used to construct the element matrices, and the system matrices are established using the element matrix assembly rule of the FEM (finite element method). The reduced-order model is applied to reduce the calculation time. IHB (incremental harmonic balance) method is utilized to solve the nonlinear equations of motion of the composite shaft-disk system. The nonlinear vibration characteristics of the Jeffcott rotor are analyzed using the proposed method and compared with the results of previous researches, and the results are very similar. Based on these considerations, the nonlinear vibration phenomena of the composite shaft-disk system with multi-degrees-of-freedom are considered at the several resonance points.

Appendix

The every element matrix in Eq. (31) is expressed as follows.

The mass matrix is as follows.

$$ {\mathbf{M}}^{e} = {\mathbf{M}}_{d}^{e} + {\mathbf{M}}_{s}^{e} $$

(60)

Here, ${\mathbf{M}}_{d}^{e}$ is the mass matrix of the disk and expressed as follows.

$$ {\mathbf{M}}_{d}^{e} = \left[ {\begin{array}{*{20}c} {\tilde{M}_{di} } & {0} & {0} & {0} & {0} \\ {0} & {\tilde{M}_{di} } & {0} & {0} & {0} \\ {0} & {0} & {\tilde{M}_{di} } & {0} & {0} \\ {0} & {0} & {0} & {\tilde{I}_{di} } & {0} \\ {0} & {0} & {0} & {0} & {\tilde{I}_{di} } \\ \end{array} } \right] $$

(61)

${\mathbf{M}}_{s}^{e}$ is the mass matrix of shaft element and expressed as follows.

$$ {\mathbf{M}}_{s}^{e} = \left[ {\begin{array}{*{20}c} {{\mathbf{C}}^{\left( 1 \right)} } & {0} & {0} & {0} & {0} \\ {0} & {{\mathbf{C}}^{\left( 1 \right)} } & {0} & {0} & {0} \\ {0} & {0} & {{\mathbf{C}}^{\left( 1 \right)} } & {0} & {0} \\ {0} & {0} & {0} & {\tilde{I}_{1} {\mathbf{C}}^{\left( 1 \right)} } & {0} \\ {0} & {0} & {0} & {0} & {\tilde{I}_{1} {\mathbf{C}}^{\left( 1 \right)} } \\ \end{array} } \right] $$

(62)

The gyroscopic matrix is as follows.

$$ {\mathbf{G}}^{e} = {\mathbf{G}}_{d}^{e} + {\mathbf{G}}_{s}^{e} $$

(63)

Here, ${\mathbf{G}}_{d}^{e}$ is the gyroscopic matrix of the disk and expressed as follows.

$$ {\mathbf{G}}_{d}^{e} = \left[ {\begin{array}{*{20}c} {0} & {0} & {0} & {0} & {0} \\ {0} & {0} & {0} & {0} & {0} \\ {0} & {0} & {0} & {0} & {0} \\ {0} & {0} & {0} & {0} & { - \tilde{I}_{dpi} } \\ {0} & {0} & {0} & {\tilde{I}_{dpi} } & {0} \\ \end{array} } \right] $$

(64)

${\mathbf{G}}_{s}^{e}$ is the gyroscopic matrix of shaft element and expressed as follows.

$$ {\mathbf{G}}_{s}^{e} = \left[ {\begin{array}{*{20}c} {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} \\ {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} \\ {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} \\ {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & { - \tilde{I}_{2} {\mathbf{C}}^{\left( 1 \right)} } \\ {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\tilde{I}_{2} {\mathbf{C}}^{\left( 1 \right)} } & {\mathbf{0}} \\ \end{array} } \right] $$

(65)

The stiffness matrix of shaft element is as follows.

$$ {\mathbf{K}}_{s}^{e} = \left[ {\begin{array}{*{20}c} {\tilde{E}_{{eqs}} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{A}}^{{\left( 1 \right)}} } & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} \\ {\mathbf{0}} & {\tilde{G}_{{eq}} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{A}}^{{\left( 1 \right)}} } & {\mathbf{0}} & {\mathbf{0}} & { - \tilde{G}_{{eq}} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{I}}} \\ {\mathbf{0}} & {\mathbf{0}} & {\tilde{G}_{{eq}} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{A}}^{{\left( 1 \right)}} } & {\tilde{G}_{{eq}} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{I}}} & {\mathbf{0}} \\ {\mathbf{0}} & {\mathbf{0}} & {\tilde{G}_{{eq}} {\mathbf{I}}^{T} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{A}}^{{\left( 1 \right)}} } & {\tilde{E}_{{eq}} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{A}}^{{\left( 1 \right)}} } & {\mathbf{0}} \\ {\mathbf{0}} & { - \tilde{G}_{{eq}} {\mathbf{I}}^{T} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{A}}^{{\left( 1 \right)}} } & {\mathbf{0}} & {\mathbf{0}} & {\tilde{E}_{{eq}} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} {\mathbf{A}}^{{\left( 1 \right)}} } \\ \end{array} } \right] $$

(66)

The nonlinear stiffness matrix of shaft element is as follows.

$$ {\mathbf{K}}_{3}^{e} {\text{ = }}\frac{{\tilde{E}_{{eqs}} }}{{\text{2}}}\left[ {\begin{array}{*{20}c} {\mathbf{0}} & {\psi {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{v}}}} \right){\mathbf{A}}^{{\left( 1 \right)}} } & {\psi {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{w}}}} \right){\mathbf{A}}^{{\left( 1 \right)}} } & {\mathbf{0}} & {\mathbf{0}} \\ {2\psi {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{v}}}} \right){\mathbf{A}}^{{\left( 1 \right)}} } & {\psi ^{2} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{v}}}} \right)^{2} {\mathbf{A}}^{{\left( 1 \right)}} } & {\psi ^{2} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{v}}}} \right)\left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{w}}}} \right){\mathbf{A}}^{{\left( 1 \right)}} } & {\mathbf{0}} & {\mathbf{0}} \\ {2\psi {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{v}}}} \right){\mathbf{A}}^{{\left( 1 \right)}} } & {\psi ^{2} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{w}}}} \right)\left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{v}}}} \right){\mathbf{A}}^{{\left( 1 \right)}} } & {\psi ^{2} {\mathbf{A}}^{{\left( 1 \right)T}} {\mathbf{C}}^{{\left( 1 \right)}} \left( {{\mathbf{A}}^{{\left( 1 \right)}} {\mathbf{\tilde{w}}}} \right)^{2} {\mathbf{A}}^{{\left( 1 \right)}} } & {\mathbf{0}} & {\mathbf{0}} \\ {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} \\ {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} & {\mathbf{0}} \\ \end{array} } \right] $$

(67)

Here, 0 and I are zero matrix and identity matrix, respectively.