Skip to main content
SpringerLink
Log in

Bifurcation analysis of a diffusive predator–prey model with ratio-dependent Holling type III functional response

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

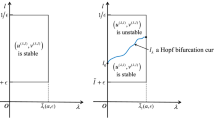
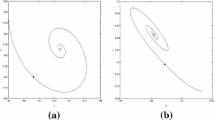
The spatial, temporal, and spatiotemporal dynamics of a reaction–diffusion predator–prey system with ratio-dependent Holling type III functional response, under homogeneous Neumann boundary conditions, are studied in this paper. Preliminary analysis on the local asymptotic stability and Hopf bifurcation of the spatially homogeneous model based on ordinary differential equation is presented. For the reaction–diffusion model, firstly the parameter regions for the stability or instability of the unique constant steady state are discussed. Then it is shown that Turing (diffusion-driven) instability occurs, which induces spatial inhomogeneous patterns. Next, it is proved that the model exhibits Hopf bifurcation, which produces temporal inhomogeneous patterns. Finally, the existence and nonexistence of nonconstant steady- state solutions are established by bifurcation method and energy method, respectively. Numerical simulations are presented to verify and illustrate the theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Camara, B.I., Aziz-Alaoui, M.A.: Turing and Hopf patterns formation in a predator–prey model with Leslie–Gower-type functional response. Dynam. Cont. Discrete Ser. B 16, 479–488 (2009)

    MathSciNet  MATH  Google Scholar 

  2. Cantrell, R.S., Cosner, C.: Spatial Ecology Via Reaction–Diffusion Equations. Wiley, Hoboken (2004)

  3. Casal, A., Eilbeck, J.C., López-Gómez, J., et al.: Existence and uniqueness of coexistence states for a predator–prey model with diffusion. Differ. Integral Equ. 7, 411–439 (1994)

    MATH  Google Scholar 

  4. Catllá, A.J., McNamara, A., Topaz, C.M.: Instabilities and patterns in coupled reaction–diffusion layers. Phys. Rev. E 85, 026215 (2012)

    Article  Google Scholar 

  5. Davidson, F.A., Rynne, B.P.: A priori bounds and global existence of solutions of the steady-state Sel’kov model. Proc. R. Soc. Edinb. A 130, 507–516 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  6. Doelman, A., Kaper, T.J., Zegeling, P.A.: Pattern formation in the one-dimensional Gray–Scott model. Nonlinearity 10, 523–563 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  7. Du, Y., Lou, Y.: Some uniqueness and exact multiplicity results for a predator–prey model. Trans. Am. Math. Soc. 349, 2443–2475 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  8. Du, Y., Lou, Y.: S-shaped global bifurcation curve and Hopf bifurcation of positive solutions to a predator–prey model. J. Differ. Equ. 144, 390–440 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  9. Du, Y., Shi, J.P.: Some recent results on diffusive predator–prey models in spatially heterogeneous environment. Nonlinear Dyn. Evol. Equ. Fields Inst. Commun. 48, 95–135 (2006)

    MathSciNet  Google Scholar 

  10. Golovin, A.A., Matkowsky, B.J., Volpert, V.A.: Turing pattern formation in the Brusselator model with superdiffusion. SIAM J. Appl. Math. 69, 251–272 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  11. Guo, G.H., Li, B.F., Wei, M.H., Wu, J.H.: Hopf bifurcation and steady-state bifurcation for an autocatalysis reaction–diffusion model. J. Math. Anal. Appl. 391, 265–277 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  12. Hale, J.K., Peletier, L.A., Troy, W.C.: Stability and instability in the Gray–Scott model: the case of equal diffusivities. Appl. Math. Lett. 12, 59–65 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  13. Hassard, B.D., Kazarinoff, N.D., Wan, Y.H.: Theory and applications of Hopf bifurcation, vol. 2. Cambridge University Press, London (1981)

  14. Iron, D., Wei, J.C., Winter, M.: Stability analysis of Turing patterns generated by the Schnakenberg model. J. Math. Biol. 49, 358–390 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  15. Jang, J., Ni, W.M., Tang, M.X.: Global bifurcation and structure of Turing patterns in the 1-D Lengyel–Epstein model. J. Dyn. Differ. Equ. 16, 297–320 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  16. Jin, J.Y., Shi, J.P., Wei, J.J., Yi, F.Q.: Bifurcations of patterned solutions in diffusive Lengyel–Epstein system of CIMA chemical reaction. Rocky Mount. J. Math. 43, 1637–1674 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  17. Kolokolnikov, T., Erneux, T., Wei, J.: Mesa-type patterns in the one-dimensional Brusselator and their stability. Phys. D 214, 63–77 (2006)

  18. Levin, S.A.: Dispersion and population interactions. Am. Nat. 108, 207–228 (1974)

    Article  Google Scholar 

  19. Levin, S.A., Segel, L.A.: Pattern generation in space and aspect. SIAM Rev. 27, 45–67 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  20. Li, L.: Coexistence theorems of steady states for predator–prey interacting systems. Trans. Am. Math. Soc. 305, 143–166 (1988)

    Article  MATH  Google Scholar 

  21. Li, X., Jiang, W.H., Shi, J.P.: Hopf bifurcation and Turing instability in the reaction–diffusion Holling–Tanner predator–prey model. IMA J. Appl. Math. 78, 287–306 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  22. López-Gómez, J., Molina-Meyer, M.: Bounded components of positive solutions of abstract fixed point equations: mushrooms, loops and isolas. J. Differ. Equ. 209, 416–441 (2005)

    Article  MATH  Google Scholar 

  23. Malchow, H., Petrovskii, S.V., Venturino, E.: Spatiotemporal Patterns in Ecology and Epidemiology: Theory, Models, and Simulation. Chapman & Hall/CRC Press, London (2008)

    Google Scholar 

  24. McGough, J.S., Riley, K.: Pattern formation in the Gray–Scott model. Nonlinear Anal. Real World Appl. 5, 105–121 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  25. Medvinsky, A.B., Petrovskii, S.V., Tikhonova, I.A., Malchow, H., Li, B.L.: Spatiotemporal complexity of plankton and fish dynamics. SIAM Rev. 44, 311–370 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  26. Muratori, S., Rinaldi, S.: Remarks on competitive coexistence. SIAM J. Appl. Math. 49, 1462–1472 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  27. Ni, W.M., Tang, M.X.: Turing patterns in the Lengyel–Epstein system for the CIMA reaction. Trans. Am. Math. Soc. 357, 3953–3969 (2005). (electronic)

    Article  MathSciNet  MATH  Google Scholar 

  28. Owen, M.R., Lewis, M.A.: How predation can slow, stop or reverse a prey invasion. Bull. Math. Biol. 63, 655–684 (2001)

    Article  Google Scholar 

  29. Peng, R.: Qualitative analysis of steady states to the Sel’kov model. J. Differ. Equ. 241, 386–398 (2007)

    Article  MATH  Google Scholar 

  30. Peng, R., Shi, J.P.: Non-existence of non-constant positive steady states of two Holling type-II predator–prey systems: strong interaction case. J. Differ. Equ. 247, 866–886 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  31. Peng, R., Shi, J.P., Wang, M.X.: On stationary patterns of a reaction–diffusion model with autocatalysis and saturation law. Nonlinearity 21, 1471–1488 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  32. Peng, R., Sun, F.Q.: Turing pattern of the Oregonator model. Nonlinear Anal. 72, 2337–2345 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  33. Peng, R., Wang, M.X., Yang, M.: Positive steady-state solutions of the Sel’kov model. Math. Comput. Model. 44, 945–951 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  34. Sambath, M., Balachandran, K.: Bifurcations in a diffusive predator-prey model with predator saturation and competition response. Math. Methods Appl. Sci. 38, 785–798 (2015)

    Article  MathSciNet  Google Scholar 

  35. Sambath, M., Gnanavel, S., Balachandran, K.: Stability and Hopf bifurcation of a diffusive predator–prey model with predator saturation and competition. Appl. Anal. 92, 2439–2456 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  36. Schnakenberg, J.: Simple chemical reaction systems with limit cycle behaviour. J. Theor. Biol. 81, 389–400 (1979)

    Article  MathSciNet  Google Scholar 

  37. Segel, L.A., Jackson, J.L.: Dissipative structure: an explanation and an ecological example. J. Theor. Biol. 37, 545–559 (1972)

    Article  Google Scholar 

  38. Sherratt, J.A., Eagan, B.T., Lewis, M.A.: Oscillations and chaos behind predator–prey invasion: Mathematical artifact or ecological reality? Philos. Trans. R. Soc. B 352, 21–38 (1997)

    Article  Google Scholar 

  39. Shi, H.B., Yan, L.: Global asymptotic stability of a diffusive predator–prey model with ratio-dependent functional response. Appl. Math. Comput. 250, 71–77 (2015)

    Article  MathSciNet  Google Scholar 

  40. Shi, J.P., Wang, X.F.: On global bifurcation for quasilinear elliptic systems on bounded domains. J. Differ. Equ. 246, 2788–2812 (2009)

    Article  MATH  Google Scholar 

  41. Song, Y.L., Zou, X.F.: Bifurcation analysis of a diffusive ratio-dependent predator–prey model. Nonlinear Dyn. 78, 49–70 (2014)

    Article  MathSciNet  Google Scholar 

  42. Turing, A.M.: The chemical basis of morphogenesis. Philos. Trans. R. Soc. B 237, 37–72 (1952)

    Article  Google Scholar 

  43. Tyson, J.J., Chen, K., Novak, B., et al.: Network dynamics and cell physiology. Nat. Rev. Mol. Cell Biol. 2, 908–916 (2001)

    Article  Google Scholar 

  44. Wang, J.F., Shi, J.P., Wei, J.J.: Dynamics and pattern formation in a diffusive predator–prey system with strong Allee effect in prey. J. Differ. Equ. 251, 1276–1304 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  45. Wang, M.X.: Non-constant positive steady states of the Sel’kov model. J. Differ. Equ. 190, 600–620 (2003)

    Article  MATH  Google Scholar 

  46. Wang, X.C., Wei, J.J.: Diffusion-driven stability and bifurcation in a predator–prey system with Ivlev-type functional response. Appl. Anal. 92, 752–775 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  47. Ward, M.J., Wei, J.C.: The existence and stability of asymmetric spike patterns for the Schnakenberg model. Stud. Appl. Math. 109, 229–264 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  48. Wei, J.C.: Pattern formations in two-dimensional Gray–Scott model: existence of single-spot solutions and their stability. Phys. D 148, 20–48 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  49. Wei, J.C., Winter, M.: Flow-distributed spikes for Schnakenberg kinetics. J. Math. Biol. 64, 211–254 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  50. Wiggins, S., Golubitsky, M.: Introduction to Applied Nonlinear Dynamical Systems and Chaos, vol. 2. Springer, Berlin (1990)

    MATH  Google Scholar 

  51. Wollkind, D.J., Collings, J.B., Barba, M.C.B.: Diffusive instabilities in a one-dimensional temperature-dependent model system for a mite predator–prey interaction on fruit trees: dispersal motility and aggregative preytaxis effects. J. Math. Biol. 29, 339–362 (1991)

  52. Xu, L., Zhang, G., Ren, J.F.: Turing instability for a two dimensional semi-discrete Oregonator model. WSEAS Trans. Math. 10, 201–209 (2011)

    Google Scholar 

  53. Yi, F.Q., Wei, J.J., Junping Shi, J.P.: Diffusion-driven instability and bifurcation in the Lengyel–Epstein system. Nonlinear Anal. Real World Appl. 9, 1038–1051 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  54. Yi, F.Q., Wei, J.J., Junping Shi, J.P.: Bifurcation and spatiotemporal patterns in a homogeneous diffusive predator–prey system. J. Differ. Equ. 246, 1944–1977 (2009)

    Article  MATH  Google Scholar 

  55. Yi, F.Q., Wei, J.J., Junping Shi, J.P.: Global asymptotical behavior of the Lengyel–Epstein reaction–diffusion system. Appl. Math. Lett. 22, 52–55 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  56. You, Y.C.: Global dynamics of the Oregonator system. Math. Methods Appl. Sci. 35, 398–416 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  57. Zhou, J., Mu, C.L.: Pattern formation of a coupled two-cell Brusselator model. J. Math. Anal. Appl. 366, 679–693 (2010)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Southwest University, Chongqing, 400715, People’s Republic of China

    Jun Zhou

Authors
  1. Jun Zhou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jun Zhou.

Additional information

This work is partially supported by NSFC Grant 11201380, Project funded by China Postdoctoral Science Foundation Grant 2014M550453 and the Second Foundation for Young Teachers in Universities of Chongqing.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhou, J. Bifurcation analysis of a diffusive predator–prey model with ratio-dependent Holling type III functional response. Nonlinear Dyn 81, 1535–1552 (2015). https://doi.org/10.1007/s11071-015-2088-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-015-2088-z

Keywords

Search

Navigation