Skip to main content
SpringerLink
Log in

A Hamiltonian system of three degrees of freedom with eight channels of escape: The Great Escape

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

In this work, we try to shed some light to the nature of orbits in a three-dimensional (3D) potential of a perturbed harmonic oscillator with eight possible channels of escape, which was chosen as an interesting example of open 3D Hamiltonian systems. In particular, we conduct a thorough numerical investigation distinguishing between regular and chaotic orbits as well as between trapped and escaping orbits, considering unbounded motion for several values of the energy. In an attempt to discriminate safely and with certainty between ordered and chaotic motion, we use the Smaller ALingment Index (SALI) detector, computed by integrating numerically the basic equations of motion as well as the variational equations. Of particular interest is to locate the basins of escape toward the different escape channels and connect them with the corresponding escape periods of the orbits. We split our study into three different cases depending on the initial value of the \(z\) coordinate which was used for launching the test particles. We found that when the orbits are started very close to the primary \((x,y)\) plane the respective grids exhibit a high degree of fractalization, while on the other hand for orbits with relatively high values of \(z_0\) several well-formed basins of escape emerge thus reducing significantly the fractalization of the grids. It was also observed that for values of energy very close to the escape energy the escape times of orbits are large, while for energy levels much higher than the escape energy the vast majority of orbits escape extremely fast or even immediately to infinity. We hope our outcomes to be useful for a further understanding of the escape process in open 3D Hamiltonian systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28
Fig. 29
Fig. 30

Similar content being viewed by others

Notes

  1. Generally, any dynamical method requires a sufficient time interval of numerical integration in order to distinguish safely between ordered and chaotic motion. Therefore, if the escape rate of orbits is very low or even worse if the orbits escape directly from the system then, any chaos indicator (the SALI in our case) will fail to work properly due to insufficient integration time. Nevertheless, we decided to apply the SALI method regardless of the escape rate of orbits.

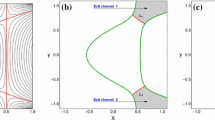
  2. The term “escape positions” refers to the \((x,y,z)\) points of the 3D space at which the orbits intersect the cutoff surface with velocity pointing outwards and escape.

  3. The stability of a periodic orbit is determined by computing the stability index (S.I.) [58]. A periodic orbit is stable if only the S.I. is between \(-2\) and \(+\)2.

References

  1. Aguirre, J., Sanjuán, M.A.F.: Limit of small exits in open Hamiltonian systems. Phys. Rev. E 67, 056201-1–056201-7 (2003)

    Article  Google Scholar 

  2. Aguirre, J., Vallejo, J.C., Sanjuán, M.A.F.: Wada basins and chaotic invariant sets in the Hénon–Heiles system. Phys. Rev. E 64, 066208-1–066208-11 (2001)

    Google Scholar 

  3. Aguirre, J., Vallejo, J.C., Sanjuán, M.A.F.: Wada basins and unpredictability in Hamiltonian and dissipative systems. Int. J. Mod. Phys. B 17, 4171–4175 (2003)

    Google Scholar 

  4. Arribas, M., Elipe, A., Floria, L., Riaguas, A.: Oscillators in resonance \(p{:}q{:}r\). Chaos Solitons Fractals 27, 1220–1228 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  5. Barrio, R., Blesa, F., Serrano, S.: Fractal structures in the Hénon–Heiles Hamiltonian. Europhys. Lett. 82, 10003- 1–10003-6 (2008)

    Google Scholar 

  6. Barrio, R., Blesa, F., Serrano, S.: Bifurcations and safe regions in open Hamiltonians. New J. Phys. 11, 053004-1–053004-12 (2009)

    Article  Google Scholar 

  7. Benet, L., Trautman, D., Seligman, T.: Chaotic scattering in the restricted three-body problem. I. The Copenhagen problem. Celest. Mech. Dyn. Astron. 66, 203–228 (1996)

    Article  Google Scholar 

  8. Benet, L., Seligman, T., Trautman, D.: Chaotic scattering in the restricted three-body problem. II. Small mass parameters. Celest. Mech. Dyn. Astron. 71, 167–189 (1998)

    Article  Google Scholar 

  9. Bleher, S., Grebogi, C., Ott, E., Brown, R.: Fractal boundaries for exit in Hamiltonian dynamics. Phys. Rev. A 38, 930–938 (1988)

    Article  MathSciNet  Google Scholar 

  10. Blesa, F., Seoane, J.M., Barrio, R., Sanjuán, M.A.F.: To escape or not to escape, that is the question—perturbing the Hénon–Heiles Hamiltonian. Int. J. Bifurcation Chaos 22, 1230010-1–1230010-9 (2012)

    Article  Google Scholar 

  11. Caranicolas, N.D.: The 1:1 resonance in galactic type Hamiltonian systems. Astron. Astrophys. 267, 388–391 (1993)

    Google Scholar 

  12. Caranicolas, N.D., Barbanis, B.: Periodic orbits in nearly axisymmetric stellar systems. Astron. Astrophys. 114, 360–366 (1982)

    MATH  MathSciNet  Google Scholar 

  13. Caranicolas, N.D., Karanis, G.I.: Chaos in barred galaxy models. Astrophys. Space Sci. 259, 45–56 (1998)

    Article  MATH  Google Scholar 

  14. Caranicolas, N.D., Karanis, G.I.: Motion in a potential creating a weak bar structure. Astron. Astrophys. 342, 389–394 (1999)

    Google Scholar 

  15. Caranicolas, N.D., Zotos, E.E.: Investigating the nature of motion in 3D perturbed elliptic oscillators displaying exact periodic orbits. Nonlinear Dyn. 69, 1795–1805 (2012)

    Article  MathSciNet  Google Scholar 

  16. Churchill, R., Pecelli, G., Rod, D.: Isolated unstable periodic orbits. J. Differ. Equ. 17, 329–348 (1975)

    Article  MATH  MathSciNet  Google Scholar 

  17. Contopoulos, G.: Asymptotic curves and escapes in Hamiltonian systems. Astron. Astrophys. 231, 41–55 (1990)

    MathSciNet  Google Scholar 

  18. Contopoulos, G.: Order and Chaos in Dynamical Astronomy. Springer, Berlin (2002)

    Book  MATH  Google Scholar 

  19. Contopoulos, G., Efstathiou, K.: Escapes and recurrence in a simple Hamiltonian system. Celest. Mech. Dyn. Astron. 88, 163–183 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  20. Contopoulos, G., Kaufmann, D.: Types of escapes in a simple Hamiltonian system. Astron. Astrophys. 253, 379–388 (1992)

    MATH  MathSciNet  Google Scholar 

  21. Contopoulos, G., Kandrup, H.E., Kaufmann, D.: Fractal properties of escape from a two-dimensional potential. Phys. D 64, 310–323 (1993)

    Article  MATH  Google Scholar 

  22. Contopoulos, G., Harsoula, M., Lukes-Gerakopoulos, G.: Periodic orbits and escapes in dynamical systems. Celest. Mech. Dyn. Astron. 113, 255–278 (2012)

    Article  MathSciNet  Google Scholar 

  23. de Moura, A.P.S., Letelier, P.S.: Fractal basins in Hénon–Heiles and other polynomial potentials. Phys. Lett. A 256, 362–368 (1999)

    Article  MathSciNet  Google Scholar 

  24. Deprit, A.: The Lissajous transformation. I. Basics. Celest. Mech. Dyn. Astron. 51, 202–225 (1991)

    Google Scholar 

  25. Deprit, A., Elipe, A.: The Lissajous transformation. II. Normalization. Celest. Mech. Dyn. Astron. 51, 227–250 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  26. Eckhardt, B.: Irregular scattering. Phys. D 33, 89–98 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  27. Elipe, A.: Complete reduction of oscillators in resonance \(p{:}q\). Phys. Rev. E 61, 6477–6484 (2000)

    Article  Google Scholar 

  28. Elipe, A., Deprit, A.: Oscillators in resonance. Mech. Res. Commun. 26, 635–640 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  29. Ernst, A., Just, A., Spurzem, R., Porth, O.: Escape from the vicinity of fractal basin boundaries of a star cluster. Mon. Notices R. Astron. Soc. 383, 897–906 (2008)

    Article  Google Scholar 

  30. Ferrer, S., Lara, M., Palacián, J., Juan, J.S., Viartola, A., Yanguas, P.: The Hénon–Heiles problem in three dimensions. I. Periodic orbits near the origin. Int. J. Bifurcation Chaos 8, 1199–1213 (1998)

    Article  MATH  Google Scholar 

  31. Ferrer, S., Lara, M., Palacián, J., Juan, J.S., Viartola, A., Yanguas, P.: The Hénon–Heiles problem in three dimensions. II. Relative equilibria and bifurcations in the reduced problem. Int. J. Bifurcation Chaos 8, 1215–1229 (1998)

    Article  MATH  Google Scholar 

  32. Giorgilli, A., Galgani, L.: From integrals from an autonomous Hamiltonian system near an equilibrium point. Celest. Mech. 17, 267–280 (1978)

    Article  MATH  MathSciNet  Google Scholar 

  33. Hénon, M., Heiles, C.: The applicability of the third integral of motion: some numerical experiments. Astron. J. 69, 73–79 (1964)

    Google Scholar 

  34. Jung, C., Scholz, H.: Cantor set structures in the singularities of classical potential scattering. J. Phys. A 21, 3607–3617 (1988)

    Article  MathSciNet  Google Scholar 

  35. Kandrup, H.E., Siopis, C., Contopoulos, G., Dvorak, R.: Diffusion and scaling in escapes from two-degrees-of-freedom Hamiltonian systems. Chaos 9, 381–392 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  36. Karanis, G.I., Vozikis, ChL: Fast detection of chaotic behavior in galactic potentials. Astron. Nachr. 329, 403–412 (2007)

    Article  Google Scholar 

  37. Kennedy, J., Yorke, J.A.: Basins of Wada. Phys. D 51, 213–225 (1991)

    Google Scholar 

  38. Motter, A.E., Lai, Y.C.: Dissipative chaotic scattering. Phys. Rev. E 65, R015205-1–R015205-4 (2002)

    Google Scholar 

  39. Ott, E., Tél, T.: Chaotic scattering: an introduction. Chaos 3, 417–426 (1993)

    Article  Google Scholar 

  40. Petit, J.-M., Hénon, M.: Satellite encounters. Icarus 66, 536–555 (1986)

    Article  Google Scholar 

  41. Poon, L., Campos, J., Ott, E., Grebogi, C.: Wada basins boundaries in chaotic scattering. Int. J. Bifurcation Chaos 6, 251–266 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  42. Press, H.P., Teukolsky, S.A., Vetterling, W.T., Flannery, B.P.: Numerical Recipes in FORTRAN 77, 2nd edn. Cambridge University Press, Cambridge (1992)

    Google Scholar 

  43. Saito, N., Ichimura, A.. In: Casati, G., Ford, J. (eds.) Stochastic Behavior in Classical and Quantum Hamiltonian Systems. Lecture Notes in Physics, vol. 93. Springer, Berlin (1979)

  44. Seoane, J.M.: Effects of weak perturbations in open Hamiltonian systems. PhD thesis, Universidad Rey Juan Carlos, Spain (2007)

  45. Seoane, J.M., Aguirre, J., Sanjuán, M.A.F., Lai, Y.C.: Basin topology in dissipative chaotic scattering. Chaos 16, 023101-1–023101-8 (2006)

    Article  Google Scholar 

  46. Seoane, J.M., Sanjuán, M.A.F., Lai, Y.C.: Fractal dimension in dissipative chaotic scattering. Phys. Rev. E 76, 016208-1–016208-6 (2007)

    Google Scholar 

  47. Seoane, J.M., Sanjuán, M.A.F.: Exponential decay and scaling laws in noisy chaotic scattering. Phys. Lett. A 372, 110–116 (2008)

    Google Scholar 

  48. Seoane, J.M., Huang, L., Sanjuán, M.A.F., Lai, Y.C.: Effects of noise on chaotic scattering. Phys. Rev. E 79, 047202-1–047202-4 (2009)

    Article  Google Scholar 

  49. Seoane, J.M., Sanjuán, M.A.F.: Escaping dynamics in the presence of dissipation and noisy in scattering systems. Int. J. Bifurcation Chaos 9, 2783–2793 (2010)

    Google Scholar 

  50. Siopis, C.V., Contopoulos, G., Kandrup, H.E.: Escape probabilities in a Hamiltonian with two channels of escape. N. Y. Acad. Sci. Ann. 751, 205–212 (1995)

    Article  Google Scholar 

  51. Siopis, C.V., Kandrup, H.E., Contopoulos, G., Dvorak, R.: Universal properties of escape. N. Y. Acad. Sci. Ann. 773, 221–230 (1995)

    Article  Google Scholar 

  52. Siopis, C.V., Kandrup, H.E., Contopoulos, G., Dvorak, R.: Universal properties of escape in dynamical systems. Celest. Mech. Dyn. Astron. 65, 57–681 (1996)

    Article  MathSciNet  Google Scholar 

  53. Skokos, C.: Alignment indices: a new, simple method for determining the ordered or chaotic nature of orbits. J. Phys. A Math. Gen. 34, 10029–10043 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  54. Skokos, Ch., Antonopoulos, Ch., Bountis, T.C., Vrahatis, M.N.: Detecting order and chaos in Hamiltonian systems by the SALI method. J. Phys. A Math. Gen. 37, 6269–6284 (2004)

    Article  MathSciNet  Google Scholar 

  55. Zotos, E.E.: Application of new dynamical spectra of orbits in Hamiltonian systems. Nonlinear Dyn. 69, 2041–2063 (2012)

    Article  MathSciNet  Google Scholar 

  56. Zotos, E.E.: Trapped and escaping orbits in axially symmetric galactic-type potential. PASA 29, 161–173 (2012)

    Google Scholar 

  57. Zotos, E.E.: The Fast Norm Vector Indicator (FNVI) method: a new dynamical parameter for detecting order and chaos in Hamiltonian systems. Nonlinear Dyn. 70, 951–978 (2012)

    Article  MathSciNet  Google Scholar 

  58. Zotos, E.E.: Revealing the evolution, the stability and the escapes of families of resonant periodic orbits in Hamiltonian systems. Nonlinear Dyn. 73, 931–962 (2013)

    Article  MathSciNet  Google Scholar 

  59. Zotos, E.E., Caranicolas, N.D.: Are semi-numerical methods an effective tool for locating periodic orbits in 3D potentials? Nonlinear Dyn. 70, 279–287 (2012)

    Article  MathSciNet  Google Scholar 

  60. Zotos, E.E., Caranicolas, N.D.: Order and chaos in a new 3D dynamical model describing motion in non-axially symmetric galaxies. Nonlinear Dyn. 74, 1203–1221 (2013)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgments

The author would like to thank the two anonymous referees for the careful reading of the manuscript and for all the apt suggestions and comments which allowed us to improve both the quality and the clarity of the paper.

Author information

Authors and Affiliations

  1. Department of Physics, School of Science, Aristotle University of Thessaloniki, 541 24 , Thessaloniki, Greece

    Euaggelos E. Zotos

Authors
  1. Euaggelos E. Zotos
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Euaggelos E. Zotos.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zotos, E.E. A Hamiltonian system of three degrees of freedom with eight channels of escape: The Great Escape. Nonlinear Dyn 76, 1301–1326 (2014). https://doi.org/10.1007/s11071-013-1211-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-013-1211-2

Keywords

Search

Navigation