Different dynamics of a periodic mass-in-mass nonlinear chain during a single mode excitation

Abstract

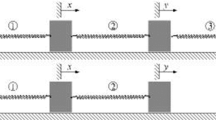
The multiple scale dynamics of a periodic chain composed of nonlinear mass-in-mass cells is studied. Based on a continuous approach of the one-dimensional chain, dispersion equation is obtained which provides the general form of solutions of the linearized system. Taking into account a single harmonic of the chain around a 1:1 resonance with a targeted mode, fast dynamics of the system leads to the detection of the slow invariant manifold and its stability borders. Slow dynamics permits to predict singularities and equilibrium points leading to frequency responses. The developments predict periodic and non-periodic responses and permit to tune parameters of the chain for the aim of localization of vibrating energy and design of periodic or non-periodic responses.

Appendices

Appendix 1

1.1 Continuous approach of the system with modal projection

Let us consider the eigenfunction of the system which is defined in Eq. 23. We normalize this function via

$$\begin{aligned} \begin{array}{lcc} \int _{0}^{L}\left( \tilde{\rho }_k\cos (\omega _k x + \varTheta _k)\right) ^2dx=1 \end{array} \end{aligned}$$

(51)

leading to $\tilde{\rho }_k=\sqrt{2/L}$. We define:

$$\begin{aligned} \left\{ \begin{array}{lcc} U(x,\tau )=\tilde{\rho }_{1,k}(\tau )\sqrt{\frac{2}{L}}\cos (\omega _kx+\varTheta _k)\\ \\ V(x,\tau )=\tilde{\rho }_{2,k}(\tau )\sqrt{\frac{2}{L}}\cos (\omega _kx+\varTheta _k) \end{array} \right. \end{aligned}$$

(52)

$\omega _k$ being defined in Eq. 20.

Fig. 11

Forced response of the cell 27 around the first mode ($k=1$). The SIM of the system (green line) accompanied by numerical results (red line). Black ‘+’ symbols present amplitudes of possible singularities of the system. Unstable zone of the SIM is represented in orange line. System is under external excitation with the amplitude of $f_j=0.03 \cos (\omega _1(j-1))$ and the frequency of $\nu =0.064$ with zero initial conditions. Parameters of the system are listed in configuration number 2 of Table 1. (Color figure online)

Fig. 12

Analytical predictions of equilibrium points of the continuous system (green line) for system under external excitation with the amplitude of $f_j=0.03 \cos (\omega _1(j-1))$ and the frequency of $\nu =0.064$. Unstable zone of the SIM is represented in orange line. Parameters of the system are listed in configuration number 2 of Table 1. (Color figure online)

Fig. 13

Analytical predictions of equilibrium points of the continuous system (green line) accompanied by those obtained from direct numerical integration (black ‘+’ symbols) when $\tau \rightarrow \infty$ ($\tau >9.988\times 10^5$) with zero initial conditions under external excitation with the amplitude of $f_j=0.03 \cos (\omega _1(j-1))$ and the frequency of $\nu =0.064$. Unstable zone of the SIM is represented in orange line. Parameters of the system are listed in configuration number 2 of Table 1. (Color figure online)

Fig. 14

Periodic variations of $u_j(\tau )$ of the discrete system (numerical results) for different j ($j=x+1$) around equilibrium points of the system with zero initial condition under external excitation with the amplitude of $f_j=0.03 \cos (\omega _1(j-1))$ and the frequency of $\nu =0.064$ when $\tau \rightarrow \infty$ ($\tau >9.988\times 10^5$). Parameters of the system are listed in configuration number 2 of Table 1

Fig. 15

Transient response of $v_j(\tau )$ ($j=x+1$) of the discrete system (numerical results). Some cells present modulated responses before going to the equilibrium point which is represented in Fig. 13b. System is under external excitation with the amplitude of $f_j=0.03 \cos (\omega _1(j-1))$ and frequency of $\nu =0.064$ with zero initial conditions. Parameters of the system are listed in configuration number 2 of Table 1

Fig. 16

Forced responses of the cells 1 and 8 of the chain under external forcing around the third mode ($k=3$) with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$. a, c Frequency response curves of the cells 1 and 8 respectively. Backbone curves are represented by thick black lines while equilibrium points falling in unstable zone of the SIM are represented in orange line. b, d Time histories of amplitudes of concerned cells obtained from numerical results for system with the frequency of $\nu =0.1895$ and zero initial condition. System characteristics are given in configuration 3 in Table 1. (Color figure online)

Fig. 17

Forced responses of the cell 7 of the chain under external forcing around the third mode ($k=3$) with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$. a Frequency response curve of the cell. Backbone curves are represented by thick black lines while equilibrium points falling in unstable zone of the SIM are represented in orange line. b Time histories of amplitudes obtained from numerical results for system with the frequency of $\nu =0.1895$ and zero initial condition. System characteristics are given in configuration 3 in Table 1. (Color figure online)

Fig. 18

Forced response of the cell 1 in (a) and cell 8 in (b) around the third ($k=3$). The SIM of the system (green line) accompanied by numerical results (red line). Black ‘+’ symbols present amplitudes of possible singularities of the system. Unstable zone of the SIM is represented in orange line. Blue and red diamonds indicate maximal and minimal values of $N_1$ and $N_2$ respectively. System under external excitation with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$ and the frequency of $\nu =0.1895$ with zero initial condition. Parameters of the system are listed in configuration number 3 of Table 1. (Color figure online)

Fig. 19

Forced response of the cell 7 around the third mode ($k=3$). The SIM of the system (green line) accompanied by numerical results (red line). Black ‘+’ symbols present amplitudes of possible singularities of the system. Unstable zone of the SIM is represented in orange line. Blue and red diamonds indicate maximal and minimal values of $N_1$ and $N_2$. System under external excitation with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$ and the frequency of $\nu =0.1895$ with zero initial condition. Parameters of the system are listed in configuration number 3 of Table 1. (Color figure online)

Fig. 20

Poincaré diagrams ($u_j$ versus $\frac{du_j}{d\tau }$, $j\in \{1,8,7\}$): a cell 1; b cell 8; c cell 7. System is under external excitation with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$ and the frequency of $\nu =0.1895$ with zero initial condition. Parameters of the system are listed in configuration number 3 of Table 1

We can project second equation of Eq. 7 on the $k^{th}$ mode as:

$$\begin{aligned} \begin{array}{lcc} \int _{0}^{L}\left( \frac{\partial ^2 (U-V)}{\partial \tau ^2}(x,\tau ) - \varLambda V^3(x,\tau )- \chi _2 \frac{\partial V}{\partial \tau }(x,\tau )\right) \sqrt{\frac{2}{L}}\cos (\omega _kx+\varTheta _k)dx\\ \\ \quad =\frac{d^2\tilde{\rho }_{1,k}}{d\tau ^2}(\tau )-\frac{d^2\tilde{\rho }_{2,k}}{d\tau ^2}(\tau )-\chi _2\frac{d\tilde{\rho }_{2,k}}{d\tau }(\tau )-\frac{3\varLambda }{2L} {\tilde{\rho }_{2,k}}^3(\tau )=0 \end{array} \end{aligned}$$

(53)

New complex variables of Manevitch [21] are introduced as:

$$\begin{aligned} \left\{ \begin{array}{lcc} \displaystyle \varphi _{1} (\tau ) e ^{i\nu \tau }= \frac{d \tilde{\rho }_{1,k}}{d \tau }(\tau )+i\nu \tilde{\rho }_{1,k}(\tau ) \\ \\ \displaystyle \varPsi _{1} (\tau ) e ^{i\nu \tau }= \frac{d \tilde{\rho }_{2,k}}{d \tau }(\tau )+i\nu \tilde{\rho }_{2,k}(\tau ) \\ \end{array} \right. \end{aligned}$$

(54)

We are looking for the SIM and set $\nu =\omega _k$.

Taking the first harmonic of Eq. 53 expressed with complex variables provides:

$$\begin{aligned} \begin{array}{lcc} \frac{d}{d\tau }(\varphi _1-\varPsi _1)+i\omega _k(\varphi _1-\varPsi _1)-\chi _2\varPsi _1+\frac{9i\varLambda }{8L\omega _k^3}\varPsi _1^2\varPsi _1^*=0 \end{array} \end{aligned}$$

(55)

We seek for fixed points (see Eq. 30) so we can write:

$$\begin{aligned} \begin{array}{lcc} \varphi _1=\varPsi _1-\frac{i\chi _2}{2}\varPsi _1+\frac{9\varLambda }{8L\omega _k^4}\varPsi _1^2\varPsi _1^* \end{array} \end{aligned}$$

(56)

We introduce complex variables in polar domain as:

$$\begin{aligned} \left\{ \begin{array}{lcc} \varphi _1(\tau )=\mathcal {N}_1(\tau )e^{i\vartheta _1(\tau )}\\ \varPsi _1(\tau )=\mathcal {N}_2(\tau )e^{i\vartheta _2(\tau )} \end{array} \right. \end{aligned}$$

(57)

with $\mathcal {N}_1,\mathcal {N}_2 \in \mathbb {N}^2$ and $\vartheta _1,\vartheta _2 \in \mathbb {Z}^2$. Finally, this approach leads to the SIM expression:

$$\begin{aligned} \begin{array}{lcc} \mathcal {N}_1(\tau _1,\dots )=\sqrt{\left( \mathcal {N}_2(\tau _1,\dots )-\frac{9\varLambda }{8L \omega _k^4}\mathcal {N}_2^3(\tau _1,\dots )\right) ^2+\left( \frac{\chi _2 }{\omega _k}\mathcal {N}_2(\tau _1,\dots )\right) ^2} \end{array} \end{aligned}$$

(58)

The SIM does not depend to space variable x because of the modal projection. The expression is compared to numerical results using Runge Kutta algorithm in free and forced cases using the same methodology as in Sect. 4.

In the free case shown in Fig. 24 for the first mode ($k=1$) and for the same physical parameters listed in configuration 2 in Table 1 and initial deformation defined as $u_j(\tau =0)=0.13\cos (\omega _1(j-1))$, we can observe that the numerical results (in red line) are not really following the SIM (in green line) defined in Eq. 58 except in the part close to $\mathcal {N}_1(\tau )=\mathcal {N}_2(\tau )=0$, when all masses are in small displacements and system behave in a classical manner. We can observe that there are jumps in the numerical curve creating steps like aspect on the curve. In fact, these jumps correspond to the crossing of some cells through one bifurcation point. Indeed, each cell is at a different level of energy characterized by different amplitudes of $N_1(x,\tau )$ and $N_2(x,\tau )$, as defined in first part in Eq. 27. For this reason, each cell is susceptible to cross through bifurcation points at different instants than another cells. When projecting on the mode, if some of the cells are in the unstable zone, then when a cell faces a bifurcation point, there will be a jump in the numerical curve obtained after modal projection. This phenomena is the cause of the gap between the numerical integration and the SIM that is appearing “step by step” like on the numerical curve. This is confirmed by superposing time history of numerically obtained $\sqrt{\frac{2}{L}}\mathcal {N}_2(\tau )$ of projected discrete system with different numerically obtained discrete $N_2(x=j,\tau )$ time histories of twenty five first cells of the chain in Fig. 25. This figure illustrates well the influence of each cell on the modal projection results.

Same results are observable in the forced case: when forcing is added to the system, the numerical results are also less in agreement with the SIM except in the part close to $\mathcal {N}_1(\tau )=\mathcal {N}_2(\tau )=0$, when all masses are in small displacements and system behave in classical manner, as shown in Fig. 26 for excitation around the third modal frequency $\omega _3$. This time, jumps happen around the first bifurcation point $(N_{21},N_{11})$ for some cells creating the visible non-regularities on the numerical curve obtained after modal projection of discrete system in Fig. 26. This is confirmed in Fig. 29 where time history of numerically obtained $\sqrt{\frac{2}{L}}\mathcal {N}_2(\tau )$ of projected discrete system is compared to numerically obtained discrete $N_2(x=j,\tau )$ time histories of twenty five first cells of the chain. Moreover, when some cell amplitudes of the chain are in the unstable zone of the SIM while others are behaving in a classical manner, Fig. 29 permits to understand that the modal projection is less effective because it has to traduce two different types of behaviors at the same time. When all cells are behaving in a classical manner close to $N_1=N_2=0$ or at high amplitudes, spatial projection will provide good results, but does not have advantages in the analytical study compared to study in Sect. 3 while considering only one mode. We can remark for example from Fig. 29 that the numerical curve obtain after modal projection won’t be in agreement with the SIM when the distribution of the number of cells behaving in a classical manner close to $N_1=N_2=0$ and the number of cell having a modulated response is equitable. The SIM obtained without projection of the continuous system provides better results (see Figs. 27, 28).

For these reasons, the initial study has been conducted without modal projection.

Appendix 2: Discrete consideration of the system

1.1 General analytical determination of discrete system modes

In this part, we are providing the dynamical study in a discrete way. Let us consider the L-cell discrete system described in Eq. 2. We express the system in the matrix form. The study is limited to the order $\varepsilon ^0$. Equation 2 has the matrix form:

$$\begin{aligned} \left\{ \begin{array}{lcc} \frac{d^2{\textbf {U}}}{d \tau ^2}(\tau )+{\textbf {M}}_L.{\textbf {U}}=0\\ \\ \frac{d^2{\textbf {U}}}{d \tau ^2}(\tau )-\frac{d^2{\textbf {V}}}{d \tau ^2}(\tau )-\varLambda {\textbf { V}} ^3(\tau ) - \chi _2 \frac{d{\textbf {V}}}{d \tau }(\tau )=0\\ \\ {\textbf {U}}_j(\tau )=u_j(\tau )\hbox {, } {\textbf {V}}_j(\tau )=u_j(\tau )-v_j(\tau )\hbox { and } {\textbf {V}}^3_j(\tau )=(u_j(\tau )-v_j(\tau ))^3\\ \\ {\textbf {M}}_L=\begin{pmatrix} 2&{}-1&{} 0&{} &{} &{}\dots &{}&{}0&{}-1\\ -1&{}2&{}-1&{}0 &{}&{}\dots &{}&{}&{}0\\ 0&{}0&{}\ddots &{}\ddots &{}\ddots &{}0&{}&{} \dots &{}0\\ 0&{}\dots &{}0&{}-1&{}2&{}-1&{}0&{}\dots &{}0\\ 0&{}\dots &{}0&{}0&{}&{} \ddots &{}\ddots &{}\ddots &{}0\\ 0&{}&{}&{}\dots &{}&{}0&{}-1&{}2&{}-1\\ -1&{}0&{}&{}\dots &{}&{} &{}0&{}-1&{}2\\ \end{pmatrix} \hbox {a}\, L\times L \,\hbox {matrix} \end{array} \right. \end{aligned}$$

(59)

Following the same procedure as described in Eq. 14,15, the linearized system reads:

$$\begin{aligned} \left\{ \begin{array}{lcc} \frac{d^2{\textbf {U}}_l}{d \tau ^2}=-\lambda . {\textbf {U}}_l=-{\textbf {M}}_L.{\textbf {U}}_l \hbox { or } {\textbf {A}}_L.{\textbf {U}}_l=({\textbf {M}}_L-\lambda . {\textbf {Id}}_L).{\textbf {U}}_l=0\\ {\textbf {U}}_l(\tau )=\begin{pmatrix} u_1\\ \vdots \\ u_L \end{pmatrix}e^{i\sqrt{\lambda }\tau } \end{array} \right. \end{aligned}$$

(60)

${\textbf {Id}}_{L}$ being the LxL identity matrix. In order to use modal projection, we are interested by the eigenvalues and eigenvectors of the matrix ${\textbf {M}}_L$.

Fig. 21

Instantaneous frequency of $m_1$ located at different positions. a cell 1; b cell 8; c cell 7. System is under external excitation with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$ and the frequency of $\nu =0.1895$ with zero initial condition. Parameters of the system are listed in configuration number 3 of Table 1

Fig. 22

Analytical predictions of equilibrium points of the continuous system (green line) for system under external excitation with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$ and the frequency of $\nu =0.1895$. Unstable zone of the SIM is represented in orange line. Parameters of the system are listed in configuration number 3 of Table 1. (Color figure online)

Fig. 23

Analytical predictions of equilibrium points of the continuous system (green line) accompanied by those obtained from direct numerical integration (blue and red diamonds indicate maximal and minimal values of $N_1$ and $N_2$). Unstable zone of the SIM is represented in orange line. System is under external excitation with the amplitude of $f_j=0.01 \cos (\omega _3(j-1))$ and the frequency of $\nu =0.1895$ with zero initial condition. Parameters of the system are listed in configuration number 3 of Table 1. (Color figure online)

Fig. 24

The SIM of the continuous system after projection on its first mode ($k=1$), see Eq. 58, accompanied by numerical results. Numerical data are collected by projections of results (obtained from direct numerical integration of Eq. 2) on the first mode. Initial conditions are $u_j(\tau =0)=0.13\cos (\omega _1 (j-1))$ with $f_j=0$. System characteristics are listed in configuration 2 of Table 1

Fig. 25

Time histories of $\sqrt{2/L}\mathcal {N}_2(\tau )$ of the projected discrete system accompanied by those of $N_2(x=j,\tau )$, $j=1,\dots ,25$ of discrete system. $\mathcal {N}_2(\tau )$ numerical data are collected by projections of results (obtained from of direct numerical integration of Eq. 2) on the first mode. Definition of $N_2(\tau )$ and $\mathcal {N}_2(\tau )$ are in Eqs. 27 and 57 respectively. Initial conditions are $u_j(\tau =0)=0.13\cos (\omega _1 (j-1))$ with $f_j=0$. System characteristics are listed in configuration 2 of Table 1

Fig. 26

SIM of the continuous system after projection on third mode ($k=3$) in green line accompanied by numerical results of discrete system during repeated bifurcations. Numerical data are collected by projections of results (obtained from of direct numerical integration of Eq. 2) on third mode. External excitation has an amplitude $f_j=0.02\cos (\omega _3 (j-1))$ with frequency $\nu =0.1885$ and zero initial conditions. System characteristics are listed in configuration 2 in Table 1. (Color figure online)

Fig. 27

Forced response of the cell 1 around the first mode ($k=1$). The SIM of the system (green line) accompanied by numerical results (red line) during repeated bifurcations. Black $+$ symbols present amplitudes of possible singularities of the system. Unstable zone of the SIM is represented in orange line. System is under external excitation with the amplitude of $f_j=0.02\cos (\omega _3(j-1))$ and the frequency of $\nu =0.1885$ with zero initial condition. System characteristics are listed in configuration 2 of Table 1. (Color figure online)

Fig. 28

Forced response of the cell 11 around the first mode ($k=1$). The SIM of the system (green line) accompanied by numerical results (red line). Black $+$ symbols present amplitudes of possible singularities of the system. Unstable zone of the SIM is represented in orange line. System is under external excitation with the amplitude of $f_j=0.02\cos (\omega _3(j-1))$ and the frequency of $\nu =0.1885$ with zero initial condition. System characteristics are listed in configuration 2 of Table 1. (Color figure online)

Fig. 29

Time histories of $\sqrt{2/L}\mathcal {N}_2(\tau )$ of the projected discrete system accompanied by those of $N_2(x=j,\tau )$, $j=1,\dots ,25$ of discrete system. $\mathcal {N}_2(\tau )$ numerical data are collected by projections of results (obtained from of direct numerical integration of Eq. 2) on the third mode. System is under external excitation with the amplitude of $f_j=0.02\cos (\omega _3(j-1))$ and the frequency of $\nu =0.1885$ with zero initial condition. System characteristics are listed in configuration 2 of Table 1

Let us evaluate eigenvalues of ${\textbf {M}}_L$. For this purpose, we define the tri-diagonal matrix:

$$\begin{aligned} \begin{array}{lcc} {\textbf {B}}_L=\begin{pmatrix} \alpha &{}-1&{} 0&{}\dots &{}0 \\ -1&{}\alpha &{}-1&{}\ddots &{}\vdots \\ 0&{}\ddots &{}\ddots &{}\ddots &{}0\\ \vdots &{}\ddots &{}-1&{}\alpha &{}-1\\ 0&{}\dots &{} 0&{}-1&{}\alpha \\ \end{pmatrix}_{L \times L} \end{array} \end{aligned}$$

(61)

We define $\alpha =2-\lambda$ and using Gauss pivoting methodology, we decompose:

$$\begin{aligned} \begin{array}{lcc} \det ({\textbf {A}}_L)=\alpha . \det ({\textbf {B}}_{L-1})-(-1)^3\det (\begin{pmatrix} -1&{}-1&{} 0&{}\dots &{}\dots &{}0 \\ 0&{}\alpha &{}-1&{}0 &{}&{}\vdots \\ 0&{}-1&{}\alpha &{}-1&{}\ddots &{}\vdots \\ \vdots &{}\ddots &{}\ddots &{}\ddots &{}\ddots &{}0\\ 0&{}\dots &{}0&{}-1&{}\alpha &{}-1\\ -1&{}\dots &{}\dots &{}0&{}-1&{}\alpha \\ \end{pmatrix}_{L-1 \times L-1})\\ -(-1)^{L+1}\det (\begin{pmatrix} -1&{}\alpha &{}-1&{}0 &{} \dots &{}0 \\ 0&{}-1&{}\alpha &{}-1 &{}\ddots &{}\vdots \\ \vdots &{}\ddots &{}\ddots &{}\ddots &{}\ddots &{}0\\ \vdots &{}&{}\ddots &{}\ddots &{}\ddots &{}-1\\ 0&{}\dots &{}\dots &{}0&{}-1&{}\alpha \\ -1&{}\dots &{}\dots &{}\dots &{}0&{} -1\\ \end{pmatrix}_{L-1 \times L-1}) \end{array} \end{aligned}$$

(62)

where:

$$\begin{aligned} \begin{array}{lcc} \det (\begin{pmatrix} -1&{}-1&{} 0&{}\dots &{}\dots &{}0 \\ 0&{}\alpha &{}-1&{}0 &{}&{}\vdots \\ 0&{}-1&{}\alpha &{}-1&{}\ddots &{}\vdots \\ \vdots &{}\ddots &{}\ddots &{}\ddots &{}\ddots &{}0\\ 0&{}\dots &{}0&{}-1&{}\alpha &{}-1\\ -1&{}\dots &{}\dots &{}0&{}-1&{}\alpha \\ \end{pmatrix}_{L-1 \times L-1})=(-1)\det ({\textbf {B}}_{L-2})+\\ \\ (-1)(-1)^{L}\det (\begin{pmatrix} -1&{}0&{} 0&{}\dots &{}\dots &{}0 \\ \alpha &{}-1&{}0&{}&{} &{}\vdots \\ -1&{}\alpha &{}-1&{}\ddots &{}&{}\vdots \\ 0&{}\ddots &{}\ddots &{}\ddots &{}\ddots &{}0\\ \vdots &{}\ddots &{}\ddots &{}\ddots &{}\ddots &{}0\\ 0&{}\dots &{}0&{}-1&{}\alpha &{}-1\\ \end{pmatrix}_{L-2 \times L-2})\\ \\ =-\det ({\textbf {B}}_{L-2})+(-1)^{2L-1}=-\det ({\textbf {B}}_{L-2})-1 \end{array} \end{aligned}$$

(63)

and:

$$\begin{aligned} \begin{array}{lcc} \det (\begin{pmatrix} -1&{}\alpha &{}-1&{}0 &{} \dots &{}0 \\ 0&{}-1&{}\alpha &{}-1 &{}\ddots &{}\vdots \\ \vdots &{}\ddots &{}\ddots &{}\ddots &{}\ddots &{}0\\ \vdots &{}&{}\ddots &{}\ddots &{}\ddots &{}-1\\ 0&{}\dots &{}\dots &{}0&{}-1&{}\alpha \\ -1&{}\dots &{}\dots &{}\dots &{}0&{} -1\\ \end{pmatrix}_{L-1 \times L-1})=-1(-1)^{L}\det ({\textbf {B}}_{L-2})+\\ \\ (-1)\det (\begin{pmatrix} -1&{}\alpha &{}-1&{}0 &{}\dots &{}0 \\ 0&{}-1&{}\alpha &{}-1&{}\ddots &{}\vdots \\ \vdots &{}\ddots &{}\ddots &{}\ddots &{}\ddots &{}0\\ \vdots &{}&{}\ddots &{}\ddots &{}\ddots &{}-1\\ \vdots &{}&{}&{}\ddots &{}\ddots &{}\alpha \\ 0&{}\dots &{}\dots &{}\dots &{}0&{}-1\\ \end{pmatrix}_{L-2 \times L-2})\\ \\ =(-1)^{L+1}\det ({\textbf {B}}_{L-2})+(-1)(-1)^{L-2}=(-1)^{L+1}\det ({\textbf {B}}_{L-2})+(-1)^{L-1} \end{array} \end{aligned}$$

(64)

Finally, by re-injecting in Eq. 62 the previous simplified determinants, the following simple expression is obtained:

$$\begin{aligned} \begin{array}{lcc} \det ({\textbf {A}}_L)=\alpha .\det ({\textbf {B}}_{L-1})-2(\det ({\textbf {B}}_{L-2})+1) \end{array} \end{aligned}$$

(65)

Let us now evaluate $D_L=\det ({\textbf {B}}_L)$. In order to obtain non-trivial solutions of the system, we have to verify $\det ({\textbf {A}}_L)=0$. Therefore, Eq. 65 leads to a second degree characteristic polynomial and we set the general expression of the solutions of the constant recursive sequence:

$$\begin{aligned} \begin{array}{lcc} D_L=\varGamma {X_1}^n+\varSigma {X_2}^n\hbox {, }\{\varGamma ,\varSigma \}\in \mathbb {C}^2 \hbox {, }n\in \mathbb {N} \end{array} \end{aligned}$$

(66)

where $X_1$ and $X_2$ are the solutions of the characteristic polynomial.

We have the firsts relations:

$$\begin{aligned} \left\{ \begin{array}{lcc} D_0=\varGamma +\varSigma =1\\ D_1=\varGamma X_1+\varSigma X_2=\alpha \end{array} \right. \end{aligned}$$

(67)

Solving the system up to higher orders shows that $X_1$ and $X_2$ can’t have real expressions. Therefore, $X_1$ and $X_2$ must be conjugated complexes and we use polar expressions for the two unknowns:

$$\begin{aligned} \left\{ \begin{array}{lcc} X_1={\eta } e^{i\zeta }\\ X_2=\eta e^{-i\zeta } \end{array} \right. \end{aligned}$$

(68)

with $\eta ,\zeta \in \mathbb {R}^2$. Solving Eq. 67 leads to the two expressions:

$$\begin{aligned} \left\{ \begin{array}{lcc} \varGamma =\frac{\alpha -\eta e^{-i\zeta }}{2i \sin (\zeta )}\\ \varSigma =-\frac{\alpha -\eta e^{i\zeta }}{2i \sin (\zeta )} \end{array} \right. \end{aligned}$$

(69)

We can now also remark that:

$$\begin{aligned} \left\{ \begin{array}{lcc} D_0=\varGamma +\varSigma =\rho =1\\ D_2=\varGamma X_1^2+ \varSigma X_2^2=(2\alpha \cos (\zeta )-1)=\alpha ^2-1 \end{array} \right. \end{aligned}$$

(70)

The solution of the second relation leads to $\alpha =0$ or $\alpha =2 \cos (\zeta )$. Since $\alpha =2-\lambda$, we have the expression of the eigenvalues:

$$\begin{aligned} \begin{array}{lcc} \lambda =2(1-\cos (\zeta )) &\hbox {or}&\lambda =2 \end{array} \end{aligned}$$

(71)

$D_L$ reads:

$$\begin{aligned} D_L= & {} \frac{1}{2i\sin (\zeta )}\eta ^L\left( \alpha 2i\sin (L\zeta )-\eta 2i\sin ((L-1)\zeta )\right) \nonumber \\= & {} \frac{\sin ((L+1)\zeta )}{\sin (\zeta )} \end{aligned}$$

(72)

We can finally recompose the expression of det(${\textbf {A}}_L$):

$$\begin{aligned} \det ({\textbf {A}}_L)= & {} \alpha \frac{\sin ((L)\zeta )}{\sin (\zeta )}-2\left( \frac{\sin ((L-1)\zeta )}{\sin (\zeta )}+1\right) \nonumber \\= & {} 2(\cos (L\zeta )-1) \end{aligned}$$

(73)

$\det ({\textbf {A}}_L)$=0 if $\zeta =\frac{2k\pi }{L}$, $k=0,1,\dots ,L-1$.

According to Eq. 71, we have $L+1$ equations but we only need L solutions.

The solution $\lambda =2$ can be eliminated as general solution for every chain because it can’t verify the conditions of periodicity without being linked to the number of cells of the chain (except for the solution $\lambda =0$). However, the eigenvalue $\lambda =2$ can be solution when $\frac{k}{L}=\frac{1}{4} \implies \zeta =\frac{\pi }{2} \implies \lambda =2$ which in this case verifies periodicity conditions. We can conclude that all solutions can be found solving:

$$\begin{aligned} \begin{array}{lcc} \lambda =2(1-\cos (\zeta )) \end{array} \end{aligned}$$

(74)

with $\zeta =\frac{2k\pi }{L}$, $k=0,1,\dots ,L-1$.

Moreover, we can remark that:

$$\begin{aligned} \begin{array}{lcc} \cos \left( \frac{2k\pi }{L}\right) =\cos \left( \frac{2(L-k)\pi }{L}\right) \hbox { for }k=0,1,\dots ,L-1 \end{array} \end{aligned}$$

(75)

Therefore, we only have $\left\lfloor \dfrac{L}{2}\right\rfloor +1$ different eigenvalues, $L\in \mathbb {N}^*$. The first eigenvalue is $\lambda =0$, corresponding to the rigid body dynamic of the chain.

The treatment of ${\textbf {M}}_L.{\textbf {X}}=\lambda .{\textbf {X}}$ leads to the L eigenvectors. We can identify two cases:

• L is uneven, implying that the only eigenvalue associated to only one eigenvector is $\lambda _0$. Every other eigenvalues have two associated eigenvectors.

• L is even, implying that 2 eigenvalues $\lambda _0$ and $\lambda _{\left\lfloor L/2\right\rfloor }$ have both one associated eigenvector. Every other eigenvalues have 2 associated eigenvectors. We note ${\textbf {P}}$ the orthonormal transfer matrix and we adopt the following decomposition:

  $$\begin{aligned} \begin{array}{lcc} {\textbf {P}}=(\underbrace{{\textbf {P}}_0}_{\lambda _0}, \underbrace{{\textbf {P}}_1, {\textbf {P}}_2}_{\lambda _1},\ldots ,\underbrace{{\textbf {P}}_{2j-1},{\textbf {P}}_{2j}}_{\lambda _j},\ldots ,\underbrace{{\textbf {P}}_L}_{\lambda _{\left\lfloor L/2\right\rfloor } \hbox { if}\, L\, \hbox {is pair}})\\ \end{array} \end{aligned}$$

  (76)

  In all cases, we can use for the first eigenvector:

  $$\begin{aligned} \begin{array}{lcc} {{\textbf {P}}}_0 = \sqrt{\frac{1}{L}} \begin{pmatrix} 1&\,&\dots&\,&\,&1 \end{pmatrix} ^T \end{array} \end{aligned}$$

  (77)

On the contrary, during the last mode of the chain with an even number of cells, all cells present equal amplitudes while each cell is out of phase with its neighbors. We can use:

$$\begin{aligned} \begin{array}{lcc} {{\textbf {P}}}_L = \sqrt{\frac{1}{L}} \begin{pmatrix} -1&\,&1&\,&\dots (-1)^j&\,&\dots&-1&\,&1 \end{pmatrix} ^T \end{array} \end{aligned}$$

(78)

This modal decomposition is caused by the periodicity conditions of the chain. Indeed, the decomposition of the physical behavior according to each eigenvalue needs two different eigenvectors and this joins the continuous approach: we showed that the general expression of principal displacement was $U_l(x)=A_k\cos (\omega _k x) + B_k\sin (\omega _k x)$ (see Eq. 21). The determination of $A_k$ and $B_k$ can’t be done until an excitation is applied. Therefore, the general form of expression of deformation has to be described using $\cos (\omega _kx)$ and $\sin (\omega _kx)$ components. The same process is happening in the discrete study. Indeed, for each double eigenvalue $\lambda _k=\omega _k^2$, the 2 eigenvectors can be assimilated to $\sqrt{2/L}\cos (\omega _kx)$ and $\sqrt{2/L}\sin (\omega _kx)$, as shown on Figs. 30 and 31. On the first figure, ${\textbf {P}}_1$ and ${\textbf {P}}_2$ are represented in red and black ‘+’ symbols respectively while $\sqrt{2/L}\sin (\omega _1x)$ and $\sqrt{2/L}\cos (\omega _1x)$ are plotted in red and black respectively. On the second figure, ${\textbf {P}}_5$ and ${\textbf {P}}_6$ are presented in red and black ‘+’ symbols while $\sqrt{2/L}\sin (\omega _3x)$ and $\sqrt{2/L}\cos (\omega _3x)$ are plotted in red and black respectively. Eigenvectors are obtained using “eig” function of Matlab$^\circledR$ and system characteristics are configuration 1 in Table 1. Both discrete eigenvectors and continuous eigenfunctions have been normalized.

Fig. 30

Comparison between eigenvectors of the discrete system at first mode ($k=1$), ${\textbf {P}}_1$ and ${\textbf {P}}_2$, and components of the corresponding continuous system (see Eq. 52) in the form of $\sin (\omega _1x)$ and $\cos (\omega _1x)$. ${\textbf {P}}_1$ and ${\textbf {P}}_2$ are respectively in red and black ‘+’ symbols and normalized; $\sqrt{{2}/{L}}\sin (\omega _1x)$ and $\sqrt{{2}/{L}}\cos (\omega _1x)$ are represented in red and black continuous lines respectively and normalized to be compared. System characteristics are listed in configuration 1 of Table 1. (Color figure online)

Fig. 31

Comparison between eigenvectors of the discrete system at third mode ($k=3$), ${\textbf {P}}_5$ and ${\textbf {P}}_6$, and components of the corresponding continuous system (see Eq. 52) in the form of $\sin (\omega _3x)$ and $\cos (\omega _3x)$. ${\textbf {P}}_5$ and ${\textbf {P}}_6$ are respectively in red and black ‘+’ symbols and normalized; $\sqrt{{2}/{L}}\sin (\omega _3x)$ and $\sqrt{{2}/{L}}\cos (\omega _3x)$ are represented in red and black continuous lines respectively and normalized to be compared. System characteristics are listed of configuration 1 in Table 1. (Color figure online)

Modal frequencies obtain from continuous and discrete approach are compared for different sizes of chain in Table 2. When the number of cells is high ($L=100$ cells for example), there is a good agreement between $\omega _k$ defined in the Eq. 20 and the corresponding discrete pulsation $\sqrt{\lambda _k}$. As expected, when the number of cells decreases, as well as when the considered harmonic increases, results obtained from discrete and continuous approaches depart.

Table 2 Comparison between discrete and continuous modal values

1.2 The SIM of the discrete chain

We can now work from second equation of Eq. 59. ${\textbf {U}}$ and ${\textbf {V}}$ and their derivatives have the modal decomposition:

$$\begin{aligned} \left\{ \begin{array}{lcc} \frac{d^n{{\textbf {U}}}}{d\tau ^n}(\tau )=\sum _{j}\frac{d^n{\mathrm {u}}_j}{d\tau ^n}(\tau ){\textbf {P}}_j\\ \frac{d^n{{\textbf {V}}}}{d\tau ^n}(\tau )=\sum _{j}\frac{d^n{\mathrm {v}}_j}{d\tau ^n}(\tau ){\textbf {P}}_j \end{array} \right. \end{aligned}$$

(79)

with $n\in \mathbb {N}$. In our case, we only consider one $j^{th}$ eigenvalue:

$$\begin{aligned} \left\{ \begin{array}{lcc} \frac{d^n{\textbf {U}}}{d\tau ^n}(\tau )=\frac{d^n{\mathrm {u}}_{2j-1}}{d\tau ^n}(\tau ){\textbf {P}}_{2j-1}+\frac{{d^n\mathrm {u}}_{2j}}{d\tau ^n}(\tau ){\textbf {P}}_{2j}\\ \\ \frac{d^n{\textbf {V}}}{d\tau ^n}(\tau )=\frac{d^n{\mathrm {v}}_{2j-1}}{d\tau ^n}(\tau ){\textbf {P}}_{2j-1}+\frac{d^n{\mathrm {v}}_{2j}}{d\tau ^n}(\tau ){\textbf {P}}_{2j}\\ \\ \end{array} \right. \end{aligned}$$

(80)

We project the second equation of Eq. 59 on the 2 eigenvectors associated to the $j^{th}$ eigenvalue:

$$\begin{aligned} \left\{ \begin{array}{lcc} {\textbf {P}}_{2j-1}^T.\left( \frac{d^2{\textbf {U}}}{d\tau ^2}(\tau )-\frac{d^2{\textbf {V}}}{d\tau ^2}(\tau )-\varLambda {\textbf {V}} ^3(\tau ) - \chi _2 \frac{d{\textbf {V}}}{d\tau }(\tau )\right) =0\\ \\ {\textbf {P}}_{2j}^T.\left( \frac{d^2{\textbf {U}}}{d\tau ^2}(\tau )-\frac{d^2{\textbf {V}}}{d\tau ^2}(\tau )-\varLambda {\textbf {V}} ^3(\tau ) - \frac{d{\textbf {V}}}{d\tau }(\tau )\right) =0 \end{array} \right. \end{aligned}$$

(81)

We can now introduce new complex variables of Manevitch [21] defined as:

$$\begin{aligned} \left\{ \begin{array}{lcc} \displaystyle \phi _{2j-1} (\tau ) e ^{i\nu \tau }= \frac{d \mathrm {u}_{2j-1}}{d \tau }(\tau )+i\nu \mathrm {u}_{2j-1}(\tau ) \\ \displaystyle \phi _{2j} (\tau ) e ^{i\nu \tau }= \frac{d \mathrm {u}_{2j}}{d \tau }(\tau )+i\nu \mathrm {u}_{2j}(\tau ) \\ \displaystyle \psi _{2j-1} (\tau ) e ^{i\nu \tau }= \frac{d \mathrm {v}_{2j-1}}{d \tau }(\tau )+i\nu \mathrm {v}_{2j-1}(\tau ) \\ \displaystyle \psi _{2j} (\tau ) e ^{i\nu \tau }= \frac{d \mathrm {v}_{2j}}{d \tau }(\tau )+i\nu \mathrm {v}_{2j}(\tau ) \\ \end{array} \right. \end{aligned}$$

(82)

with $\nu =\sqrt{\lambda _j}$.

After keeping the first harmonic of the system, the complex variables are expressed in polar domain as:

$$\begin{aligned} \left\{ \begin{array}{lcc} \displaystyle \phi _{2j-1} (\tau ) = \mathscr {N}_{2j-1}e^{i\xi _{2j-1}}\\ \\ \displaystyle \phi _{2j} (\tau ) = \mathscr {N}_{2j}e^{i\xi _{2j}} \\ \\ \displaystyle \psi _{2j-1} (\tau ) = \mathscr {M}_{2j-1}e^{i\delta _{2j-1}} \\ \\ \displaystyle \psi _{2j} (\tau ) = \mathscr {M}_{2j}e^{i\delta _{2j}}\\ \end{array} \right. \end{aligned}$$

(83)

The methodology of the study is the same as in Sect. 3.

1.3 Application to a simple case

We consider a chain with parameters reported in configuration 1 of Table 1. We suppose that initial deformation of the chain is defined as

${\textbf {U}}(\tau =0)=\mathrm {u}_1(\tau =0){\textbf {P}}_1$ and that there is no other external excitation. Therefore, ${\textbf {U}}$ and ${\textbf {V}}$ are expressed only using ${\textbf {P}}_1$: we are able to set $\mathrm {u}_2(\tau )=0$ and $\mathrm {v}_2(\tau )=0$. We use the function “Eig” from Mathematica$^\circledR$ in order to obtain exact eigenvalues and eigenvectors and we normalize the vectors of the modal base. So, we are here investigating the following case:

$$\begin{aligned} \left\{ \begin{array}{lcc} L=10\\ {\textbf {U}}(\tau )=\mathrm {u}_1(\tau ){\textbf {P}}_1\\ {\textbf {V}}(\tau )=\mathrm {v}_1(\tau ){\textbf {P}}_1\\ \lambda _1=\frac{1}{2}(3-\sqrt{5})\hbox { first non-zero eigenvalue}\\ \end{array} \right. \end{aligned}$$

(84)

We obtain the following relation by injecting Eq. 84 in Eq. 81 and choosing $j=1$:

$$\begin{aligned} \begin{array}{lcc} \frac{d^2\mathrm {u}_1}{d\tau ^2}(\tau )-\frac{d^2\mathrm {v}_1}{d\tau ^2}(\tau )-\frac{3 \varLambda }{20}\mathrm {v}_1^3(\tau )-\chi _2\frac{d\mathrm {v}_1}{d\tau }(\tau )=0 \end{array} \end{aligned}$$

(85)

Noticing that we’ve set $L=10$, we can see that we obtain exactly the same equation as Eq. 53 during projection of the continuous system. The rest of the study will lead to the same equation of the SIM. Both continuous and discrete approaches provide similar results.