Nonlinear normal modes for damage detection

Abstract

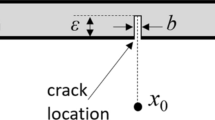
The sensitivity of the nonlinear dynamic response to damage is investigated in simply supported beams by computing the nonlinear normal modes (NNMs) and exploiting the rich information they unfold about the nonlinear system behavior. Damage is introduced as a reduction of the flexural stiffness within a small segment of the beam span. The problem is formulated in a piece-wise fashion and analytically tackled by using the method of multiple scales to compute the NNMs and the backbone curves. The latter describe the frequency versus free oscillation amplitude for each mode and as such they represent the skeleton of the nonlinear frequency response around each beam modal frequency. The bending of the backbones is regulated by the so-called effective nonlinearity coefficients associated with each mode. The comparison between the damaged and undamaged beams shows a sensitivity of the effective nonlinearity coefficients higher than the sensitivity of the linear natural frequencies. Moreover, the nonlinear frequency trends unfold an interesting dependence of the nonlinear free response on the stiffness reduction at the damage site. Such dependence yields useful information about the damage position. An effective identification strategy for the damage position is proposed by computing the damage-induced discontinuities in the second derivative of the NNMs (i.e., first order estimate of the bending curvature), without resorting to a baseline model of the undamaged beam. This goal is achieved by computing a high order derivative of the Nadaraya–Watson kernel estimator, which is evaluated from a finite set of sampled beam deflections associated with each individual NNM. The results show that the second derivatives of higher NNMs are more sensitive to damage-induced discontinuities than the linear modal curvatures. The robustness against noise of the damage identification process is also discussed.

Appendix

The resonant nonlinear force generated by the jth mode at third order can be piece-wise expressed as

$$\begin{aligned} g_{j,k}(x)&= -2\omega _j^2\varphi _{j,k}' \left( \sum _{i=1}^{k-1}{\int _{x_{i-1}}^{x_i}{\varphi _{j,i}'^2 \hbox {d}x}}+\int _{x_{k-1}}^x{\varphi _{j,k}'^2 \hbox {d}x}\right) \nonumber \\&\quad -3\omega _j^2\varphi _{j,k}{''} \Big (\sum _{i=1}^{n_e-k}{\int _{x_{n_e-i+1}}^{x_{n_e-i}} {\varphi _{j,n_e-i+1} \varphi _{j,n_e-i+1}'\hbox {d}x} +\int _{x_k}^x{\varphi _{j,k} \varphi _{j,k}'\hbox {d}x}}\Big )\nonumber \\&\quad -2\omega _j^2\varphi _{j,k}{''}\left[ \sum _{i=1}^{n_e-k}{\int _{x_{n_e-i+1}}^{x_{n_e-i}} {\left( \sum _{l=1}^{n_e-i}{\int _{x_{l-1}}^{x_l}{\varphi _{j,l}'^2\hbox {d}x}} +\int _{x_{n_e-i}}^x{\varphi _{j,n_e-i+1}'^2\hbox {d}x}\right) \hbox {d}x}}\right. \nonumber \\&\quad \left. +\int _{x_k}^x{\left( \sum _{i=1}^{k-1}{\int _{x_{i-1}}^{x_i}{\varphi _{j,i}'^2 \hbox {d}x}+\int _{x_{k-1}}^x{\varphi _{j,k}'^2 \hbox {d}x}}\right) \hbox {d}x}\right] -\tfrac{3}{2}\omega _j^2\varphi _{j,k}'^2\nonumber \\&\quad +\tfrac{3}{2}\alpha _{n_e} \varphi _{j,k}{''} \left. \varphi _{j,n_e}{''}^2\right| _{x=1}-\tfrac{9}{2}\alpha _k\varphi _{j,k}{''}^3-\tfrac{3}{2}\alpha _k \varphi _{j,k}'^2 \varphi _{j,k}{''''}\nonumber \\&\quad -3\alpha _{n_e} \left. \varphi _{j,n_e}'\right| _{x=1}\varphi _{j,k}{''} \left. \varphi _{j,n_e}{'''}\right| _{x=1}-9\alpha _k \varphi _{j,k}' \varphi _{j,k}{''} \varphi _{j,k}{'''}. \end{aligned}$$

(34)

If the lower summation index in Eq. 34 is greater than the upper index in the summations, the associated result is set to zero.

The function $f_j(x)$ representing the span-wise force distribution associated with the third harmonic $A_j^3 \hbox {e}^{3{\mathrm {i}} \omega _j T_0}$ is expressed as

$$\begin{aligned} f_j(x)&= -2\omega _j^2\varphi _j'\int _0^x{\varphi _j'^2\hbox {d}x} -\omega _j^2\varphi _j{''}\int _1^x{\varphi _j\varphi _j'\hbox {d}x}\nonumber \\&\quad -2\omega _j^2\varphi _j{''}\int _1^x{\int _0^x{\varphi _j'^2\hbox {d}x^2}} -\tfrac{1}{2}\omega _j^2\varphi _j\varphi _j'^2+\tfrac{1}{2}\alpha _{n_e} \varphi _j{''} \left. \varphi _j{''}^2\right| _{x=1}\nonumber \\&\quad -\tfrac{3}{2}\alpha _k\varphi _j{''}^3-\alpha _{n_e} \left. \varphi _j'\right| _{x=1} \varphi _j{''} \left. \varphi _j{'''}\right| _{x=1}-3\alpha _k \varphi _j' \varphi _j{''} \varphi _j{'''}-\tfrac{1}{2}\alpha _k \varphi _j'^2 \varphi _j{''''}. \end{aligned}$$

(35)