Abstract
A transient inverse heat conduction problem concerning jet impingement heat transfer has been solved analytically in this paper. Experimentally obtained transient temperature history at the non-impinging face, assumed to be the exposed surface in real practice, is the only input data. Aim of this study is to estimate two unknown thermo-physical parameters—overall heat transfer coefficient and adiabatic wall temperature—at the impinging face simultaneously. The approach of Green’s Function to accommodate both the transient convective boundary conditions and transient radiation heat loss is used to derive the forward model, which is purely an analytical method. Levenberg–Marquardt algorithm, a basic approach to optimisation, is used as a solution procedure to the inverse problem. An in-house computer code using MATLAB (version R2014a) is used for analysis. The method is applied for a case of a methane–air flame impinging on one face of a flat 3-mm-thick stainless steel plate, keeping Reynolds number of the gas mixture 1000 and dimensionless burner tip to impinging plate distance equals to 4, while maintaining the equivalence ratio one. Inclusion of both radiation and convection losses in the Green’s function solution for the forward problem enhances the accuracy in the forward model, thereby increasing the possibility of estimating the parameters with better accuracy. The results are found to be in good agreement with the literature. This methodology is independent of flow and heating conditions, and can be applied even to high-temperature applications.









Similar content being viewed by others
Abbreviations
- a:
-
Plate thickness (m)
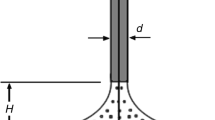
- d:
-
Inner diameter of burner (m)
- G:
-
Green’s function
- h:
-
Heat transfer coefficient (W m−2 K−1)
- k:
-
Thermal conductivity of plate material (W m−1 K−1)
- r:
-
Radius or radial distance from stagnation point (m)
- t:
-
Time (s)
- T:
-
Temperature (K)
- x:
-
Axial representation
- Z:
-
Burner tip to impinging plate distance (m)
- α :
-
Thermal diffusivity (m2 s−1)
- ε :
-
Emissivity
- \(\lambda_{n}\) :
-
Eigenvalue
- \(\sigma\) :
-
Stefan-Boltzmann constant (W m−2 K−4)
- \(\theta\) :
-
Transformed temperature (K)
- \(\tau\) :
-
Variable time in Green’s function (s)
- amb:
-
Ambient
- aw:
-
Adiabatic wall
- conv:
-
Convection
- nat:
-
Natural
- o:
-
Overall
- rad:
-
Radiation
References
Bee JM, Chigier NA. Impinging jet flames. Combust Flame. 1968;12:575–86.
Milson A, Chigier NA. Studies of methane and methane-air flames impinging on a cold plate. Combust Flame. 1973;21:295–305.
Jackson EG, Kilham JK. Heat transfer from combustion products by forced convection. Ind Eng Chem. 1956;48:2077–9.
Viskanta R. Heat transfer to impinging isothermal gas and flame jets. Exp Thermal Fluid Sci. 1993;6:111–34.
Baukal CE, Gebhart B. A review of semi-analytical solutions for flame impingement heat transfer. Int J Heat Mass Transf. 1996;39:2989–3002.
Baukal CE Jr, Gebhart B. A review of empirical flame impingement heat transfer correlations. Int J Heat Fluid Flow. 1996;17:386–96.
Chander S, Ray A. Flame impingement heat transfer: a review. Energy Convers Manag. 2005;46:2803–37.
Fay JA, Riddell FR. Theory of stagnation point heat transfer in dissociated air. J Aerospace Sci. 1958;25:73–85.
Sibulkin M. Heat transfer near the forward stagnation point of a body revolution. J Aeronautical Sci. 1952;9:570–1.
Conolly R, Davies RM. A study of convective heat transfer from flames. Int J Heat Mass Transf. 1972;15:2155–72.
Hargrave GK, Kilham JK. The effect of turbulence intensity on convective heat transfer from premixed methane-air flames. Inst. Chem. Eng. Symp. 1984;2:1025–34.
Van der Meer TH. Stagnation point heat transfer from turbulent low reynolds number jets and flame jets. Exp Thermal Fluid Sci. 1991;4:115–216.
Jambunathan K, Lai E, Moss MA, Button BL. A review of heat transfer data for single circular jet impingement. Int J Heat Fluid Flow. 1992;13:106–15.
Zuckerman N, Lior N. Jet impingement heat transfer: physics, correlations, and numerical modeling. Adv Heat Transf. 2006;39:565–631.
Qiu D, Wang C, Luo L, Wang S, Zhao Z, Wang Z. On heat transfer and flow characteristics of jets impinging onto a concave surface with varying jet arrangements. J Thermal Anal Calorim. 2019. https://doi.org/10.1007/s10973-019-08901-6.
Ghasemian M, Ramiar A, Ranjbar AA. Numerical investigation of boiling heat transfer in a quenching process of jet impingement considering solid temperature distribution. J Therm Anal Calorim. 2019;136(6):2409–20. https://doi.org/10.1007/s10973-018-7900-9.
Mozumder AK, Monde M, Woodfield PL, Islam MA. Maximum heat flux in relation to quenching of a high temperature surface with liquid jet impingement. Int J Heat Mass Transf. 2006;49(17–18):2877–88.
Karwa N, Gambaryan-Roisman T, Stephan P, Tropea C. Experimental investigation of circular free-surface jet impingement quenching: transient hydrodynamics and heat transfer. Exp Thermal Fluid Sci. 2011;35(7):1435–43.
Gan T, Ming T, Fang W, Liu Y, Miao L, Ren K, Ahmadi MH. Heat transfer enhancement of a microchannel heat sink with the combination of impinging jets, dimples, and side outlets. J Thermal Anal Calorim. 2019. https://doi.org/10.1007/s10973-019-08754-z.
Kuntikana P, Prabhu SV. Isothermal air jet and premixed flame jet impingement: heat transfer characterisation and comparison. Int J Therm Sci. 2016;100:401–15.
Kadam AR, Tajik AR, Hindasageri V. Heat transfer distribution of impinging flame and air jets–a comparative study. Appl Therm Eng. 2016;92:42–9.
Hadipour A, Zargarabadi MR, Dehghan M. Effect of micro-pin characteristics on flow and heat transfer by a circular jet impinging to the flat surface. J Thermal Anal Calorim. 2020. https://doi.org/10.1007/s10973-019-09232-2.
Kowsary F, Razzaghi H, Ashjaee M. Experimental design for estimation of the distribution of the convective heat transfer coefficient for a bubbly impinging jet. J Therm Anal Calorim. 2020;140:439–56. https://doi.org/10.1007/s10973-019-08819-z.
Mohaghegh MR, Rahimi AB. Single-and two-phase water jet impingement heat transfer on a hot moving surface. J Therm Anal Calorim. 2019;137(4):1401–11. https://doi.org/10.1007/s10973-019-08072-4.
Zhen HS, Leung CW, Cheung CS. Heat transfer characteristics of an impinging premixed annular flame jet. Appl Therm Eng. 2012;36:386–92.
Chander S, Ray A. Influence of burner geometry on heat transfer characteristics of methane/air flame impinging on flat surface. Exp Heat Transf. 2006;19:15–38.
Huang XQ, Leung CW, Chan CK, Probert SD. Thermal characteristics of a premixed impinging circular laminar-flame jet with induced swirl. Appl Energy. 2006;83:401–11.
Remie MJ, Särner G, Cremers MF, Omrane A, Schreel KR, Aldén LE, De Goey LP. Heat-transfer distribution for an impinging laminar flame jet to a flat plate. Int J Heat Mass Transf. 2008;51:3144–52.
Loubat R, Reulet P, Estebe B, Millan P. Heat flux characterisation in hot jet and flame/wall interaction by IHCP resolution coupled with infrared measurements. Quant Infrared Thermogr J. 2004;1:205–28.
Hindasageri V, Vedula RP, Prabhu SV. Heat transfer distribution for impinging methane–air premixed flame jets. Appl Therm Eng. 2014;73:461–73.
Kadam AR, Prabhu SV, Hindasageri V. Simultaneous estimation of heat transfer coefficient and reference temperature from impinging flame jets. Int J Therm Sci. 2018;131:48–57.
Fernandes AP, Dos Santos MB, Guimarães G. An analytical transfer function method to solve inverse heat conduction problems. Appl Math Model. 2015;39:6897–914.
Ozısık MN. Heat conduction. New York: John Wiley & Sons; 1993.
Morse PM, Feshbach H. Methods of theoretical physics, vol. 1–2. London: McGraw-Hill; 1953.
Ozisik MN. Boundary value problems of heat conduction. New York: Courier Corporation; 2002.
Carslaw HS, Jaeger JC. Conduction of heat in solids. 2nd ed. Oxford: Clarendon Press; 1959.
Colaço MJ, Orlande HR, Dulikravich GS. Inverse and optimization problems in heat transfer. J Braz Soc Mech Sci Eng. 2006;28:1–24.
Orlande HR. Inverse problems in heat transfer: new trends on solution methodologies and applications. J Heat Transf. 2012;134:25–47.
Naphon P, Wiriyasart S, Arisariyawong T, Nakharintr L. ANN, numerical and experimental analysis on the jet impingement nanofluids flow and heat transfer characteristics in the micro-channel heat sink. Int J Heat Mass Transf. 2019;131:329–40.
Beck JV, Blackwell B, Clair CR Jr. Inverse heat conduction: Ill-posed problems. New Jersey: Wiley; 1985.
Marquardt DW. An algorithm for least-squares estimation of nonlinear parameters. J Soc Ind Appl Math. 1963;11:431–41.
Bejan A. Convection heat transfer. 4th ed. New Jersey: John wiley & sons; 2013.
Hindasageri V, Vedula RP, Prabhu SV. Blowoff stability of methane-air premixed flame on tube burners. Int J Emerg Multidiscip Fluid Sci. 2011;3:45–57.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix: Derivation of Green’s function
Appendix: Derivation of Green’s function
In this section, the Green’s function of the problem under consideration is derived using classic separation of variable (SoV) technique. The advantage of Green’s function is that solution of a differential equation (say, a transient heat conduction problem) can be represented in terms of Green’s function. The solution procedure to derive the Green’s function for an one-dimensional transient heat conduction problem with transient convective boundary condition at one face and transient heat flux at another boundary is discussed in following five steps.
Step 1 Consider the homogenous version of the PDEs at Eqs. (3, 3.1, 3.2, 3.3) suitable for SoV technique and redefine as below.
Step 2 The solution of the formulations at step 1 can be readily referred in literature [33] (Sect. 2-4, 2-5), and the same is given by
where\(\lambda_{n} \tan (\lambda_{n} a) = H, \, n \, = \, 1,2,3, \ldots\)
The eigenvalues ‘\(\lambda_{n}\)’ are the roots of the transcendental equation above.
Step 3 The equation at step 2 can be rewritten as,
Step 4 From the fundamentals of Green’s function [33] (Chapter 6), the standard expression for the solution to problem at step 1, having homogenous differential equation and boundary conditions with a non-homogenous initial condition, is generalised as,
where ‘G’ is the Green’s function and ‘F’ is the initial condition.
Step 5 Comparing equation at step 4 and equation at step 3, appropriate Green’s function is given by,
In this step, as a standard procedure, \(t\) is to be replaced by \(t - \tau\).
Rights and permissions
About this article
Cite this article
Parida, R.K., Madav, V. & Hindasageri, V. Analytical solution to transient inverse heat conduction problem using Green’s function. J Therm Anal Calorim 141, 2391–2404 (2020). https://doi.org/10.1007/s10973-020-09803-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10973-020-09803-8