Introduction

The structure and activity of membrane-active peptides are closely related to the ability to associate with cell membrane [1–5]. Thus, the structural studies of such peptides by solution NMR spectroscopy require membrane-mimicking environment for proper folding and stability. And here, the structural complexity of biological membranes becomes quite a challenge. Among the most prominent membrane models suitable for the peptide–membrane interaction studies, the similarity to biological membranes decreases in the order: liposomes, bicelles, mixed micelles, and micelles [6]. The NMR studies of peptides in the presence of liposomes are usually limited to the solid state due to their considerable size. In solution NMR, the slow molecular tumbling resulting from the liposome size leads to a broadening of the NMR resonances, which makes achievement of a high-resolution 3D structure of the liposome-bound peptide difficult or even impossible [7, 8]. In turn, micelles stand for a good compromise between suitable membrane model and the need for relatively fast tumbling system in view of their short rotational correlation time [9]. The most commonly used micellar membrane-mimicking systems are aqueous solutions of surfactants such as dodecylphosphocholine (DPC) and sodium dodecyl sulfate (SDS) [10–14]. DPC provides a zwitterionic surface on the micelles that adequately mimic biological membranes of the vertebrates, whereas the SDS micelles, with negatively charged headgroups, adequately imitate the bacterial membrane [15]. However, to mimic electrostatic properties of the vertebrate plasma, a membrane model characterized by a slight prevalence of the negative charge, such as that in the DPC/SDS mixed micelles, can be used as well [16–18]. The use of SDS to imitate membrane environment is not always rational because it has no structural analogues among the phospholipids that are components of biological membranes. However, SDS is the only commercially available detergent that is completely deuterated and negatively charged.

In the present work, we use the isothermal titration calorimetry (ITC) to study the micellization of DPC, SDS, and DPC/SDS mixed systems as a function of the temperature. The relative arrangement and interactions of zwitterionic DPC with anionic SDS were studied by NMR. The diffusion coefficient measurements were used to determine the size of the micellar aggregates.

Materials and methods

Reagents

The DPC was purchased from Avanti Polar Lipids, Inc, whereas SDS from Sigma-Aldrich.

ITC measurements

All ITC experiments were performed over the 293–323 K temperature range using an AutoITC isothermal titration calorimeter (MicroCal Inc. GE Healthcare, Northampton, USA). The data were analyzed with Origin 7 software (MicroCal, Inc.). Specifically, the experiment consisted of injecting 10.02 μL (29 injections, 2 μL for the first injection only) of a 20 mM buffered surfactant solution into a 1.4491-mL cell containing a buffer solution (PBS, a 10 mM phosphate buffer, pH 7.4, containing 2.7 mM potassium chloride and 137 mM sodium chloride). Each injection lasted 20 s. The titrant was injected at 4-min intervals to ensure that the titration peak returned to the baseline before the next injection. To ensure a homogeneous mixing in the cell, the stirrer speed was kept constant at 300 rpm. The same procedure was repeated for aqueous buffer-free solutions of surfactants studied at 298 K. A calibration of the AutoITC calorimeter was carried out electrically by using electrically generated heat pulses. The CaCl2–EDTA titration was performed to check the apparatus, and the results (n—stoichiometry, K, ΔH) were compared with those obtained for the same samples (a test kit) at MicroCal Inc. GE Healthcare.

Surface tension measurements

The critical micelle concentration (cmc) of the mixed micelles composed of DPC and SDS taken at mole ratios of 1:0, 9:1, and 5:1 was determined by plotting the surface tension as a function of the logarithm of total surfactant concentration and was found as the intersection of two lines that best fit through the pre- and post-cmc data. Concentrated solutions of the surfactants of known concentration were progressively diluted. All surfactant solutions were prepared in water purified by HLP5 system. The surface tension of each sample was determined at 298 K by the Du Noüy ring method on an Easy Dyne tensiometer (Krüss GmbH, Germany).

NMR experiments

Diffusion measurements

To extract information about the self-diffusion coefficients (D tr) of the DPC-containing micelles, the DPFGDSTE (double pulsed field gradient double stimulated echo) experiments were conducted at 293, 303, and 313 K on an Agilent VNMRS 600 NMR (1H resonance frequency 599.93 MHz) spectrometer equipped with a 5-mm probehead (DOTY Scientific Ltd.) dedicated for diffusion measurements. The echo attenuation intensity (I) as a function of gradient amplitude (g) is expressed by Stejskal–Tanner equation [19]: \( I = I_{0} \exp [ - Dg^{2} \gamma^{2} \delta^{2} (\Delta - \delta /3)] \), where I 0 is the echo intensity in the absence of field gradient pulse, \( \gamma = 2.675 \times 10^{8}\, {\text{rad T}}^{ - 1} {\text{s}}^{ - 1} \) is the gyromagnetic ratio of the 1H nucleus, δ is the duration of the field gradient pulse, and ∆ is the time period between the two field gradient pulses. In our experiments, the diffusion time ∆ was 50 ms and the diffusion gradient time δ had a maximum duration of 2 ms. A single exponential decay of the integral intensity of the signal at 3.23 ppm [N(CH3)3] versus diffusion gradients \( g^{2} \gamma^{2} \delta^{2} (\Delta - \delta /3) \) was used to determine the translational self-diffusion coefficients.

1H and 31P relaxation measurements for DPC-containing micelles

The longitudinal (T 1) and transverse (T 2) relaxation times were measured on a Varian Unity Plus 500 MHz spectrometer (1H resonance frequency 499.83 MHz) using the inversion recovery (π–τ–π/2) and the Carr–Purcell–Meiboom–Gill (CPMG) pulse sequences, respectively. The protonated DPC and SDS were used in all the experiments. The 1:0, 9:1, and 5:1 DPC/SDS mixed systems were studied at a total surfactant concentration fixed at 150 mM. All NMR measurements were taken in a 10 mM deuterated phosphate buffer (pD 7.4).

Results and discussion

ITC results

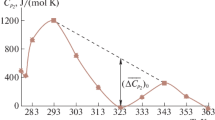
The thermodynamic properties of the micelles were determined using ITC in a 10 mM phosphate buffer (PBS), pH 7.4, containing 2.7 mM potassium chloride and 137 mM sodium chloride. In the ITC experiment, the first injections of the micellar surfactant solution into buffer solution result in a heat being the sum of three processes, i.e., dilution of micelles, demicellization and dilution of the resultant monomers. The release of counterions is included in the observed reaction but cannot be separated [20]. Once the total concentration of the surfactant in the reaction cell reaches cmc, a sharp change in the heat of injection is observed. At the end of the experiment, the enthalpy change is solely the result of micelle dilution without breaking down the micelles [21]. The difference between the enthalpy before and after micellization gives the enthalpy of demicellization process, ΔH demic. The heat of micelle formation has the opposite sign, i.e., ΔH mic = −ΔH demic. The calculation of the first derivative of the ITC curve with respect to the total surfactant concentration in the cell allows us to determine the critical micelle concentration (cmc). The procedure of determining the enthalpy of micellization and cmc is shown in Fig. 1.

Fig. 1
figure 1

ITC experiment for the determination of the cmc of DPC in 10 mM PBS at pH 7.4 at 298 K. a Raw data from ITC titration (heat flow vs. time), b integrated injection heats versus DPC concentration in the reaction cell, and c first derivative of ITC titration curve calculated

Full size image

The standard Gibbs energy of micellization process (ΔG mic) is related to the critical micelle concentration (cmc) by the following equation [22–24]:

$$ \Delta G_{\text{mic}} = {\text{RT}}\ln X_{\text{cmc}} $$
(1)

where RT is the thermal energy and X cmc is the critical micelle concentration in mole fraction units with respect to water. The entropy of micelle formation was determined from a standard thermodynamic relationship [23, 24]:

$$ \Delta G_{\text{mic}} = \Delta H_{\text{mic}} - T\Delta S_{\text{mic}} $$
(2)

The mixed micelles in PBS reveal a two-step aggregation mechanism: cmc and ΔH mic

The enthalpy of micellization is the result of several processes. The endothermic contribution to the micellization is related to release of water from the “hydrophobically hydrated” nonpolar hydrocarbon chains of surfactant monomers during their transfer from solution into the micellar core. The stabilization of the monomers in the micelle, the counterion binding to the micelle, and the associated hydration give an exothermic contribution to the process [25]. Thus, the resultant enthalpy, ΔH mic, becomes more negative with increasing temperature. The temperature at which the enthalpy of micellization, ΔH mic, changes from endothermic to exothermic depends on the nature of the surfactant.

In the case of SDS, the micellization process is always exothermic over the studied temperature range, whereas for the DPC, the micellization is endothermic (ΔH mic > 0) at lower temperatures and exothermic (ΔH mic < 0) at higher ones (Table 1). The DPC/SDS mixed micelles exhibit two inflection points in ITC curves up to a temperature of ca. 313 K. The analysis of the first derivative of ITC curve clearly shows the presence of two extrema indicating two cmc values denoted by cmc1 and cmc2 (Fig. 2).

Table 1 Thermodynamic parameters of micelle formation for DPC, SDS, and DPC/SDS mixed micelles
Full size table
Fig. 2
figure 2

ITC experiment for the determination of the cmc of the 9:1 DPC/SDS in 10 mM PBS at pH 7.4 at three temperatures. Top panel corresponds to the experimental raw data (heat flow vs. time), bottom panel corresponds to the integrated injection heats and the first derivative of the ITC titration curve calculated from the interpolated values. Two-step aggregation mechanism is observed for the 9:1 DPC/SDS system over the temperature range of 293–308 K. Increasing temperature results in a single-step micellization process

Full size image

The second transition region at cmc2 is much broader with no abrupt change in enthalpy. Consequently, cmc2 and enthalpy of the second step of micellization could be measured less accurately. The presence of two cmcs indicates a two-step aggregation mechanism. Surfactants with at least two cmcs are known as “multiple-cmc systems” [26] and have previously been reported elsewhere [20, 25, 27–29]. Both steps of the micellization processes in the DPC/SDS mixed micelles are endothermic (ΔH mic = –ΔH demic) at low temperatures, and due to proximity of both cmc values, the separation of two reaction enthalpies is difficult (Fig. 2). In turn, over the ranges of 303–313 and 298–308 K for the 9:1 DPC/SDS and 5:1 DPC/SDS, respectively, the first step of micellization becomes gradually more exothermic, whereas the second one gradually less endothermic. This facilitates determination of the enthalpy of each step separately (Fig. 2). At higher temperatures, micellization takes place as a single-step exothermic process with only one cmc (Table 1). The similar tendency was previously observed for alkyl triphenylphosphonium bromides [29].

The cmc value of pure DPC in 10 mM PBS at 298 K is 1.36 mM and is consistent with the recent results [30]. In the case of SDS, cmc depends strongly on the solution ionic strength. Previous studies have shown that in water, the cmc of SDS is ca. 8 mM, whereas in buffered solution or in the presence of 150 mM NaCl, it decreases to about 1–2.5 mM [23, 30–33]. In our studies, the cmc of SDS in PBS at 298 K is 1.1 mM, which is consistent with the reported data. Addition of SDS to the DPC micelle results in a decrease in the cmc values to 0.94 and 0.65 mM at 298 K for the 9:1 and 5:1 DPC/SDS systems, respectively (assuming the cmc1 values for both mixtures). As it has been earlier reported, the cmc for the mixture of two oppositely charged surfactants becomes significantly smaller than that of separate surfactants due to mutual association of the surfactants induced by electrostatic attraction [34–36]. The present results can also be explained assuming that the association between the zwitterionic DPC and anionic SDS is favorable due to electrostatic attractions between the cationic fragment of DPC and the dodecyl sulfate anion. Consequently, those electrostatic interactions are considered to be largely responsible for the synergism in the formation of the DPC/SDS mixed micelles.

The cmc data of the micelles studied are summarized as a function of temperature in Fig. 3. In pure SDS, the distinct presence of the minimum in the cmc (vs. temperature) is not observed up to the lower studied temperature of 293 K. For both DPC and 9:1 DPC/SDS micelles, the minimum of cmc occurs at 308 K and is shifted to 297 K for 5:1 DPC/SDS (taking into account the cmc1 for the mixed micelles). In the case of the 9:1 DPC/SDS, the value of cmc2 is distinctly greater than cmcs of both DPC and SDS surfactants at lower temperatures. However, at 308 and 313 K, it is very close to that obtained for pure DPC. In turn, the position of the cmc2 for the 5:1 DPC/SDS is always between cmc values determined for pure DPC and SDS and does not change with increasing temperature. It is worth emphasizing that the cmc determined previously in PBS solution for 9:1 DPC/SDS system at 298 K (1.35 mM) [30] has been found to be an average of both cmcs obtained by calorimetry in this paper.

Fig. 3
figure 3

Temperature-dependent values of ln X cmc for the micelles studied in PBS pH 7.4 by calorimetric methods. The data were fitted with a second-degree polynomial function

Full size image

The temperatures (T 0) at which the enthalpy of micellization vanishes and the process is entropically driven are derived from the intersection of second polynomial approximation of the temperature dependence of ΔG mic and −TΔS mic terms (ΔG mic1 and −TΔS mic1 for the DPC/SDS mixed micelles) as shown in Fig. 4. The T 0 temperatures were found to be 309, 302, 300, and 292 K for DPC, 9:1 DPC/SDS, 5:1 DPC/SDS, and SDS, respectively. As seen, it shifts to lower values as the concentration of negatively charged SDS increases. Application of the same method to the published ITC data for micellization of SDS in 0.1 M NaCl and 50 mM HEPES buffer of pH 7.4 gives T 0 values at around 292 K [23] and 291 K [32], respectively. The temperatures T 0 deduced from the enthalpy curves are more or less consistent with those obtained from the temperature dependence of cmcs. However, like previous reporting [23], if the cmc is a directly measured quantity, the minimum may not be easily detected and the transition temperature (T 0) determined from the temperature dependence of ΔH mic provides a more precise determination for the temperature of the cmc minimum.

Fig. 4
figure 4

Thermodynamic parameters for micellization of a DPC, b SDS, c 9:1 DPC/SDS, and d 5:1 DPC/SDS as a function of temperature. Intersection of the second polynomial approximation of ΔG mic and −TΔS mic versus temperature gives the transition temperature, T 0

Full size image

Heat capacity of micellization

The first derivative of ΔH mic with respect to temperature at constant pressure yields the change in heat capacity ΔC p,mic, and this, in turn, at 298 K is shown as a linear function of the hydrophobic surface in micelle not exposed to the water. The number of “dry” hydrogen atoms (n H) in the micelle of the surfactant with a straight hydrocarbon chain, which are not in contact with water, is expressed by the following equation [23, 37]:

$$ \Delta C_{{p,{\text{mic}}}} = 33 n_{\text{H}}\,\left( {{\text{J}} {\text{mol}}^{ - 1} {\text{K}}^{ - 1} } \right) $$
(3)

In the present study, all the ΔC p,mic values were found to be negative throughout the whole temperature range, which is normally observed for self-association of surfactants leading to micelle formation.

Figure 5 illustrates the temperature dependence of ΔC p,mic (ΔC p,mic1 for the DPC/SDS mixed micelles). The ΔC p,mic values become less negative with increasing temperature as ΔH mic is not a linear function of temperature. At 298 K, ΔC p,mic are −484, −521, −428, and −439 J mol−1 K−1 for DPC, SDS, 9:1 DPC/SDS, and 5:1 DPC/SDS, respectively. This means that about 15, 16, 13, and 13 hydrogen atoms are not in contact with water in DPC, SDS, 9:1 DPC/SDS, and 5:1 DPC/SDS, respectively, which corresponds to the terminal methyl group plus six for pure DPC and SDS micelles and five methylene groups for both mixed micelles. Thus, the DPC/SDS mixed micelles seem to be hydrated deeper by one methylene group than the pure ones, which may suggest either a less compact structure or a lower aggregation number. However, these calculations are oversimplified because they are based on the assumption that only the hydrophobic chain affects the heat capacity. Meanwhile, the heat capacity change during the micellization can also be related to changes in the so-called hydrophilic hydration. During the micellization process, the condensation of ions onto micelles reduces the number of water molecules in the solvation shell of both counterions and polar headgroups since the counterions can share hydration water with the headgroups [31]. “Hydrophilic dehydration” produces an increase in the heat capacity and behaves in an opposite manner to “hydrophobic dehydration”, which decreases the heat capacity. For pure zwitterionic DPC, the changes in ΔC p,mic value are negligible within the whole temperature range, which suggests that ΔC p,mic can be assumed to solely reflect the change in the exposure of hydrophobic tails to water during the micellization. The largest changes in ΔC p,mic with increasing temperature are observed for pure anionic SDS, where the “hydrophilic dehydration” is associated with dehydration of sodium ions and sulfate polar headgroups. In the case of the DPC/SDS mixed micelles, besides the possible dehydration of sodium ions and sulfate headgroups, also the dehydration of phosphate group should be taken into account. The partitioning of the SDS into the zwitterionic DPC micelle may result in the formation of a salt bridge between the quaternary amine group of DPC and negatively charged sulfate group of SDS. Consequently, the sodium ions can bind to the phosphate group of DPC. In this case, dehydration of phosphate groups may contribute to ΔC p,mic. The change in heat capacity for sodium ions during dehydration was estimated to be 42 J K−1 mol−1 [38]. Hence, assuming that the counterion binding degree of SDS is ca. 0.7 [32], the heat capacity associated with dehydration of sodium ions is only 6 % of the total contribution of ΔC p,mic in the micellization process of SDS in PBS buffer. In turn, there is no quantitative data on the heat capacity associated with dehydration of sulfate and phosphate groups of SDS and DPC, respectively. In this case, we can compare only values calculated for dehydration of the sulfate (SO 2−4 ) and phosphate (PO 3−4 ) ions and these are, respectively, 193 and 341 J K−1 mol−1 [38]. Considering these data, an obvious impact of “hydrophilic dehydration” on the heat capacity change during micellization of the SDS and DPC/SDS mixed micelle is suggested. This would reduce the number of methylene groups in contact with water in SDS-containing micelles. Following this consideration, less negative ΔC p,mic values obtained for the DPC/SDS mixed micelle than for other micelles can be a consequence of the overcompensating effect of the enhanced “hydrophilic dehydration”.

Fig. 5
figure 5

Changes in heat capacity observed for the micellization of surfactants as a function of temperature. The lines were obtained from a second-order polynomial fit for the micellization enthalpies

Full size image

Enthalpy–entropy compensation

The free energy ΔG mic is negative over the whole temperature range studied (Tab.1), which means that micellization is thermodynamically favorable and occurs spontaneously. At lower temperature, the contribution of the enthalpy to the Gibbs energy is small compared to the entropy term. However, as mentioned above, with increasing temperature, the enthalpy of micellization becomes more negative and its contribution to the Gibbs energy increases. In turn, the entropy of micellization is positive and decreases at elevated temperature. Thus, its contribution to the Gibbs energy also decreases. The ΔG mic exhibits only slightly temperature dependence (Fig. 4) which results from the enthalpy–entropy compensation [39].

The enthalpy–entropy compensation plots for the micelles studied are shown in Fig. 6. This linear relationship can be ascribed by equation [40]:

$$ \Delta H_{\text{mic}} = T_{\text{c}} \Delta S_{\text{mic}} + \Delta H_{\text{mic}}^{*} $$
(4)
Fig. 6
figure 6

Enthalpy–entropy compensation plot for the micelles studied

Full size image

The slope in the equation is the compensation temperature (T c) and represents solute–solvent interactions. It can be interpreted as a measure of dehydration of the hydrocarbon chain (desolvation part of micellization). The intercept, \( \Delta H_{\text{mic}}^{*} \), characterizes the solute–solute interactions, i.e., aggregation of hydrocarbon tails into micelle (chemical part of micellization) [40]. The T c values are 305 K, 300 K, 322 K, and 300 K for DPC, SDS, 9:1 DPC/SDS, and 5:1 DPC/SDS, respectively, and are consistent with those reported for various surfactants [39]. The higher T c value found for the 9:1 DPC/SDS micelle than for the other systems can be attributed to some difference in the water–surfactant interaction mode during micellization process. The corresponding \( \Delta H_{\text{mic}}^{*} \) values are −27.0, −27.2, −29.5, and −28.3 kJ mol−1. As seen, the difference between zwitterionic DPC and anionic SDS is here negligible, thus supporting the opinion that under the condition of a fixed number of carbon atoms in a straight hydrocarbon tail, the surfactants with different polar headgroups exhibit a small variation in the intercept \( \Delta H_{\text{mic}}^{*} \) due to relatively small effects of the hydrophilic group on the chemical part of the micellization process [41]. However, the slight decrease in \( \Delta H_{\text{mic}}^{*} \) upon addition of SDS to DPC can be explained in terms of a higher stability of the zwitanionic micelles. Given that \( \Delta H_{\text{mic}}^{*} \) accounts for the solute–solute interactions, the observed decrease in \( \Delta H_{\text{mic}}^{*} \) corresponds to enhancement of these interactions.

Mixed micelles in unbuffered solution

The ITC measurements of DPC-containing micelles were also taken in unbuffered solution at 298 K. It is interesting to note that only a single-step micellization process is observed with the mixed micelles under these conditions (Fig. 7). The cmc values determined by titration calorimetry in the buffer-free solution are comparable with those obtained by the surface tension method (Table 2 and Fig. 1S, Supplementary Material). The results indicate that the cmc of the zwitterionic DPC as well as zwitanionic 5:1 DPC/SDS decreases with an increasing ionic strength of solution. The presence of the electrolyte generally results in a decrease in the cmc of most surfactants, wherein the strongest effect could be noticed with the ionic ones. An increase in the solution ionic strength results in an effective screening of the headgroup repulsion leading to tight packing and reduction in cmc. As reported elsewhere, the cmc of pure SDS in the presence of electrolytes may decrease up to eight times as compared to that in water [23, 30–32]. In the case of zwitterionic DPC, this effect is less pronounced because of the overall neutral charge of the surfactant. Therefore, it is proposed that the drop in the cmc of DPC might be due to salting out and the implication of phosphocholine headgroups in organization of the surrounding water molecules [42]. It is worth noting that for the 9:1 DPC/SDS system, the change in cmc observed upon transfer from the unbuffered to buffered solution is negligible (taking into account cmc1 in PBS).

Fig. 7
figure 7

ITC experiments for the determination of the cmc of the DPC/SDS mixed micelles in aqueous buffer-free solution at 298 K. Top panels correspond to the experimental raw data (heat flow vs. time), while bottom ones correspond to the integrated injection heats and the first derivative of the ITC titration curve calculated from the interpolated values. In contrast to the buffer solution, a single-step aggregation mechanism is observed for the mixed micelles at 298 K

Full size image
Table 2 A summary of critical micelle concentration values determined for DPC-containing micelles from surface tension method and ITC in water at 298 K
Full size table

Surfactant–surfactant interactions in the mixed micelle

Interaction parameter β obtained from the Rubingh’s regular solution theory (RST) [43] has commonly been used to characterize the strength and nature of interactions between two different surfactants in nonideal mixtures. Positive values of β correspond to repulsive electrostatic interactions, preventing the micelle formation, whereas the negative values indicate attractive electrostatic interactions favorable for the micelle formation. For the nonideal mixing behavior, parameter β can be calculated from the following expression [43]:

$$ \beta = \ln \left( {\frac{{\alpha_{\text{SDS}} \cdot cmc_{\text{mix}} }}{{\chi_{\text{SDS}} \cdot cmc_{\text{SDS}} }}} \right)\left( {1 - \chi_{\text{SDS}} } \right)^{ - 2} $$
(5)

where α SDS is the total mole fraction of SDS and χ SDS is the mole fraction of SDS in the mixed micelle. χ SDS parameter can be calculated by solving the following numerical equation [43]:

$$ \frac{{\chi_{\text{SDS}}^{2} \ln \left( {\frac{{\alpha_{\text{SDS}} \cdot cmc_{\text{mix}} }}{{\chi_{\text{SDS}} \cdot cmc_{\text{SDS}} }}} \right)}}{{\left( {1 - \chi_{\text{SDS}} } \right)^{2} \ln \left( {\frac{{\left( {1 - \alpha_{\text{SDS}} } \right)cmc_{\text{mix}} }}{{\left( {1 - \chi_{\text{SDS}} } \right)cmc_{\text{DPC}} }}} \right)}} = 1 $$
(6)

The knowledge of β and χ SDS parameters allows us to determine the activity coefficients f SDS and f DPC by [43]:

$$ f_{\text{SDS}} = \exp \left[ {\beta \left( {1 - \chi_{\text{SDS}} } \right)^{2} } \right] $$
(7)
$$ f_{\text{DPC}} = \exp \left[ {\beta \chi_{\text{SDS}}^{2} } \right] $$
(8)

Accordingly, experimental values of cmc of two surfactants along with their activity coefficients in the mixture allow us to calculate cmc mix of a nonideal mixed micellar system [43]:

$$ \frac{1}{{cmc_{\text{mix}} }} = \frac{{\alpha_{\text{SDS}} }}{{f_{\text{SDS}} \cdot cmc_{\text{SDS}} }} + \frac{{\left( {1 - \alpha_{\text{SDS}} } \right)}}{{f_{\text{DPC}} \cdot cmc_{\text{DPC}} }} $$
(9)

For an ideally mixed system, activity coefficients f SDS and f DPC are equal to 1 and Eq. (9) is reduced to give a Clint’s model [44]:

$$ \frac{1}{{cmc_{\text{mix}}^{\text{ideal}} }} = \frac{{\alpha_{\text{SDS}} }}{{cmc_{\text{SDS}} }} + \frac{{\left( {1 - \alpha_{\text{SDS}} } \right)}}{{cmc_{\text{DPC}} }} $$
(10)

For the mixed systems studied, mole fractions of SDS in the mixed micelles (χ SDS), interaction parameter (β), and activity coefficients (f SDS and f DPC) are listed in Table 1S (Supplementary Material). The mole fraction of SDS (χ SDS) is always greater than the total mole fraction α of 0.1 and 0.17 for the 9:1 DPC/SDS and 5:1 DPC/SDS mixed micelles, respectively. This results from nonideal behavior and indicates that more SDS is transferred into the mixed micelle than is expected for an ideally mixed system. Over the whole temperature range studied, β parameters are negative and their absolute values are greater than those of ln(cmc SDS/cmc DPC) for both mixed systems. This clearly indicates that SDS and DPC are synergetic in the formation of the mixed micelle in PBS solution. The synergy could also arise from the remarkable deviation between the experimental cmc values and those calculated for the ideally mixed system described by the Clint’s model (Fig. 2S, Supplementary Material).

NMR characterization of mixed micelles

Self-diffusion measurements

To get some insight into the micelle’s size upon mixing of two surfactants, translational diffusion coefficients (D tr) were determined with the DPFGDSTE (double pulsed field gradient double stimulated echo) experiment for DPC-containing micellar systems. Due to the two-step aggregation mechanism observed for the DPC/SDS mixed micelles over the temperature range 293–313 K, we decided to conduct self-diffusion measurements at three temperatures within that range and to compare them with the results obtained for pure DPC, which reveals only a single-step aggregation mechanism. The signal attenuation as a function of diffusion gradients, \( g^{2} \gamma^{2} \delta^{2} (\Delta - \delta /3) \), associated with the choline methyl groups is mono-exponential for each studied system over the whole range of temperature (Fig. 3S, Supplementary Material). This results from free bulk diffusion (unrestricted system) with the diffusion coefficient independent of the diffusion time [45, 46].

The determined self-diffusion coefficients of the studied micelles together with the hydrodynamic radius (R h) are given in Table 2S (Supplementary Material). The radius was estimated based on experimentally determined D tr according to Stokes–Einstein’s equation. The calculated values for the radius of the DPC micelle as well as its two mixtures with SDS vary between 32 and 36 Å. Thus, addition of SDS only slightly increases the size of the micelle. The results obtained for DPC are in excellent agreement with those of the previous measurements, where the diffusion coefficient for the 228 mM DPC in a 10 mM phosphate buffer solution was found to be 9.17 × 10−11 m2 s−1 at 310 K [11]. When corrected for temperature due to viscosity differences, this leads to an equivalent of D tr = 9.87 × 10−11 m2 s−1 at 313 K. This value is close to that obtained for DPC at 313 K (D tr = 10.69 × 10−11 m2 s−1) in this study.

Molecular mobility of DPC-containing micelles

The 1H spectrum of DPC displays six well-resolved signals (Fig. 4S, Supplementary Material). The 1H NMR signals from the C3–C11 fragment of the hydrocarbon chain (1.25 ppm) are strongly overlapped; therefore, average relaxation times were calculated for this range of chemical shifts. Furthermore, the signals from H2, H3–H11, and H12 protons of DPC overlap those arising from SDS in the DPC/SDS mixed micelles. Nevertheless, both surfactants have the same length of hydrocarbon chain and, in our opinion, the protons in the corresponding positions of the hydrocarbon chain of DPC and SDS can be treated as identical.

As follows from our data, the 1H T 1 profiles are similar for all the micellar systems studied at three temperatures (Fig. 5S, Supplementary Material). The data exhibit a plateau with an average value of 0.6–0.8 s for the 1H signals within the polar fragment of DPC. The short T 1s within the polar segment of the micelles are a consequence of its restricted mobility. Besides, small differences in the T 1 values suggest either that DPC headgroup is a compact entity or that the motions take place in a synchronized manner. Remarkably higher values of T 1 observed for the hydrogens of terminal methyl group suggest enhanced dynamics and flexibility.

Examination of the data of spin–spin relaxation (T 2) shows that methylene protons on the polar headgroup (Hβ and Hα) and H1 on the hydrocarbon chain in the micellar systems studied reveal a heterogeneous dynamic behavior reflected in a two-component T 2 relaxation (Figs. 6S and 7S, Supplementary Material). An increase in the temperature results in a bi-exponential T 2 model for H2 methylene protons and the terminal methyl group. The choline methyl groups (3.23 ppm) and the methylene groups in the C3–C11 fragment of the hydrocarbon chain (the overlapped signal at 1.25 ppm) relax mono-exponentially within the temperature range studied (Fig. 7S, Supplementary Material). The bi-exponential 1H T 2 relaxations are characterized by a short component with T 2 of 15–80 ms and a long one with T 2 of 200–900 ms. A weighting factor for the fast-relaxing component ranges from 10 to 20 % at 293 K and increases approximately three times at 313 K. The fractions of the fast-relaxing methylene protons on the polar headgroup (Hβ and Hα) and H1 on the hydrocarbon chain reveal similar weighting factors of about 15, 25, and 50 % at 293, 303, and 313 K, respectively. A significant difference is observed for H2 at 313 K (at 293 and 303 K H2 protons relax mono-exponentially), where the weighting factor of the fast-relaxing component is reduced by ca. 20–30 % as compared to those of Hβ, Hα, and H1. The T 2 decay curve of the terminal methyl group exhibits bi-exponentiality only at 313 K, similar to H2 methylene protons, with the weighting factor of the fast-relaxing component in the range of 10–15 %. Since the T 2 values could previously be treated as a measure of spatial distribution of identical protons [47], one could construct a picture of chain packing in the micelle based on the 1H T 2 values of a particular part of surfactant molecule. Moreover, knowing that 1H spin–spin relaxation is sensitive to molecular motion, bi-exponentiality of T 2 relaxation time indicates existence of two types of molecular motions in solution. The relaxation component with a short T 2 value can be attributed to the restricted chain segments, i.e., undergoing a slow motion, whereas that with the long T 2 value corresponds to the segments that have a fast motion due to higher mobility, e.g., the more hydrated segments [47–49]. Following this interpretation, we concluded that the mono-exponential behavior of the choline methyl groups at each temperature studied with a high T 2 value of 415–670 ms, irrespective of the micelle composition, indicates protons exposed to aqueous phase. The decrease in the T 2 values for subsequent protons indicates a tighter packing as expected for groups either approaching or forming hydrophobic core of the micelle. Thus, the T 2s values observed for the H1 and H2 protons are by more than twice lower than those of the (CγH3)3 methyl groups. Some inconsistencies appear with the Hβ and Hα methylene protons at 313 K, where the slow-relaxing component is characterized by T 2s values higher than those calculated for the choline methyl groups (Fig. 7S, Supplementary Material). However, as seen, both T 2 values are subjected to considerable error. The T 2 value of the terminal methyl group in the hydrocarbon chain is distinctly greater than those for the remaining segments of the hydrophobic micellar core. This indicates its greater mobility. This conclusion is compatible with theoretical studies on DPC micelle [50]. The theoretical DPC model indicates that the surfactant chains are significantly disordered and that the terminal methyl groups of the surfactant alkyl chains are broadly distributed throughout the micelle’s volume. Besides, the tendency of the hydrocarbon chain to “swing” toward the interfacial region increases the hydration number of the terminal methyl group. In turn, the change in the relaxation behavior of protons with increasing temperature, i.e., increase in the weighting factors for the fast-relaxing components, indicates gradual dehydration of the micelle making it more compact.

The T 1/T 2 ratio can be related to the degree of restricted motion of respective nuclei. Thus, the variation in the T 1/T 2 ratios along the whole DPC molecule can correlate with the molecular packing of a particular part of the surfactant in the micelle. The T 1/T 2 ratios were calculated by using T 2s values for the bi-exponentially relaxing protons. We considered the data obtained at 293 K because at this temperature, the slow-relaxing component of the bi-exponentially decaying protons predominates with weighting factors greater than 80 %.

1H T 1/T 2 ratios are remarkably larger for the hydrocarbon chains than for polar headgroups of DPC (Fig. 8), indicating more restricted motions of the former and their tighter packing. The largest T 1/T 2 ratio was determined for the H1 methylene protons, and it gradually decreased upon going to the end of the hydrocarbon chain. This suggests that H1 protons are the main constituent of the rigid interfacial layer core protecting the penetration of water into the hydrophobic interior. An addition of SDS leads to an increase in the T 1/T 2 ratios beginning from Hα methylene protons in the polar headgroup of DPC and ending at the terminal methyl group of the hydrocarbon chain, while the T 1/T 2 ratios of the choline methyl groups and Hβ methylene protons of the phosphocholine headgroup remain unchanged. These data suggest that the hydrocarbon chain of SDS is more or less covered with that of DPC, as expected based on their identical lengths.

Fig. 8
figure 8

1H T 1/T 2 ratios in pure DPC, 9:1 DPC/SDS, and 5:1 DPC/SDS micelles at 293 K

Full size image

Mixing SDS with DPC shortens only slightly the T 1 values of the 31P nucleus. The change variations do not exceed 6 % (Table 3). A much greater reduction is seen in the transverse relaxation time T 2, where a decrease in the range of 9–44 % has been noticed. The reduction in T 2 values of 31P increases upon raising the SDS concentration. Since T 2 is sensitive to variations of both overall and local correlation times, whereas T 1 depends mainly on local correlation times, the results indicate that the average local dynamics of the DPC molecule is not affected by the presence of SDS. However, its presence leads to an increase in the overall correlation time, i.e., in the hydrodynamic volume of the micelle.

Table 3 Longitudinal (T 1) and transverse (T 2) relaxation times of the phosphorus 31P in the DPC and DPC/SDS mixed micelles at different mole ratios with a total concentration of 150 mM
Full size table

Conclusions

In the present paper, we studied the micellization of DPC, SDS, and the DPC/SDS mixed micelles at two molar ratios 9:1 and 5:1 using isothermal calorimetric titration (ITC). NMR relaxation measurements were used to explore the molecular mobility of the DPC-containing micelles. The diffusion measurements were taken to determine the size of the micelles at a high concentration, well above their cmc.

According to ITC, the DPC/SDS mixed systems show the tendency to form two kinds of micelles in PBS solution at lower temperatures. At 293 K, both steps of micellization are endothermic. An increase in the temperature causes an endothermic-to-exothermic transition only of the first step of micellization, while the second step becomes still endothermic. Further increase in temperature results in only a single micellization process with exothermic heat change. This reveals the formation of only one kind of the micelle at a higher temperature. Existence of two structures is usually attributed to either changes in the composition in the mixed micelles or transition of the shape with the increase in a total surfactant concentration [20, 28, 29]. As the size of the pure DPC and the DPC/SDS mixed micelles is comparable, as determined by diffusion measurements, we suppose that both kinds of the mixed micelles found at lower temperatures by ITC differ from each other in the content of individual surfactants. In our opinion, the cmc1 corresponds to the mixed micelle with a higher content of SDS. It is worth noting that in unbuffered aqueous solution, only one kind of the micelle occurs for the mixture of DPC and SDS and this phenomenon requires further investigations.