Abstract
The fractal dimensions of polymer chains and high-temperature graphs in the Ising model both in three dimension are determined using the conformal bootstrap applied for the continuation of the O(N) models from \(N=1\) (Ising model) to \(N=0\) (polymer). Even for non-integer N, the O(N) sum rule allows one to study the unitarity bound formally defined from the positivity, which may be violated in a non-unitary CFT. This unitarity bound of the scaling dimension for the O(N)-symmetric-tensor develops a kink as a function of the fundamental field as in the case of the energy operator dimension in the \(\mathbb {Z}_2\) (Ising) sum rule. Although this kink structure becomes less pronounced as N tends to zero, we found instead an emerging asymmetric minimum in the current central charge \(C_J\). Despite the non-unitarity of the O(N) model at non-integer N, we find the \(C_J\)-kink along the unitarity bound lies very close to the location of the infrared (IR) O(N) CFT estimated by other methods. It is pointed out that certain level degeneracies at the IR CFT should induce these singular shapes of the unitarity bounds. As an application to the quantum and classical spin systems, we also predict critical exponents associated with the \(\mathcal {N}=1\) supersymmetry, which could be relevant for locating the corresponding fixed point in the phase diagram.




Similar content being viewed by others
Notes
This dimension of the relevant operator in T-sector is denoted by \(\Delta _T\) following the convention in [3]. This “T” should not be confused with the stress-energy tensor \(T^{\mu \nu }\), which has spin 2 with O(D) rotations and the fixed scaling dimension D.
One further issue that we were not able to discuss here is a logarithmic nature of the \(N=0\) CFT, which is related to the collision of the dimensions \(\Delta _T \rightarrow \Delta _S\) (see the references cited in Sect. 5, especially interesting [10]). Since the logarithmic CFT may be regarded as a limit of ordinary CFT, it is also possible that a certain scaling of the CFT data including \(\lambda _{\phi \phi }^{T^{\mu \nu }}\) in \(N\rightarrow 0\) may help bootstrapping the theory at \(N=0\).
The non-renormalization of these dimensions is due to the topological property of the O(N)-vertex: \(\sum _{d} \delta _{cd}\cdot \left( \delta _{ab}\delta _{cd} + \delta _{ac}\delta _{bd} + \delta _{ad}\delta _{bc} \right) =(N+2)\delta _{ab}\), which vanishes at \(N=-2\), regardless of the space dimension D [15].
It is also well known in 2D that \(d_F=4/3\) coincides with the Flory value [40] \(d_F=\nu ^{-1}\sim (D+2)/3\).
This paper focuses on the approach to \(N=0\) through \(\Delta _T\). However, some crudest benchmark for the singlet dimension is possible using \(\Delta _S(1)=1.41264(6)\) obtained for the Ising model [42] as follows: \(\Delta _S(0)=1.41264(6)+(-1)\times (0.1238(28)+0.1017(35))/2=1.300(22)\), which is consistent with \(\Delta _T(0)\) in Sect. 4.3 as expected.
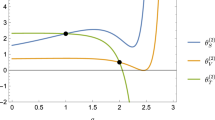
For 1 / N coefficient, there is a mismatch by a factor 2 between (4.25) and (6.8) of [9]. Our results on the slope (29) in Fig. 3 as well as [48,49,50] supports the value \(-64/9\pi ^2\) in (27) reproduced from (4.25). Also by using a Padé analysis on (28), the universal curves \(\Delta _\phi \)–\(\Delta _S\) and \(\Delta _\phi \)–\(\Delta _T\) in Fig. 1 can be drawn, which will be discussed elsewhere.
We thank Tomoki Ohtsuki for pointing out that similar kinks in \(C_J\) can be observed via the direct \(C_J\) minimization in \(D=3\) [54] for \(N\geqslant 2\), and Yu Nakayama for further discussions. For \(C_T\) in 3D, it is known that the direct \(C_T\) minimization reproduces \(C_T\) along the unitarity bound (via \(\Delta _{S}\)-maximization) for \(N=1\), but not for generic \(N>2\) [3]. It is possible to check similar characteristics are shared by \(C_J\) in 3D. It would be also interesting to study the implication of these phenomena on the solution space of the crossing symmetry.
In view of the picture that there are infinitely many \(\mathbb {Z}_2\) symmetric primary operators above \(\varepsilon =:\phi ^2:\) whose levels are separated by non-trivial intervals and may be repulsive to each other, the observed straightness of the lowest level \(\Delta _{\phi ^2}\) on the right side of the kink (\(\Delta _\phi >\Delta _{\phi ;\; \text {Ising}}\)) is also remarkable.
Another important example of a singlular OPE coefficient in \(N\rightarrow 0\) may be \(\lambda ^\varepsilon _{\varepsilon \varepsilon }\) for three energy operators, which would play a role in the mixed correlator bootstrap [43]. The physical origin of the divergence of \(\lambda ^\varepsilon _{\varepsilon \varepsilon }\) can be traced back to the strong repulsion between the loop segments \(\varepsilon \) in the \(O(N\rightarrow 0)\) loop model [60].
Around \(N=1\) the saturation of (33) may not be so serious as the kink in \(\Delta _{S}\) appears around the expected Ising position, which should be consistent with our observation that the OPE coefficient \(\lambda ^T_{1,0}\) of the (unphysical) level \(\Delta _T=1\) is negligible compared to those for other operators. However, below \(N=1\) this makes much difference: for instance, a kink in \(\Delta _S\) emerges even in \(N=0.1\), which was smoothed in the solution with the pure unitarity conditions. Again, it is obvious that this solution with \(\Delta _T=1\) can not represent a physical spectrum.
An analogous R-bifurcation of a spin-1 operator is also observed in the \(\mathcal {N}=2\) supersymmetric (SUSY) Ising model [61], where the decoupling operator of the lower branch never touches the level of the \(J_{ab}^{\mu }\) at \(\Delta =2\). It is also remarkable that the \(N=0\) model has a twisted \(\mathcal {N}=2\) SUSY in 2D [62], whose origin, the presence of underlying Osp(2M, 2M) for any M in \(N=0\) [63], is actually independent of the space dimension D.
This L-bifurcation of \(\Delta ^{(2)}_S\) (dimension for \(\mathcal {E}'=:E^2:\) with \(E=\sum _a \phi _a^2\)) is accompanied by a level crossing of \(\Delta ^{(2)}_T\) (dimension for \(:EF_{ab}:\) with \(F_{ab}=\phi _a\phi _b-E/N\)) and \(\Delta ^{(3)}_T\) for \(N\geqslant 1\). In the Ising model, \(\Delta ^{(2)}_S\) becomes \(\Delta _{\phi ^4}\sim 3.8\), which gives the correction to scaling exponent \(\omega \sim 0.8\). In the XY model (\(N=2\)), we reproduce \(\Delta ^{(2)}_T\sim 3.65\) [64,65,66]. More detailed study of the subleading spectrum is beyond the scope of this work.
A recent MC result \(\gamma =1.156957(9)\) [68] amounts to \(\Delta _\phi =0.515518(13)\) via the scaling relation \(2-\eta =\gamma /\nu \), although one may distinguish it from the direct measurement of \(\eta \) [17]. It is also possible that (37) shifts systematically to a larger value if the truncation \(\mathcal {K}\) gets higher as no extrapolations is performed for \(\Delta _\phi \).
It is equivalent to find the intercept at \(x=0\) in the linear fit for the data \((x,y)=(1/\mathcal {K}^{p}, {\Delta _{T}}^{*}(\mathcal {K}))\).
It would be interesting to study this slope a as a function of \(D<4\), though it is beyond the scope here (see [25] for related figures). One ad-hoc interpolation between the two values \(a\sim 3\) (\(D=3\)) and \(a=8/3\) (\(D=2\)) is \(a=2(6-D)/(5-D)\) inspired by the approximate coincidence of a with the critical dimensions where \(\phi ^6\) and \(\phi ^8\) becomes RG marginal.
The same approximate value \(\Delta _{\phi }=0.565\) has recently been appeared in the fermion bootstrap [71].
Another identification \(\Delta _{\phi ^2}^{\mathcal {N}=1}\rightarrow \Delta _\varepsilon \) yields \(\nu \sim 0.701\), which seem to agree with four estimates for \(\nu \) (\(\sim 0.71\)) by the functional RG [77] for the \(\mathcal {N}=1\) UV Lagrangian. Note that the latter \(\nu \) is not meant for a physical realization (e.g. the Blume-Capel model) and is just an indication that \(\Delta _{\phi ^2}^{\mathcal {N}=1}\) computed from the mass renormalization may agree with the conformal bootstrap. In the 2D \(\mathcal {N}=1\) fixed point, for instance, the observed value \(2-\alpha =d\nu =10/9\sim 1.11\) [78] follows from \(\Delta _{\phi }^{\mathcal {N}=1}=1/5\), but not from \(\Delta _{\phi ^2}^{\mathcal {N}=1}\).
These norms are considered in the AdS\(_4\)/CFT\(_3\) holography for large N. We thank Tassos Petkou for pointing out that there would be an interesting physics also for small N, where the bulk degrees of freedom in this higher-spin holography may be related with a polymer-like product of the singletons [87] in the replica limit.
The same idea applies to the case \(N >1\). For some \(N\geqslant 2\), \(\Delta _T\) has been determined from the conformal bootstrap [3]. For instance, we will have \(d_F=1.76437(108)\) for the high-temperature graphs in the XY model (\(N=2\)), which agrees with the simulations \(d_F=1.7626(66)\) [11], 1.7655(20) [88], and 1.765(3) [17].
In the process, this computation naturally reproduces the \(\tau \)-series for \(y_2=3-\Delta _T\) in [90].
References
El-Showk, S., Paulos, M.F., Poland, D., Rychkov, S., Simmons-Duffin, D., Vichi, A.: Solving the 3D Ising model with the conformal bootstrap. Phys. Rev. D 86, 025022 (2012)
El-Showk, S., Paulos, M.F., Poland, D., Rychkov, S., Simmons-Duffin, D., Vichi, A.: Solving the 3D Ising model with the conformal bootstrap II. c-minimization and precise critical exponents. J. Stat. Phys. 157, 869 (2014)
Kos, F., Poland, D., Simmons-Duffin, D.: Bootstrapping the O(N) vector models. JHEP 1406, 091 (2014)
Belavin, A.A., Polyakov, A.M., Zamolodchikov, A.B.: Infinite conformal symmetry in two-dimensional quantum field theory. Nucl. Phys. B 241, 333 (1984)
Bauer, M., Bernard, D.: 2D growth processes: SLE and Loewner chains. Phys. Rep. 432, 115 (2006)
Beffara, V.: The dimension of the SLE curves. Ann. Probab. 36, 1421 (2008)
Duplantier, B., Saleur, H.: Exact critical properties of two-dimensional dense self-avoiding walks. Nucl. Phys. B 290, 291 (1987)
de Gennes, P.G.: Exponents for the excluded volume problem as derived by the Wilson method. Phys. Lett. A 38, 339 (1972)
Petkou, A.: Conserved currents, consistency relations and operator product expansions in the conformally invariant \(O(N)\) vector model. Ann. Phys. 249, 180 (1996)
Hogervorst, M., Paulos, M., Vichi, A.: The ABC (in any D) of Logarithmic CFT. arXiv:1605.03959
Winter, F., Janke, W., Schakel, A.M.J.: Geometric properties of the three-dimensional Ising and XY models. Phys. Rev. E 77, 061108 (2008)
Kamiya, Y., Kato, Y., Nasu, J., Motome, Y.: Magnetic three states of matter: a quantum Monte Carlo study of spin liquids. Phys. Rev. B 92, 100403(R) (2015)
Friedan, D., Qiu, Z., Shenker, S.: Superconformal invariance in two dimensions and the tricritical Ising model. Phys. Lett. B 151, 37 (1985)
Grover, T., Sheng, D.N., Vishwanath, A.: Emergent space-time supersymmetry at the boundary of a topological phase. Science 344(6181), 280 (2014)
Balian, R., Toulouse, G.: Critical exponents for transitions with \(n=-2\) components of the order parameter. Phys. Rev. Lett. 30, 544 (1973)
Lawler, G.F., Schramm, O., Werner, W.: Conformal invariance of planar loop-erased random walks and uniform spanning trees. Ann. Probab. 32, 939 (2004)
Liu, Q., Deng, Y., Garoni, T.M., Blöte, H.W.J.: The O(n) loop model on a three-dimensional lattice. Nucl. Phys. B 859, 107 (2012)
Guida, R., Zinn-Justin, J.: Critical exponents of the N-vector model. J. Phys. A 31, 8103 (1998)
Clisby, N.: Accurate estimate of the critical exponent \(\nu \) for self-avoiding walks via a fast implementation of the pivot algorithm. Phys. Rev. Lett. 104, 055702 (2010)
Fedorenko, A.A., Le Doussal, P., Wiese, K.J.: Field theory conjecture for loop-erased random walks. J. Stat. Phys. 133, 805 (2008)
Wilson, D.B.: Dimension of the loop-erased random walk in three dimensions. Phys. Rev. E 82, 062102 (2010)
Gliozzi, F.: Constraints on conformal field theories in diverse dimensions from the bootstrap mechanism. Phys. Rev. Lett. 111, 161602 (2013)
El-Showk, S., Paulos, M.: Extremal bootstrapping: go with the flow. arXiv:1605.08087
Maldacena, J., Zhiboedov, A.: Constraining conformal field theories with a higher spin symmetry. J. Phys. A 46, 214011 (2013)
El-Showk, S., Paulos, M., Poland, D., Rychkov, S., Simmons-Duffin, D., Vichi, A.: Conformal field theories in fractional dimensions. Phys. Rev. Lett. 112, 141601 (2014)
Hogervorst, M., Rychkov, S., van Rees, B.C.: Unitarity violation at the Wilson-Fisher fixed point in 4-epsilon dimensions. Phys. Rev. D 93, 125025 (2016)
Kennedy, T.: Conformal invariance of the 3D self-avoiding walk. Phys. Rev. Lett. 111, 165703 (2013)
Itzykson, C., Drouffe, J.: Interacting fields and random walks, chap. 1.2.2. In: Statistical Field Theory. Cambridge University Press, Cambridge (1989)
Parisi, G.: Particle-field duality, chap. 16. In: Statistical field theory. Addison-Wesley, (1988)
Feynman, R.P.: Order–disorder theory, chap. 5. In: Statistical Mechanics: A Set of Lectures. Westview Press, Boulder (1998)
Kiskis, J., Narayanan, R., Vranas, P.: The Hausdorff dimension of random walks and the correlation length critical exponent in Euclidean field theory. J. Stat. Phys. 73, 765 (1993)
Hikami, S., Abe, R.: Crossover exponent of the spin anisotropic n-vector model with short range interaction in \(1/n\) expansion. Prog. Theor. Phys. 52, 369 (1973)
Cardy, J.: Scaling and Renormalization in Statistical Physics, chap. 3.3. Cambridge University Press, Cambridge (1996)
Nienhuis, B.: Exact critical point and critical exponents of \(O(n)\) models in two dimensions. Phys. Rev. Lett. 49, 1062 (1982)
Di Francesco, P., Saleur, H., Zuber, J.B.: Relations between the Coulomb gas picture and conformal invariance of two-dimensional critical models. J. Stat. Phys. 49, 57 (1987)
Guttmann, A.J., Bursill, R.J.: Critical exponent for the loop erased self-avoiding walk by Monte Carlo methods. J. Stat. Phys. 59, 1 (1990)
Agrawal, H., Dhar, D.: Distribution of sizes of erased loops of loop-erased random walks in two and three dimensions. Phys. Rev. E 63, 056115 (2001)
Grassberger, P.: Scaling of loop-erased walks in 2 to 4 dimensions. J. Stat. Phys. 136, 399 (2009)
Rychkov, S.: Conformal bootstrap in three dimensions? arXiv:1111.2115
Flory, P.J.: Principles of Polymer Chemistry. Cornell University Press, Ithaca (1967)
Antonenko, S.A., Sokolov, A.I.: Critical exponents for a three-dimensional \(O(n)\)-symmetric model with \(n>3\). Phys. Rev. E 51, 1894 (1995)
Simmons-Duffin, D.: A semidefinite program solver for the conformal bootstrap. JHEP 06, 174 (2015)
Kos, F., Poland, D., Simmons-Duffin, D., Vichi, A.: Bootstrapping the \(O(N)\) archipelago. JHEP 11, 106 (2015)
Rattazzi, R., Rychkov, S., Vichi, A.: Bounds in 4D conformal field theories with global symmetry. J. Phys. A 44, 035402 (2011)
Vichi, A.: Improved bounds for CFT’s with global symmetries. JHEP 1201, 162 (2012)
Poland, D., Simmons-Duffin, D., Vichi, A.: Carving out the space of 4D CFTs. JHEP 1205, 110 (2012)
Hogervorst, M., Rychkov, S.: Radial coordinates for conformal blocks. Phys. Rev. D 87, 106004 (2013)
Cha, M.C., Fisher, M.P.A., Girvin, S.M., Wallin, M., Young, A.P.: Universal conductivity of two-dimensional films at the superconductor-insulator transition. Phys. Rev. B 44, 6883 (1991)
Huh, Y., Strack, P., Sachdev, S.: Erratum: Conserved current correlators of conformal field theories in 2+1 dimensions. Phys. Rev. B 88, 155109, : Phys. Rev. B 90(2014), 199902 (2013)
Katz, E., Sachdev, S., Sorensen, E.S., Witczak-Krempa, W.: Conformal field theories at nonzero tempeature: operator product expansions, Monte Carlo, and holography. Phys. Rev. B 90, 245109 (2014)
Vilasis, X.: Renormalisation group flows and conserved vector currents. Nucl. Phys. B 435, 735 (1995)
Zamolodchikov, A.B.: Irreversibility of the flux of the renormalization group in a 2D field theory. JETP Letter 43, 730 (1986)
Moshe, M., Zinn-Justin, J.: Quantum field theory in the large N limit: a review. Phys. Rep. 385, 69 (2003)
Nakyama, Y., Ohtsuki, T.: Five dimensional \(O(N)\)-symmetric CFTs from conformal bootstrap. Phys. Lett. B 734, 193 (2014)
Ferrara, S., Gatto, R., Grillo, A.F.: Positivity restrictions on anomalous dimensions. Phys. Rev. D 9, 3564 (1974)
Mack, G.: All unitary ray representations of the conformal group SU(2,2) with positive energy. Commun. Math. Phys. 55, 1 (1977)
Metsaev, R.R.: Massless mixed symmetry bosonic free fields in d-dimensional anti-de Sitter space-time. Phys. Lett. B 354, 78 (1995)
Minwalla, S.: Restrictions imposed by superconformal invariance on quantum field theories. Adv. Theor. Math. Phys. 2, 781 (1998)
Paulos, M.F.: JuliBootS: a hands-on guide to the conformal bootstrap. arXiv:1412.4127
Shimada, H.: Disordered \(O(n)\) loop model and coupled conformal field theories. Nucl. Phys. B 820, 707 (2009)
Bobev, N., El-Showk, S., Mazac, D., Paulos, M.F.: Bootstrapping the three dimensional supersymmetric Ising model. Phys. Rev. Lett. 115, 051601 (2015)
Saleur, H.: Polymers and percolation in two dimensions and twisted \(N=2\) supersymmetry. Nucl. Phys. B 382, 486 (1992)
Parisi, G., Sourlas, N.: Self-avoiding walk and supersymmetry. J. Phys. Lett. 41, L403 (1980)
Calabrese, P., Pelissetto, A., Vicari, E.: The critical behavior of magnetic systems described by Landau–Ginzburg-Wilson field theories. arXiv: cond-mat/0306273
Brezin, E., Zinn-Justin, J., Le Guillou, J.C.: Anomalous dimensions of composite operators near two dimensions for ferromagnets with \(O(n)\) symmetry. Phys. Rev. B 14, 4976 (1976)
Echeverri, A.C., von Harling, B., Serone, M.: The effective bootstrap. arXiv:1606.02771
Pelissetto, A., Vicari, E.: Critical phenomena and renormalization-group theory. Phys. Rep. 368, 549 (2002)
Clisby, N., Conway, A.R., Guttmann, A.J.: Three-dimensional terminally attached self-avoiding walks and bridges. J. Phys. A 49, 015004 (2015)
Calabrese, P., Pelissetto, A., Vicari, E.: Critical structure factors of bilinear fields in \(O(N)\) vector models. Phys. Rev. E 65, 046115 (2002)
Bashkirov, D.: Bootstrapping the \(\cal{N}=1\) SCFT in three dimensions. arXiv:1310.8255
Iliesiu, L., Kos, F., Poland, D., Pufu, S.S., Simmons-Duffin, D., Yacoby, R.: Bootstrapping 3D fermions. arXiv:1508.00012
Kato, Y., Misawa, T.: Quantum tricriticality in antiferromagnetic Ising model with transverse field:a quantum Monte Carlo study. Phys. Rev. B 92, 17441 (2015)
Riedel, E.K., Wegner, F.: Tricritical exponents and scaling fields. Phys. Rev. Lett. 29, 349 (1972)
Blume, M.: Theory of the first-order magnetic phase change in UO\(_2\). Phys. Rev. 141, 517 (1966)
Capel, H.W.: On the possibility of first-order phase transitions in Ising systems of triplet ions with zero-field splitting. Physica 32, 966 (1966)
Liendo, P., Rastelli, L., van Rees, B.C.: The bootstrap program for boundary \(CFT_d\). JHEP 07, 113 (2013)
Hellwig, T., Wipf, A., Zanusso, O.: Scaling and superscaling solutions from the functional renormalization group. Phys. Rev. D 92, (2015) 085027. Phys. Rev. Lett. 111, 161602 (2013)
Burkhardt, T.W., Knops, H.J.F.: Renormalization-group results for the Blume-Capel model in two and three dimensions. Phys. Rev. B 15, 1602 (1977)
Gracey, J.A.: Critical exponents for the supersymmetric sigma-model. J. Phys. A 23, 2183 (1990)
Hikami, S.: Localization, nonlinear sigma model and string theory. Prog. Theor. Phys. Suppl. 107, 213 (1992)
Fei, L., Giombi, S., Klebanov, I.R., Tarnopolsky, G.: Yukawa CFTs and Emergent Supersymmetry. arXiv:1607.05316
Cardy, J.: Logarithmic conformal field theories as limits of ordinary CFTs and some physical applications. J. Phys. A 46, 494001 (2013)
Cardy, J.: Logarithmic correlations in quenched random magnets and polymers.arXiv:cond-mat/9911024
Vasseur, R., Jacobsen, J.L.: Operator content of the critical Potts model in d dimensions and logarithmic correlations. Nucl. Phys. B 880, 435 (2014)
Kos, F., Poland, D., Simmons-Duffin, D., Vichi, A.: Precision Islands in the Ising and \(O (N)\) models. arXiv:1603.04436
Dotsenko, V.S., Fateev, V.A.: Conformal algebra and multipoint correlation functions in 2D statistical models. Nucl. Phys. B 240, 312 (1984)
Leigh, R.G., Petkou, A.C.: Singleton deformation of higher-spin theory and the phase structure of the three-dimensional \(O(N)\) vector model. Phys. Rev. D 88, 046006 (2013)
Prokof’ev, N., Svistunov, B.: Comment on “Hausdorff dimension of critical fluctuations in abelian gauge theories”. Phys. Rev. Lett. 96, 219701 (2006)
Le Guillou, J.C.: Zinn-Justin, critical exponents from field theory. J Phys. Rev. B 21, 3976 (1980)
Calabrese, P., Parruccini, P.: Harmonic crossover exponents in \(O(n)\) models with the pseudo-\(\epsilon \) expansion approach. Phys. Rev. B 71, 064416 (2005)
Kleinert, H., Schulte-Frohlinde, V.: Exact five-loop renormalization group functions of \(\phi ^4\)-theory with O(N)-symmetric and cubic interactions. Critical exponents up to \(\epsilon ^5\). Phys. Lett. B 342, 284 (1995)
Kirkham, J.E.: Calculation of crossover exponent from Heisenberg to Ising behaviour using the fourth-order \(\epsilon \) expansion. J. Phys. A 14, L437 (1981)
Acknowledgements
The work of H. S. is supported by JSPS KAKENHI Grant-in-Aid 15K13540. S. H. and H. S. are supported by JSPS KAKENHI Grant-in-Aid 16K05491. We thank Édouard Brézin, Ferdinando Gliozzi, Yasuyuki Kato, Yoshitomo Kamiya, Shinsuke Kawai, Andreas Läuchli, Jonathan Miller, Yu Nakayama, Tassos Petkou, Hirotaka Sugawara, and Slava Rychkov for valuable discussions and correspondences. We thank useful comments from Nikolay Bobev, Giacomo Gori, Matthijs Hogervorst, Jesper Jacobsen, Tomoki Ohtsuki, Miguel Paulos, Marco Serone, Andrea Trombettoni, and Alessandro Vichi. We thank the Galileo Galilei Institute for Theoretical Physics for the hospitality and the INFN for partial support during the completion of this work.
Author information
Authors and Affiliations
Corresponding author
Computations on the N-derivatives by the Fixed Dimension RG
Computations on the N-derivatives by the Fixed Dimension RG
We use the fixed dimension RG (\(D=3\)) augmented by the pseudo-\(\epsilon \) series [89] to circumvent the accumulation of the intermediate systematic error due to the determination of the coupling \(g^*\) at the non-trivial fixed point. The beta function is generalized by a new parameter \(\tau \) such that at \(\tau =1\) it reduces to the original beta function \(\beta (g)\) in 3D:
where \(\beta _2(g)\equiv \beta (g)+g\) starts at order \(g^2\) with a positive coefficient of order 1. Then the critical exponents can be expanded in \(\tau \) by eliminating g by using \(g=g^*(\tau )\) which solves \(\beta (g^*,\tau )=0\). We compute the \(\tau \)-series for the derivatives of \(\Delta _S\) and \(\Delta _T\) at the degeneration point (\(N=0\)) based on the six-loop 3D RG results for \(\beta (g)\), \(\eta \), \(\gamma ^{-1}\) [41] and \(\eta _T=\eta +\phi _2/\nu -2\) [69]. The results are,Footnote 21
where we also show the derivatives computed from \(\epsilon =4-d\) expansion up to known orders [91, 92] just for comparison. Since both \(\tau \)-series do not show strong asymptotic behaviors with factorial growth of coefficients up to the orders presented, even the naive direct summation of the series would be of some use; in particular, it is clearly better than the direct sum of the \(\epsilon \)-expansion.
A simple Padé analysis, however, may improve the stability of analysis as usual. This can be irrustrated as follows. We show the values from the Padé approximants [M / L] for these derivatives in Tables 5 and 6, respectively. The positive real poles closest to \(\tau =1\) are shown in brackets for the six-loops (anti-diagonals \(L+M=6\)) and five-loops (\(L+M=5\)) order approximants. For each derivative, the data occuring with a pole in [0.5, 1.5] (indicated by \(^*\)) is omitted since it is rather close to \(\tau =1\), where the series is to be evaluated. As a simple estimate, we take the average of the six and five-loops and the maximum deviation as an error. This gives the value quoted in (10) and (11) in the text.
Rights and permissions
About this article
Cite this article
Shimada, H., Hikami, S. Fractal Dimensions of Self-Avoiding Walks and Ising High-Temperature Graphs in 3D Conformal Bootstrap. J Stat Phys 165, 1006–1035 (2016). https://doi.org/10.1007/s10955-016-1658-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10955-016-1658-x