Skip to main content
SpringerLink
Log in

Virtual Element Method for Control Constrained Dirichlet Boundary Control Problem Governed by the Diffusion Problem

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

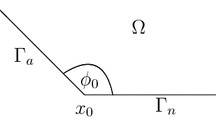
This article develops a conforming virtual element method for a control-constrained Dirichlet boundary optimal control problem governed by the diffusion problem. An energy-based cost functional is used to approximate the control problem which results in a smooth control in contrast to the \(L^2(\Gamma )\) approach which can lead to a control with discontinuities at the corners (Gong in SIAM J Numer Anal 60:450-474, 2022) . We use virtual element discretization of control, state, and adjoint variables along with a discretize-then-optimize approach to compute the optimal control is used to solve the problem. A new framework for the a priori error analysis is presented, which is optimal up to the regularity of the continuous solution. A primal-dual algorithm is used to solve the Dirichlet optimal control problem, and numerical experiments are conducted to illustrate the theoretical findings on general polygonal meshes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Data Availability

Enquiries about data availability should be directed to the authors.

References

  1. Gong, W., Mateos, M., Singler, J., Zhang, Y.: Analysis and approximations of Dirichlet boundary control of stokes flows in the energy space. SIAM J. Numer. Anal. 60(1), 450–474 (2022)

    MathSciNet  Google Scholar 

  2. Beirão da Veiga, L., Brezzi, F., Cangiani, A., Manzini, G., Marini, L.D., Russo, A.: Basic principles of virtual element methods. Math. Models Methods Appl. Sci. 23(01), 199–214 (2013)

    MathSciNet  Google Scholar 

  3. Beirão da Veiga, L., Brezzi, F., Marini, L.D., Russo, A.: The hitchhiker’s guide to the virtual element method. Math. Models Methods Appl. Sci. 24(08), 1541–1573 (2014)

    MathSciNet  Google Scholar 

  4. Beirão da Veiga, L., Lovadina, C., Russo, A.: Stability analysis for the virtual element method. Math. Models Methods Appl. Sci. 27(13), 2557–2594 (2017)

    MathSciNet  Google Scholar 

  5. Ahmad, B., Alsaedi, A., Brezzi, F., Marini, L.D., Russo, A.: Equivalent projectors for virtual element methods. Comput. Math. Appl. 66(03), 376–391 (2013)

    MathSciNet  Google Scholar 

  6. Beirão da Veiga, L., Brezzi, F., Marini, L., Russo, A.: Virtual element method for general second-order elliptic problems on polygonal meshes. Math. Models Methods Appl. Sci. 26(04), 729–750 (2016)

    MathSciNet  Google Scholar 

  7. Cangiani, A., Manzini, G., Sutton, O.J.: Conforming and nonconforming virtual element methods for elliptic problems. IMA J. Numer. Anal. 37(03), 1317–1354 (2016)

    MathSciNet  Google Scholar 

  8. Vacca, G., Beirão da Veiga, L.: Virtual element methods for parabolic problems on polygonal meshes. Numer. Methods Part. Differ. Equ. 31(6), 2110–2134 (2015)

    MathSciNet  Google Scholar 

  9. Vacca, G.: Virtual element methods for hyperbolic problems on polygonal meshes. Comput. Math. Appl. 74(05), 882–898 (2017)

    MathSciNet  Google Scholar 

  10. Ling, M., Wang, F., Han, W.: The nonconforming virtual element method for a stationary stokes hemivariational inequality with slip boundary condition. J. Sci. Comput. 85(03), 1–19 (2020)

    MathSciNet  Google Scholar 

  11. Da Veiga, L.B., Lovadina, C., Vacca, G.: Virtual elements for the Navier-stokes problem on polygonal meshes. SIAM J. Numer. Anal. 56(03), 1210–1242 (2018)

    MathSciNet  Google Scholar 

  12. Antonietti, P.F., Da Veiga, L.B., Mora, D., Verani, M.: A stream virtual element formulation of the stokes problem on polygonal meshes. SIAM J. Numer. Anal. 52(01), 386–404 (2014)

    MathSciNet  Google Scholar 

  13. Benedetto, M.F., Berrone, S., Pieraccini, S., Scialò, S.: The virtual element method for discrete fracture network simulations. Comput. Methods Appl. Mech. Eng. 280, 135–156 (2014)

    MathSciNet  Google Scholar 

  14. Wriggers, P., Rust, W.T., Reddy, B.D.: A virtual element method for contact. Comput. Mech. 58(06), 1039–1050 (2016)

    MathSciNet  Google Scholar 

  15. Gain, A.L., Talischi, C., Paulino, G.H.: On the virtual element method for three-dimensional linear elasticity problems on arbitrary polyhedral meshes. Comput. Methods Appl. Mech. Eng. 282, 132–160 (2014)

    MathSciNet  Google Scholar 

  16. Tushar, J., Kumar, A., Kumar, S.: Virtual element methods for general linear elliptic interface problems on polygonal meshes with small edges. Comput. Math. Appl. 122, 61–75 (2022)

    MathSciNet  Google Scholar 

  17. Cao, S., Chen, L., Guo, R.: A virtual finite element method for two-dimensional maxwell interface problems with a background unfitted mesh. Math. Models Methods Appl. Sci. 31, 1–30 (2021)

    MathSciNet  Google Scholar 

  18. Tröltzsch, F.: Optimal control of partial differential equations: theory, methods, and applications, vol. 112. American Mathematical Society, Rhode Island (2010)

    Google Scholar 

  19. Casas, E., Tröltzsch, F.: “Error estimates for linear-quadratic elliptic control problems,” in IFIP International Information Security Conference, pp. 89–100, Springer, (2002)

  20. Hinze, M.: A variational discretization concept in control constrained optimization: the linear-quadratic case. Comput. Optim. Appl. 30, 45–61 (2005)

    MathSciNet  Google Scholar 

  21. Meidner, D., Vexler, B.: A priori error estimates for space-time finite element discretization of parabolic optimal control problems part ii: problems with control constraints. SIAM J. Control Optim. 47(03), 1301–1329 (2008)

    MathSciNet  Google Scholar 

  22. Dond, A.K., Gudi, T., Sau, R.C.: An error analysis of discontinuous finite element methods for the optimal control problems governed by Stokes equation. Numer. Funct. Anal. Optim. 40(04), 421–460 (2019)

    MathSciNet  Google Scholar 

  23. Kumar, S., Ruiz Baier, R., Sandilya, R.: A priori error estimates for discontinuous finite volume Discretizations of the brinkman optimal control problem. J. Sci. Comput. 78, 64–93 (2015)

    Google Scholar 

  24. Wang, Q., Zhou, Z.: Adaptive virtual element method for optimal control problem governed by general elliptic equation. J. Sci. Comput. 88(01), 1–33 (2021)

    MathSciNet  Google Scholar 

  25. Tushar, J., Kumar, A., Kumar, S.: Variational and virtual discretizations of optimal control problems governed by diffusion problems. Appl. Math. Optim. 85(02), 1–36 (2022)

    MathSciNet  Google Scholar 

  26. Tushar, J., Kumar, A., Kumar, S.: Approximations of quasi-linear elliptic optimal control problems on polygonal meshes under variational and virtual discretizations. Int. J. Appl. Comput. Math. 8(01), 1–35 (2022)

    MathSciNet  Google Scholar 

  27. Tushar, J., Kumar, A., Kumar, S.: Mixed virtual element methods for optimal control of darcy flow. Comput. Math. Appl. 140, 134–153 (2023)

    MathSciNet  Google Scholar 

  28. Brenner, S.C., Sung, L.-Y., Tan, Z.: A \({C}^1\) virtual element method for an elliptic distributed optimal control problem with pointwise state constraints. Math. Models Methods Appl. Sci. 31(14), 2887–2906 (2021)

    MathSciNet  Google Scholar 

  29. Antil, H., Nochetto, R.H., Venegas, P.: Controlling the kelvin force: basic strategies and applications to magnetic drug targeting. Optim. Eng. 19(03), 559–589 (2018)

    MathSciNet  Google Scholar 

  30. John, C., Wachsmuth, D.: Optimal Dirichlet boundary control of stationary Navier-Stokes equations with state constraint. Numer. Funct. Anal. Optim. 30(11–12), 1309–1338 (2009)

    MathSciNet  Google Scholar 

  31. Deckelnick, K., Günther, A., Hinze, M.: Finite element approximation of Dirichlet boundary control for elliptic PDEs on two and three-dimensional curved domains. SIAM J. Control Optim. 48(04), 2798–2819 (2009)

    MathSciNet  Google Scholar 

  32. May, S., Rannacher, R., Vexler, B.: Error analysis for a finite element approximation of elliptic Dirichlet boundary control problems. SIAM J. Control Optim. 51(03), 2585–2611 (2013)

    MathSciNet  Google Scholar 

  33. Casas, E., Mateos, M., Raymond, J.-P.: Penalization of Dirichlet optimal control problems. ESAIM Control Optim. Calc. Var. 15(04), 782–809 (2009)

    MathSciNet  Google Scholar 

  34. Of, G., Phan, T.X., Steinbach, O.: An energy space finite element approach for elliptic Dirichlet boundary control problems. Numerische Mathematik 129(04), 723–748 (2015)

    MathSciNet  Google Scholar 

  35. Chowdhury, S., Gudi, T., Nandakumaran, A.K.: Error bounds for a Dirichlet boundary control problem based on energy spaces. Math. Comput. 86(305), 1103–1126 (2017)

    MathSciNet  Google Scholar 

  36. Gudi, T., Sau, R.C.: Finite element analysis of the constrained Dirichlet boundary control problem governed by the diffusion problem. ESAIM Control Optim. Calc. Var. 26, 1–19 (2020)

    MathSciNet  Google Scholar 

  37. Brenner, S.C., Guan, Q., Sung, L.-Y.: Some estimates for virtual element methods. Comput. Methods Appl. Math. 17(04), 553–574 (2017)

    MathSciNet  Google Scholar 

  38. Mora, D., Rivera, G., Rodríguez, R.: A virtual element method for the Steklov eigenvalue problem. Math. Models Methods Appl. Sci. 25(08), 1421–1445 (2015)

    MathSciNet  Google Scholar 

  39. Di Pietro, D. A., Droniou, J.: “The hybrid high-order method for polytopal meshes,” Number 19 in Modeling, Simulation and Application, (2020)

  40. Tushar, J., Khan, A., Mohan, M. T.: Optimal control of stationary doubly diffusive flows on two and three dimensional bounded lipschitz domains: a theoretical study. arXiv preprint arXiv:2308.02178 (2023)

  41. Drouet, G., Hild, P.: Optimal convergence for discrete variational inequalities modelling Signorini contact in 2D and 3D without additional assumptions on the unknown contact set. SIAM J. Numer. Anal. 53(03), 1488–1507 (2015)

    MathSciNet  Google Scholar 

  42. Ciarlet, P.G.: The finite element method for elliptic problems, vol. 40. SIAM, Philadelphia (2002)

    Google Scholar 

  43. Brenner, S., Scott, R.: The mathematical theory of finite element methods, vol. 15. Springer, Berlin (2007)

    Google Scholar 

  44. Auliac, S., Belhachmi, Z., Belgacem, F. Ben., Hecht, F.: Quadratic finite elements with non-matching grids for the unilateral boundary contact. ESAIM. Math. Model. Numer. Anal. 47(04), 1185–1203 (2013)

    MathSciNet  Google Scholar 

  45. Belhachmi, Z., Belgacem, F.B.: Quadratic finite element approximation of the Signorini problem. Math. Comput. 72(241), 83–104 (2003)

    MathSciNet  Google Scholar 

Download references

Acknowledgements

The third author is supported by the Department of Science and Technology (DST-SERB) India (grant number CRG/2019/003863). The first author would like to thank Dr. Rekha Khot for a helpful discussion and acknowledges BITS-Pilani, K K Birla Goa Campus where the majority of this work was carried out during his stay there.

Author information

Authors and Affiliations

  1. School of Mathematics, Monash University, Melbourne, Australia

    Jai Tushar

  2. Department of Mathematics, The Chinese University of Hong Kong, Hong Kong, China

    Ramesh Chandra Sau

  3. Department of Mathematics, BITS Pilani-KK Birla Goa Campus, Zuarinagar, Goa, India

    Anil Kumar

Authors
  1. Jai Tushar
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ramesh Chandra Sau
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Anil Kumar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jai Tushar.

Ethics declarations

Conflicts of interest

The authors have not disclosed any competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tushar, J., Sau, R.C. & Kumar, A. Virtual Element Method for Control Constrained Dirichlet Boundary Control Problem Governed by the Diffusion Problem. J Sci Comput 98, 21 (2024). https://doi.org/10.1007/s10915-023-02410-3

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-023-02410-3

Keywords

Mathematics Subject Classification

Search

Navigation