Skip to main content
SpringerLink
Log in

An energy space finite element approach for elliptic Dirichlet boundary control problems

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

Abstract

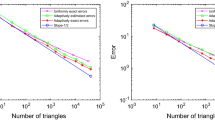
In this paper we present a finite element analysis for a Dirichlet boundary control problem where the Dirichlet control is considered in a convex closed subspace of the energy space \(H^{1/2}(\Gamma )\). As an equivalent norm in \(H^{1/2}(\Gamma )\) we use the energy norm induced by the so-called Steklov–Poincaré operator which realizes the Dirichlet to Neumann map, and which can be implemented by using standard finite element methods. The presented stability and error analysis of the discretization of the resulting variational inequality is based on the mapping properties of the solution operators related to the primal and adjoint boundary value problems, and their finite element approximations. Some numerical results are given, which confirm on one hand the theoretical estimates, but on the other hand indicate the differences when modelling the control in \(L_2(\Gamma )\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Adams, R.A.: Sobolev Spaces. Academic Press, New York (1975)

    MATH  Google Scholar 

  2. Arkhipova, A.A., Uraltseva, N.N.: Regularity of the solution of a problem with a two-sided constraint on the boundary for elliptic and parabolic equations. Proc. Steklov Inst. Math. 2, 1–19 (1989)

    Google Scholar 

  3. Ben Belgacem, F., El Fekih, H., Metoui, H.: Singular perturbations for the Dirichlet boundary control of elliptic problems. Math. Model. Numer. Anal. 37, 833–850 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  4. Berggren, M.: Approximations of very weak solutions to boundary value problems. SIAM J. Numer. Anal. 42, 860–877 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  5. Brenner, S., Scott, R.L.: The Mathematical Theory of Finite Element Methods. Springer, New York (1994)

    Book  MATH  Google Scholar 

  6. Brezis, H.: Problémes unilateraux. J. Math. Pures Appl. 51, 1–168 (1972)

    MATH  MathSciNet  Google Scholar 

  7. Brezzi, F., Hager, W.W., Raviart, P.A.: Error estimates for the finite element solution of variational inequalities. Numer. Math. 28, 431–443 (1977)

    Article  MATH  MathSciNet  Google Scholar 

  8. Casas, E., Raymond, J.P.: Error estimates for the numerical approximation of Dirichlet boundary control for semilinear elliptic equations. SIAM J. Control Optim. 45, 1586–1611 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  9. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. North-Holland, Amsterdam (1978)

    MATH  Google Scholar 

  10. Deckelnick, K., Günther, A., Hinze, M.: Finite element approximation of Dirichlet boundary control for elliptic PDEs on two- and three-dimensional curved domains. SIAM J. Control Optim. 48, 2798–2819 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  11. Falk, R.S.: Error estimates for the approximation of a class of variational inequalities. Math. Comput. 28, 963–971 (1974)

    Article  MATH  MathSciNet  Google Scholar 

  12. Fursikov, A.V., Gunzburger, M.D., Hou, L.S.: Boundary value problems and optimal boundary control for the Navier-Stokes system: the two-dimensional case. SIAM J. Control Optim. 36, 852–894 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  13. Glowinski, R.: Numerical Methods for Nonlinear Variational Problems. Springer, Berlin (1980)

    Google Scholar 

  14. Gunzburger, M.D., Hou, L.S., Swobodny, T.: Analysis and finite element approximation of optimal control problems for the stationary Navier–Stokes equations with Dirichlet controls. Math. Model. Numer. Anal. 25, 711–748 (1991)

    MATH  Google Scholar 

  15. Gunzburger, M.D., Hou, L., Svobodny, T.P.: Boundary velocity control of incompressible flow with an application to viscous drag reduction. SIAM J. Control Optim. 30, 167–181 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  16. Hinze,M., Pinnau,R., Ulbrich,M., Ulbrich,S.: Optimization with PDE Constraints. Mathematical Modelling: Theory and Applications, vol. 23. Springer, Heidelberg (2009)

  17. Holt, L.S., Ravindran, S.S.: Penalty methods for numerical approximations of optimal boundary flow control problems. Int. J. Comput. Fluid Dyn. 11, 157–167 (1998)

    Article  MathSciNet  Google Scholar 

  18. Hoppe, R.H.W.: Multigrid algorithms for variational inequalities. SIAM J. Numer. Anal. 24, 1046–1065 (1987)

    Article  MATH  MathSciNet  Google Scholar 

  19. Hou, L.S., Ravindran, S.S.: A penalized Neumann control approach for solving an optimal Dirichlet control problem for the Navier-Stokes equations. SIAM J. Control Optim. 36, 1795–1814 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  20. Hou, L.S., Ravindran, S.S.: Numerical approximation of optimal flow control problems by a penalty method: error estimates and numerical results. SIAM J. Sci. Comput. 20, 1753–1777 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  21. Hou, L.S., Svobodny, T.P.: Optimization problems for the Navier–Stokes equations with regular boundary controls. J. Math. Anal. Appl. 177, 342–367 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  22. Hsiao, G.C., Wendland, W.L.: Boundary Integral Equations. Springer, Heidelberg (2008)

    Book  MATH  Google Scholar 

  23. Ito, K., Kunisch, K.: Semi-smooth Newton methods for the Signorini problem. Appl. Math. 53, 455–468 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  24. Kinderlehrer, D.: Remarks about Signorini’s problem in linear elasticity. Ann. Scuola Norm. Sup. Pisa cl. Sci 4, 605–645 (1981)

    MathSciNet  Google Scholar 

  25. Kunisch, K., Vexler, B.: Constrained Dirichlet boundary control in \(L^2\) for a class of evolution equations. SIAM J. Control Optim. 46, 1726–1753 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  26. Lee, H.-C., Kim, S.: Finite element approximation and computations of optimal Dirichlet boundary control problems for the Boussinesq equations. J. Korean Math. Soc. 41, 681–715 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  27. Lewy, H., Stampacchia, G.: On the regularity of the solution of a variational inequality. Commun. Pure Appl. Math. 22, 153–188 (1969)

    Article  MATH  MathSciNet  Google Scholar 

  28. Lions, J.L.: Optimal Control of Systems Governed by Partial Differential Equations. Springer, Berlin (1971)

    Book  MATH  Google Scholar 

  29. Lions, J.L., Stampacchia, G.: Variational inequalities. Commun. Pure Appl. Math. 20, 493–519 (1967)

    Article  MATH  MathSciNet  Google Scholar 

  30. May, S., Rannacher, R., Vexler, B.: Error analysis for a finite element approximation of elliptic Dirichlet boundary control problems. SIAM J. Control Optim. 51, 2585–2611 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  31. McLean, W.: Strongly Elliptic Systems and Boundary Integral Equations. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  32. Of, G., Phan, T.X., Steinbach, O.: Boundary element methods for Dirichlet boundary control problems. Math. Methods Appl. Sci. 33, 2187–2205 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  33. Of, G., Phan, T.X., Steinbach, O.: Finite and boundary element energy approximations of Dirichlet control problems. In: Bock, H.G., Hoang, X.P., Rannacher, R., Schlöder, J.P. (eds.) Modeling, Simulation and Optimization of Complex Processes. Proceedings of the Fourth International Conference on High Performance Scientific Computing, March 2–6, 2009, Hanoi. Springer, Heidelberg, pp. 219–231 (2012)

  34. Schöberl, J.: Solving the Signorini problem on the basis of domain decomposition techniques. Computing 60, 323–344 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  35. Scott, R.L., Zhang, S.: Finite element interpolation of nonsmooth functions satisfying boundary conditions. Math. Comput. 54, 483–493 (1990)

    Article  MATH  MathSciNet  Google Scholar 

  36. Steinbach, O.: Stability estimates for hybrid coupled domain decomposition methods. Lecture Notes in Mathematics, vol. 1809. Springer, Heidelberg (2003)

  37. Steinbach, O.: Numerical Approximation Methods for Elliptic Boundary Value Problems. Finite and Boundary Elements. Springer, New York (2008)

    Book  MATH  Google Scholar 

  38. Steinbach, O.: Boundary element methods for variational inequalities. Numer. Math. 126, 173–197 (2014)

    Article  MATH  MathSciNet  Google Scholar 

  39. Strang, G., Fix, G.J.: An Analysis of the Finite Element Method. Prentice Hall, Englewood Cliffs (1973)

    MATH  Google Scholar 

  40. Tartar, L. : An Introduction to Sobolev Spaces and Interpolation Spaces. Lecture Notes of the Unione Matematica Italiana, vol. 3. Springer, Berlin (2007)

  41. Tröltzsch, F.: Optimale Steuerung partieller Differentialgleichungen. Anwendungen. Vieweg, Braunschweig, Theorie, Verfahren (2005)

  42. Ulbrich, M.: Semismooth Newton methods for operator equations in function spaces. SIAM J. Optim. 13, 805–841 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  43. Uraltseva, N.N.: Regularity of solutions of variational inequalities. Russ. Math. Surv. 42, 191–219 (1987)

    Article  MathSciNet  Google Scholar 

  44. Vexler, B.: Finite element approximation of elliptic Dirichlet optimal control problems. Numer. Funct. Anal. Optim. 28, 957–973 (2007)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Acknowledgments

This work has been supported by the Austrian Science Fund (FWF) under the Grant SFB Mathematical Optimisation and Applications in Biomedical Sciences, Subproject Fast Finite Element and Boundary Element Methods for Optimality Systems. The authors would like to thank K. Kunisch, A. Rösch, F. Tröltzsch, B. Vexler, and W. Zulehner for many helpful discussions.

Author information

Authors and Affiliations

  1. Institut für Numerische Mathematik, TU Graz, Steyrergasse 30, 8010 , Graz, Austria

    G. Of & O. Steinbach

  2. School of Applied Mathematics and Informatics, Hanoi University of Science and Technology, No. 1 Dai Co Viet, Hanoi, Vietnam

    T. X. Phan

Authors
  1. G. Of
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. T. X. Phan
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. O. Steinbach
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to O. Steinbach.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Of, G., Phan, T.X. & Steinbach, O. An energy space finite element approach for elliptic Dirichlet boundary control problems. Numer. Math. 129, 723–748 (2015). https://doi.org/10.1007/s00211-014-0653-x

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-014-0653-x

Mathematics Subject Classification

Search

Navigation