Optimal Spectral Schemes Based on Generalized Prolate Spheroidal Wave Functions of Order $-1$

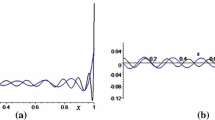
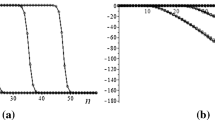
Abstract

We introduce a family of generalized prolate spheroidal wave functions (PSWFs) of order $-1,$ and develop new spectral schemes for second-order boundary value problems. Our technique differs from the differentiation approach based on PSWFs of order zero in Kong and Rokhlin (Appl Comput Harmon Anal 33(2):226–260, 2012); in particular, our orthogonal basis can naturally include homogeneous boundary conditions without the re-orthogonalization of Kong and Rokhlin (2012). More notably, it leads to diagonal systems or direct “explicit” solutions to 1D Helmholtz problems in various situations. Using a rule optimally pairing the bandwidth parameter and the number of basis functions as in Kong and Rokhlin (2012), we demonstrate that the new method significantly outperforms the Legendre spectral method in approximating highly oscillatory solutions. We also conduct a rigorous error analysis of this new scheme. The idea and analysis can be extended to generalized PSWFs of negative integer order for higher-order boundary value and eigenvalue problems.

Appendix 1: Proof of Lemma 4.1

We first recall the following bound in [12, Appendix].

Proposition 4.1

Let $q_n$ be defined as in (4.1). If $q_n\le 1$, then for all positive integer k such that $k(k+3)\le \chi _n^{(1)}(c)$ and $n+k$ is even, we have

$$\begin{aligned} |\partial _x^k\psi _n^{(1)}(0)|\le \sqrt{2}\left( \chi _n^{(1)}(c)\right) ^{k/2}. \end{aligned}$$

(4.26)

Note that if $n+k$ is odd, then $\partial _x^k\psi _n^{(1)}(0)=0.$

Proposition 4.2

Let $\{\beta _k^n\}$ be defined in (2.16). For given $c>0$ and $n\in {\mathbb N},$ let

$$\begin{aligned} k(k+3)+c^2\le \chi _n^{(1)}(c). \end{aligned}$$

(4.27)

We have

• (i) If both n and k are even, then $\beta _{0}^n, \beta _2^n, \beta _4^n,\ldots$ have the same sign, and $|\beta _k^n|\le |\beta _{k+2}^n|$ for all $k=0,2,4,\ldots ,$ satisfying (4.27);

• (ii) If both n and k are odd, then $\beta _{1}^n, \beta _3^n, \beta _5^n,\ldots$ have the same sign, and $|\beta _k^n|\le |\beta _{k+2}^n|$ for all $k=1,3,5,\ldots ,$ satisfying (4.27).

Note that if $n+k$ is odd, then $\beta _{k}^n=0.$

Proof

From (2.16) and the parity of $\psi _n^{(1)}(x)$ and $P_k^{(1)}(x),$ we have $\beta _{k}^n=0,$ if $n+k$ is odd.

We first justify the statement (i). By (2.18),

$$\begin{aligned} \beta _{k+2}^n=\frac{1}{F(k+2)c^2}\left\{ \left( \chi _n^{(1)}(c)-k(k+3)-G(k)c^2\right) \beta _{k}^n-{F({{k}}) c^2}\beta _{k-2}^n\right\} ,\;\;\; k\ge 0, \end{aligned}$$

(4.28)

with $\beta _{-2}^n=\beta _{-1}^n=0$, where

$$\begin{aligned} F(k)=\sqrt{\frac{(k-1)k(k+1)(k+2)}{(2k-1)(2k+1)^2(2k+3)}},\quad G(k)=\frac{2k(k+3)+1}{(2k+1)(2k+5)}. \end{aligned}$$

(4.29)

One verifies that for $k\ge 2$,

$$\begin{aligned} \frac{1}{5}<\frac{(k-1)(k+2)}{(2k+1)^2}<F(k)=\sqrt{\frac{k(k+1)}{(2k+1)^2}}\sqrt{\frac{(k-1)(k+2)}{(2k-1)(2k+3)}}<\sqrt{\frac{1}{4}}\sqrt{\frac{1}{4}}=\frac{1}{4}, \end{aligned}$$

(4.30)

and for $k\ge 1,$

$$\begin{aligned} \frac{2}{5}\le \frac{k}{2k+1}<\frac{2k(k+3)}{(2k+1)(2k+5)}<G(k)<\frac{(2k+1)(k+\frac{5}{2})}{(2k+1)(2k+5)}=\frac{1}{2}. \end{aligned}$$

(4.31)

We proceed with the proof by induction. For $k=0$, we find from (4.28) that

$$\begin{aligned} \begin{aligned} \beta _{2}^n=\frac{5}{2c^2}\sqrt{\frac{7}{2}}\left( \chi _n^{(1)}-\frac{c^2}{5}\right) \beta _{0}^n. \end{aligned} \end{aligned}$$

(4.32)

It is evident that with a looser condition than (4.27), the factor in front of $\beta _{0}^n$ is positive, so $\beta _2^n$ has the same sign as $\beta _0^n.$ Moreover, by (4.27) with $k=0,$

$$\begin{aligned} \begin{aligned}&|\beta _{2}^n|=\frac{5}{2c^2}\sqrt{\frac{7}{2}}\left( \chi _n^{(1)}-\frac{c^2}{5}\right) |\beta _{0}^n|\ge 2\sqrt{\frac{7}{2}}|\beta _{0}^n|\ge |\beta _{0}^n|, \end{aligned} \end{aligned}$$

(4.33)

We next assume that $\beta _{k-2}^n\beta _k^n>0$ and $|\beta _{k-2}^n|\le |\beta _k^n|$ for all $k\ge 2.$ Then we derive from (4.28), (4.30)–(4.31) and (4.27) that

$$\begin{aligned} \begin{aligned} \beta _{k}^n\beta _{k+2}^n&=\frac{1}{F(k+2)c^2}\left\{ \left( \chi _n^{(1)}(c)-k(k+3)-G(k)c^2\right) (\beta _{k}^n)^2-{F({{k}}) c^2}\beta _{k-2}^n\beta _{k}^n\right\} \\&\ge \frac{1}{F(k+2)c^2}\left\{ \chi _n^{(1)}(c)-k(k+3)-G(k)c^2-F({{k}})c^2\right\} (\beta _{k}^n)^2\\> \frac{1}{F(k+2)c^2}\left\{ \chi _n^{(1)}(c)-k(k+3)-\frac{3}{4} c^2\right\} (\beta _{k}^n)^2>0, \end{aligned} \end{aligned}$$

(4.34)

which implies $\beta _{k+2}^n\,\beta _k^n>0.$ Now, we show that $|\beta _{k}^n|\le |\beta _{k+2}^n|$. We show this by contradiction. Assuming that $|\beta _{k}^n|>|\beta _{k+2}^n|,$ we find from (4.34) and (4.30) that

$$\begin{aligned} \begin{aligned} 0> \frac{1}{F(k+2)c^2}\left\{ \chi _n^{(1)}(c)-k(k+3)-\frac{3}{4} c^2\right\} |\beta _{k}^n|-|\beta _{k+2}^n|\\>\frac{1}{F(k+2)c^2}\left\{ \chi _n^{(1)}-k(k+3)-\frac{3c^2}{4}-F(k+2)c^2\right\} |\beta _{k}^n|\\&>\frac{1}{F(k+2)c^2}\left\{ \chi _n^{(1)}-k(k+3)-c^2\right\} |\beta _{k}^n|, \end{aligned} \end{aligned}$$

(4.35)

which contradicts to (4.27). Thus, we have $|\beta _{k}^n|\le |\beta _{k+2}^n|$ for even n and k.

The statement (ii) can be justified similarly. In fact, the derivations in (4.34)–(4.35) also hold for odd n, k,  so it suffices to verify the initial of the induction. Like (4.32)–(4.33), we can show that

$$\begin{aligned} \beta _{3}^n=\frac{7}{2c^2}\sqrt{\frac{3}{2}}\left( \chi _n^{(1)}-4-\frac{3}{7}c^2\right) \beta _{1}^n, \end{aligned}$$

and

$$\begin{aligned} |\beta _{3}^n|=\frac{7}{2c^2}\sqrt{\frac{3}{2}} \left( \chi _n^{(1)}-4-\frac{3}{7}c^2\right) |\beta _{1}^n|\ge \sqrt{6}|\beta _{1}^n|\ge |\beta _{1}^n|, \end{aligned}$$

which implies $\beta _3^n$ has the same sign as $\beta _1^n$, and $|\beta _1^n|\le |\beta _3^n|.$ Then, we use (4.34)–(4.35) to complete the proof of Proposition A.2.$\square$

Proof of Lemma 4.1

With the above propositions, we are now ready to prove Lemma 4.1. $\square$

The bound for $\beta _0^n$ follows directly from (2.23) and (4.26) with $k=0.$

We carry out the proof by estimating the moment: $\int _{-1}^1t^m\psi _n^{(1)}(t)\omega _1(t)dt.$ Note that

$$\begin{aligned} t^j=\sum _{k=0}^j\hat{p}_{jk}P_k^{(1)}(t),\;\;\; \mathrm{where}\;\;\; \hat{p}_{jk}=\int _{-1}^1 t^j P_k^{(1)}(t)(1-t^2)dt, \end{aligned}$$

(4.36)

where we can find the formula of $\hat{p}_{jk}$ from [12, (16)-(17)], and have

$$\begin{aligned} \hat{p}_{jk}=0,\;\; \text {if } k+j\text { is odd};\;\;\; \hat{p}_{jk}>0,\;\; \text {if } k+j\text { is even};\;\;\; \hat{p}_{jj}=\frac{\sqrt{2\pi (2j+3)j!(j+2)!}}{2^{j+2}\Gamma (j+5/2)}. \end{aligned}$$

(4.37)

Thus, we obtain from (2.16) that

$$\begin{aligned} \int _{-1}^1t^j\psi _n^{(1)}(t)\omega _1(t)dt=\sum _{k=0}^j\hat{p}_{jk}\beta _k^n = {\left\{ \begin{array}{ll} 0,\quad &{}\text {if }n+j\text { is odd},\\ \displaystyle \sum _{k=0}^{j/2}\hat{p}_{j,2k}\,\beta _{2k}^n,\;\; &{} \text { if }n,j\text { are even},\\ {\displaystyle \sum _{k=0}^{{(j-1)}/2}} \hat{p}_{j, 2k+1}\beta _{2k+1}^n,\; &{} \text { if }n,j\text { are odd}, \end{array}\right. } \end{aligned}$$

(4.38)

where we used the property: $\beta _k^n=0$, if $k+n$ is odd.

On the other hand, taking the jth derivative at $x=0$ on both sides of (2.8) with $\alpha =1$, yields

$$\begin{aligned} \begin{aligned} \int _{-1}^1 t^j\,\psi _n^{(1)}(t)(1-t^2)\,dt&=(-1)^j\, \mathrm{i}^{n+j} c^{-j}\,\lambda _n^{(1)}(c)\,\partial _x^j\psi _n^{(1)}(0), \end{aligned} \end{aligned}$$

(4.39)

which vanishes, if $j+n$ is odd.

Thus, we have from Lemma A.2 (i), and (4.37)–(4.39) that for even n, j, 

$$\begin{aligned} \left| \displaystyle \sum _{k=0}^{j/2}\hat{p}_{j,2k}\,\beta _{2k}^n\right| =\displaystyle \sum _{k=0}^{j/2}\hat{p}_{j,2k}\,\left| \beta _{2k}^n\right| = c^{-j}\lambda _n^{(1)}(c)\,\left| \partial _x^j\psi _n^{(1)}(0)\right| , \end{aligned}$$

(4.40)

and further by (4.26),

$$\begin{aligned} \hat{p}_{jj} |\beta _j^n|\le \sum _{k=0}^{j/2}\hat{p}_{j,2k}\,\left| \beta _{2k}^n\right| =c^{-j}\lambda _n^{(1)}(c)|\partial _x^j\psi _n^{(1)}(0)|\le \sqrt{2}\left( \frac{1}{ \sqrt{q_n}}\right) ^{j}\lambda _n^{(1)}(c). \end{aligned}$$

(4.41)

Similarly, for odd n, j, 

$$\begin{aligned} \hat{p}_{jj} |\beta _j^n|\le \sum _{k=0}^{(j-1)/2}\hat{p}_{j,2k+1}\,\left| \beta _{2k+1}^n\right| =c^{-j}\lambda _n^{(1)}(c)|\partial _x^j\psi _n^{(1)}(0)|\le \sqrt{2}\left( \frac{1}{ \sqrt{q_n}}\right) ^{j}\lambda _n^{(1)}(c). \end{aligned}$$

(4.42)

Thus, by (4.37), and (4.3)–(4.4),

$$\begin{aligned} \hat{p}_{jj}^{-1}= \frac{2^{j+2}\sqrt{j}}{\sqrt{2\pi (2j+3)}}\sqrt{\Upsilon _j^{5/2,1}\Upsilon _j^{5/2,3}} \le \Upsilon _j^{5/2,1}\; \frac{2^{j+1}}{\sqrt{\pi }}. \end{aligned}$$

(4.43)

Then we obtain the bound (4.2).