We correct two theorems which provide criteria for strong attractors given in [1].

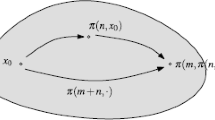
We use the same assumptions and notation as in [1], i.e. let \(\varphi \) be a continuous random dynamical system on a Polish space (E, d) over a metric dynamical system \((\Omega , {\mathscr {F}}, (\vartheta _t)_{t\in {\mathbb {R}}}, P)\). We use the same letter d for the complete metric on E and the Hausdorff semi-distance on subsets of E. For a subset A of E we denote the closed \(\delta \)-neighborhood of A by \(A^\delta \).

In the article the following two types of strong attractors are studied:

  • B-attractors, i.e. attractors that attract all bounded subsets of E,

  • C-attractors, i.e. attractors that attract all compact subsets of E.

In [1, Theorem 3.1, Theorem 3.2] the following two theorems have been stated:

FormalPara Theorem 1

(Original erroneous formulation) The following are equivalent:

  1. (i)

    \(\varphi \) has a strong B-attractor.

  2. (ii)

    For every \(\varepsilon >0\) there exists a compact subset \(C_\varepsilon \) such that for each \(\delta >0\) and each bounded and closed subset B of E it holds that

    $$\begin{aligned} P\left\{ \bigcup _{s\ge 0} \bigcap _{t\ge s} \varphi (t,\vartheta _{-t} \omega ) B \subseteq C_{\varepsilon }^{\delta } \right\} \ge 1 - \varepsilon . \end{aligned}$$
  3. (iii)

    There exists a compact strongly B-attracting set \(\omega \mapsto K(\omega )\).

FormalPara Theorem 2

(Original erroneous formulation) The following are equivalent:

  1. (i)

    \(\varphi \) has a strong C-attractor.

  2. (ii)

    For every \(\varepsilon >0\) there exists a compact subset \(C_\varepsilon \) such that for each \(\delta >0\) and each compact subset B of E it holds that

    $$\begin{aligned} P\left\{ \bigcup _{s\ge 0} \bigcap _{t\ge s} \varphi (t,\vartheta _{-t} \omega ) B \subseteq C_{\varepsilon }^{\delta } \right\} \ge 1 - \varepsilon . \end{aligned}$$
  3. (iii)

    There exists a compact strongly C-attracting set \(\omega \mapsto K(\omega )\).

The following example shows that the original formulations of Theorem 1 and Theorem 2 are incorrect.

FormalPara Example 1

Choose \(E={\mathbb {R}}\), \(\Omega =\{0\}\) and consider \(\varphi (t,\omega )x:= x+t\) for all \(t\ge 0, x\in E\), \(\omega \in \Omega \). This continuous RDS satisfies for all bounded subsets \(B\subset {\mathbb {R}}\)

$$\begin{aligned} \begin{aligned} \bigcup _{T\ge 0}\bigcap _{t\ge T} \varphi (t, \vartheta _{-t}\omega ) B&\subseteq \bigcap _{T\ge 0}\bigcup _{t\ge T} \varphi (t, \vartheta _{-t}\omega ) B \\&\subseteq \Omega _B(\omega ):= \bigcap _{T\ge 0}\overline{\bigcup _{t\ge T} \varphi (t, \vartheta _{-t}\omega ) B} = \emptyset . \end{aligned} \end{aligned}$$

This RDS has no C-attractor and hence also no B-attractor.

In particular, (i) and (ii) of Theorems 1 and 2 of the original formulation are not equivalent. This example shows in particular that also the following stronger property is not sufficient to ensure strong B-attractors:

  1. (ii)’

    For every \(\varepsilon >0\) there exists a compact subset \(C_\varepsilon \) such that for each \(\delta >0\) and each bounded and closed subset B of E it holds that

    $$\begin{aligned} P\left\{ \Omega _B(\omega ) \subseteq C^\delta _{\varepsilon } \right\} \ge 1 - \varepsilon . \end{aligned}$$

The following is a corrected version of Theorem 1: The condition (ii) is modified. In addition, condition (iii) is formulated more precisely than in the original formulation.

FormalPara Theorem 1

(Corrected formulation) The following are equivalent:

  1. (i)

    \(\varphi \) has a strong B-attractor.

  2. (ii)

    For every \(\varepsilon >0\) there exists a compact subset \(C_\varepsilon \) such that for each \(\delta >0\) and each bounded and closed subset B of E there exists a \(T>0\) such that

    $$\begin{aligned} P\left\{ \bigcup _{t\ge T} \varphi (t,\vartheta _{-t} \omega ) B \subseteq C_{\varepsilon }^{\delta } \right\} \ge 1 - \varepsilon . \end{aligned}$$
  3. (iii)

    There exists a random set \(K \subseteq E\times \Omega \) such that \(K(\omega )\) is P-a.s. compact and K attracts all bounded subsets, i.e.

    $$\begin{aligned} \lim _{t\rightarrow \infty } d(\varphi (t,\vartheta _{-t} \omega ) B, K(\omega )) = 0 \quad P\text {-a.s.} \end{aligned}$$

    for every bounded subset B.

FormalPara Remark 1

By [2, Lemma 3.5] and its proof we have that

$$\begin{aligned} \bigcup _{t\ge T} \varphi (t,\vartheta _{-t} \omega ) B \in {\mathcal {B}} \otimes {\bar{{\mathscr {F}}}} \quad \text {and} \quad \Omega _B(\omega ) \in {\mathcal {B}} \otimes {\bar{{\mathscr {F}}}} \end{aligned}$$

for all bounded closed subsets B of E. Here \({\mathcal {B}}\) denotes the Borel \(\sigma \)-algebra of E and \({\bar{{\mathscr {F}}}}\) the P-completion of \({\mathscr {F}}\). Therefore, we have by the measurable projection theorem that

$$\begin{aligned} \begin{aligned}&\Omega \backslash \left\{ \omega \in \Omega \,\,\bigg | \,\, \bigcup _{t\ge T} \varphi (t,\vartheta _{-t} \omega ) B \subseteq C_{\varepsilon }^\delta \right\} \\&\quad = \text {pr}_{\Omega }\left( \left\{ \bigcup _{t\ge T} \varphi (t,\vartheta _{-t} \omega ) B \right\} \cap \left\{ (E \backslash C^\delta _{\varepsilon })\times \Omega \right\} \right) \in {\bar{{\mathscr {F}}}} \end{aligned} \end{aligned}$$

where \(\text {pr}_{\Omega }: E \times \Omega \rightarrow \Omega \) denotes the projection onto \(\Omega \). Hence, the expression in (ii) of Theorem 1 is well-defined.

FormalPara Proof

The proof is similar to the proof presented in [1].

Equivalence of (i) and (iii) is proven in [3, Theorem 13], see also [4, Theorem 3.4, Remark 3.5].

We first show (i) \(\implies \) (ii): Let \(\varepsilon >0\) be arbitrary. Since E is a Polish space and the attractor A is a random variable taking values in the compact sets, there exists a compact subset \(C_{\varepsilon } \subseteq E\) such that

$$\begin{aligned} P\{A(\omega ) \subseteq C_{\varepsilon } \} \ge 1-\varepsilon /2 \end{aligned}$$
(1)

(see Crauel [5, Proposition 2.15]). Let \(B\subseteq E\) be a bounded and closed set. Then we have by (i)

$$\begin{aligned} \lim _{t\rightarrow \infty } d(\varphi (t,\vartheta _{-t} \omega ) B, A(\omega )) = 0 \quad P\text {-a.s.}, \end{aligned}$$

i.e. for every \(\delta >0\) there exists a \(T(\omega )>0\) such that for all \(t\ge T(\omega )\) we have \(d(\varphi (t,\vartheta _{-t} \omega ) B, A(\omega )) \le \delta \) P-almost surely. Hence, there exists some deterministic \(T>0\) such that

$$\begin{aligned} P\left\{ \bigcup _{t\ge T} \varphi (t,\vartheta _{-t} \omega ) B \subseteq A(\omega )^{\delta } \right\} \ge 1 - \varepsilon /2. \end{aligned}$$
(2)

Combining (1) and (2) implies (ii).

Now we show (ii) \(\implies \) (iii): Let \((B_k)_{k\in {\mathbb {N}}}\) be a sequence of bounded closed subsets of E such that \(B_0 \subseteq B_1 \subseteq B_2 \dots \) and such that for any bounded subset \(B\subseteq E\) there exists some \(k\in {\mathbb {N}}\) such that \(B\subseteq B_k\). We modify the random attractor constructed in the proof given in [1] to ensure that it is indeed a random set: We define \(A(\omega )\) to be the (unique) smallest closed random set that contains \(\bigcup _{k\in {\mathbb {N}}} \Omega _{B_k}(\omega )\), see [3, Proposition 17]. By (ii) for all \(\varepsilon >0\) there exists a compact set \(C_{\varepsilon } \subseteq E\) such that for every \(\delta >0\) and for every \(k\in {\mathbb {N}}\) there exist \( T(k)>0\) such that

$$\begin{aligned} P\left\{ \bigcup _{t\ge T(k)} \varphi (t,\vartheta _{-t} \omega ) B_k \subseteq C_{\varepsilon }^{\delta } \right\} \ge 1 - \varepsilon . \end{aligned}$$

Using that \(C_{\varepsilon }\) is closed, this implies that \(P\{\Omega _{B_k}(\omega ) \subseteq C_{\varepsilon }\} \ge 1-\varepsilon \). As \(\Omega _{B_k}(\omega ) \subseteq \Omega _{B_{k+1}}(\omega )\) this implies \(P\{\bigcup _{k\in {\mathbb {N}}} \Omega _{B_k}(\omega ) \subseteq C_{\varepsilon }\} \ge 1-\varepsilon \). This implies by the properties of \(A(\omega )\) given by [3, Proposition 17] that \(A(\omega )\) is a compact random set.

It remains to prove that \(A(\omega )\) attracts all bounded sets. To this end consider an arbitrary bounded subset B of E and let \(k\in {\mathbb {N}}\) be such that \(B\subseteq B_k\). Let \(\varepsilon >0\) be arbitrary. By (ii) there exists for every \(m\in {\mathbb {N}}\) some \(T_m>0\) such that

$$\begin{aligned} P\left\{ d\left( \bigcup _{t\ge T_m} \varphi (t, \vartheta _{-t} \omega ) B_k, C_{\varepsilon }\right) \le 1/m\right\} \ge 1-\varepsilon . \end{aligned}$$

which implies

$$\begin{aligned} P\left\{ \sup _{t\ge T_m} d(\varphi (t, \vartheta _{-t} \omega ) B_k, C_{\varepsilon })\le 1/m \text { for infinitely many m}\right\} \ge 1-\varepsilon . \end{aligned}$$

To obtain the previous inequality we used for \(M_m:= \{\sup _{t\ge T_m} d(\varphi (t, \vartheta _{-t} \omega ) B_k, C_{\varepsilon })\le 1/m \}\) that

$$\begin{aligned} P\left[ \bigcap _{n\in {\mathbb {N}}} \bigcup _{m=n}^\infty M_m \right] = \lim _{n\rightarrow \infty } P\left[ \bigcup _{m=n}^\infty M_m \right] \ge \limsup _{m\rightarrow \infty } P[M_m] \ge 1 - \varepsilon . \end{aligned}$$

Hence, we have

$$\begin{aligned} P\left\{ \lim _{t\rightarrow \infty } d(\varphi (t,\vartheta _{-t}\omega )B_k, C_\varepsilon )=0 \right\} \ge 1-\varepsilon . \end{aligned}$$

Due to \(\Omega _{B_k}\subseteq A\) and compactness of \(C_\varepsilon \) this implies

$$\begin{aligned} P[\lim _{t\rightarrow \infty } d(\varphi (t,\vartheta _{-t}\omega )B_k, A(\omega ))\ne 0]<\varepsilon . \end{aligned}$$

The assertion follows as the previous inequality holds for arbitrary \(\varepsilon >0\). \(\square \)

The following is a corrected version of Theorem 2. It follows from the proof given in [1] and the corrected proof of Theorem 1. (One can use e.g. [3, Lemma 8] to verify that \(\Omega _B\) is invariant.)

FormalPara Theorem 2

(Corrected formulation) The following are equivalent:

  1. (i)

    \(\varphi \) has a strong C-attractor.

  2. (ii)

    For every \(\varepsilon >0\) there exists a compact subset \(C_\varepsilon \) such that for each \(\delta >0\) and each compact subset B of E there exists a \(T>0\) such that

    $$\begin{aligned} P\left\{ \bigcup _{t\ge T} \varphi (t,\vartheta _{-t} \omega ) B \subset C_{\varepsilon }^{\delta } \right\} \ge 1 - \varepsilon . \end{aligned}$$
  3. (iii)

    There exists a random set \(K \subseteq E\times \Omega \) such that \(K(\omega )\) is P-a.s. compact and K attracts all compact subsets.